Two paricles of equal mass $m$ go round a circle of radius R under the action of their mutual gravitional attraction. The speed of each particle isSolution in Gujrati

A

$\frac{1}{\sqrt{3}} v_{0}$

B

$\frac{\sqrt{3}}{2} v_{0}$

C

$\frac{2}{\sqrt{3}} v_{0}$

D

$\frac{\sqrt{7}}{3} v_{0}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

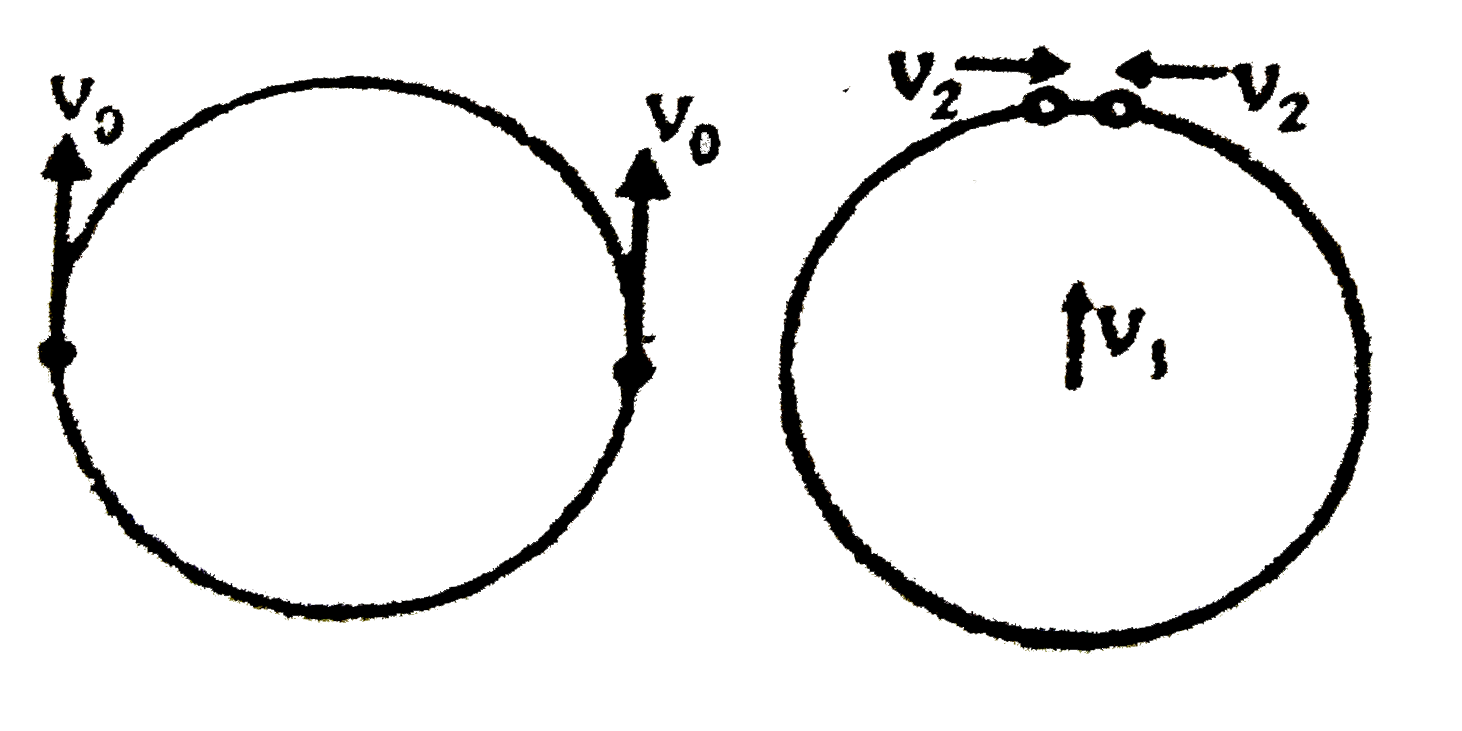
$C O L M \Rightarrow 2 m v_{0} = 3 m v_{1} \Rightarrow v_{1} = \frac{2}{3} v_{0}$
$C O M E \Rightarrow 2 \times \frac{1}{2} m v_{0}^{2} = 2 \times \frac{1}{2} m (v_{1}^{2} + v_{2}^{2}) + \frac{1}{2} m v_{1}^{2}$
$\Rightarrow 2 m v_{0}^{2} = 2 m {(\frac{2}{3} v_{0})}_{0}^{2} + 2 m v_{2}^{2} + m {(\frac{2}{3} v_{0})}_{0}^{2}$
$\Rightarrow 2 v_{2}^{2} = 2 v_{0}^{2} - 3 {(\frac{2}{3} v_{0})}_{0}^{2} \Rightarrow v_{2} = \frac{v_{0}}{\sqrt{3}}$
Velocity of particle $= \sqrt{v_{1}^{2} + v_{2}^{2}} = \sqrt{\frac{4 v_{0}^{2}}{9} + \frac{v_{0}^{2}}{3}} = \frac{\sqrt{7}}{3} v_{0}$

|

Updated on:21/07/2023

Class 11 PHYSICS CENTRE OF MASS

Topper's Solved these Questions

Similar Questions

ALLEN -CENTRE OF MASS-EXERCISE-I

Home
Ask Doubt
Profile