The given two fixed rings of radius R lie in XY plane as shown in the figure. Linear charge density of the rings A and B varies as per the relationλ=λ0sinθ and λ=λ0cosθ respectively where theta is measured from +x axis. Distance between the centre of the two rings is r>>R
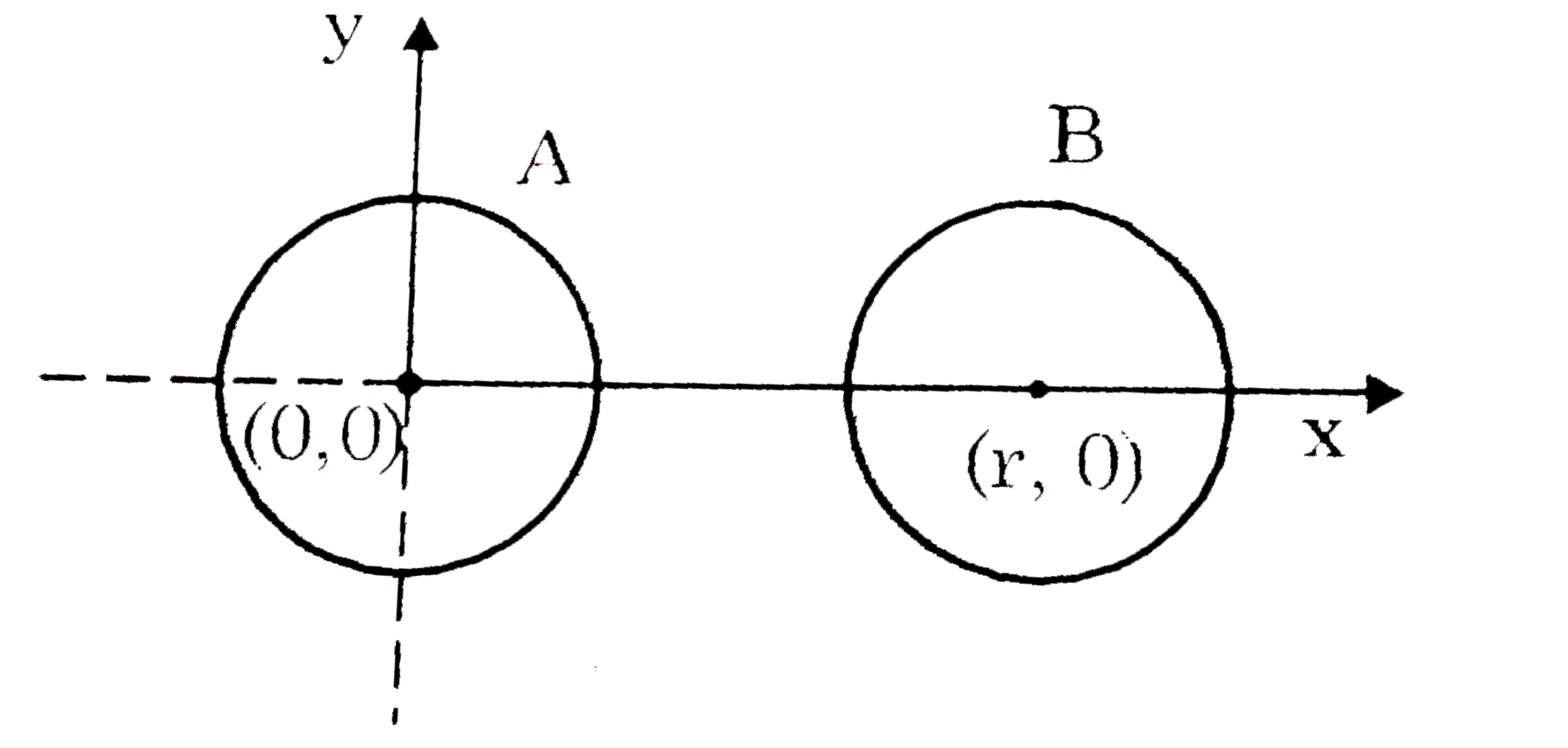Solution in Gujrati
Force of interaction between the two ring is34×λ20π∈0(Rr)4
Force of interection between the two rings is32×λ20π∈0(Rr)4
Potential energy of interaction between the two ring of πλ20R44ε0r3
Potential energy of interaction between the two ring is zero.
The correct Answer is:A, D
∫dp=∫dq(2Rsinθ)
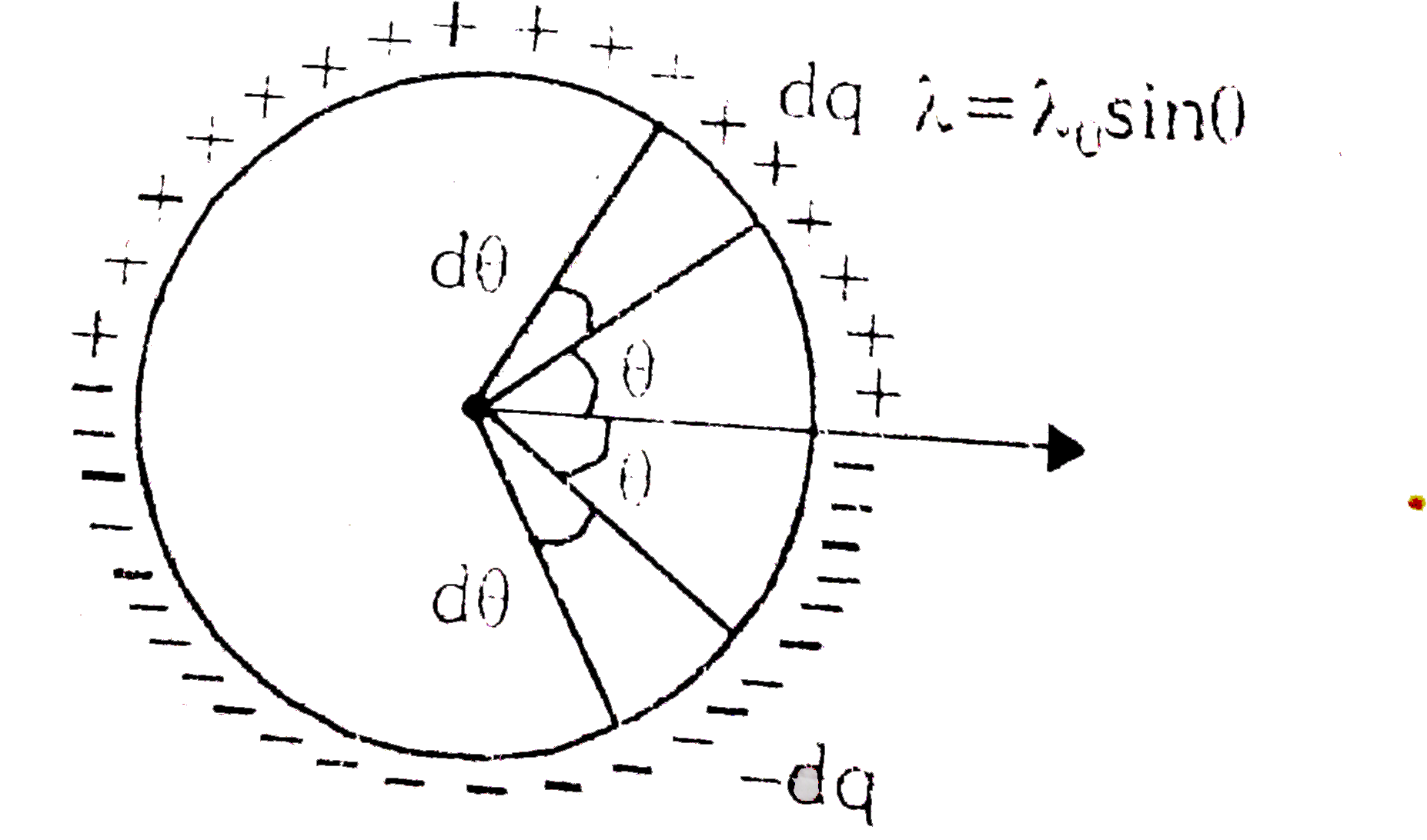
p=π∫0(λ0sinθ×Rdθ)(2Rsinθ)
p=πλ0R2 .....(i)
F=3kp2r4
=3λ20πR44ε0r4 .....(ii)
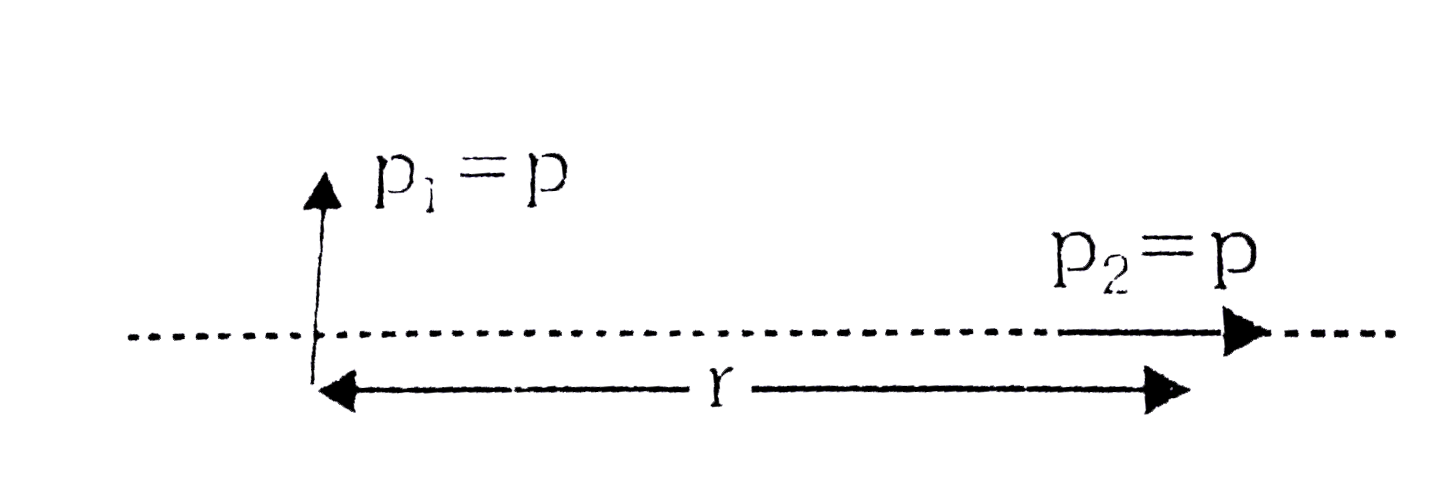
U=−P2E1cos90∘=0 ......(iii)
Topper's Solved these Questions
- TEST PAPERSBOOK - ALLEN CHAPTER - TEST PAPERSEXERCISE - part-2 physic
48 Videos
- TEST PAPERSBOOK - ALLEN CHAPTER - TEST PAPERSEXERCISE - PHYSICS
16 Videos
- TEST PAPERSBOOK - ALLEN CHAPTER - TEST PAPERSEXERCISE - Part-1 Physics
15 Videos
- TEST PAPER 4BOOK - ALLEN CHAPTER - TEST PAPER 4EXERCISE - PHYSICS
45 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLEN CHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (J-A)
7 Videos
Similar Questions
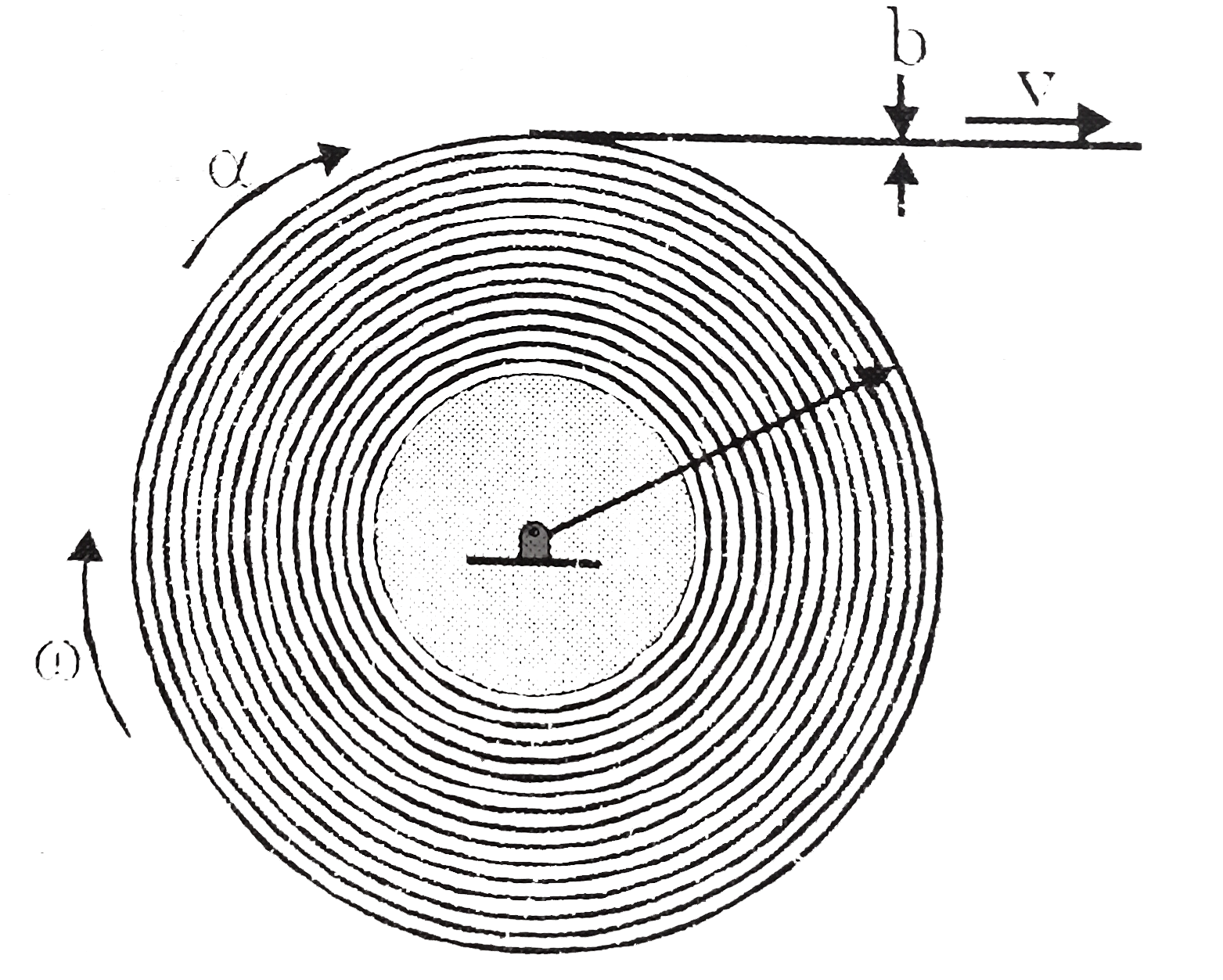
In a continuous printing process paper is drawn into the press at a constant speed v. Denoting by 'r' the radius of the paper roll at any given time and by 'b' the thickness of the paper. What is the angular acceleraion of the paper roll?
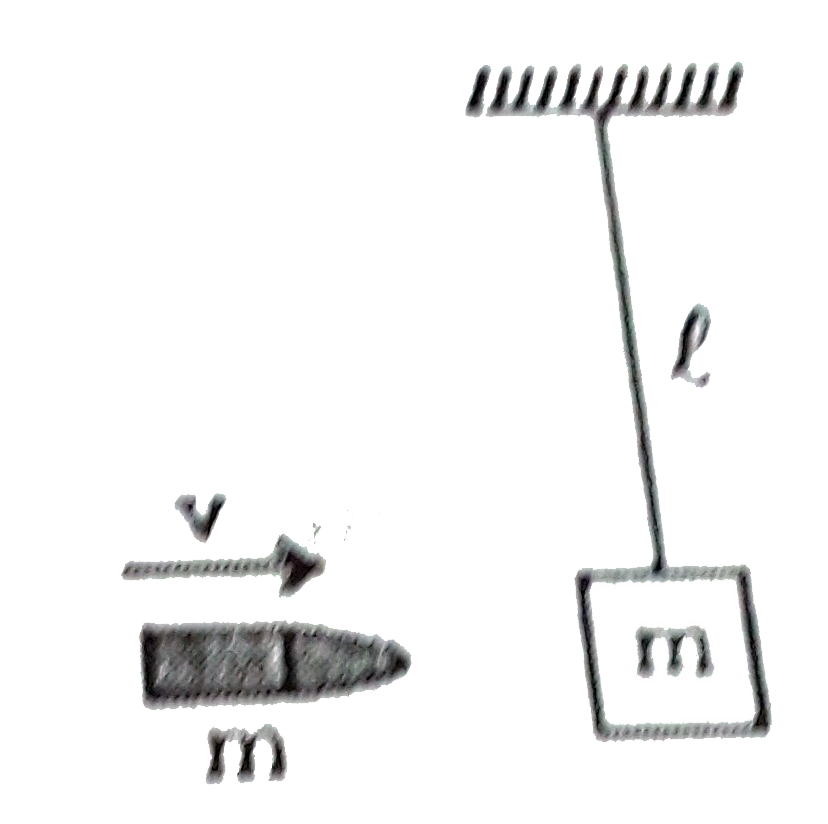
View SolutionA block of mass m is hanging from a light string of length l . A bullet of mass m strikes the hanging mass and gets embedded into it. The value of v such that tension in string and the speed of combined mass becomes zero simultaneously during the subsequent motion , is :-
View SolutionA quantity of 2 mole of helium gas undergoes a thermodynamic process, in which molar specific heat capacity of the gas depends on absolute temperatureT , according to relation:
C=3RT4T0
where T0 is initial temperature of gas. It is observed that when temperature is increased. volume of gas first decrease then increase. The total work done on the gas until it reaches minimum volume is :-
View SolutionTwo forces, each of magnitude F have a resultant of the same magnitude F. The angle between the two forces is
View SolutionMatch List I with List II and select the correct answer using the codes given below the lists :
View SolutionA wire having a uniform linear charge density λ is bent in the form of a ring of radius R. Point A as shown in (Fig. 3.145) is in the plane of the ring but not at the center. Two elements of the ring of lengths a1 and a2 subtend very small same angle at point A. they are at distances r1 and r2 from point A, respectively (r2>r1).
.
View SolutionA thin non-conducting ring of radius R has a linear charge density lambda=lambda_(0) cos theta , where theta is measured as shown. The total electric dipole moment of the charge distribution is
View SolutionA thin nonconducting ring of radius R has a linear charge density λ=λ0cosφ, where λ0 is a constant , ϕ is the azimutahl angle. Find the magnitude of the electric field strength
(a) at the centre of the ring ,
(b) on the axis of the ring as a function of the distance x from its centre. Investegation the obtained function at x>>R.
View SolutionA half ring of radius r has a linear charge density λ.The potential at the centre of the half ring is
View SolutionThe linear charge density of a uniform semicircular wire varies with theta shown in the figure as lambda=lambda_(0)costheta if R is the radius of the loop ,of the charge on the wire is
View SolutionTwo semicircular rings lying in same plane, of uniform linear charge density λ have radius r and 2r. They are joined using two straight uniformly charged wires of linear charge density λ and length r as shown in figure. The magnitude of electric field at common centre of semi circular rings is -
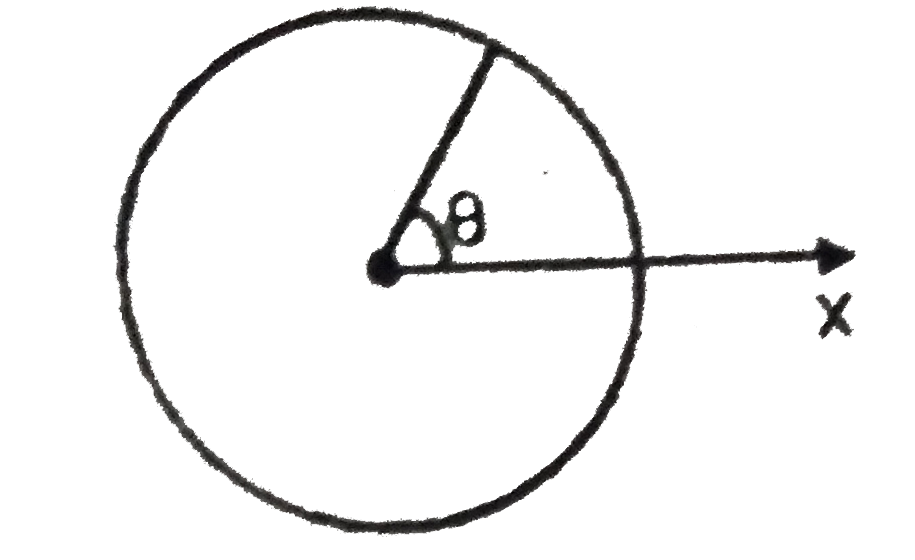
View SolutionThe linear charge density on a ring of radius R is λ=λ0sin(θ) where λ0 is a constant and θ is angle of radius vector of any point on the ring with x-axis. The electric potential at centre of ring is
View SolutionCharge on the ring is given as lambda=lambda_(0)cos theta C/m .Find dipole moment of the ring
View SolutionA ring of radius R carries a non - uniform charge of linear density λ=λ0cosθ sec in the figure) Magnitude of the net dipolement of the ring is :
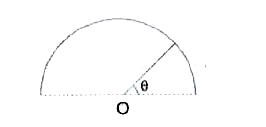
View SolutionFigure shows a semicircular ring of radius R with linear charge distribution λ given by λ=λ0sinθ. Find the electric field intensity at the centre of the ring.
View SolutionA thin ring of radius R is placed in the xy plane in such a way that its center remains at the base. x lt 0 On the half ring containing the field +lambda C//m Is the homogeneous linear charge density and the remaining half of the ring ( x gt 0 In area) -lambda C//m Is the homogeneous linear charge density.
View Solution