Particle of masses m,2m,3m,…,nm grams are placed on the same line at distance l,2l,3l,…..,nlcm from a fixed point. The distance of centre of mass of the particles from the fixed point in centimeters is :Solution in Gujrati
(2n+1)3
ln+1
n(n2+1)l2
2ln(n2+1)
The correct Answer is:A
Ans.(1)
Distance of centre of mass Xcm=∑m1x1∑m1
ml+2m(2l)+3m(3l)+.....+(nm)(nl)m+2m+3m+.....+nm
(l2+22+32+.....+(n2)1+2+3+.....+n)l=2n(n+1)(2n+1)6(n)(n+1)l
=(2n+1)3l
Topper's Solved these Questions
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
- TEST PAPER 1BOOK - ALLEN CHAPTER - TEST PAPER 1EXERCISE - PHYSICS
11 Videos
Similar Questions
Choose the correct statement:
View SolutionChoose the correct statement:
View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
View SolutionWhy are electric field lines perpendicular at a point on an equipotential surface of a conductor ?
View SolutionA particle of mass 1kg is placed at a distance of 4m from the centre and on the axis of a uniform ring mass 5kg and radius 3m. The work done to increase the distance of the particle from 4m to 3√3m is
View SolutionMass M is distributed uniformly along a line of length 2L. A particle of mass m is at a point that is at a distance a above the centre of the line on the its perpendicular bisector (Point P in figure). The gravitational force that the line exert on the particle is
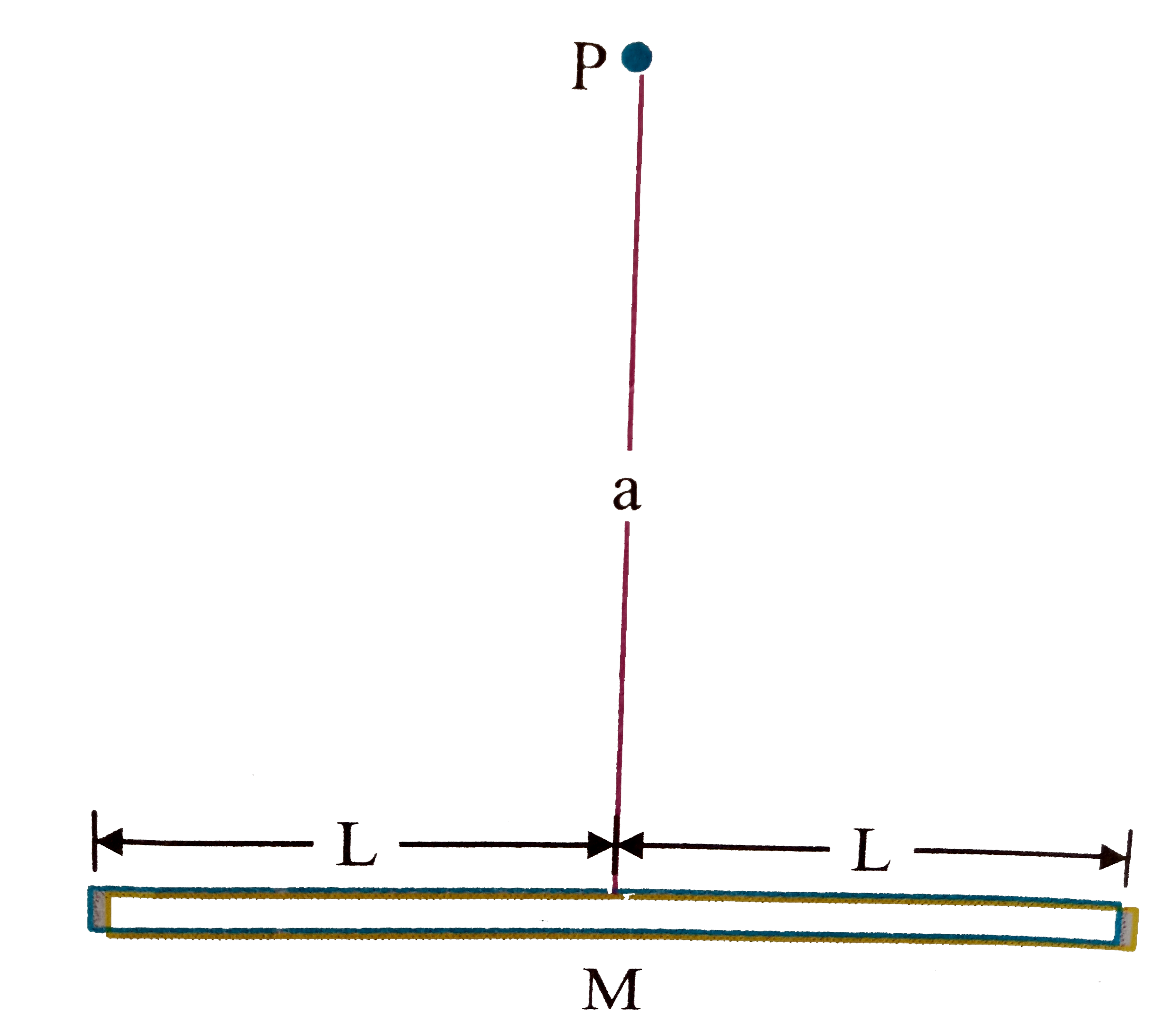
View SolutionChoose the wrong statements about the centre of mass (CM) of a system of two particles The CM lies on the line joining the two particles midway between them The CM lies on the line joining them at a point whose distance from each particle is proportional to the square of the mass of that particle The CM is on the line joining them at a point whose distance from each particle is proportional to the mass of that particl The CM lies on the line joining them at a point whose distance from each particle is inversely proportional to the mass of that particle
View SolutionA particle is projected from the mid-point of the line joining two fixed particles each of mass m. If the separation between the fixed particles is l, the minimum velocity of projection of the particle so as to escape is equal to
View SolutionA particle of mass M is placed at the centre of a spherical shell of same mass and radius a. What will be the magnitude of the gravitational potential at a point situated at a/2 distance from the centre ?
View SolutionA point particle of mass m is released from a distance sqrt(3)R along the axis of a fixed ring of mass M and radius R as shown in figure. Find the speed of particle as it passes through the centre of the ring.
View SolutionA particle of mass 100 gm and charge 2muC is released from a distance of 50 cm from a fixed charge of 5muC . Find the speed of the particle when its distance from the fixed charge becomes 3 m.
View SolutionA particle of mass 100 gm and charge 2muC is released from a distance of 50 cm from a fixed charge of 5muC . Find the speed of the particle when its distance from the fixed charge becomes 3 m.
View Solutionm, 2m, 3m, ......., nm ग्राम द्रव्यमान के कण एक स्थिर बिन्दु से एक रेखा में क्रमशः 1, 21, 31, ......., n/ सेमी दूरी पर रखे हैं, तब इस निकाय का द्रव्यमान केन्द्र (स्थिर बिन्दु से से.मी. में) कितनी दूरी पर होगा
View SolutionM,2m,3m………….nm ग्राम द्रव्यमान के कण एक स्थिर बिंदु से एक रेखा में क्रमशः l,2l,3l,……….nl सेमी दूरी पर रखे हैं तब इस निकाय का द्रव्यमान केंद्र (स्थिर बिंदु से सेमी में) कितनी दूरी पर होगा?
View SolutionA particle of mass M is placed at the centre of a spherical shell of same mass and radius a. What will be the magnitude of the gravitational potential at a point situated at a/2 distance from the centre ?
View SolutionFor a group of particles of different masses,if all the masses are placed at different points at equal distance R from the origin, then the distance of the centre of mass from the origin will be
View Solution
