Two springs of force constants k1 and k2, are connected to a mass m as shown. The frequency of oscillation of the mass is f. If both k1 and k2 are made four times their original values, the frequency of oscillation becomes
_E01_383_Q01.png) Solution in Gujrati
Solution in Gujrati
f/2
f/4
4f
2f
The correct Answer is:D
_E01_384_S01.png)
The orginal frequency of osillation
f=12π√k1+k2m
On increasing the k1 and k2 by 4 times, then f
f'=12π√(4k1k2m)=2f
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-05 [B]
12 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - MCQ s one or more than one correct answers
5 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-04 [B]
104 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
25 Videos
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
A particle of mass (m) is executing oscillations about the origin on the x axis. Its potential energy is V(x)=k|x|3 where k is a positive constant. If the amplitude of oscillation is a, then its time period (T) is.
View SolutionThree simple harmonic motions in the same direction having the same amplitude and same period are superposed. If each differ in phase from the next by 45∘, then
View SolutionIn the arrangement shown in figure, pulleys are light and spring are ideal. K1, k2, k3and k4 are force constant of the spring. Calculate period of small vertical oscillations of block of mass m.

View SolutionThe frequency of a radar is 780 MHz. After getting reflected from an approaching aeroplane, the apparent frequency is more than the actual frequency by 2.6 kHz. The aeroplane has a speed of
View SolutionChoose the correct statement:
View SolutionIn the arrangement shown in figure, pulleys are light and spring are ideal. K_(1) , k_(2) , k_(3) and k_(4) are force constant of the spring. Calculate period of small vertical oscillations of block of mass m .
View SolutionTwo springs are jonied and connected to a mass m as shown in figure. If the force constants of the two springs are k_(1) and k_(2) , shown that frequency of oscillation of mass m is
View SolutionAs shown in the figure, two light springs of force constant k1 and k2 oscillate a block of mass m. Its effective force constant will be
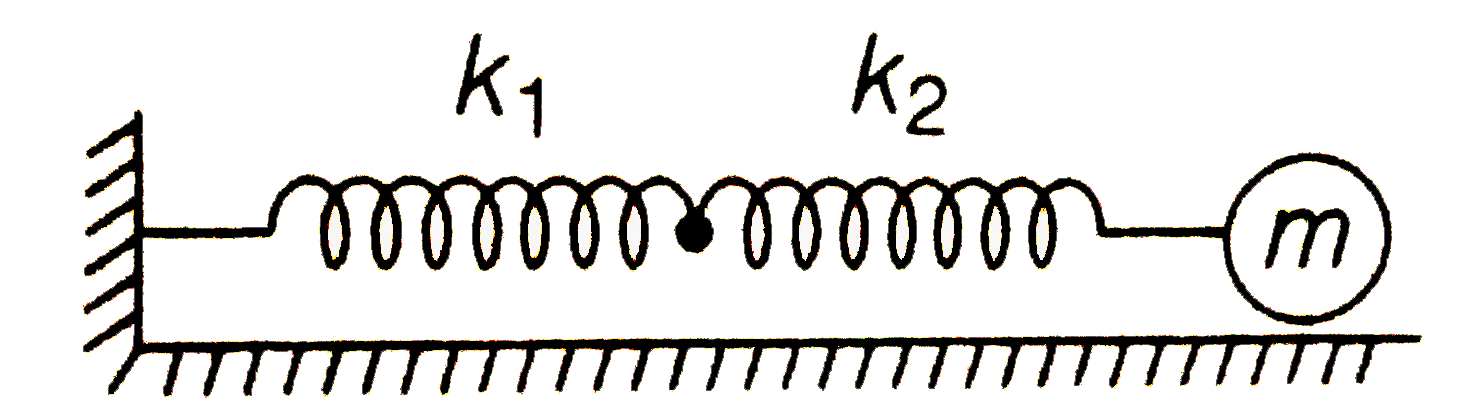
View SolutionTwo springs of force constants k_(1) and k_(2) , are connected to a mass m as shown. The frequency of oscillation of the mass is f . If both k_(1) and k_(2) are made four times their original values, the frequency of oscillation becomes
View SolutionTwo springs, of force constants k_(1) and k_(2) , are connected to a mass ma as shown. The frequency of oscillation of mass is f . If both k_(1) and k_(2) are made four times their original values, the frequency of oscillation becomes
View SolutionTwo springs of force constants K_(1) " and " K_(2) are connected to a mass m as shown I the figure. If both K_(1) " and " K_(2) are made four times their original values, the frequency of oscillation becomes,
View SolutionA block of mass m is connected between two springs (constants K1 and K2) as shown in the figure and is made to oscillate, the frequency of oscillation of the system shall be-
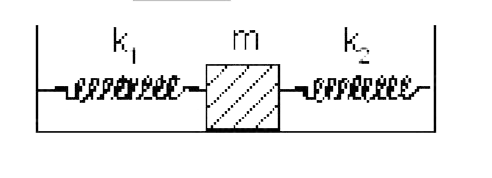
View Solutionबल नियतांक K1 व kK2 वाली दो स्प्रिंगें एक द्रव्यमान m से चित्र 15.56 के अनुसार बँधी हैं | द्रव्यमान की दोलन आवृत्ति है | यदि K1 व rK2 के मान प्रारम्भ के चार गुने कर दिए जाएँ तो दोलन आवृत्ति हो जाएगी :

View SolutionIf T1andT2 are the time of oscillations for two springs of constants k1andk2 connected to the mass m individually then calculate the time period of oscillations when both the spring are connected to the same mass and subjected to oscillations.
View SolutionA block of mass M is placed on a smooth table. Its two sides are attached to fixed walls by means of collinear horizontal springs of spring constants k_(1) and k_(2)(k_(1)gt k_(2)) as shown in the Figure. The block is made to oscillate horizontally along the line of the two springs. The frequency of its oscillation is :
View SolutionTwo spring of spring constants k_(1) and k_(2) ar joined and are connected to a mass m as shown in the figure. Calculate the frequency of oscillation of mass m.
View Solution
