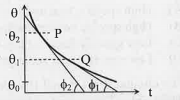
A body cools in a surrounding which is at a constant temperature of .Assume that it obeys Newton's law of cooling. Its temperature is plotted against time t. Tangents are drawn to the curve at the points and . These tangents meet the time axis at angles of and as shown
 Solution in Marathi
Solution in Marathi
Step by step video & image solution for A body cools in a surrounding which is at a constant temperature of theta_0.Assume that it obeys Newton's law of cooling. Its temperature theta is plotted against time t. Tangents are drawn to the curve at the points P(theta=theta_1) and Q(theta=theta_2). These tangents meet the time axis at angles of 0/_2 and 0/_1 as shown by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Wave motion is a propagation of disturbance through the medium in terms of __________
View SolutionAccording to Newton's law of cooling, the rate of cooling of a body is proportional to (Δθ)n, where Δθ is the difference of the temperature of the body and the surroundings, and n is equal to
View SolutionA liquid in a beaker has temperature θ(t) at time t and θ0 is temperature of surroundings, then according to Newton's law of cooling the correct graph between loge(θ−θ0) and t is :
View SolutionA body with an initial temperature θ1 is allowed to cool in a surrounding which is at a constant temperature of θ0(θ<θ1) Assume that Newton's law of cooling is obeyed Let k= constant The temperature of the body after time t is best experssed by .
View SolutionA body obeying Newton's law of cooling cools in a surrounding which is at a constant temperature Its temperature theta is plotted against time There are two points on the curve with temperatures theta_(2) and theta_(1)(theta_(2) gttheta_(1)) such that tangents on these points make angles of 2phi and half of its with time axis respectively Find the temperature of the surrounding .
View SolutionA body at temperature θ0 having Newton's cooling constant K is placed in a surrounding having temperature T0 at time t = 0. The graph of temperature of the body as a function of time t is shown in the adjacent figure. A tangent on the curve is drawn at t = 0 as shown in the figure. Find the value of τ.

View Solutionएक बीकर में एक द्रव का ताप समय t पर θ है तथा वातावरण का ताप θ0 है तब न्यूटन के शीतलन नियम के अनुसार loge(θ−θ0) तथा t के बीच सही ग्राफ है:
View SolutionIf the temperature of the body theta and time of cooling 't' and room temperature of the surrounding theta_(0) . The cooling curve is
View SolutionInstantaneous temperature difference between cooling body and the surroundings obeying Newton's law of cooling is theta . Which of the following represents the variation of In theta with time t?
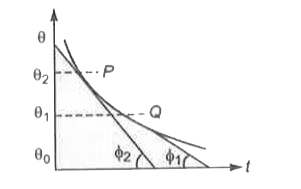
View Solutionएक पिण्डθ0 ताप वाले परिवेश में ठण्डा होता है। तथा यह न्यूटन के शीतलन नियम का पालन करता है। इसके ताप θ को समय t के सापेक्ष आरेखित किया जाता है। बिन्दु P(θ=θ2) तथा Q(θ=θ1) पर वक्र पर स्पर्शियां खींची गयी हैं तथा यह समय अक्ष को कोण ϕ2व ϕ1 पर मिलती हैं। तब
View SolutionA liquid in a beaker has temperature θ(t) at time t and θ0 is temperature of surroundings, then according to Newton's law of cooling the correct graph between loge(θ−θ0) and t is :
View SolutionThe temperature of water in calorimeter is θ and the temperature of surrounding is θc(<θ). Observation are recorded for temperature difference (θ−θ0) and time t. The cooling curve is best represented in graph.
View SolutionA body with an initial temperature θ, is allowed to cool in a surrounding which is at a constant temperature of θ0(θ0<θi). Assume that Newton.s law of cooling is obeyed. Let k = constant. The temperature of the body after time t is best expressed by
View SolutionAccording to Newton's law of cooling, the rate of cooling of a body is proportional to (Delta theta)^(n) , where Delta theta is difference of temperature of body and surroundings, and n is equal to
View SolutionA liquid in beaker has temperature θ(t) at time t and q0 is temperature of surroundings, then according to Newton's law of cooling, the correct graph between loge(θ−θ0) and t is
View SolutionInstantaneous temperature difference between cooling body and the surroundings obeying Newton's law of cooling is theta . Which of the following represents the variation of In theta with time t?
View Solution
