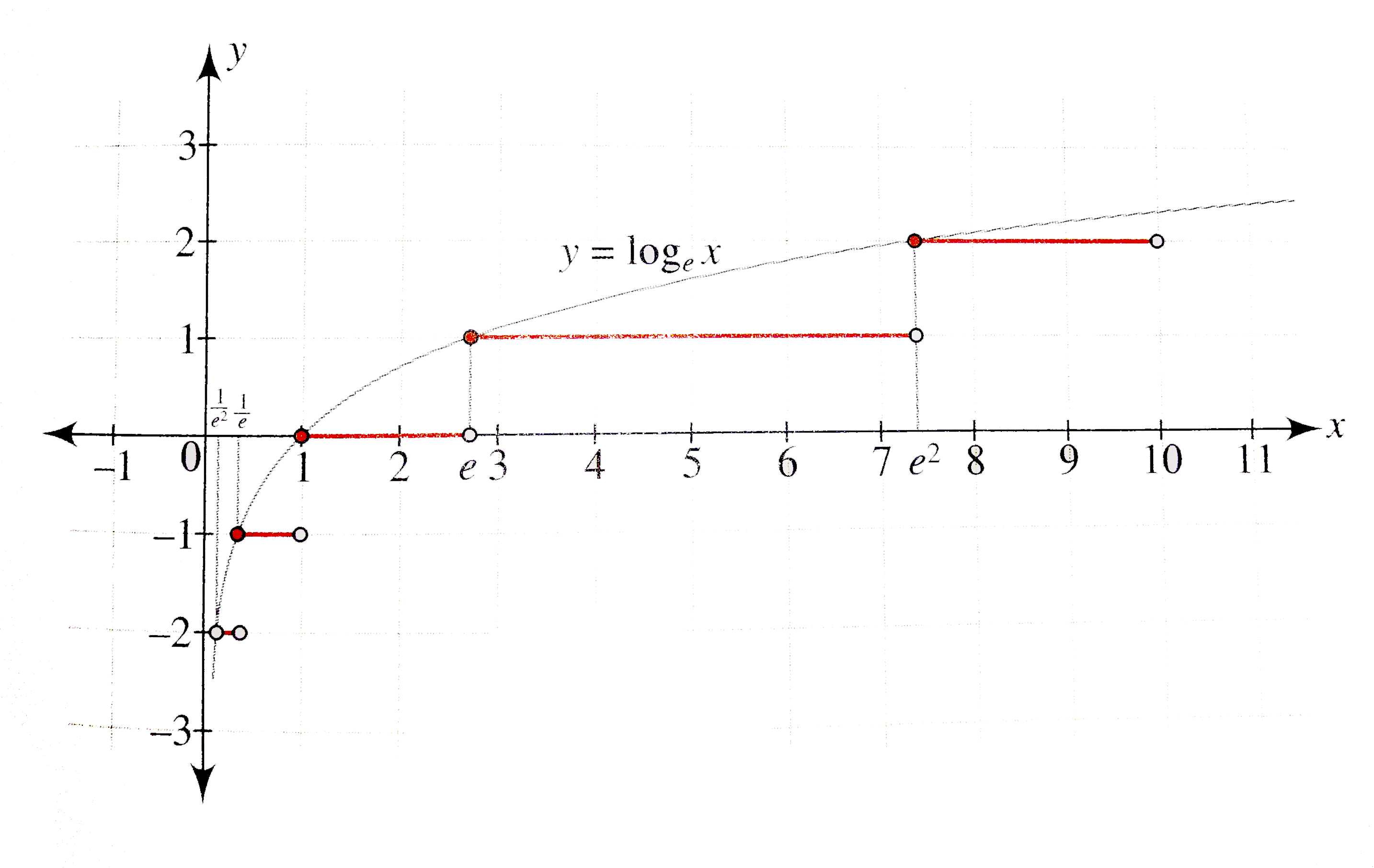
Draw the graph of f(x)=[logex],e−2<x<10, where [⋅] represents the greatest integer function. Solution in Bengali
We have f(x)=[logex],e−2<x<10 ltBrgt logex is an incresing function in its entire domain.
So [logex] is discontinuous when logex is an integer.
Now e−2<x<10
∴ −2<logex<10
So [logex] is discontinuous when logex=−1,0,1,2.
∴ x=e−1,1,e,e2
∴ f(x)=[logex]=⎧⎪
⎪
⎪
⎪
⎪
⎪
⎪
⎪
⎪⎨⎪
⎪
⎪
⎪
⎪
⎪
⎪
⎪
⎪⎩−2,x∈[e−2,e−1)orlogex∈[−2,−1)−1,x∈[e−1,1)orlogex∈[−1,0)0,x∈[1,e)orlogex∈[0,1)1,x∈[e,e2)orlogex∈[1,2)2,x∈[e2,10)orlogex∈[2,loge10)
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - EXERCISES34videos
GRAPHS OF ELEMENTARY FUNCTIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises17videos
GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercises22videos
GRAPHS OF TRIGONOMETRIC FUNCTIONS
Similar Questions
Draw the graph of y=1(1/x).
03:48View SolutionDraw the graph of y=(x−1)(x2−x+1).
View SolutionDraw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
02:23View SolutionDraw the graph of f(x)=[logex],e−2<x<10, where [⋅] represents the greatest integer function.
03:20View SolutionDraw the graph of the function f(x)=2x2−loge|x|
07:05View SolutionIf f(x)=x(e|x|+[x]−2|x|+[x]) then (where [.] represent the greatest integer function)
03:46View SolutionDraw the graph of the function, f(x)=logex(x>0).
04:30View SolutionDraw the graph of f(x)=(2^(x))/(2^([x])) where [.] represents greatest integer function and find the domain and range.
04:34View SolutionFind the domain of the function f(x)=log_(e)(x-[x]) , where [.] denotes the greatest integer function.
04:39View Solutionयदि f(x)=|logex|={−logexयदि0<x<1logexयदिx≥1 तो दिखाइए कि फलन x=1 पर अवकलनीय नहीं है।
05:15View SolutionDraw the graph of f(x)=cosπ[x], where [⋅] represents the greatest integer function. Find the period of the function.
03:54View SolutionDraw the graph of f(x)=[logex],e−2<x<10, where [⋅] represents the greatest integer function.
04:22View SolutionDraw the graph of the function f(x)=2x2−loge|x|
05:06View SolutionIf f(x)=x(e|x|+[x]−2|x|+[x]) then (where [.] represent the greatest integer function)
06:31View SolutionDraw the graph of f(x) = [log_(e)x], e^(-2) lt x lt 10 , where [*] represents the greatest integer function.
03:20View Solution

