Two circles intersect in A and B, and the measure of the common chord ¯¯¯¯¯¯AB = 10 cm. The line joining the centre cuts the circles in P and Q. If PQ = 3 cm, and the measure of the radius of one circle is 13 cm. Find the radius of the other circle.
294 cm
418 cm
203 cm
8 cm
To find the radius of the second circle, we can follow these steps:
Step 1: Understand the Geometry
We have two circles that intersect at points A and B. The common chord AB measures 10 cm. The line joining the centers of the circles intersects the circles at points P and Q, with PQ measuring 3 cm. The radius of the first circle (with center O) is given as 13 cm. We need to find the radius of the second circle (with center K).
Step 2: Find the Midpoint of the Chord
Let M be the midpoint of the chord AB. Since AB = 10 cm, we can find AM:
AM=AB2=10 cm2=5 cm
Hint: The midpoint of a line segment divides it into two equal parts.
Step 3: Use the Pythagorean Theorem
In triangle OAM, we can apply the Pythagorean theorem. Here, OA is the radius of the first circle (13 cm), and AM is 5 cm. We need to find OM:
OA2=OM2+AM2
132=OM2+52
169=OM2+25
OM2=169−25=144
OM=√144=12 cm
Hint: The Pythagorean theorem relates the lengths of the sides of a right triangle.
Step 4: Find MQ
The distance from O to Q (the center of the first circle to the point where the line PQ intersects the first circle) is the radius of the first circle minus OM:
OQ=13 cm(radius of the first circle)
MQ=OQ−OM=13 cm−12 cm=1 cm
Hint: To find a segment of a line, subtract the known length from the total length.
Step 5: Find PM
We know that PQ = 3 cm. Therefore, we can find PM:
PQ=PM+MQ
3 cm=PM+1 cm
PM=3 cm−1 cm=2 cm
Hint: When you have a total length and part of it, you can find the other part by subtraction.
Step 6: Set Up for the Second Circle
Let R be the radius of the second circle. In triangle AMK, we can express MK:
MK=RK−PM=R−2 cm
Hint: When dealing with segments, express unknown lengths in terms of known lengths.
Step 7: Apply the Pythagorean Theorem Again
Using the Pythagorean theorem in triangle AMK:
AM2+MK2=AK2
52+(R−2)2=R2
25+(R2−4R+4)=R2
25+4−4R=0
29−4R=0
4R=29
R=294 cm
Hint: When simplifying equations, combine like terms and isolate the variable.
Final Answer
The radius of the second circle is:
R=294 cm≈7.25 cm
We have two circles that intersect at points A and B. The common chord AB measures 10 cm. The line joining the centers of the circles intersects the circles at points P and Q, with PQ measuring 3 cm. The radius of the first circle (with center O) is given as 13 cm. We need to find the radius of the second circle (with center K).
Let M be the midpoint of the chord AB. Since AB = 10 cm, we can find AM:
AM=AB2=10 cm2=5 cm
In triangle OAM, we can apply the Pythagorean theorem. Here, OA is the radius of the first circle (13 cm), and AM is 5 cm. We need to find OM:
OA2=OM2+AM2
132=OM2+52
169=OM2+25
OM2=169−25=144
OM=√144=12 cm
The distance from O to Q (the center of the first circle to the point where the line PQ intersects the first circle) is the radius of the first circle minus OM:
OQ=13 cm(radius of the first circle)
MQ=OQ−OM=13 cm−12 cm=1 cm
We know that PQ = 3 cm. Therefore, we can find PM:
PQ=PM+MQ
3 cm=PM+1 cm
PM=3 cm−1 cm=2 cm
Let R be the radius of the second circle. In triangle AMK, we can express MK:
MK=RK−PM=R−2 cm
Using the Pythagorean theorem in triangle AMK:
AM2+MK2=AK2
52+(R−2)2=R2
25+(R2−4R+4)=R2
25+4−4R=0
29−4R=0
4R=29
R=294 cm
The radius of the second circle is:
R=294 cm≈7.25 cm
Topper's Solved these Questions
Similar Questions
The sum of circumferences of two circles is 132 cm. If the radius of one circles is 14 cm, find the radius of the other circle.
View Solution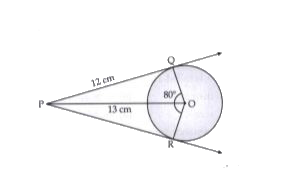
A point P is 13 cm from the centre of the circle. The two tangent PQ and PR are drawn from the point P, The length of the tangent drawn from P to the circle is 12 cm.
Find the radius of the circle.
View Solution
Knowledge Check
From a point Q, the length of the tangent to a circle is 12 cm and the distance of Q from the centre is 13 cm. The radius of the circle is 13 cm. The radius of the circle is :
A12 cmB13 cmC5 cmDNone of theseTwo circles touch each other externally. The distance between their centres is 7 cm. If the radius of one circle is 4 cm, then the radius of the other circle is
A3.5 cmB3 cmC4 cmD2 cmTwo circles touch each other externally. The distance between their centres is 7 cm. If the radius of one circle is 4 cm, then the radius of the other circle is
A3.5cmB3cm.C4cmD2cm
A point P is 13cm from the centre of the circle. The length of the tangent drawn from P to the circle is 12 cm. Find the radius of the circle.
View SolutionThe radius of a circle is 13 cm and the length of one of its chords is 10 cm. Find the distance of the chord from the centre.
View SolutionTwo concentric circles with radius 3 cm and 9.25 cm. Find the length of the chord of the bigger circle which is tangent to the other circle.
View SolutionOne chord of a circle is known to be 10.1 cm. The radius of this circle must be:
View SolutionThe radius of a circles is 13cm and the length of one of its chords is 10cm. Find the distance of the chord from the centre.
View Solution
