The displacement of a particle of mass 2kg moving in a straight line varies with times as x=(2t3+2)m. Impulse of the force acting on the particle over a time interval between t = 0 and t = 1 s is
10 N-s
12 N-s
8 N-s
6 N-s
To solve the problem, we need to calculate the impulse of the force acting on a particle of mass 2 kg, where the displacement x varies with time t as given by the equation x=2t3+2 meters. We will follow these steps:
Step 1: Identify the given values
- Mass of the particle, m=2 kg
- Displacement equation, x(t)=2t3+2 m
- Time interval, t1=0 s and t2=1 s
Step 2: Find the velocity of the particle
The velocity v is the derivative of displacement x with respect to time t:
v=dxdt=d(2t3+2)dt=6t2 m/s
Step 3: Find the acceleration of the particle
The acceleration a is the derivative of velocity v with respect to time t:
a=dvdt=d(6t2)dt=12t m/s2
Step 4: Calculate the force acting on the particle
Using Newton's second law, the force F can be calculated as:
F=m⋅a=2⋅(12t)=24t N
Step 5: Calculate the impulse of the force
Impulse J is defined as the integral of force over time:
J=∫t2t1Fdt=∫1024tdt
Calculating the integral:
J=24∫10tdt=24(t22)10=24⋅122=24⋅12=12 N⋅s
Final Answer
The impulse of the force acting on the particle over the time interval from t=0 s to t=1 s is 12 N·s.
---
- Mass of the particle, m=2 kg
- Displacement equation, x(t)=2t3+2 m
- Time interval, t1=0 s and t2=1 s
The velocity v is the derivative of displacement x with respect to time t:
v=dxdt=d(2t3+2)dt=6t2 m/s
The acceleration a is the derivative of velocity v with respect to time t:
a=dvdt=d(6t2)dt=12t m/s2
Using Newton's second law, the force F can be calculated as:
F=m⋅a=2⋅(12t)=24t N
Impulse J is defined as the integral of force over time:
J=∫t2t1Fdt=∫1024tdt
Calculating the integral:
J=24∫10tdt=24(t22)10=24⋅122=24⋅12=12 N⋅s
The impulse of the force acting on the particle over the time interval from t=0 s to t=1 s is 12 N·s.
Topper's Solved these Questions
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Only one option is correct for JEE Advanced
60 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - More a than one option is Correct
1 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Medical entrances gallery
27 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
Similar Questions
Kinetic energy of a particle moving in a straight line varies with time t as K=4t2. The force acting on the particle
View SolutionKinetic energy of a particle moving in a straight line is proportional to the time t. The magnitude of the force acting on the particle is :
View SolutionThe velocity-time graph of a particle moving in a straight line is shown in figure. The mass of the particle is 2kg. Work done by all the forces acting on the particle in time interval between t=0 to t=10s is

View SolutionForce acting on a particle of mass m moving in straight line varies with the velocity of the particle as F=K/V K is constant then speed of the particle in time t
View SolutionThe position (x) of a particle of mass 2 kg moving along x-axis at time t is given by x=(2t3) metre. Find the work done by force acting on it in time interval t=0 to t=2 is :-
View SolutionIf the displacement of a particle varies with time as √x=t+3
View SolutionDisplacement-time graph of a particle moving in a straight line is as shown in figure. Mass of the particle is 2 kg. The total impulse imparted to the particle in a time interval from t=0 to t=6 s in N-s will be
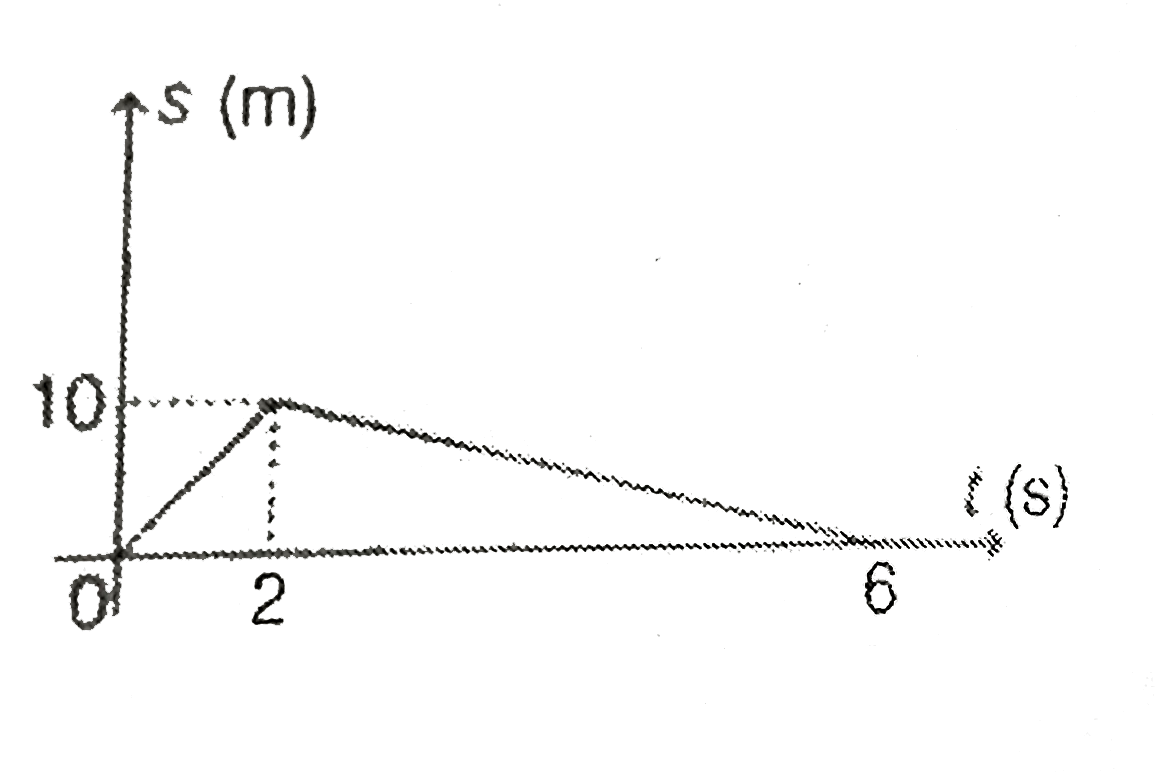
View SolutionDisplacement of a particle of mass 2 kg varies with time as s=(2t2−2t+10)m. Find total work done on the particle in a time interval from t=0 to t=2s.
View SolutionThe displacement of a body of mass 2kg varies with time t as S=t2+2t, where S is in seconds. The work done by all the forces acting on the body during the time interval t=2s to t=4s is
View SolutionThe coordinates of a particle moving in XY-plane vary with time as x=4t2,y=2t. The locus of the particle is a : -
View Solution
