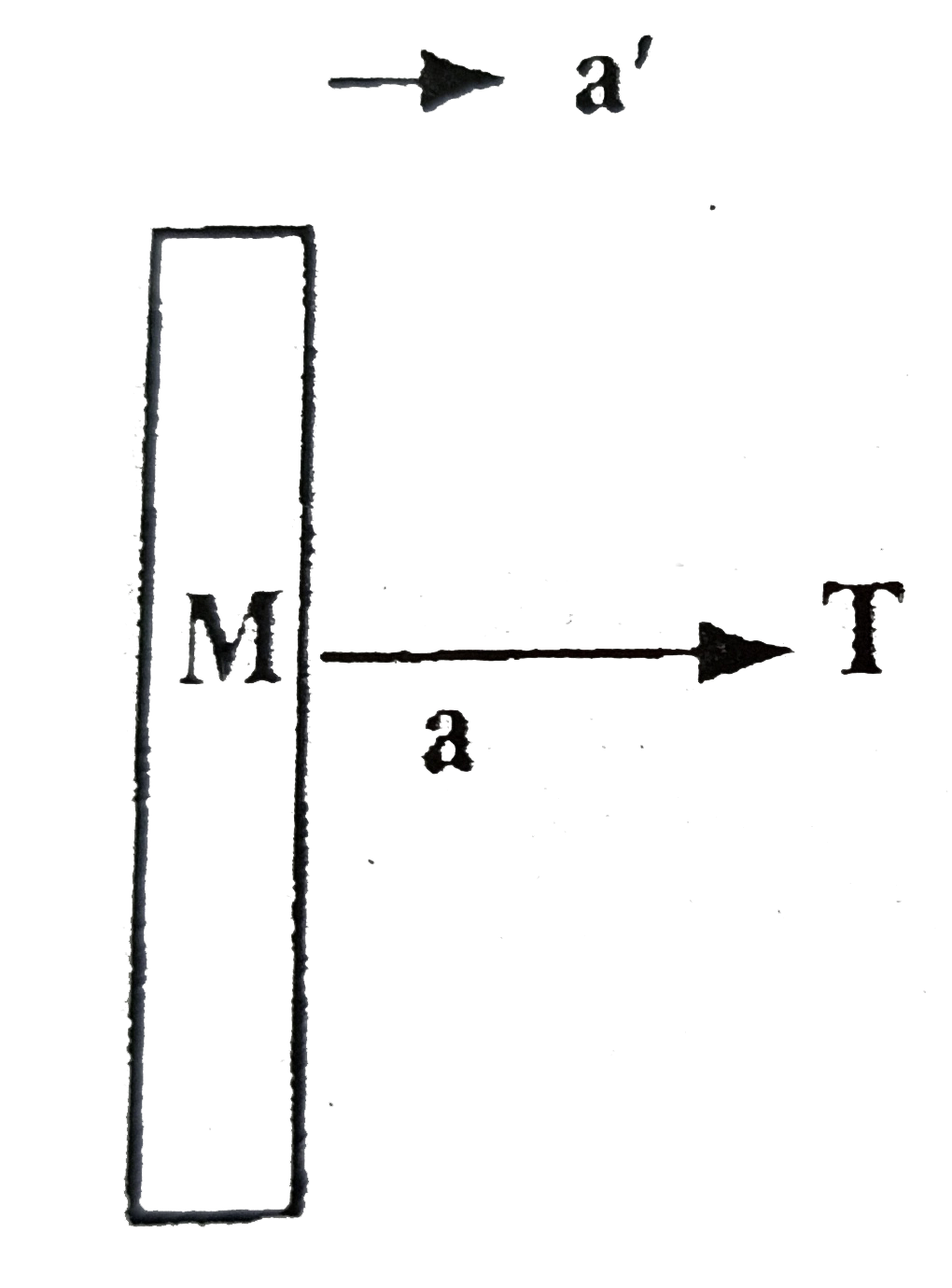
A spool of thread of mass m and moment of inertia I is attached to the block of mass M. Along a horizontal line passing through the centre of the spool, a constant force F is applied as shown. The surface under the block is smooth while beneath the spool is rough.
Show that if M=Ir(R−r), no frictional force will act on the spool. Now suppose M=2Ir(R−r), find in which direction will the frictional force act? Also find in this case the rate of increase of length 'ab' of the thread at time t after the application of force. (No slipping occurs.)

For translational motion
F−T−f=mac.m.……(1)
For rotational motion
(Tr+fR)=Iα……(2)
As per given condition if frictional force is zero then,
⇒α=ac.m.R
Hence, Tr+fR=Iac.m.R
⇒T=Iac.m.Rr−f.Rr....(3)
⇒T=Ma'
Since string ab remains tangent, hence acceleration of point a and that of point b is same.
⇒a'=ac.m.R(R−r)
⇒T=Mac.m.R(R−r)......(4)
From Eqs. (3) and (4) , we get
Mac.m.R(R−r)=Iac.m.R(R−r)=Iac.m.R.r−f.Rr.....(5)
Putting f=0 in Eq. (5)
M=Ir(R−r)
Putting the value of M=2Ir(R−r) in Eq.(5), we get
f=−Iac.m.R2.......(6)
Negative sign indicates that friction is acting in the opposite direction of our assumption i.e. in forward direction.
From Eqs. (1), (3) and (6), we get
F−2Iac.m.Rr+Iac.m.R2=mac.m.
⇒ac.m.=F(m+2IRr−IR2)⇒α=FR(m+2IRr+IR2)
∴T=ma'
d2(ab)dt2=α(R−r)−a'=F(R−r)R(m+2IRr−IR2)−2IcmmRr
=F(R−r)R(m+2IRr−IR2)−2ImRrF(m+2IRr−IR2)
=FR(m+2IRr−IR2)[R−r−2Imr]
∴ Rate of increase in length ab=(=vab)=FR(m+2IRr−IR2)[R−r−2Imr]

Topper's Solved these Questions
Similar Questions
A sphere of mass m and radius r is placed on a rough plank of mass M. The system is placed on a smooth horizontal surface. A constant force F is applied on the plank such that the sphere rolls purely on the plank. Find the acceleration of the sphere.

View SolutionA sphere of mass m and radius r is placed on a rough plank of mass M. The system is placed on a smooth horizontal surface. A constant force F is applied on the plank such that the sphere rolls purely on the plank. Find the acceleration of the sphere.

View Solution
Knowledge Check
A block of mass m is on the smooth horizontal surface of a plank of mass M The plank is on smooth horizontal surface Now, a constant horizontal force F acts on M. Now, for a person standing on the ground:
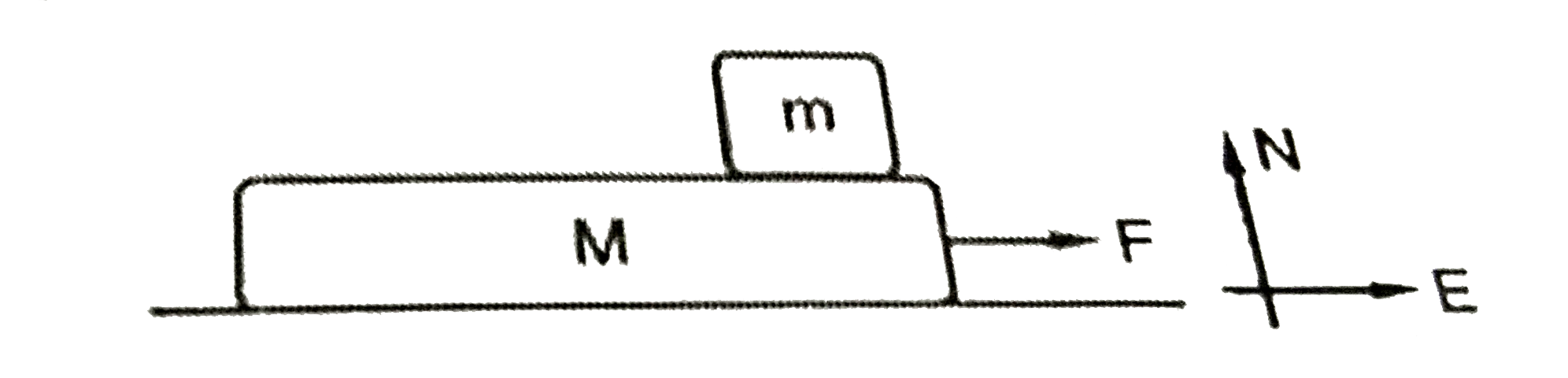 Athe acceleration of m is FM towards westBthe acceleration of m is zeroCthe acceleration of m is Fm towards eastDthe acceleration of m is FM+m towards east
Athe acceleration of m is FM towards westBthe acceleration of m is zeroCthe acceleration of m is Fm towards eastDthe acceleration of m is FM+m towards eastA block of mass m is revolving in a smooth horizontal plane with a constant speed v.If the radius of the circle path is R find the total contact force received by the block
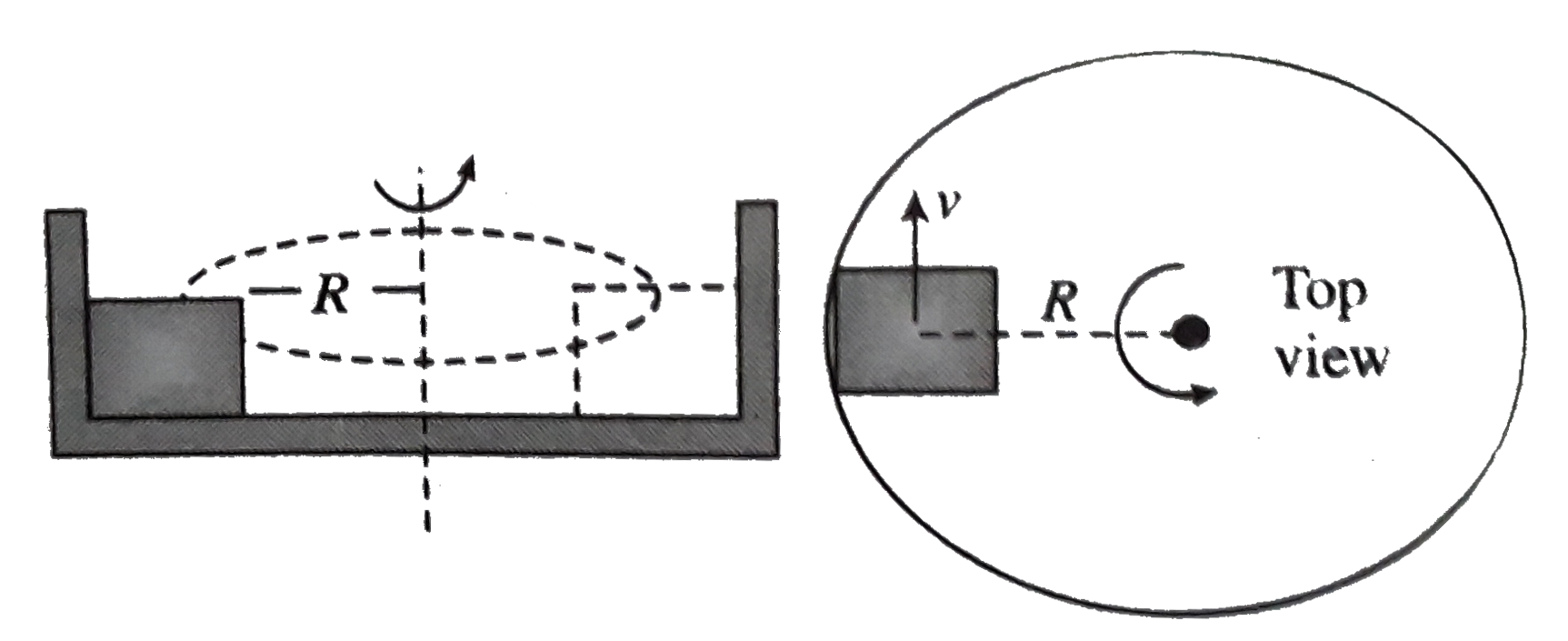 Amv2RBmgCm√v4R2+4g2Dm√v4R2+g2
Amv2RBmgCm√v4R2+4g2Dm√v4R2+g2A spool of mass m has moment of inertia I=2mR2 about its axis of symmetry. The inner and outer radius of spool R and 2R respectively. Thread is wounded on the inner cylinder and its one end is pulled a force F acting at angle θ with horizontal. The surface is sufficiently rough and th.e spool never slides on the surface.

Maximum frictional force acting on the spool is:AF2 towards rightB2F3C5F6DF6
A wheel of radius R, mass m and moment of inertia I is pulled along a horizontal surface by application of force F to as rope unwinding from the axel of radius, r as shown in figure. Friction is sufficient for pure rolling of the wheel.
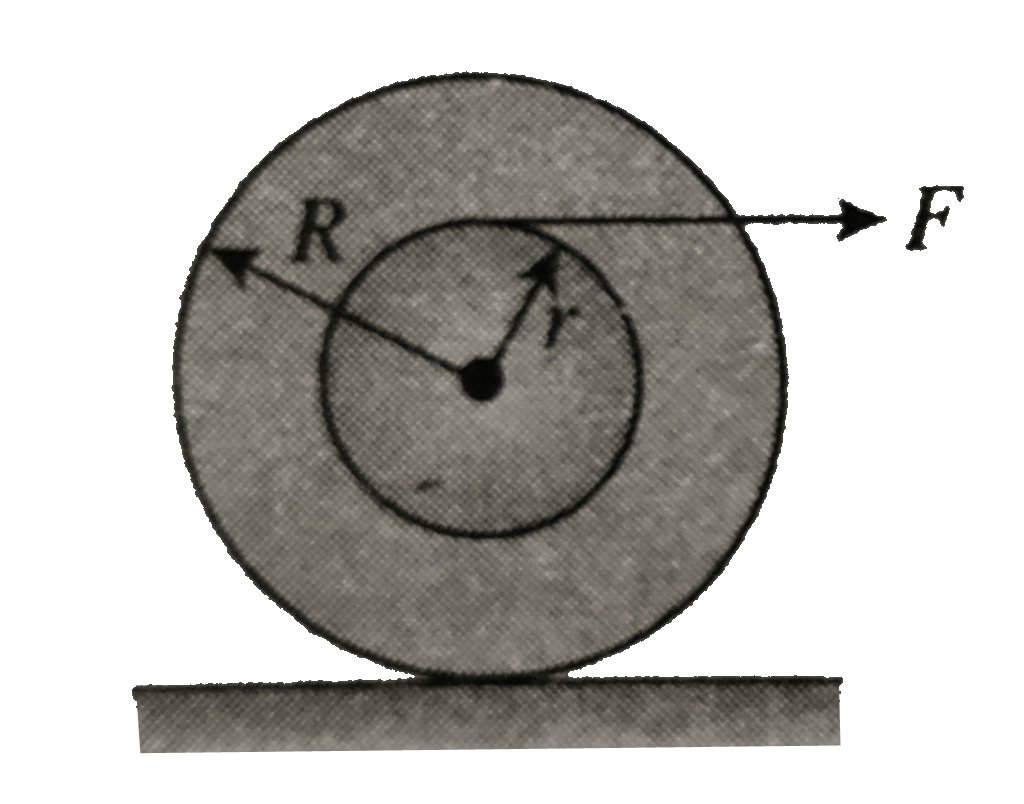
a. What is the linear acceleration of the wheel?
b. Calculate the frictional force that acts on the wheel.
View SolutionA wheel of radius R, mass m and moment of inertia I is pulled along a horizontal surface by application of force F to as rope unwinding from the axel of radius, r as shown in figure. Friction is sufficient for pure rolling of the wheel.

a. What is the linear acceleration of the wheel?
b. Calculate the frictional force that acts on the wheel.
View SolutionA block of mass m is revolving in a smooth horizontal plane with a constant speed v.If the radius of the circle path is R find the total contact force received by the block

View Solution
A spool is pulled at an angle θ with the horizontal on a rough horizontal surface as shown in the figure. If the spool remains at rest, the angle θ is equal to
View Solution
A spool is pulled at an angle θ with the horizontal on a rough horizontal surface as shown in the figure. If the spool remains at rest, the angle θ is equal to
View Solution
