A particle m starts with zero velocity along a line y=4d. The position of particle m varies as x=Asinωt, At ωt=π/2, its angular momentum with respect to the origin
mAωd
mωd/A
mAd/ω
zero
To solve the problem, we need to find the angular momentum of a particle m at the moment when ωt=π2. The particle's position varies as x=Asin(ωt), and it starts with zero velocity along the line y=4d.
1. Understand the Position Function:
The position of the particle is given by:
\(
x = A \sin(\omega t)
\)
At t=0, the particle starts with zero velocity, which means it is at rest initially.
2. Determine the Velocity:
To find the velocity of the particle, we differentiate the position function with respect to time t:
\(
v = \frac{dx}{dt} = A \cos(\omega t) \cdot \frac{d(\omega t)}{dt} = A \omega \cos(\omega t)
\)
3. Evaluate the Velocity at ωt=π2:
Substitute ωt=π2 into the velocity equation:
\(
v = A \omega \cos\left(\frac{\pi}{2}\right)
\)
Since \(\cos\left(\frac{\pi}{2}\right) = 0\), we find:
\(
v = A \omega \cdot 0 = 0
\)
Thus, the velocity of the particle at this moment is zero.
4. Calculate Angular Momentum:
The angular momentum \(L\) of a particle with respect to the origin is given by:
\(
L = r \times p
\)
where \(r\) is the position vector and \(p\) is the linear momentum. The linear momentum \(p\) is given by:
\(
p = mv
\)
Since we have found that \(v = 0\), we can conclude:
\(
p = m \cdot 0 = 0
\)
Therefore, the angular momentum is:
\(
L = r \times 0 = 0
\)
5. Final Result:
The angular momentum of the particle m with respect to the origin at ωt=π2 is:
\(
L = 0
\)
Summary:
The angular momentum of the particle at the specified time is zero.
The position of the particle is given by:
\(
x = A \sin(\omega t)
\)
At t=0, the particle starts with zero velocity, which means it is at rest initially.
To find the velocity of the particle, we differentiate the position function with respect to time t:
\(
v = \frac{dx}{dt} = A \cos(\omega t) \cdot \frac{d(\omega t)}{dt} = A \omega \cos(\omega t)
\)
Substitute ωt=π2 into the velocity equation:
\(
v = A \omega \cos\left(\frac{\pi}{2}\right)
\)
Since \(\cos\left(\frac{\pi}{2}\right) = 0\), we find:
\(
v = A \omega \cdot 0 = 0
\)
Thus, the velocity of the particle at this moment is zero.
The angular momentum \(L\) of a particle with respect to the origin is given by:
\(
L = r \times p
\)
where \(r\) is the position vector and \(p\) is the linear momentum. The linear momentum \(p\) is given by:
\(
p = mv
\)
Since we have found that \(v = 0\), we can conclude:
\(
p = m \cdot 0 = 0
\)
Therefore, the angular momentum is:
\(
L = r \times 0 = 0
\)
The angular momentum of the particle m with respect to the origin at ωt=π2 is:
\(
L = 0
\)
The angular momentum of the particle at the specified time is zero.
Topper's Solved these Questions
Similar Questions
A particle moves with a constant velocity parallel to the X-axis. Its angular momentum with respect to the origin
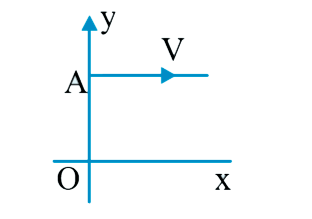
View SolutionA body of mass m is moving with a constant velocity along a line parallel to the x-axis, away from the origin. Calculate its angular momentum with respect to the origin?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A mass M moving with a constant velocity parallel to the X-axis. Its angular momentum with respect to the origin
Aa. is zeroBb. remains constantCc. goes on increasingDd. goes on decreasing
A mass m is moving with a constant velocity along a line parallel to the x-axis, away from the origin. Its angular momentum with respect to the origin.
View SolutionThe time dependence of the position of a particle of mass m = 2 is given by →r(t)=2tˆi−3t2ˆj Its angular momentum, with respect to the origin, at time t = 2 is :
View SolutionThe time dependence of the position of a particle of mass m = 2 is given by →r(t)=2tˆi−3t2ˆj Its angular momentum, with respect to the origin, at time t = 2 is :
View SolutionA particle of mass m moves in the XY plane with constant velocity v along the straight line PQ as shown in the figure. Its angular momentum with respect to origin O is(θ is angle between→v and x-axis)
 View Solution
View SolutionA xandy co-ordinates of a particle are x=Asin(ωt)andy=Asin(ωt+π/2). Then, the motion of the particle is
 View Solution
View SolutionA particle mass 1 kg is moving along a straight line y=x+4. Both x and y are in metres. Velocity of the particle is 2m/s. Find the magnitude of angular momentum of the particle about origin.
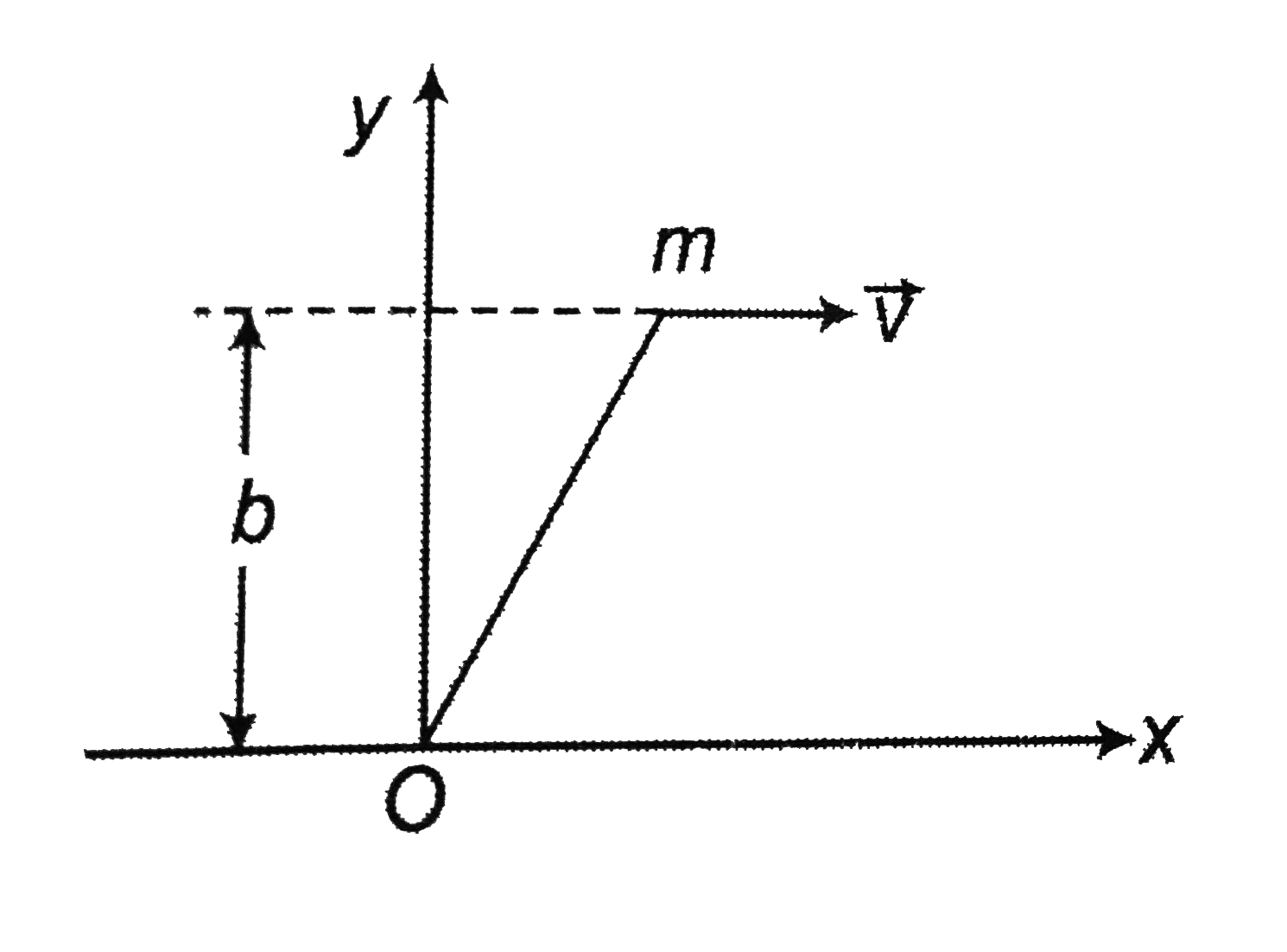
View SolutionIf the particle of mass m is moving with constant velocity v parallel to x-axis in x-y plane as shown in (figure), Find its angular momentum with respect of origin at any time t.
 View Solution
View Solution

