A body of mass m and radius r is released from rest along a smooth inclined plane of angle of inclination θ. The angular momentum of the body about the instantanoues point contact after a time t from the instant of release of equal is :
mgrtcosθ
mgrtsinθ
(3/2)mgrtsinθ
None of these
To solve the problem of finding the angular momentum of a body of mass m and radius r released from rest along a smooth inclined plane at an angle θ, we can follow these steps:
1. Understanding the Problem:
- A body of mass m and radius r is sliding down a smooth inclined plane (frictionless) at an angle θ.
- We need to find the angular momentum of the body about the instantaneous point of contact after a time t.
2. Identify Forces Acting on the Body:
- The weight of the body acts downwards with a force of mg.
- This weight can be resolved into two components:
- Perpendicular to the incline: mgcos(θ)
- Parallel to the incline: mgsin(θ)
3. Determine the Acceleration:
- Since the incline is smooth (frictionless), the only force causing the body to accelerate down the incline is the component of the weight acting parallel to the incline.
- The acceleration a of the body down the incline can be given by:
\(
a = g \sin(\theta)
\)
4. Calculate the Velocity After Time t:
- The body starts from rest, so the initial velocity u=0.
- Using the equation of motion:
\(
v = u + at = 0 + (g \sin(\theta)) t = g \sin(\theta) t
\)
5. Calculate the Angular Momentum:
- The angular momentum L about the instantaneous point of contact is given by the formula:
\(
L = mvr
\)
- Substituting the expression for v:
\(
L = m (g \sin(\theta) t) r
\)
- Simplifying this gives:
\(
L = mg r \sin(\theta) t
\)
6. Final Result:
- The angular momentum of the body about the instantaneous point of contact after time t is:
\(
L = mg r \sin(\theta) t
\)
- A body of mass m and radius r is sliding down a smooth inclined plane (frictionless) at an angle θ.
- We need to find the angular momentum of the body about the instantaneous point of contact after a time t.
- The weight of the body acts downwards with a force of mg.
- This weight can be resolved into two components:
- Perpendicular to the incline: mgcos(θ)
- Parallel to the incline: mgsin(θ)
- Since the incline is smooth (frictionless), the only force causing the body to accelerate down the incline is the component of the weight acting parallel to the incline.
- The acceleration a of the body down the incline can be given by:
\(
a = g \sin(\theta)
\)
- The body starts from rest, so the initial velocity u=0.
- Using the equation of motion:
\(
v = u + at = 0 + (g \sin(\theta)) t = g \sin(\theta) t
\)
- The angular momentum L about the instantaneous point of contact is given by the formula:
\(
L = mvr
\)
- Substituting the expression for v:
\(
L = m (g \sin(\theta) t) r
\)
- Simplifying this gives:
\(
L = mg r \sin(\theta) t
\)
- The angular momentum of the body about the instantaneous point of contact after time t is:
\(
L = mg r \sin(\theta) t
\)
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
An object of mass m is released from rest from the top of a smooth inclined plane of height h. Its speed at the bottom of the plane is proportional to
Am0BmCm2Dm−1
A spherical object of mass m and radius R is released from the top of a smooth inclined plane of height h and angle of inclination is 37° . The radius of gyration of the object is K. The speed of object at the bottom of inclined plane is
View SolutionA block is released from rest from point on a rough inclined place of inclination 37∘. The coefficient of friction is 0.5.
View SolutionA body of mass m is kept on a rough fixed inclined plane of angle of inclination θ=30∘. It remains stationary. Then magnitude of force acting on the body by the inclined plane is equal to :
View SolutionA body of mass m is projected with a velocity u at an angle θ with the horizontal. The angular momentum of the body, about the point of projection, when it at highest point on its trajectory is
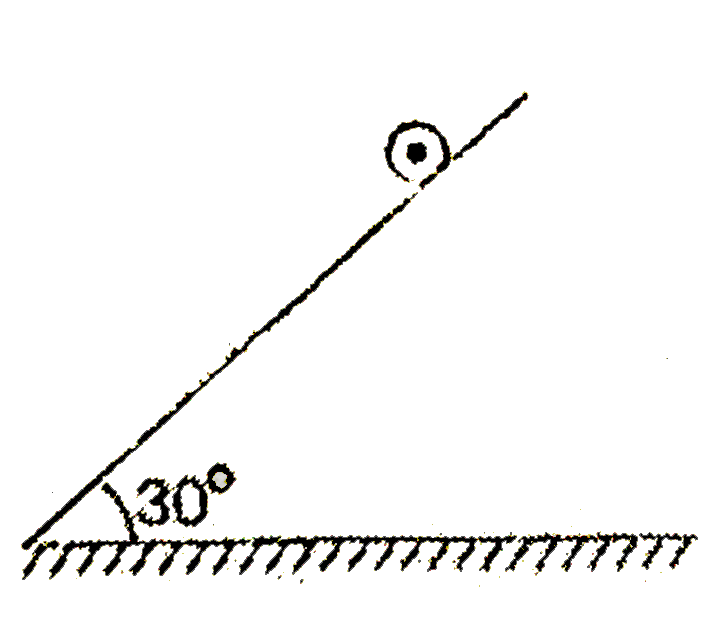
View SolutionAn uniform spherical shell of mass m and radius R starts from rest with pure rolling on long inclined plane as shown in figure. The angular momentum of shell about point of contact after 1 s of its starting is KmR. Determine the value of k(g=10m/s2).
 View Solution
View Solution
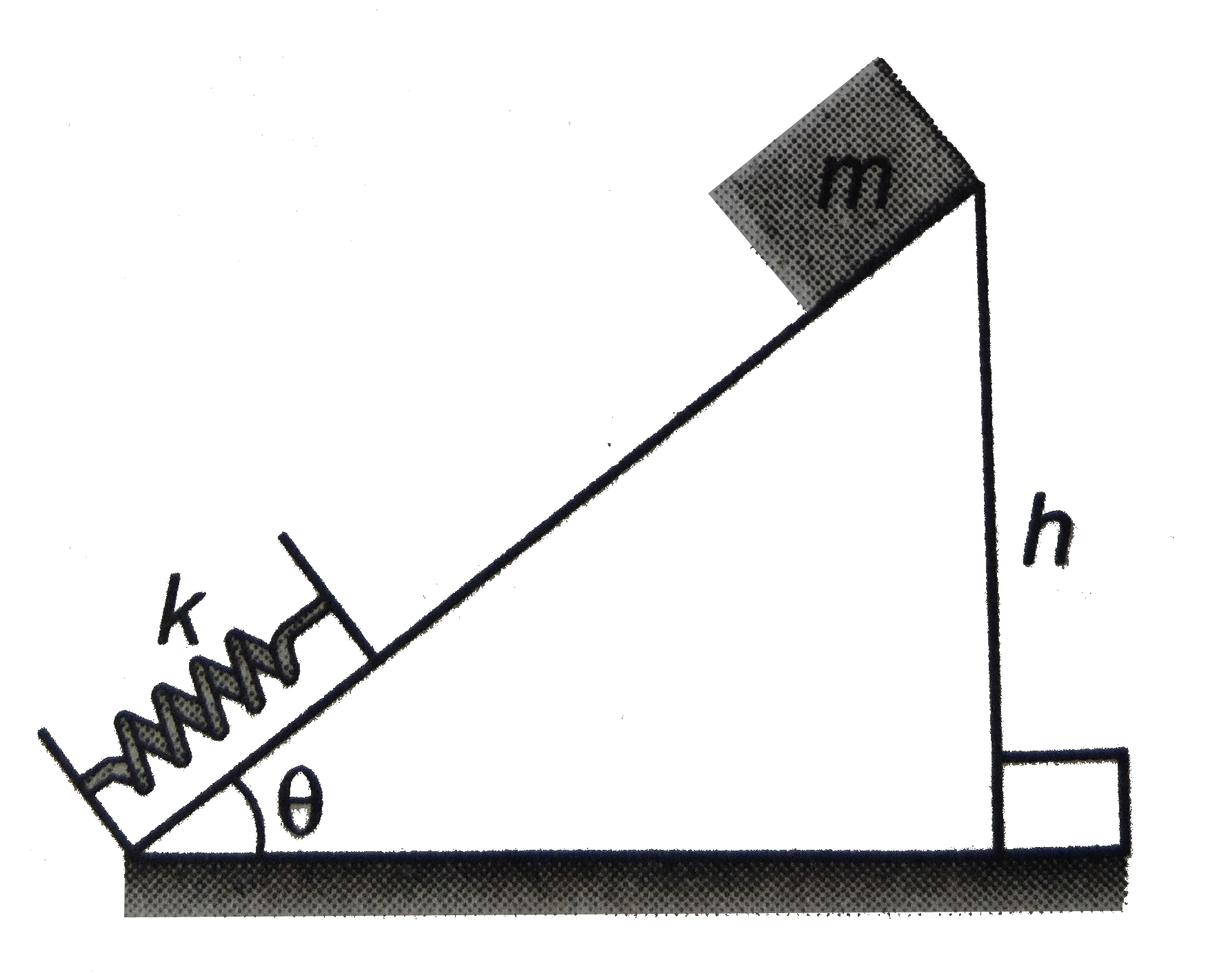
A uniform cylinder of mass M and radius R is released from rest on a rough inclined surface of inclination θ with the horizontal as shown in figure. As the cylinder rolls down the inclined surface, the maximum elongation it the spring stiffness k isView SolutionA body of mass m is released from a height h on a smooth inclined plane that is shown in the figure. The following can be true about the velocity of the block knowing that the wedge is fixed.
 .View Solution
.View Solution