A table with smooth horiztonal surface is truning at an angular speed ω abouyt its axis. A groove is made on the surface along a radius and a particle is gently placed inside the groove at a distance a from the centre. Find the speed of the speed of the particle as its distance from teh centre becomes L.Solution in Tamil
The situation is hwon in ure.
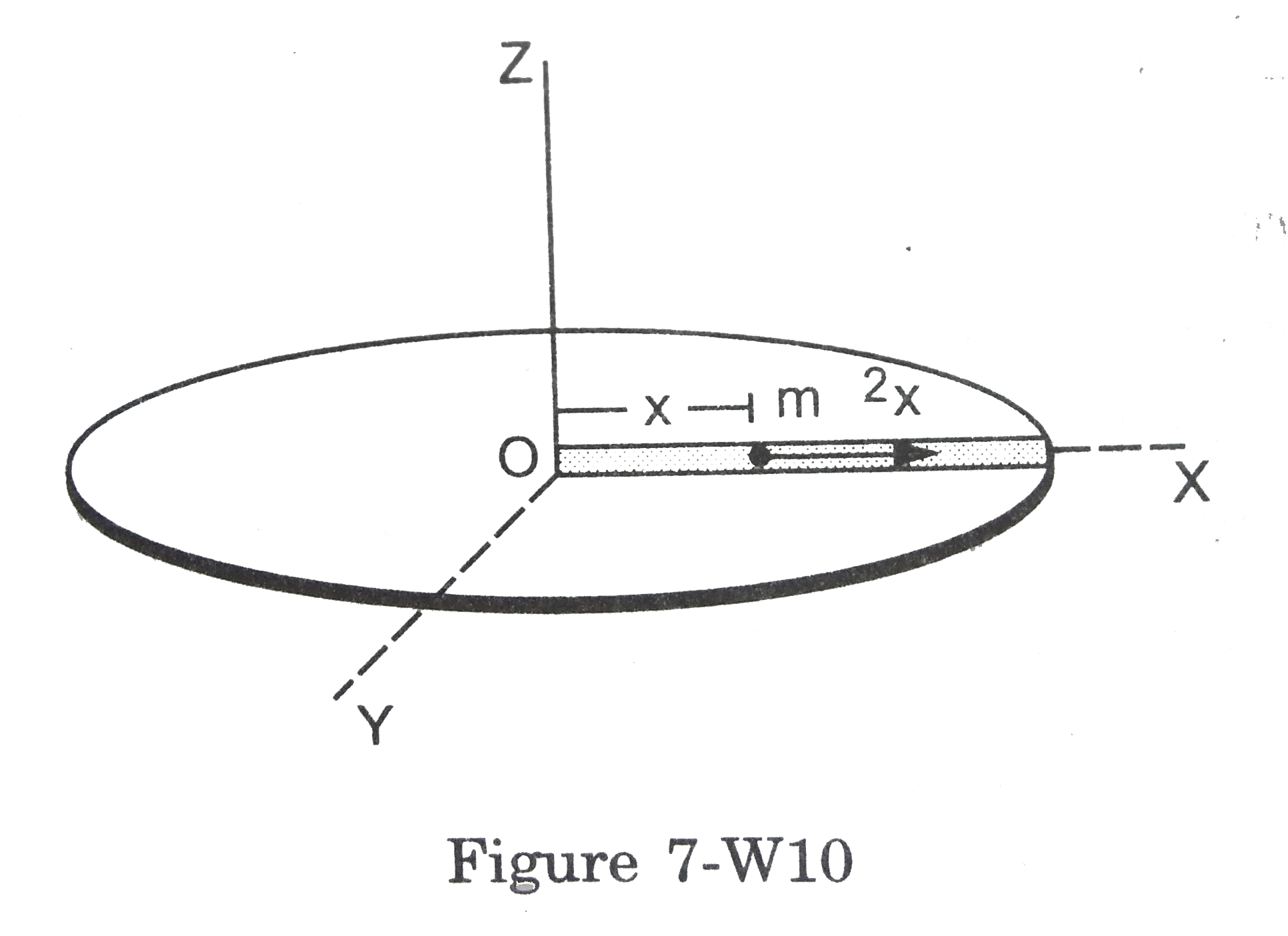
Let us work from the frame of reference of the table. Let us take the origin at the centre of rottion O an the X-axis along the groove ure. The Y-axis is along the lineperpendicular to OX coplaN/Ar with the surface of the table and the Z-axis is along the vertical.
Suppose at time t particle in the groove is at a distance x fromn the origin and is moving along The X-axis witha speed v. The forces acting on the particle (including the pseudeo forces that we must assume because we have taken our frame on the table which is rotating and is nonintertial) are
a. weight mg vetically downward
b. normal contact force N1 verticall upward by te bottom surfce of the groove,
c. normal contact force N2 parallel to the Y-axis by the side walls of the groove,
d. centrifugal force mω2x alongthe X-axis, and
e. Coriolis force along Y-axis (coriolis force is perpendicular to the velocity of the particle and the axis of rotation).
As the particle can only move in the groove. Its acceleration is along the X-axis. The only force along teh X-axis is the centrifugal force mω2x. all the other forces are perpendicular to the X-axis adn have no components along the X-axis.
Thus, the acceleration along the X-axis is
a=Fm=mω2xm=ω2x
or, dvdt=ω2x
or dvdx.dxdt=ω2x
or dvdx.v=ω2x
or, vdv=ω2xdx
or, ∫v_0vdv=∫L_aω2xdx
or, [12v2]v0=[12ω2x2]L_a
or, v22=12ω2(L2−a2)
or, v=omega sqrt(L^2-a^2)
Topper's Solved these Questions
- BOOK - HC VERMACHAPTER - CIRCULAR MOTIONEXERCISE - Question for short Answer10videos
CIRCULAR MOTION
- BOOK - HC VERMACHAPTER - CIRCULAR MOTIONEXERCISE - Objective -116videos
CIRCULAR MOTION
- BOOK - HC VERMACHAPTER - CIRCULAR MOTIONEXERCISE - Exercises30videos
CIRCULAR MOTION
- BOOK - HC VERMACHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Exercises64videos
CENTRE OF MASS, LINEAR MOMENTUM, COLLISION
- BOOK - HC VERMACHAPTER - FLUID MECHANICSEXERCISE - All Questions96videos
FLUID MECHANICS
Similar Questions
You are driving a motorcycle ona horizontal raod. It is moving with a uniform velocity. Is it possibel to accelerate the motorcycle wilthout putting higher petrol input rate into the engine?
03:20View SolutionWhen a particle moves in a circle with a uniform speed
04:31View SolutionFind the accelerationof the moon with respect to the eath from the following data, Distance between the earth and the moon =3.85×105 km and the time taken by the moon to complete one revolution around the earth =27.3days
04:01View SolutionThree particles of masses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre of mass of the system.
01:45View SolutionWater is filled in a flask up to a height of 20 cm. The bottom of the flask is circular with radius 10 cm. If the atmospheric pressure is 1.01×105 Pa, find the force exerted by the water on the bottom. Take g=10ms−2 and density of water =1000 kgm^-3.
06:24View SolutionA table with smooth horizontal surface is fixed in a cabin that rotates with a uniform angular velocity ω in a circular pathof radius R. A smooth groove AB of length L(<<R) is made on the surface of the table. The groove makes an angleθ with the radius OA of the circle in which the cabin rotates. A small particle is kept at the point A in the groove and is released to move along AB. Find the time taken by tehparticle to reach the point B.
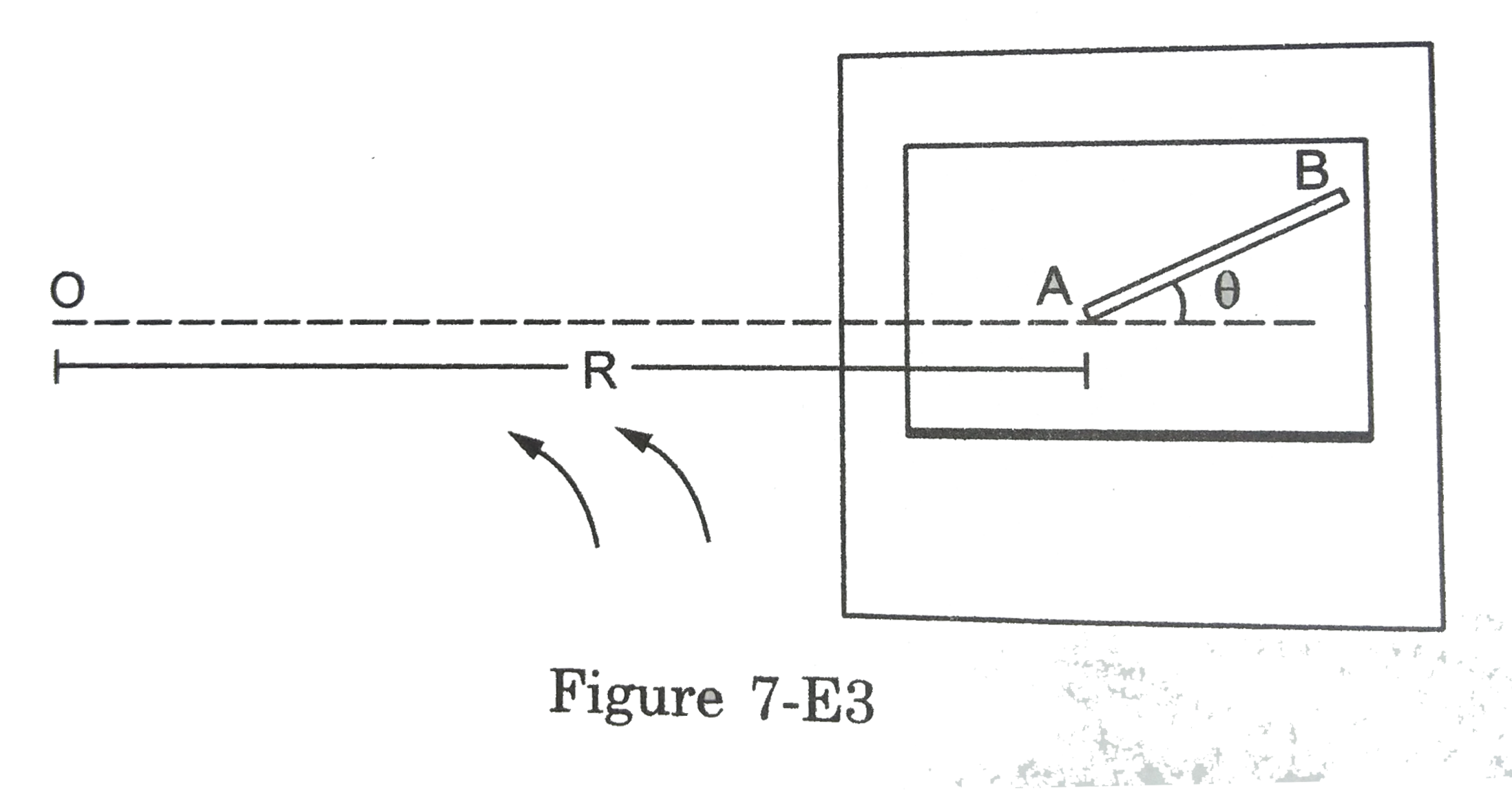 03:21View Solution
03:21View SolutionA particle is moving at a constant speed V from a large distance towards a concave mirror of radius R along its principal axis. Find the speed of the image formed by the mirror as a function of the distance x of the particle from the mirror.
09:08View SolutionFigure shows a block A of mass 6 m having a smooth semicircular groove of radius a placed on a smooth horizontal surface. A block B of mass m is released from a position in groove where its radius is horizontal. Find the speed of the bigger block when the smaller block reaches its bottom most position.
02:21View SolutionA uniform solid cone of mass m, base radius ‘R’ and height 2R, has a smooth groove along its slant height as shown in figure. The cone is rotating with angular speed ω, about the axis of symmetry. If a particle of mass ‘m’ is released from apex of cone, to slide along the groove, then angular speed of cone when particle reaches to the base of cone is
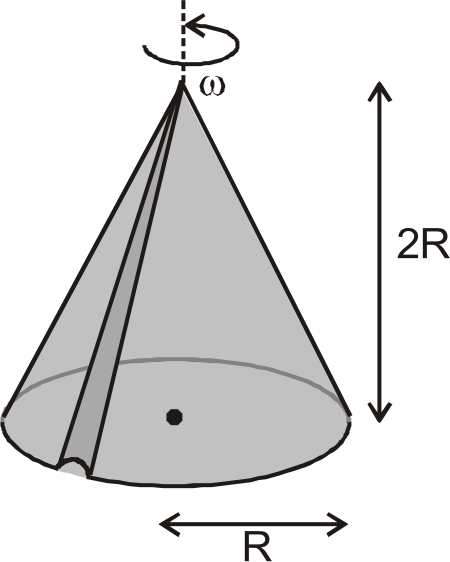 05:00View Solution
05:00View Solution_S01_013_Q01.png)
A very small groove is made in the earth, and a particle of mass m0 is placed at R2 distance from the centre. Find the escape speed of the particle fro that place.03:36View SolutionA particle moving on the inside of a smooth sphere of radius r describing a horizontal circle at a distance r//2 below the centre of the sphere. What is its speed ?
05:09View Solutionक्षैतिज सतह वाली एक वृत्ताकार टेबुल अपने अक्ष के परितः एकसमान कोणीय वेग ω से घूम रही है। इस टेबुल की सतह पर एक त्रिज्या के अनुदिश एक खाँच बनी हुई है। खाँच की दीवारों तथा तली को घर्षणरहित माने। इस खाँच में एक कण को केंद्र से a दूरी पर धीरे से रख दिया जाता है। जब कण की केंद्र से दूरी L हो जाए, उस समय कण की चाल निकालें।
View SolutionA horizontal disk is rotating with angular velocity omega about a vertical axis passing through its centre. A ball is placed at the centre of groove and pushed slightly. The velocity of the ball when it comes out of the groove -
01:39View SolutionA circular table with smooth horizontal surface is rotating at an angular speed ω about its axis. A groove is made on the surface along the radius and a small particle is gently placed inside the groove at a distance I from the centre. Find the speed of the particle with respect to the table as its distance from the centre becomes L.
02:35View SolutionA table with smooth horizontal surface is fixed in a cabin that rotates with a uniform angular velocity ω in a circular pathof radius R. A smooth groove AB of length L(<<R) is made on the surface of the table. The groove makes an angleθ with the radius OA of the circle in which the cabin rotates. A small particle is kept at the point A in the groove and is released to move along AB. Find the time taken by tehparticle to reach the point B.
 03:33View Solution
03:33View SolutionA particle is moving at a constant speed V from a large distance towards a concave mirror of radius R along its principal axis. Find the speed of the image formed by the mirror as a function of the distance x of the particle from the mirror.
03:24View Solution

