Three point charges Q1,Q2andQ3 in that order are placed equally spaced along a striaght line. Q2andQ3 are equal in magnitude but opposite is sign. If the net force on Q3 is zero, the value of Q1 is
Q1=|Q3|
Q1=√2|Q3|
Q1=2|Q3|
Q1=4|Q3|
The correct Answer is:D
To solve the problem, we need to find the value of charge Q1 such that the net force acting on charge Q3 is zero. Given that Q2 and Q3 are equal in magnitude but opposite in sign, we can denote:
- Q2=−q
- Q3=+q
Let’s denote the distance between each charge as d. Therefore, the distances between the charges are:
- Distance between Q1 and Q2 = d
- Distance between Q2 and Q3 = d
- Distance between Q1 and Q3 = 2d
Step 1: Calculate the forces acting on Q3
The force acting on Q3 due to Q2 (denoted as F23) and the force acting on Q3 due to Q1 (denoted as F13) can be calculated using Coulomb's Law:
F23=k|Q2Q3|d2=k|−q⋅q|d2=kq2d2
The direction of F23 is towards Q2 (to the left).
The force acting on Q3 due to Q1 is:
F13=k|Q1Q3|(2d)2=k|Q1⋅q|4d2
The direction of F13 is away from Q1 (to the right).
Step 2: Set the forces equal to each other for equilibrium
For the net force on Q3 to be zero, the magnitudes of these forces must be equal:
F13=F23
Substituting the expressions we obtained:
k|Q1⋅q|4d2=kq2d2
Step 3: Simplify the equation
We can cancel k and d2 from both sides:
|Q1⋅q|4=q
Step 4: Solve for Q1
Now, we can solve for Q1:
|Q1|=4
Since Q1 must be positive (as it is not specified to be negative), we conclude:
Q1=4q
Final Answer
Thus, the value of Q1 is 4×Q3.
---
- Q3=+q
- Distance between Q2 and Q3 = d
- Distance between Q1 and Q3 = 2d
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Exercise-II
56 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
42 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)
26 Videos
Similar Questions
A charge Q located at a point →r is in equilibrium under the combined field of three charges q1,q2 and q3. If the charges q1 and q2 are located at point →r1 and →r2 respectively, find the direction of the force on Q due to q3 in terms of q1,(q2),→r1→r2, and →r.
View SolutionThree charges +Q, q, +Q are placed respectively, at distance, 0, d//2 and d from the origin, on the x-axis. If the net force experienced by +Q placed at x=0, is zero, then value of q is :
View Solution
Knowledge Check
Q3 is always equal to
AP1BP25CP75DD3
Three charges +Q, q, +Q are placed respectively, at distance, 0, d//2 and d from the origin, on the x-axis. If the net force experienced by +Q placed at x=0, is zero, then value of q is :
View SolutionCharges−q,Q,and−q are placed at an equal distance on a straight liner. If the total potential energy of the system of three charges is zero, then find the ratio Q/q.
 .
.
View SolutionThree charges −q1,+q2 and −q3 are placed as shown in the figure. The x-component of the force on −q1 is proportional to

View SolutionIf the roots of the equation 1x+p+1x+q=1r are equal in magnitude but opposite in sign, show that p+q=2r & that the product of roots is equal to (−12)(p2+q2).
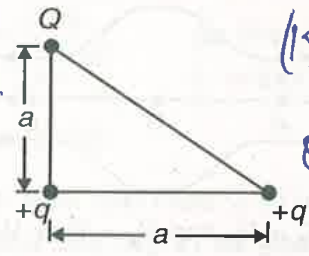
View SolutionThree charges +q , +q, and Q are placed at the comers of a right angled isosceles triangle as shown in the figure. If the net potential energy of the system is zero then value of Q is
View SolutionThreee charges +Q1,+Q2 and q are placed on a straight line such that q is somewhere in between +Q1and Q2. If this system of charges is in equlibirium what should be the magnitude and sign of charge q?
View SolutionThree charged paricles are placed on a straight line as shown in fig. q1 and q2 are fixed but q3 can be moved. Under the action of the forces from q1,q2 and q3 is in equilbrium. What is the relation between q1 and q2.
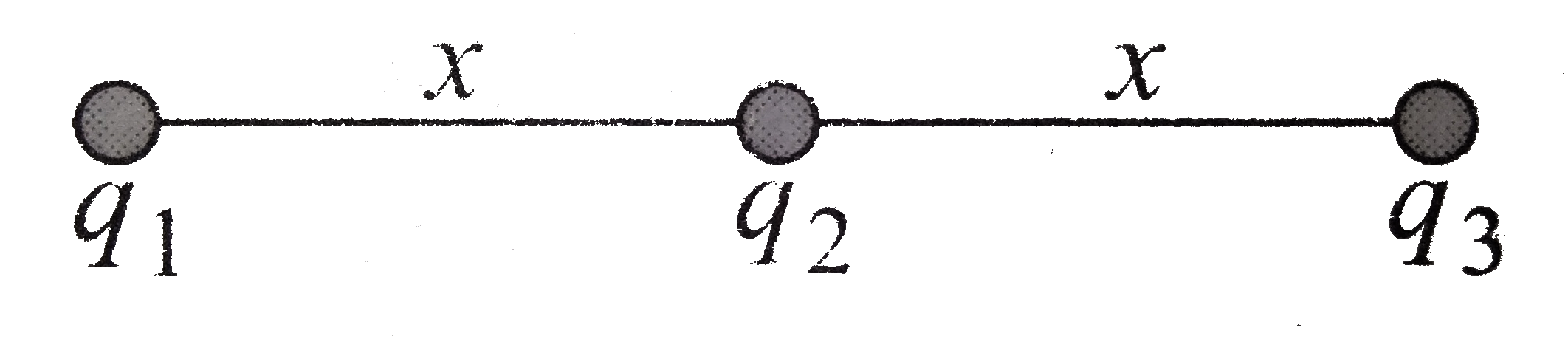
View Solution
