Three point masses 3kg, 2kg and 1kg are placed at A,B,C of a triangle. AB = 0.3,m AC = 0.5 m and BC = 0.4 m. The M.I of the system about an axis through A and normal to the plane of triangle is
0.43kgm2
0.25kgm2
0.18kgm2
0.07kgm2
The correct Answer is:A
To find the moment of inertia (M.I) of the system about an axis through point A and normal to the plane of the triangle, we will follow these steps:
1. Identify the Masses and Positions:
- Mass at A (m1) = 3 kg
- Mass at B (m2) = 2 kg
- Mass at C (m3) = 1 kg
- Distances:
- AB = 0.3 m
- AC = 0.5 m
- BC = 0.4 m
2. Determine the Distances from the Axis:
- The axis of rotation is through point A.
- Distance from A to B (r2) = AB = 0.3 m
- Distance from A to C (r3) = AC = 0.5 m
- Distance from A to A (r1) = 0 m (since it's the axis itself)
3. Calculate the Moment of Inertia:
The moment of inertia (I) about an axis is given by the formula:
I=m1r21+m2r22+m3r23
Substituting the values:
I=(3kg×02)+(2kg×(0.3m)2)+(1kg×(0.5m)2
4. Calculate Each Term:
- For mass at A:
3kg×02=0
- For mass at B:
2kg×(0.3m)2=2×0.09=0.18kg m2
- For mass at C:
1kg×(0.5m)2=1×0.25=0.25kg m2
5. Sum the Contributions:
I=0+0.18+0.25=0.43kg m2
Final Answer:
The moment of inertia of the system about an axis through A and normal to the plane of the triangle is:
I=0.43kg m2
- Mass at A (m1) = 3 kg
- Mass at B (m2) = 2 kg
- Mass at C (m3) = 1 kg
- Distances:
- AB = 0.3 m
- AC = 0.5 m
- BC = 0.4 m
- The axis of rotation is through point A.
- Distance from A to B (r2) = AB = 0.3 m
- Distance from A to C (r3) = AC = 0.5 m
- Distance from A to A (r1) = 0 m (since it's the axis itself)
The moment of inertia (I) about an axis is given by the formula:
I=m1r21+m2r22+m3r23
Substituting the values:
I=(3kg×02)+(2kg×(0.3m)2)+(1kg×(0.5m)2
- For mass at A:
3kg×02=0
- For mass at B:
2kg×(0.3m)2=2×0.09=0.18kg m2
- For mass at C:
1kg×(0.5m)2=1×0.25=0.25kg m2
I=0+0.18+0.25=0.43kg m2
The moment of inertia of the system about an axis through A and normal to the plane of the triangle is:
I=0.43kg m2
Similar Questions
Three masses of 2 kg, 3 kg and 4 kg are located at the three vertices of an equilateral triangle of side 1 m. What is the M.I. of the system about an axis along the altitude of the triangle and passing through the 3 kg mass?
View SolutionTwo small spheres of mass 5kg and 15kg are joined by a rod of length 0.5m and of negligible mass. The M.I. of the system about an axis passing through centre of rod and normal to it is
View Solution3 particles of mass 10 gm each are placed at the corners of an equilateral triangle of side 5 cm. The M.I. of the system about a perpendicular axis to the plane passing through a corner of the triangle will be : (in kg-m2)
View SolutionTwo partcles of masses 1 kg and 2 kg are placed at a distance of 3 m. Moment of inertia of the particles about an axis passing through their centre of mass and perpendicular to the line joining them is (in kg−m2) a) 6 b) 9 c) 8 d) 12
View SolutionTwo particles of masses 2 kg and 4 kg are placed along x-axis at positions x = 1 m and x = 4 m respectively. The moment of inertia of the system about an axis passing through centre of mass and parallel to y-axis is
View SolutionThree balls of masses 1kg,2kgand3kg are arranged at the corners of an equilateral triangle of side 1m. What will be the moment of inertia of the system and perpendicular to the plane of the triangle.
View SolutionThree point masses m1,m2 and m3 are located at the vertices of an equilateral triangle of side α. What is the moment of inertia of the system about an axis along the altitude of the triangle passing through m1?
View SolutionThree point masses m1,m2 and m3 are located at the vertices of an equilateral triangle of side α. What is the moment of inertia of the system about an axis along the altitude of the triangle passing through m1?
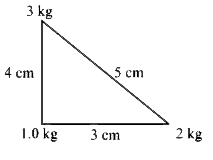
View SolutionThree point particles of masses 1.0 kg 2 kg and 3 kg are placed at three comers of a right angle triangle of sides 4.0 cm , 3.0 cm and 5.0 cm as shown in the figure . The centre of mass of the system is at point :
 View Solution
View SolutionThree point particles of masses 1.0 kg 2 kg and 3 kg are placed at three comers of a right angle triangle of sides 4.0 cm , 3.0 cm and 5.0 cm as shown in the figure . The centre of mass of the system is at point :
 View Solution
View Solution

