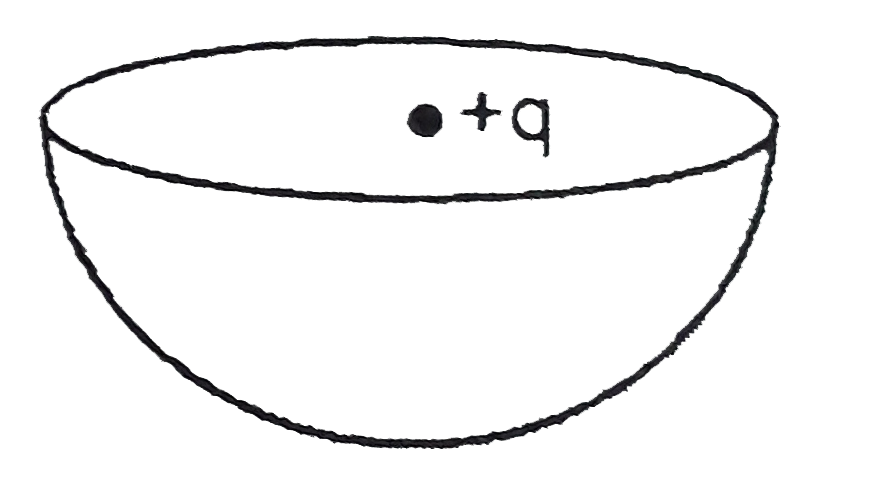
A charge q is placed at centre of non-conducting hemisphere the flux through curved surface area is
qε0
q2ε0
2qε0
πqε04
To solve the problem of calculating the electric flux through the curved surface of a non-conducting hemisphere with a charge q placed at its center, we can follow these steps:
1. Understanding the Setup:
We have a non-conducting hemisphere with a charge q placed at its center. The hemisphere has a curved surface and a flat circular base.
2. Applying Gauss's Law:
According to Gauss's Law, the total electric flux Φ through a closed surface is given by:
Φ=Qencϵ0
where Qenc is the total charge enclosed by the surface and ϵ0 is the permittivity of free space.
3. Considering the Hemisphere:
The hemisphere itself does not form a closed surface since it has an open flat base. To apply Gauss's Law effectively, we can imagine completing the hemisphere with another identical hemisphere to form a full sphere.
4. Calculating the Total Flux through the Full Sphere:
When we consider the full sphere, the charge q is now enclosed within this closed surface. The total electric flux through the entire sphere is:
Φsphere=qϵ0
5. Finding the Flux through the Curved Surface:
Since the sphere is symmetrical, the flux is uniformly distributed over the entire surface. Therefore, the flux through the curved surface of the hemisphere is half of the total flux through the sphere:
Φcurved=12Φsphere=12(qϵ0)=q2ϵ0
6. Conclusion:
The electric flux through the curved surface of the non-conducting hemisphere is:
Φ=q2ϵ0
We have a non-conducting hemisphere with a charge q placed at its center. The hemisphere has a curved surface and a flat circular base.
According to Gauss's Law, the total electric flux Φ through a closed surface is given by:
Φ=Qencϵ0
where Qenc is the total charge enclosed by the surface and ϵ0 is the permittivity of free space.
The hemisphere itself does not form a closed surface since it has an open flat base. To apply Gauss's Law effectively, we can imagine completing the hemisphere with another identical hemisphere to form a full sphere.
When we consider the full sphere, the charge q is now enclosed within this closed surface. The total electric flux through the entire sphere is:
Φsphere=qϵ0
Since the sphere is symmetrical, the flux is uniformly distributed over the entire surface. Therefore, the flux through the curved surface of the hemisphere is half of the total flux through the sphere:
Φcurved=12Φsphere=12(qϵ0)=q2ϵ0
The electric flux through the curved surface of the non-conducting hemisphere is:
Φ=q2ϵ0
Topper's Solved these Questions
Similar Questions
Knowledge Check
A charge q is located at the centre of a cube. The electric flux through any face is
Aπq6(4π∈0)Bq6(4π∈0)C2πq6(4π∈0)D4πq6(4π∈0)A charge q is located at the centre of a cube. The electric flux through any face is
Aπq6(πε0)Bq6(4πε0)C2πq6(4πε0)D4πq6(4πε0)A charge q is located at the centre of a cube. The electric flux through any face is
A4πq6(4πε0)Bπq6(4πε0)Cq6(4πε0)D2πq6(4πε0)
A point charge +q is placed at the centre of a conducting spherical shell of inner radius a and outer radius b. Find the charge appearing on the inner and outer surface of the shell.
View SolutionIf a point charge q is placed at the centre of a cube, then find out flux through any one face of cube.
View SolutionChoose the incorrect statement :
(a) The electric lines of force entering into A Gaussian surface provide nagative flux.
(b) A charge 'q' is placed at the centre of a cube .The flux through all the faces will be the same .
(c ) In uniform electric field net through a closed Gaussian surface containing no net charge ,is zero .
(d) When electric field is parallel to a Gaussian surface ,it provides a finite non -zero flux. Choose the most appropriate answer from the options given below :
View SolutionA conducting sphere that carries a total charge of -3q is placed at the centre of a conducting spherical shell that carries a total charged of +5q. The conductors are in electrostatic equilibrium. The charges on the inner and outer surfaces of the shell are respectively.

View SolutionAn electric charge q is placed at the centre of a cube of side l. The electric flux through one of its faces will be
View Solution