The area bounded by y = |sin x|, X-axis and the line |x|=π is
A
2 sq units
B
3 sq units
C
4 sq units
D
None of these
Video Solution
Text Solution
The correct Answer is:C
∴ Required area =2∫π0 sin x dx
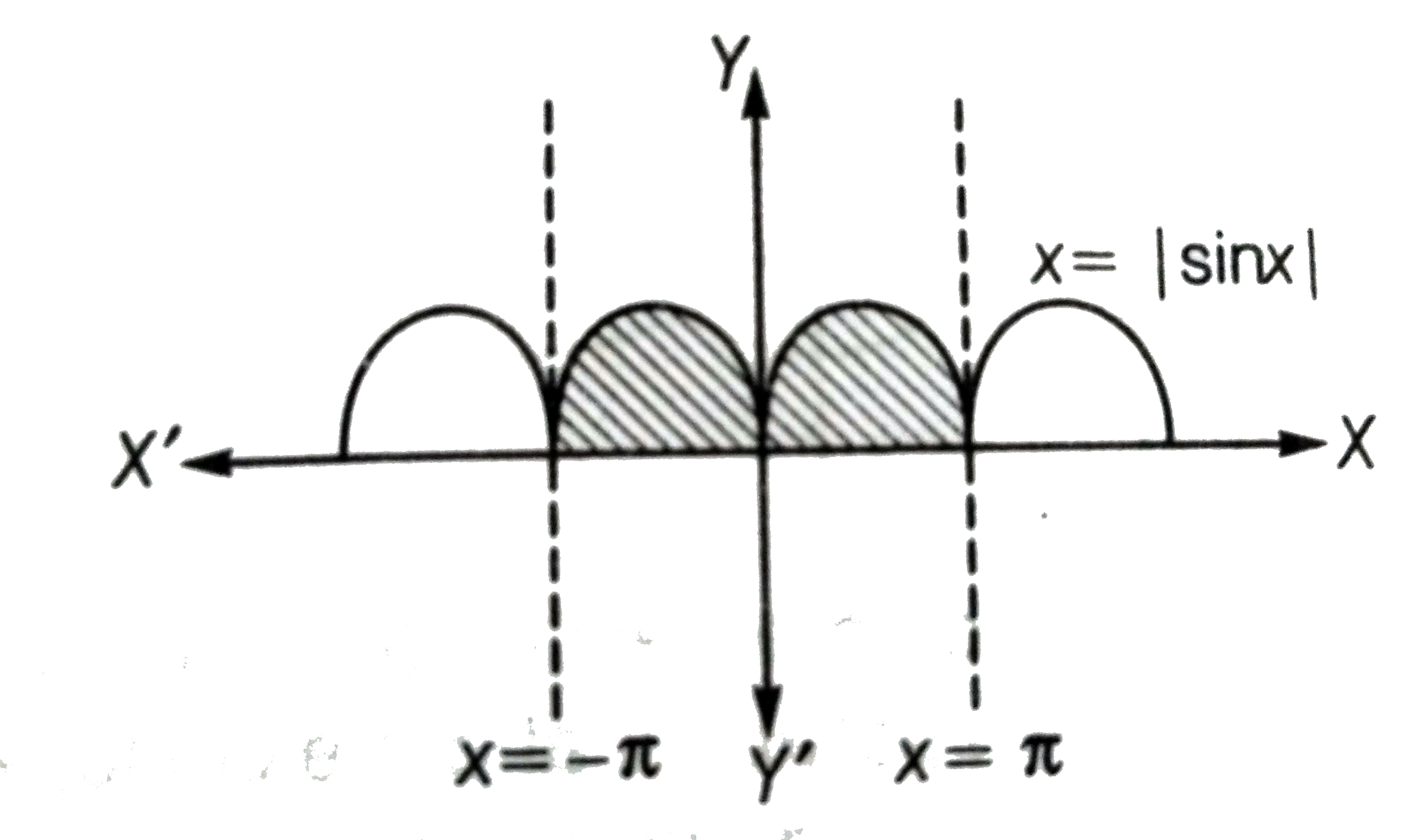
=2[−cosx]π0=2[1+1]=4 sq units
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DEFINITE INTEGRALS EXERCISE - MHT CET Corner6videos
APPLICATIONS OF DEFINITE INTEGRALS
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DEFINITE INTEGRALS EXERCISE - MHT CET Corner6videos
APPLICATIONS OF DEFINITE INTEGRALS
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - MHT CET CORNER21videos
APPLICATIONS OF DERIVATIVES
Similar Questions
Knowledge Check
- Question 1 - Select One
The area bounded by the line y = x, X-axis and the lines x = -1, x = 2 is
A52sq.unitsB32sq.unitsC13sq.unitsD23sq.units - Question 2 - Select One
The area bounded by y=sinxx, x-axis and the ordinates x = 0, x=π/4 is equal to π/4 less than π/4 is
Aequal to π/4Bless than π/4Cgreater than π/4Dless than x/4∫0tanxxdx - Question 3 - Select One
The area bounded by the curve y=|x|, X axis and the lines x=−π and x=π is
A2 sq. unitsB1 sq. unitCπ^2 sq. unitsDNone of these
Area bounded by y=[sinx+cosx] and the lines x=0,x=π is
View SolutionThe area of the region bounded by the curve y = sinx , X-axis and the lines x = 0, x=4π is …… sq. units.
View SolutionThe area bounded by y=x|sinx| and x - axis between x=0,x=2π is
View SolutionLet f (x) be a continous function such that the area bounded by the curve y=f(x) x-axis and the lines x=0 and x=aisa22+a2sina+π2cosa, then f(π2) is
View Solution(i) Find the area bounded by y=3x+2, the x-axis and the ordinates x=−2andx=1.
(ii) Find the area bounded by y=x, the x-axis and the line x=−1andx=2.View Solution

