A disc is placed on the ground. Friction coefficient is . What is the minimum force required to move the disc if it is applied at the topmost point?
To find the minimum force required to move a disc placed on the ground when the force is applied at the topmost point, we can follow these steps:
1. Identify the Forces Acting on the Disc:
- The forces acting on the disc include:
- The weight of the disc (W = mg), acting downwards.
- The normal force (N), acting upwards.
- The frictional force (F_friction), opposing the motion.
2. Understand the Role of Friction:
- The frictional force that needs to be overcome to initiate motion is given by:
Ffriction=μN
- Here, μ is the coefficient of friction and N is the normal force.
3. Determine the Normal Force:
- For a disc resting on a flat surface, the normal force N is equal to the weight of the disc:
N=mg
- Thus, the frictional force can be rewritten as:
Ffriction=μmg
4. Apply the Force at the Topmost Point:
- When a force F is applied at the topmost point of the disc, it creates a torque about the center of the disc.
- The torque due to the applied force is:
τapplied=F⋅R
- The torque due to friction (which opposes the motion) is:
τfriction=Ffriction⋅R=μmg⋅R
5. Set the Torques Equal for Motion to Start:
- For the disc to start moving, the applied torque must equal the torque due to friction:
F⋅R=μmg⋅R
6. Solve for the Minimum Force F:
- We can cancel R from both sides (assuming R≠0):
F=μmg
7. Final Result:
- Therefore, the minimum force required to move the disc when applied at the topmost point is:
F=μmg
- The forces acting on the disc include:
- The weight of the disc (W = mg), acting downwards.
- The normal force (N), acting upwards.
- The frictional force (F_friction), opposing the motion.
- The frictional force that needs to be overcome to initiate motion is given by:
Ffriction=μN
- Here, μ is the coefficient of friction and N is the normal force.
- For a disc resting on a flat surface, the normal force N is equal to the weight of the disc:
N=mg
- Thus, the frictional force can be rewritten as:
Ffriction=μmg
- When a force F is applied at the topmost point of the disc, it creates a torque about the center of the disc.
- The torque due to the applied force is:
τapplied=F⋅R
- The torque due to friction (which opposes the motion) is:
τfriction=Ffriction⋅R=μmg⋅R
- For the disc to start moving, the applied torque must equal the torque due to friction:
F⋅R=μmg⋅R
- We can cancel R from both sides (assuming R≠0):
F=μmg
- Therefore, the minimum force required to move the disc when applied at the topmost point is:
F=μmg
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 2 Single Correct
26 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 2 Multiple Correct
36 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 1 Objective
30 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
Similar Questions
A block of mass m is attached with a massless spring of force constant K. the block is placed over a rough inclined surface for which the coefficient of friction is μ=34 find the minimum value of M required to move the block up the place. (Neglect mass of string and pulley. Ignore friction in pulley).
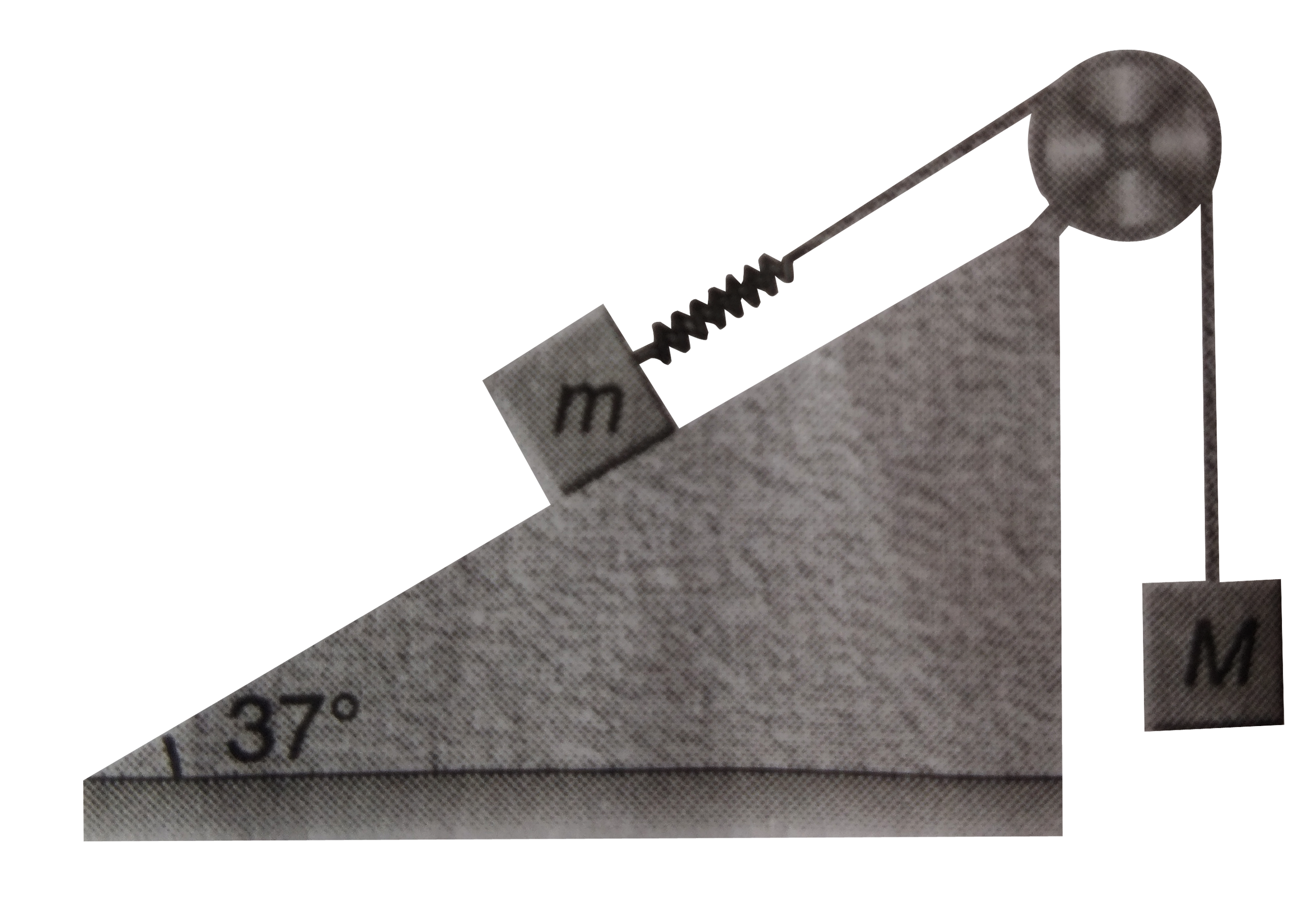 .
.
View Solution
Three blocks are connected as shown in the figure. Valculate the minimum force required to move the body with constant velocity. The coefficient of friction at all surfaces is 0.25.
View Solution
Knowledge Check
A capacitor is made of two circular plates of radius R each, separated by a distance d<<R. The capacitor is connected to a constant voltage. A thin conducting disc of radius r<<R and thickness t<<r is placed at a center of the bottom plate. Find the minimum voltage required to lift the disc if the mass of the disc is m.
A√mgdπε0r2B√mgdπε0rC√mgd2πε0r2D√mgdπε0r2
A block of mass m is attached with a massless spring of force constant k. The block is placed over a rough inclined surface for which the coefficient of friction is μ=34. The minimum value of M required to move the block up the plane is (Neglect mass of string, mass of pulley and friction in pulley)
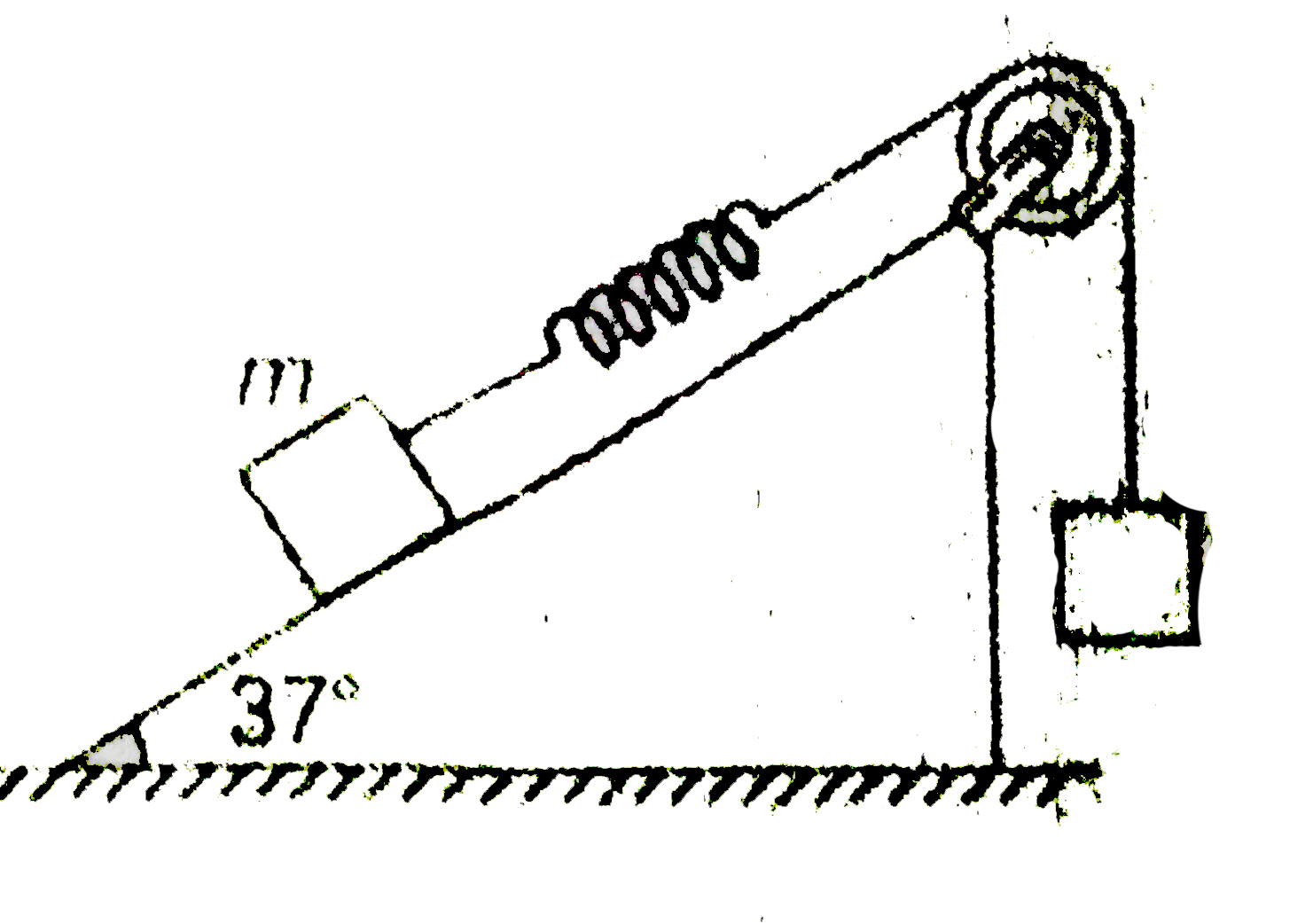
View SolutionAn isotropic point source is placed at a depth h below the water surface. A floating opaque disc is placed on the surface of water so that the source is not visible from the surface. What is the minimum radius of the disc? Take refractive index of water=μ.
View SolutionAn isotropic point source is placed at a depth h below the water surface. A floating opaque disc is placed on the surface of water so that the source is not visible from the surface. What is the minimum radius of the disc? Take refractive index of water=μ.
View SolutionIf a pushing force making an angle α with horizontal is applied on a block of mass m placed on horizontal table and angle of friction is β, then minimum magnitude of force required to move the block is
View SolutionA block of mass m is at rest on a rough inclined plane of angle of inclination θ. If coefficient of friction between the block and the inclined plane is μ, then the minimum value of force along the plane required to move the block on the plane is
View SolutionA uniform disc of radius R is spinned to the angular velocity ω and then carefully placed on a horizontal surface. How long will the disc be rotating on the surface if the friction coeffiecient is equal to k? The pressure exerted by the disc on the surface can be regarded as uniform.
View SolutionA disc of mass 3 m and a disc of mass m are connected by a massless spring of stiffness k. The heavier disc is placed on the ground with the spring vertical and lighter disc on top from its equilibrium position the upper disc is pushed down by a distance δ and released. Then.
View Solution
