A magnet is taken towards a conducting ring in such a way that a constant current of 10mA is induced in it. The total resistance of the ring is 0.5Ω. In 5s, the magnetic flux through the ring changes by
0.25mWb
25mWb
50mWb
15mWb
The correct Answer is:B
To solve the problem, we will use Faraday's law of electromagnetic induction, which states that the induced electromotive force (emf) in a closed loop is equal to the rate of change of magnetic flux through the loop.
1. Identify the Given Values:
- Induced current (I) = 10 mA = 10×10−3 A
- Resistance of the ring (R) = 0.5 Ω
- Time interval (Δt) = 5 s
2. Use Ohm's Law to Find Induced EMF:
According to Ohm's Law, the induced emf (ε) can be calculated using the formula:
emf=I×R
Substituting the known values:
emf=(10×10−3A)×(0.5Ω)=5×10−3V=5mV
3. Apply Faraday's Law:
Faraday's law states that:
emf=−ΔΦΔt
Rearranging this gives us:
ΔΦ=−emf×Δt
Since we are interested in the magnitude, we can ignore the negative sign:
ΔΦ=emf×Δt
4. Substitute the Values:
Now, substituting the values we have:
ΔΦ=(5×10−3V)×(5s)=25×10−3Wb
5. Convert to Mill Weber:
ΔΦ=25mWb
Final Answer:
The total change in magnetic flux through the ring in 5 seconds is 25mWb.
---
- Induced current (I) = 10 mA = 10×10−3 A
- Resistance of the ring (R) = 0.5 Ω
- Time interval (Δt) = 5 s
According to Ohm's Law, the induced emf (ε) can be calculated using the formula:
emf=I×R
Substituting the known values:
emf=(10×10−3A)×(0.5Ω)=5×10−3V=5mV
Faraday's law states that:
emf=−ΔΦΔt
Rearranging this gives us:
ΔΦ=−emf×Δt
Since we are interested in the magnitude, we can ignore the negative sign:
ΔΦ=emf×Δt
Now, substituting the values we have:
ΔΦ=(5×10−3V)×(5s)=25×10−3Wb
ΔΦ=25mWb
The total change in magnetic flux through the ring in 5 seconds is 25mWb.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective Questions
3 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 1 Subjective
21 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 1 Assertion And Reason
10 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery
97 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
Similar Questions
The resistance of a coil is 5 ohm and a current of 0.2A is induced in it due to a varying magnetic field. The rate of change of magnetic flux in it will be-
View SolutionThe diagram given below shows a solenoid carrying time varying current l=l0t. On the axis of the solenoid, a ring has been placed. The mutual inductance of the ring and the solenoid is M and the self inductance of the ring is L. If the resistance of the ring is R then maximum current which can flow through the ring is
View Solution
Knowledge Check
By a change of current from 5 A to 10 A in 0.1 s, the self induced emf is 10 V. The change in the energy of the magnetic field of a coil will be
A5JB6JC7.5JD9JBy a change of current from 5 A to 10 A in 0.1 s, the self induced emf is 10 V. The change in the energy of the magnetic field of a coil will be
A5JB6JC7.5JD9J
The diagram shows a solenoid carrying time varying current I=I0t (I0 is constant) on the axis of this solenoid a conducting ring is being placed as shown in the figure. The mutual inductance of the ring and the solenoid is M and self inductance of the ring is L . if the resistance of the ring is R then the maximum current which can flow through the ring is
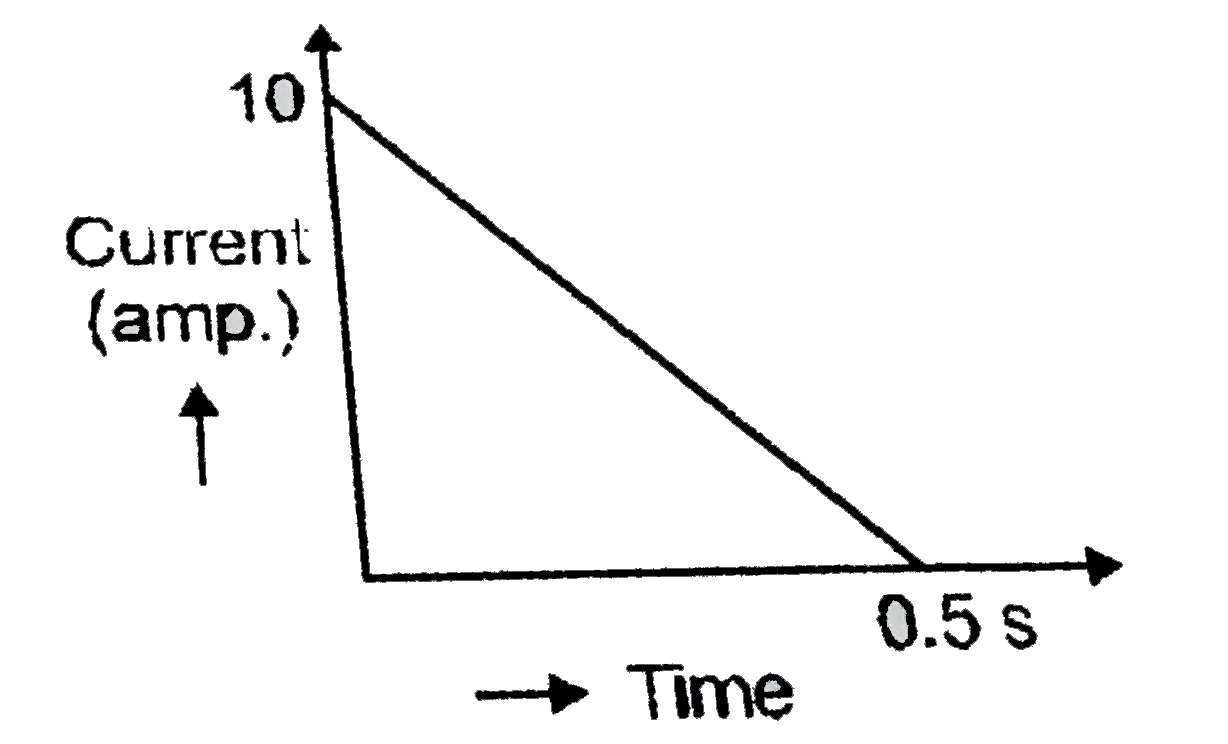
View SolutionIn a coil of resistance 100Ω , a current is induced by changing the magnetic flux through it as shown in the figure. The magnitude of change in flux through the coil is
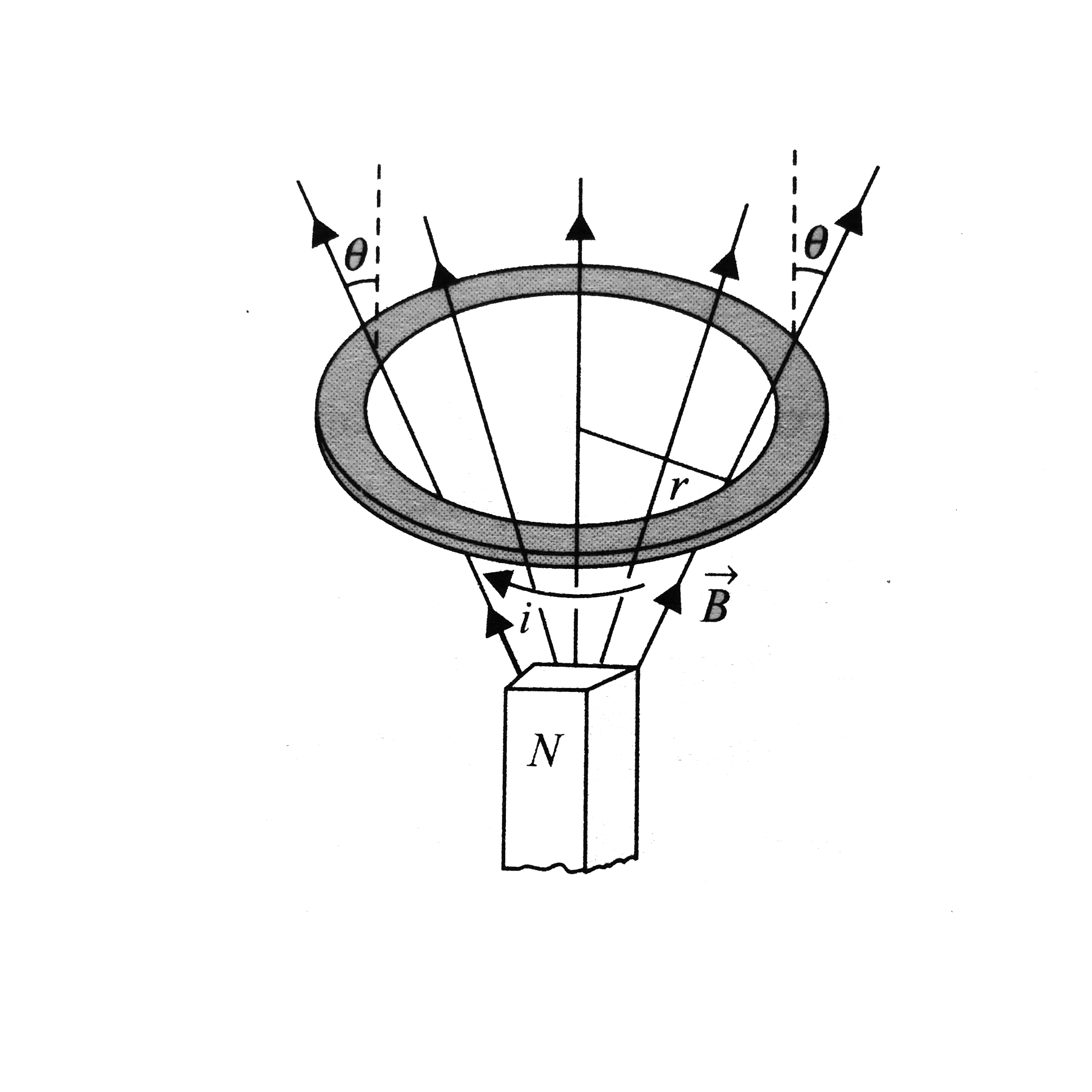
View SolutionA strong magnet is placed under a horizontal conducting ring of radius r that carries current i as shown in Fig. If the magnetic field makes an angle θ with the vertical at the ring's location, what are the magnitude and direction of the resultant force on the ring?
View SolutionMagnetic flux of 5 microweber is linked with a coil, when a current of 1mA flows through it. What is the self-inductance of the coil?
View SolutionA thin circular ring of area A is held perpendicular to a uniform magnetic field of induction B. A small cut is made in the ring and a galvanometer is connected across the ends such that the total resistance of the circuit is R. When the ring is suddenly squeezed to zero area, the charge flowing through the galvanometer is
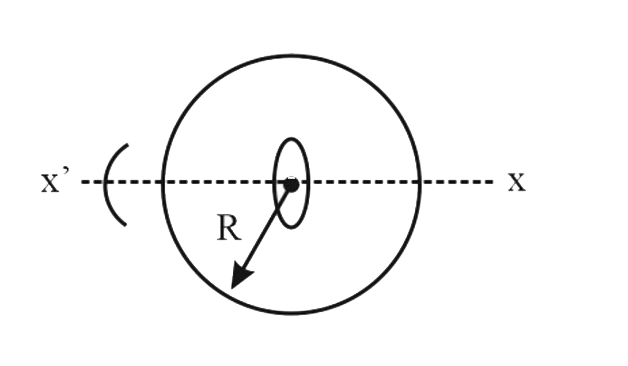
View SolutionA non-conducting ring of radius R having uniformly distributed charge Q starts rotating about x-x' axis passing through diameter with an angular acceleration α, as shown in the figure. Another small conducting ring having radius a(a<<R) is kept fixed at the centre of bigger ring is such a way that axis xx' is passing through its centre and perpendicular to its plane. If the resistance of small ring is r=1Ω , find the induced current in it in ampere.
(Given q=16×102μ0C,R=1m,a=0.1m,α=8rad s−2
View Solution
