At what displacement K.E. and P.E. are equal, for a body executing shm.
To find the displacement at which the kinetic energy (K.E.) and potential energy (P.E.) are equal for a body executing simple harmonic motion (SHM), we can follow these steps:
1. Understand the expressions for K.E. and P.E.:
- The kinetic energy (K.E.) of a body in SHM is given by:
K.E.=12mv2
- The potential energy (P.E.) is given by:
P.E.=12kx2
where m is the mass of the body, v is the velocity, k is the spring constant, and x is the displacement from the mean position.
2. Set K.E. equal to P.E.:
- We need to find the displacement x where K.E. = P.E.:
12mv2=12kx2
3. Express the velocity v in terms of displacement x:
- For a body in SHM, the velocity v can be expressed as:
v=ω√A2−x2
where A is the amplitude and ω is the angular frequency, given by ω=√km.
4. Substitute the expression for v into the K.E. equation:
- Substitute v into the K.E. expression:
K.E.=12m(ω√A2−x2)2
- This simplifies to:
K.E.=12m(km)(A2−x2)=12k(A2−x2)
5. Set the K.E. expression equal to the P.E. expression:
- Now we have:
12k(A2−x2)=12kx2
6. Simplify the equation:
- Cancel out 12k from both sides (assuming k≠0):
A2−x2=x2
- Rearranging gives:
A2=2x2
7. Solve for x:
- Rearranging the equation gives:
x2=A22
- Taking the square root:
x=A√2
Conclusion:
The displacement at which the kinetic energy and potential energy are equal for a body executing SHM is:
x=A√2
- The kinetic energy (K.E.) of a body in SHM is given by:
K.E.=12mv2
- The potential energy (P.E.) is given by:
P.E.=12kx2
where m is the mass of the body, v is the velocity, k is the spring constant, and x is the displacement from the mean position.
- We need to find the displacement x where K.E. = P.E.:
12mv2=12kx2
- For a body in SHM, the velocity v can be expressed as:
v=ω√A2−x2
where A is the amplitude and ω is the angular frequency, given by ω=√km.
- Substitute v into the K.E. expression:
K.E.=12m(ω√A2−x2)2
- This simplifies to:
K.E.=12m(km)(A2−x2)=12k(A2−x2)
- Now we have:
12k(A2−x2)=12kx2
- Cancel out 12k from both sides (assuming k≠0):
A2−x2=x2
- Rearranging gives:
A2=2x2
- Rearranging the equation gives:
x2=A22
- Taking the square root:
x=A√2
The displacement at which the kinetic energy and potential energy are equal for a body executing SHM is:
x=A√2
Topper's Solved these Questions
- BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - VERY SHORT ANSWER QUESTIONS13videos
OSCILLATIONS
- BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - SELECTED PROBLEMS (FROM THE CHARACTERISTIC OF SHM)14videos
OSCILLATIONS
- BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - LONG ANSWER QUESTIONS3videos
OSCILLATIONS
- BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - SELECTED PROBLEMS (FROM POISEUILLE.S FORMULA)19videos
MOTION IN FLUIDS
- BOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 4 ( TEMPERATURE ) UNSOLVED PROBLEMS12videos
PROPERTIES OF MATTER
Similar Questions
Derive an expression for the P.E. and K.E. of a body executing shm.
View SolutionDraw a graph between displacement and time for a body executing natural vibrations.
View SolutionDraw the velocity-displacement graph of a body executing shm.
View SolutionDraw the graph between displacement and
kinetic energy for a particle executing SHM.View SolutionDraw the graph between displacement and
total energy for a particle executing SHM.View SolutionWrite the relation between acceleration, displacement and frequency of a particle executing SHM.
View SolutionDraw the graph between displacement and
potential energy for a particle executing SHM.View SolutionWrite down the differential equation of a body executing shm. Give its solution.
View SolutionDraw the displacement-time, velocity-time and acceleration-time graph of a body executing shm.
View SolutionThe displacement (x) is plotted with time (t) for a particle executing SHM as shown below. The correct equation of SHM is
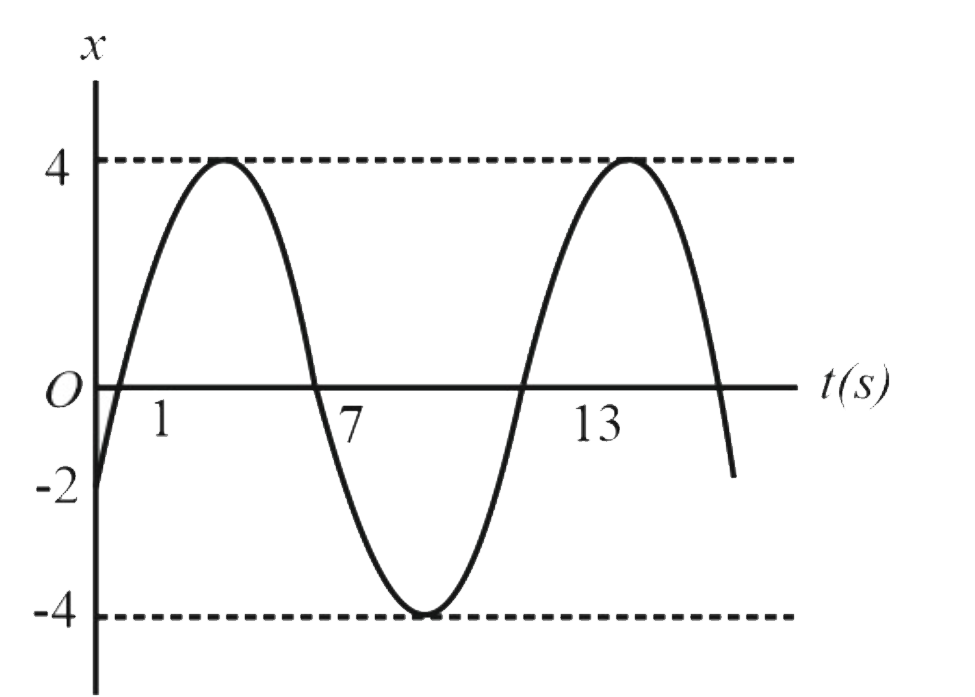 View Solution
View Solution

