The figure shows a $Δ A O B$ and the parabola $y = x^{2}$ . The ratio of the area of the $Δ A O B$ to the area of the region AOB of the parabola $y = x^{2}$ is equal to

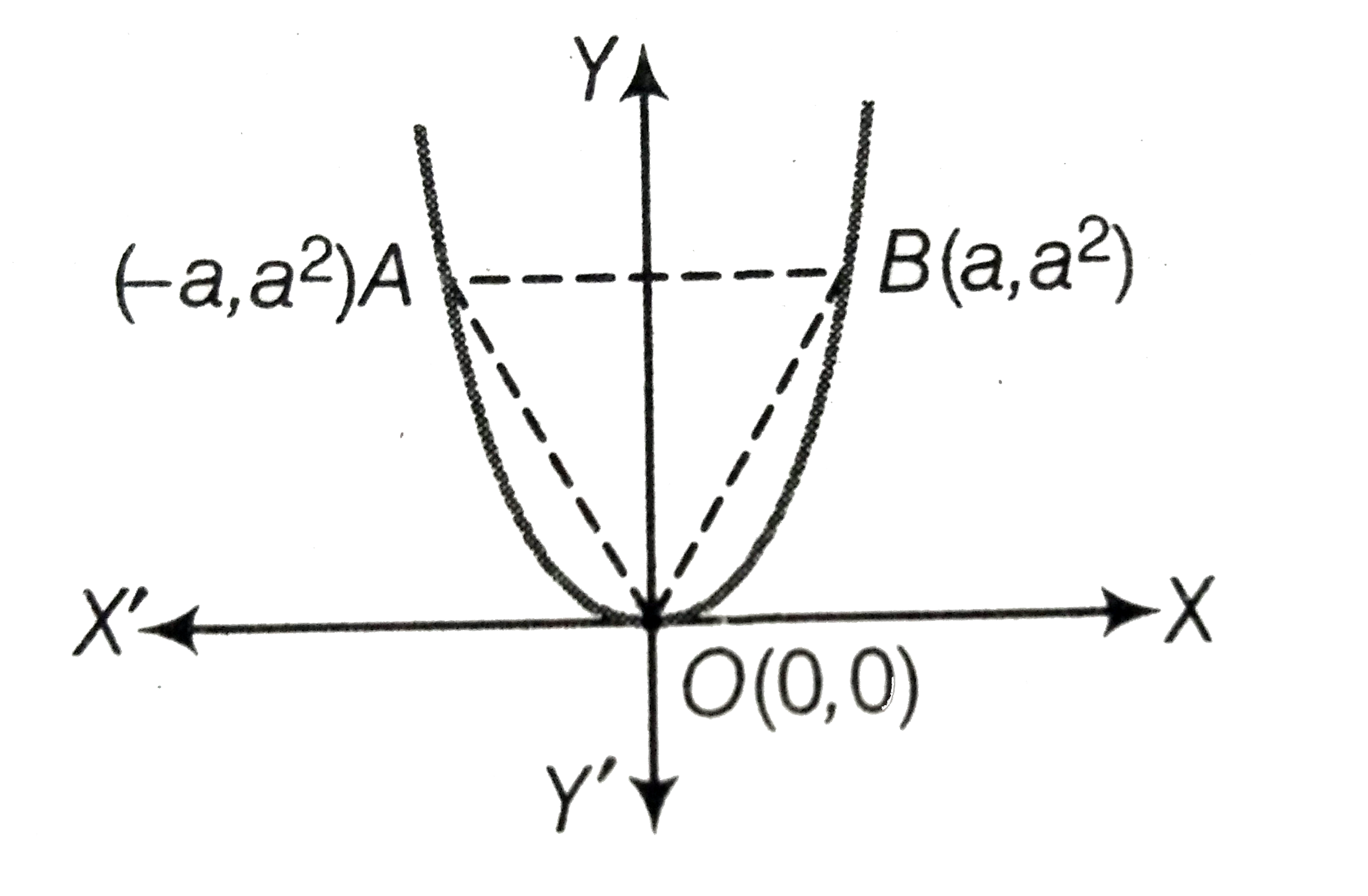
A

$\frac{3}{5}$

B

$\frac{3}{4}$

C

$\frac{7}{8}$

D

$\frac{5}{6}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Area of curve $O A B = 2 \int_{0}^{a^{2}} x d y$

$= 2 \int_{0}^{a^{2}} \sqrt{y} d y = 2 {[\frac{y^{3 / 2}}{3 / 2}]}_{0}^{a^{2}} = \frac{4}{3} [a^{3}]$
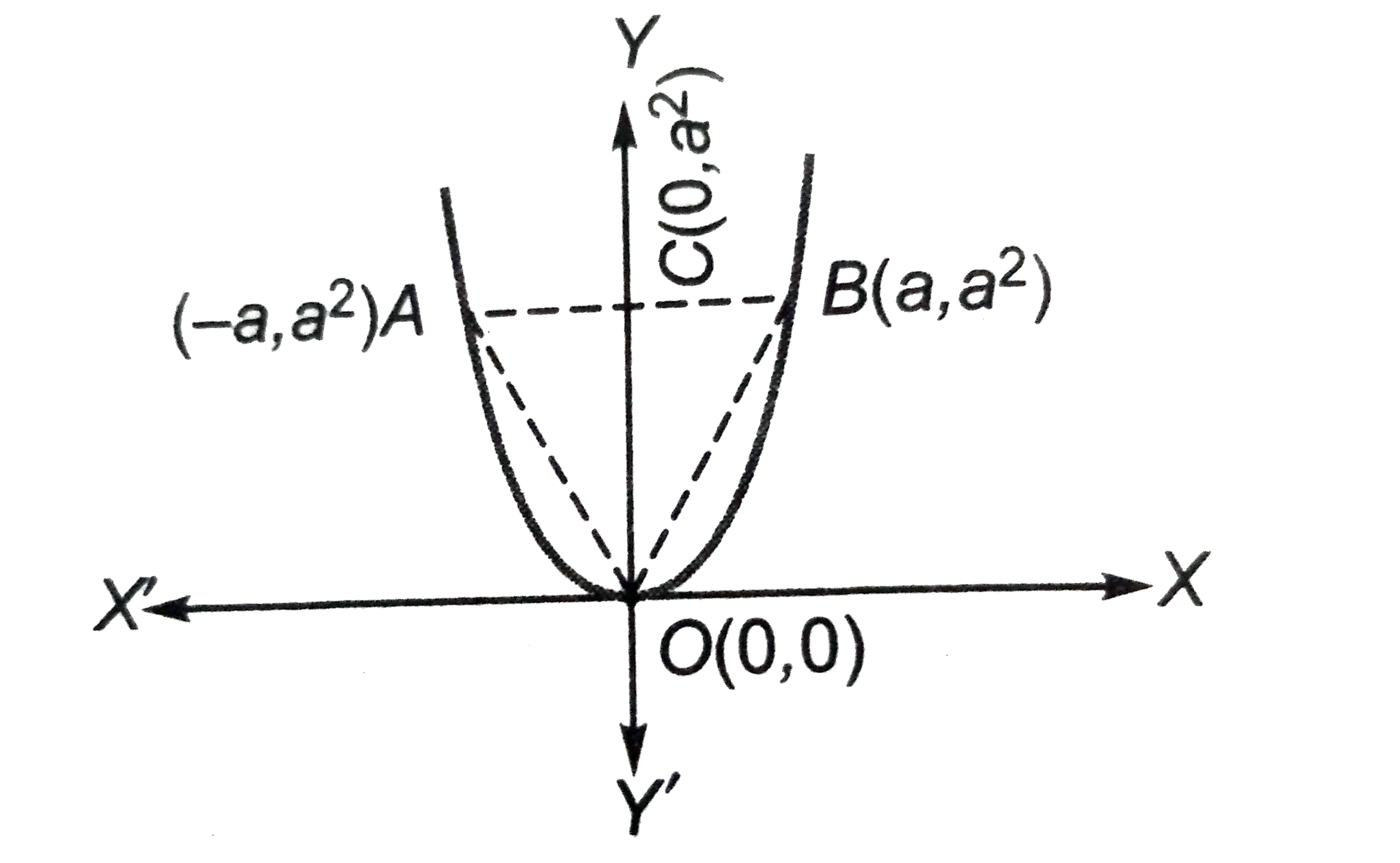
Now, area of $Δ O A B = \frac{1}{2} \times A B \times O C$
$= \frac{1}{2} \times 2 a \times a^{2} = a^{3}$
$∴ \frac{Area of Δ A O B}{Area of curve AOB} = \frac{a^{3}}{\frac{4}{3} a^{3}} = \frac{3}{4}$

|

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The area of the region bounded by y – x = 2 and $x^{2} = y$ is equal to
A $\frac{16}{3}$
B $\frac{2}{3}$
C $\frac{9}{2}$
D $\frac{4}{3}$
Question 2 - Select One
The area of the region bounded by the parabola $y = x^{2}$ and the line y = 4x is
A $\frac{32}{3} s q . u n i t s$
B $\frac{16}{3} s q . u n i t s$
C $\frac{8}{3} s q . u n i t s$
D $\frac{4}{3} s q . u n i t s$

MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS-APPLICATIONS OF DEFINITE INTEGRALS -Exercise 2

Home
Ask Doubt
Profile