Complete Solutions to Illustration of chapter LINEAR AND ANGULAR SIMPLE HARMONIC MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | LINEAR AND ANGULAR SIMPLE HARMONIC MOTION |
| CENGAGE PHYSICS | Illustration |
| Number Of Questions | 52 |
| Academic Year | 2023-24 |
What is the time - period of `x = A sin (omega t + alpha)`?
View SolutionFind time period of the function, `y=sin omega t + sin 2omega t + sin 3omega t`
View SolutionIdentify which of the following function represent simple harmonic motion.
(i) `Y = Ae^(I omega t)` (ii) `Y = a e^(- omega t)`
(iii) `y = a sin^(2) omega t` (iv) `y = a sin omega t + b cos omega t`
(v) `y = sin omega t + b cos 2 omega t`
View SolutionA partical excutes SHM with an angular frequency `omega = 4 pi rad//s`. If it is at its extereme position initially, then find the transition when it is at a distance `sqrt2//2` times its amplitude from the mean position.
View SolutionA partical excutes SHM with an angular an amplitude `8 cm` and a frequency `4 cm` initially, in the possitive direction , determine its displecement equation and the maximum velocity and acceleration.
View SolutionA particle executing SHM oscillates be between two fixed points separated by `20 cm` . If its maximum velocity be `30 cm//s`. Find its velocity when its displacement is `5 cm` from its mean position.
View SolutionA partical excuting SHM with time period of `2 s`: Find the time taken by it to move from one amplitude t half the amplitude posituion.
View SolutionWrite the equation of SHM for the situations show below:

View SolutionA partical of `mass m = 1 kg` oscillates simple harmonically with angular frequency `1 rad//s`. Find the phase of the partical at `t = 1 s and 2 s`. Start calculating time when the partical moves up passing through the mean position.
View SolutionIf `x = A//2 at t = 0`, Find phase constant `(alpha" in" x = A sin (omega t + alpha), at t = 0`, a partical excuting SHM is going along
View SolutionShows the displacement time graph of a particle executing SHM with a time period `T`. Four points `1,n 2, 3` and `4` are market on the graph where the displacement is half that of the amplitude.
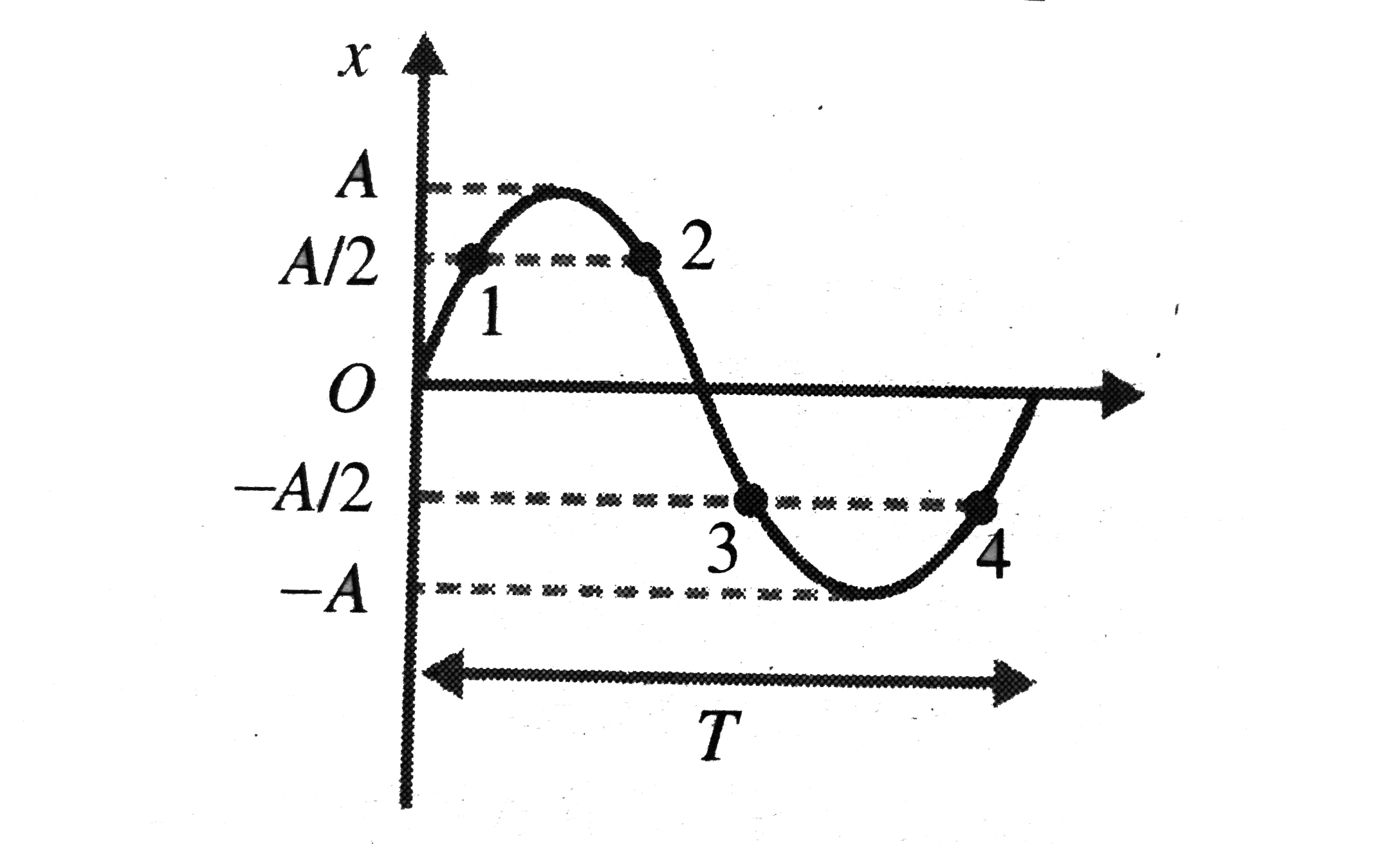
a. Identify the point of same displacement but with opposite direction of motion . Find the time difference between them.
b. Identify the point where the particles move in the same difference. Find the time difference between them.
View SolutionA partical excutes SHM with same frequency and amplitude along the same straight line. They cross each other , at a point midway between the mean and the exterme position. Find the Phase difference between them.
View SolutionA particle executes SHM with amplitude `A` and angular frequency `omega`. At an instant the particle is at a distance `A//5` from the mean position and is moving away from it. Find the time after which it will come back to this position again and also find the time after which it will pass through the mean position.
View Solutiontwo particle executing SHM with same frequency and amplitudes `A and 2A` on same straight line with same mean position cross each other in opposite direction at a distance `A//3` from mean position. Find the phase difference in the two SHM s.
View SolutionA particle of mass 0.50 kg executes a simple harmonic motion under a force `F=-(50Nm^-1)x`. If it crosses the centre of oscillation with a speed of `10ms^-1`, find the amplitude of the motion.
View SolutionA `20g` partical is oscillating simple barmonically with a period of `2 second` and maximum kinetic energy `2 J`. The total machanical energy of the partical is zero , find
a Amplitude of oscillation
b. potential energy as a punction of displacement x relative to mean position.
View SolutionA body is executing `SHM` under action of the a force of whose maximum is `50 N`. magnitude of force acting on the particle at the time when its energy is half kinetic energy and half potential is (Assume potential energy to be zero at mean position).
View SolutionA point particle if mass `0.1 kg` is executing SHM of amplitude `0.1 m`. When the particle passes through the mean position, its kinetic energy is `8 xx 10^(-3)J`. Write down the equation of motion of this particle when the initial phase of oscillation is `45^(@)`.
View SolutionIf a particle moves in a potential energy held `U = U_(0) - ax + bx^(2)`, where are a and b particle constants obtain an expression for the force acting on if as a function of position. At what point does the force vanish? Is this a point of stable equilibrium ?
Calculate the force constant and frequency of the particle.
View SolutionA partical executes SHM with an amplltude of `10 cm` and friquency `2 Hz, at t = 0`, the partical is at point where potential energy and kinetic energy are same. Find the equation of displacement of partical.
View SolutionA particle of mass `0.2 kg` undergoes SHM according to the equation `x (t) = 3 sin (pi t + pi//4)`.
i. What is the total energy of the particle if potential energy of zero at mean position ?
ii. What are the kinetic and potential energies of particle at time `t = 1 s`?
iii. At what time instants is the particles energies purely kinetic?
View SolutionA particle of mass `0.2 kg` executes simple harmonic motion along a path of length `0.2 m` at the rate of `600`oscillations per minute. Assume at `t = 0`. The particle start SHM in positive direction. Find the kinetic potential energies in joules when the displacement is `x = A//2` where, A stands for the amplitude.
View SolutionA point particle if mass `0.1 kg` is executing SHM of amplitude `0.1 m`. When the particle passes through the mean position, its kinetic energy is `8 xx 10^(-3)J`. Write down the equation of motion of this particle when the initial phase of oscillation is `45^(@)`.
View SolutionA particle of mass `m` is located in a unidimensional potential field where potential energy of the particle depends on the coordinates `x as: U (x) = U_(0) (1 - cos Ax), U_(0)` and A constants.
Find the period of small oscillation that the particle performs about the equilibrium position.
View SolutionFind the amplitude of the simple harmonic motion obtasined by combining the motions
`x_1=(2.0 cm) sinomegat`
`and x_2=(2.0cm)sin(omegat+pi/3)`
View Solution`x_(1) = 3 sin omega t implies x_(2) = 4 cos omega t`. Find (i) amplitude of resultant SHm, (ii) equation of the resultant SHm.
View SolutionTwo partical `A and B` execute simple harmonic motion according to the equation `y_(1) = 3 sin omega t` and `y_(2) = 4 sin [omega t + (pi//2)] + 3 sin omega t`. Find the phase difference between them.
View SolutionIf the displacement of a moving point at any time is givenby an equation of the form `y (t) = a cos omega t + b sin omega t`, shown that the motion is simple harmonic . If `a = 3 m, b = 4m and omega = 2`: determine the period , amplitude, maximum velocity and maximum acceleration.
View SolutionIf two SHMs are repersented by `y_(1) = 10 sin (4 pi + pi//2)` and `y_(2) = 5 (sin 2 pi t +sqrt 8 cos 2 pi t)`, compare their amplitudes .
View SolutionA force `F = - 10x + 2` acts on a particle of mass `0.1 kg` where x is in m and F in newton. If is released from rest at `x = 0`, find:
a. Amplitude:
b. Time period:
c. Equation of motion.
View SolutionA person normally weighing `60 kg` stands on a plateform which oscillates up and down har monically at a frequency `2.0 sec^(-1)` and an amplitude `5.0 cm` . If a machine on the plateform gives the person's weight against time deduce the maximum and minimum reading it will shown, `Take g = 10 m//sec^(2)`.
View SolutionA spring of stiffness constant `k` and natural length `l` is cut into two parts of length `3l//4 and l//4`, respectively, and an arrangement is made as shown in figure . If the mass is slightly displaced , find the time period of oscillation.
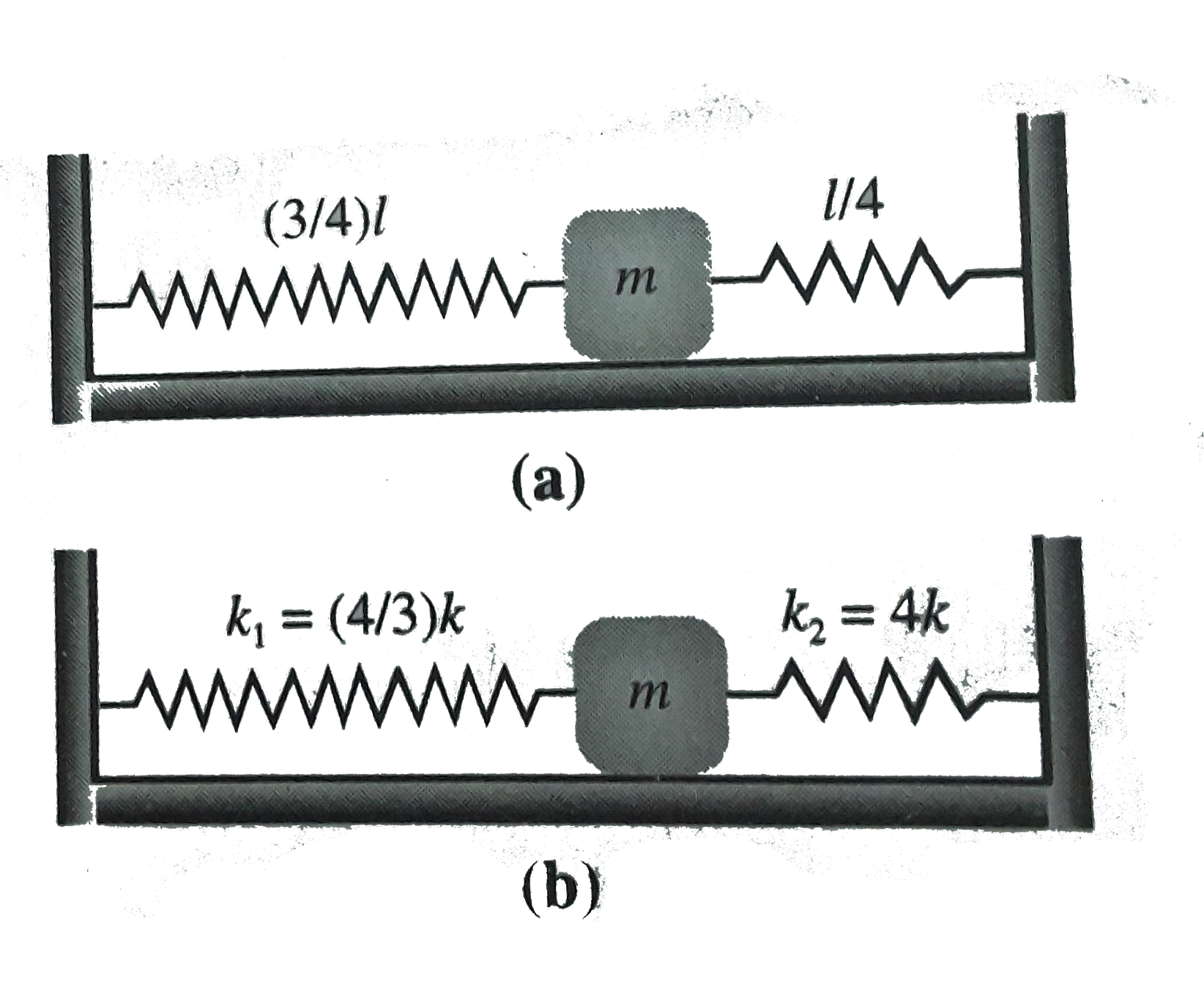
View SolutionA particle of mass `m` is attached with three springs `A,B` and `C` of equal force constancts `k` as shown in figure. The particle is pushed slightly against the spring `C` and released. Find the time period of oscillation.
 .
.
View SolutionTwo light spring of force constants `k_(1) and k_(2)` and a block of mass m are in the line AB on a smooth horizontal table such that one end of each spring is fixed on right supports and the other and is free as shown in figure
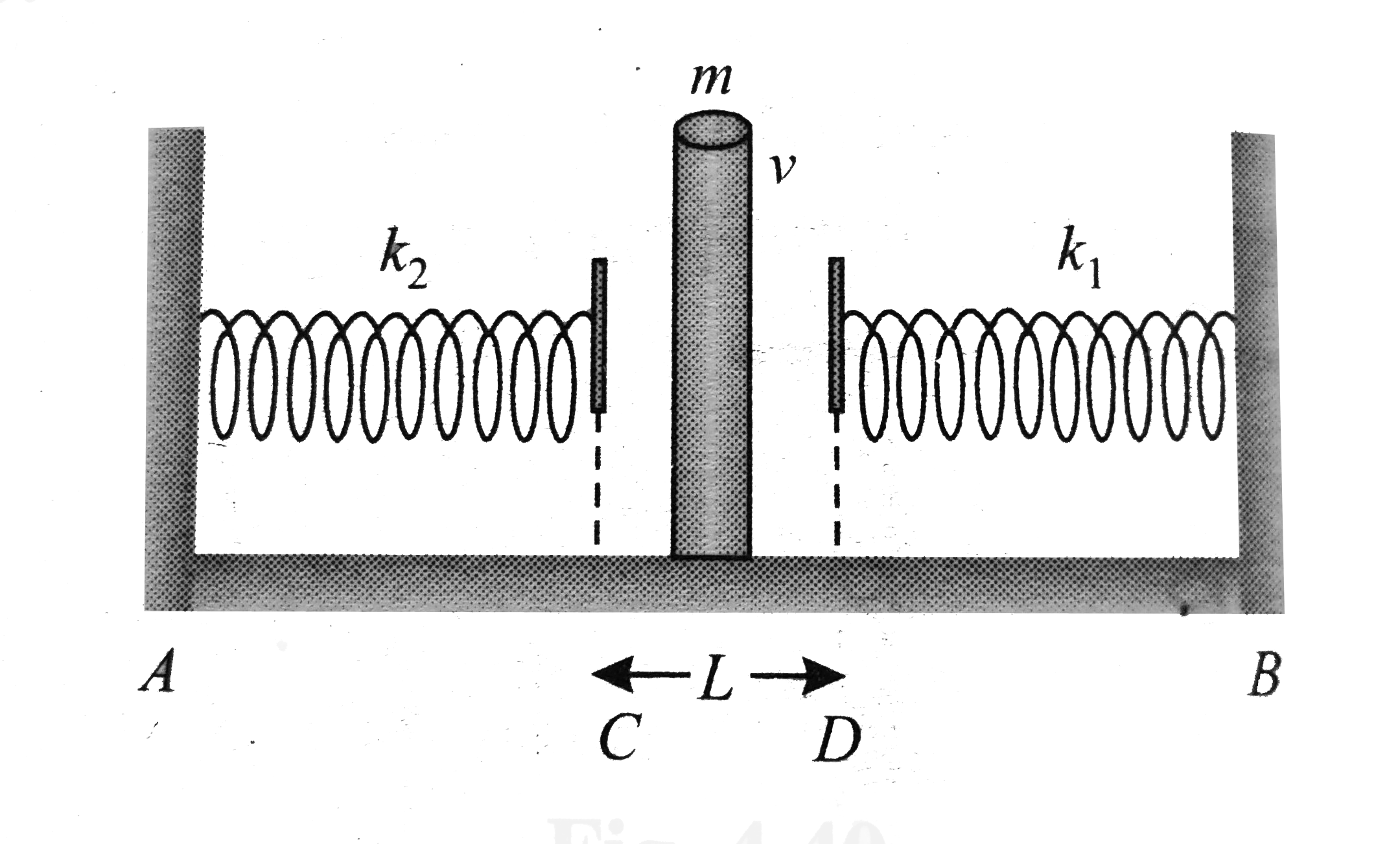
The distance CD between the free ends of the spring is `60 cm`. If the block `(k_(1) = 1.8 N//m, k_(2) = 3.2 N//m and m = 200g)`. Is the motion simple harmonic?
View Solutionfigure shown a partical mass `m = 100g` attaches with four identical spring , each of length `l = 10 cm` . Initical tension in each spring is `F_(0) = 25 N`. Neglecting gravity , Calculate the period of small obscillation of the article along a line perpenducular to the plane of the figure .

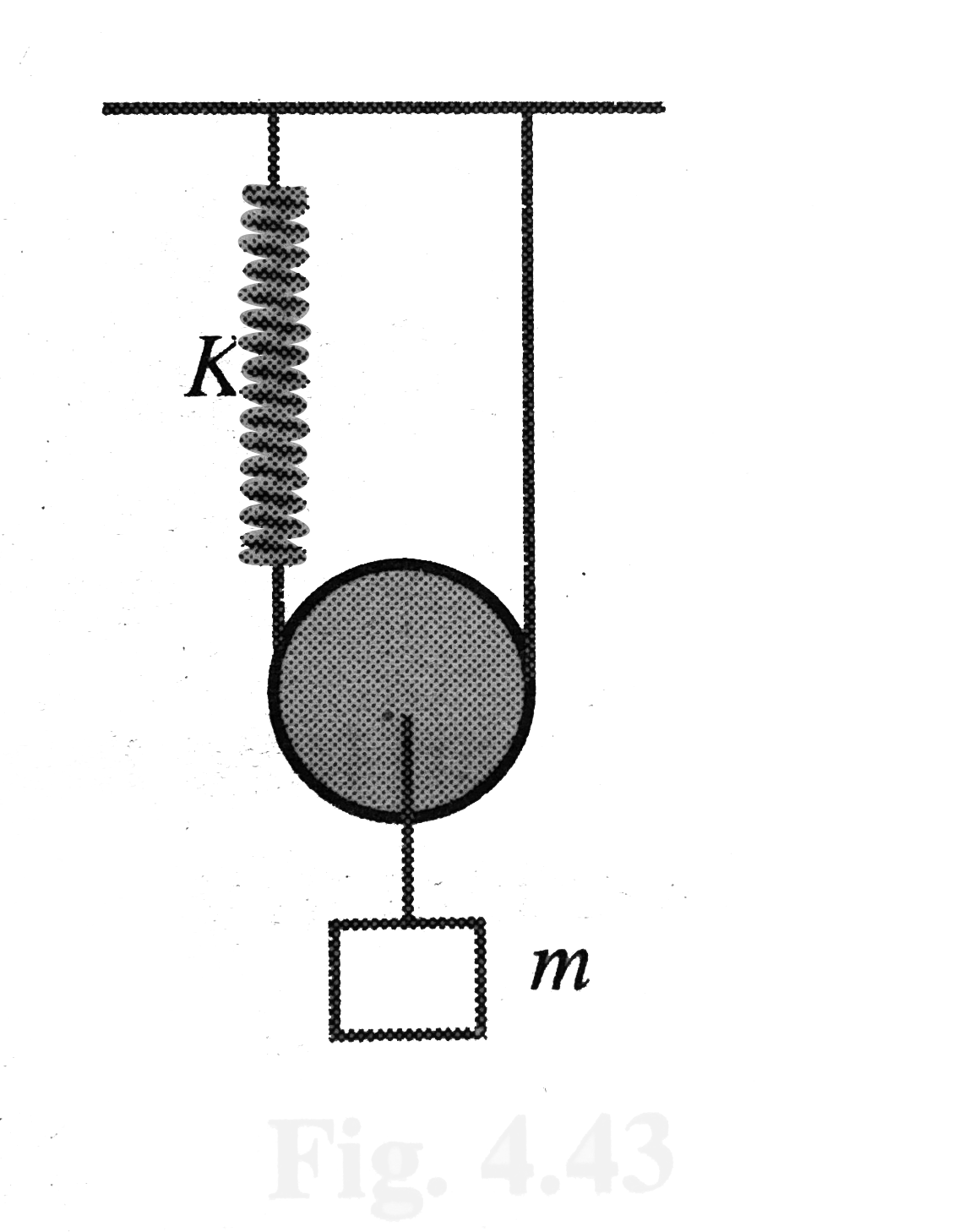
View SolutionFind the time period of `m` if pulley `P` is light and small.
View SolutionA solid cylinder of mass `m` is attached to a horizontal spring with force constant `k`. The cylinder can roll without slipping along the horizontal plane. (See the accompanying figure.) Show that the center of mass of the cylinder executes simple harmonic motion with a period `T = 2pisqrt((3m)/(2k))`, if displaced from mean position.
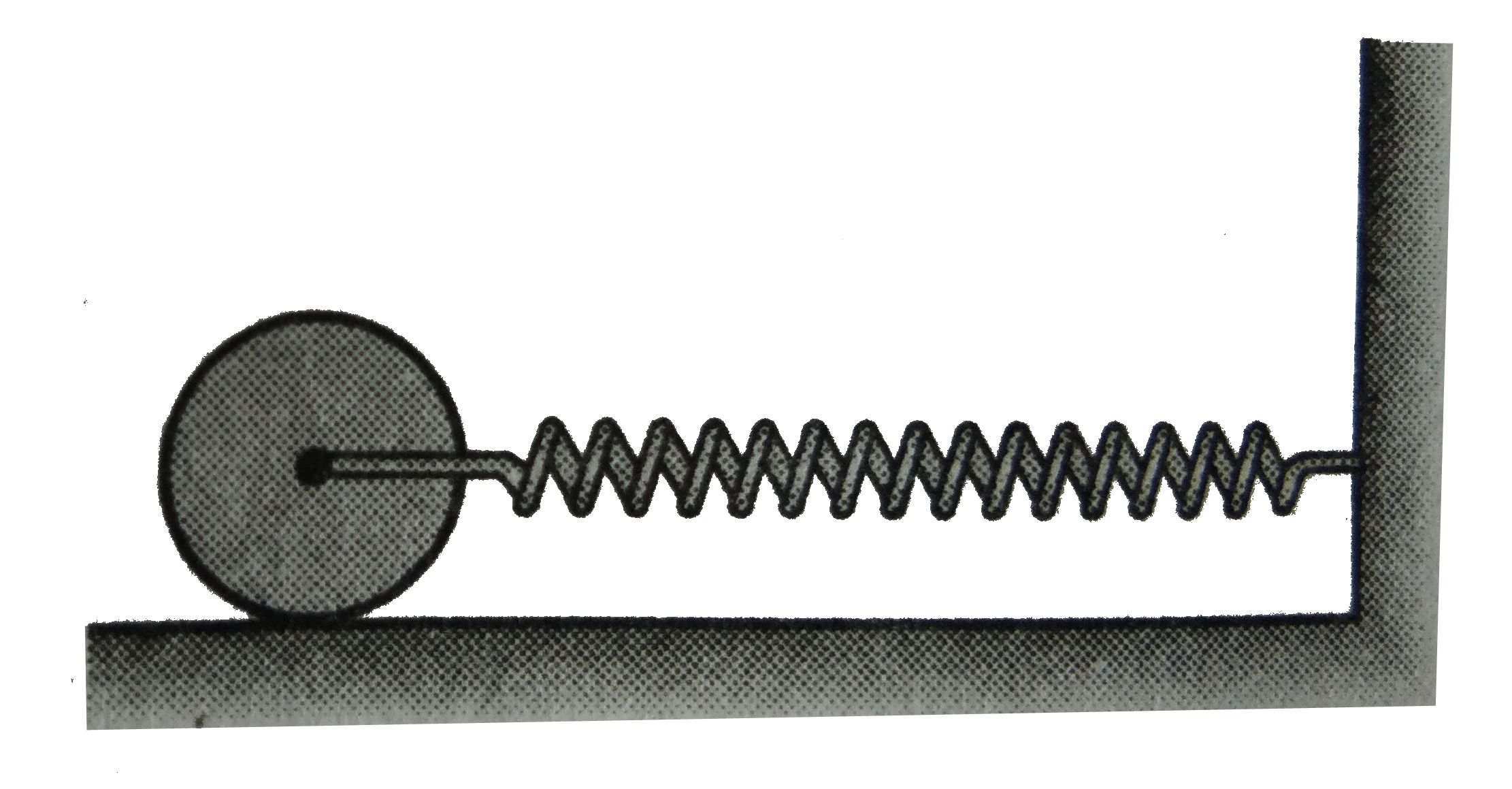
View Solutionfigure (a) and (b) represent spring- block system. If m is displacement slightly , find the time period of oscillation of the system.
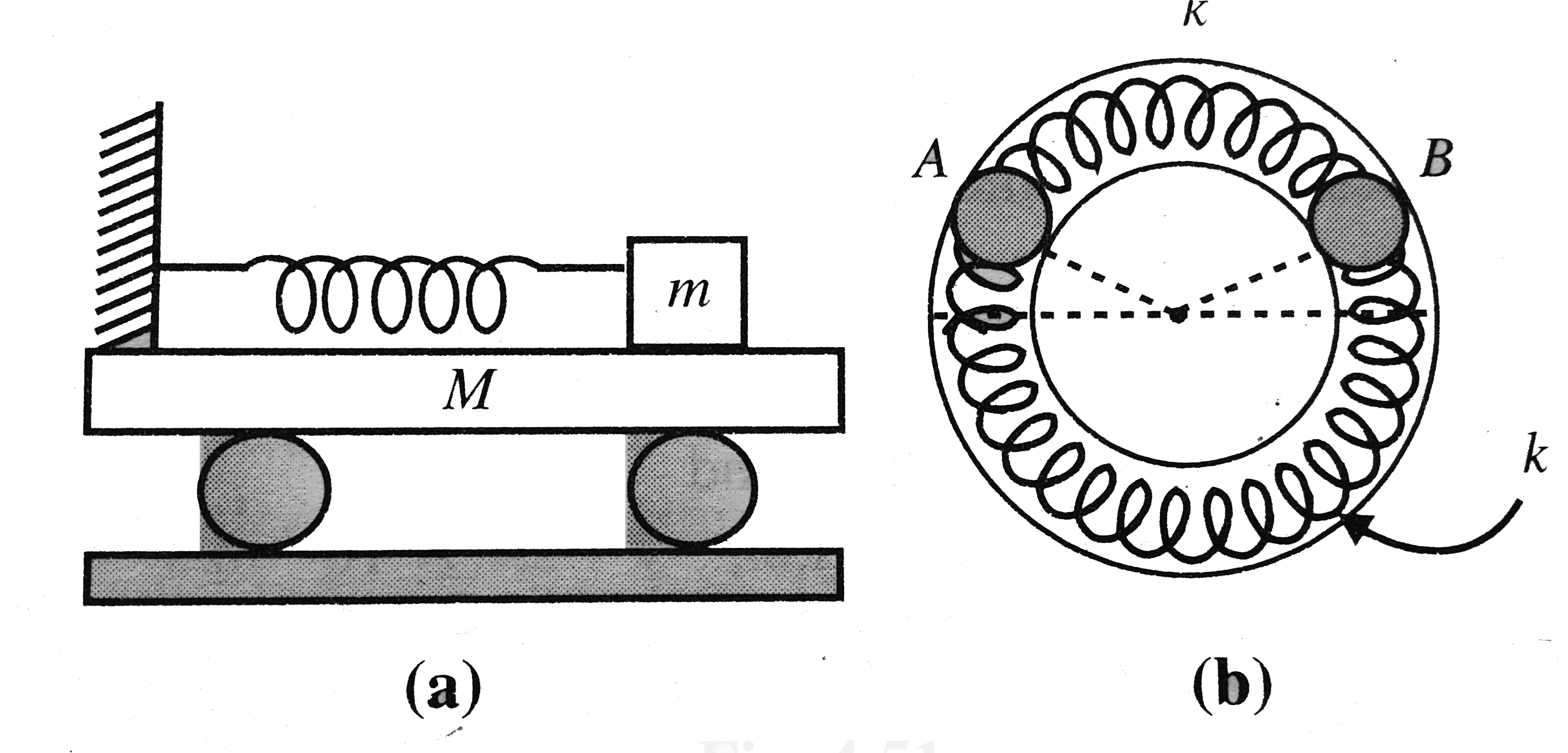
View SolutionTwo identical balls `A and B` eavh of mass `0.1 kg` are attached to two identical mass less is springs. The spring-mass system is consetrained to move inside a right smooth pipe bent in the form of a circle as shown in figure . The pipe is fixed in a horigental plane. The center of the balls can move in a circle of radius `0.06 m`. Each spring has a natural length of `0.06 pi m` and force constant `0.1 N//m`. Initially, both the balls are displaced bu an angle `theta = pi//6` dadius withrespect to diameter PQ of the circle and released from rest.
a. Calculate the frequency of oscillation of the ball B.
b. What is the total energy of the system ?
c. Find the speed of the bal A when A and B are at the two ends of the diameter PQ.

View SolutionThe system shown in the figure can move on a smooth surface. They are initially compressed by `6cm` and then released, then choose the correct options.
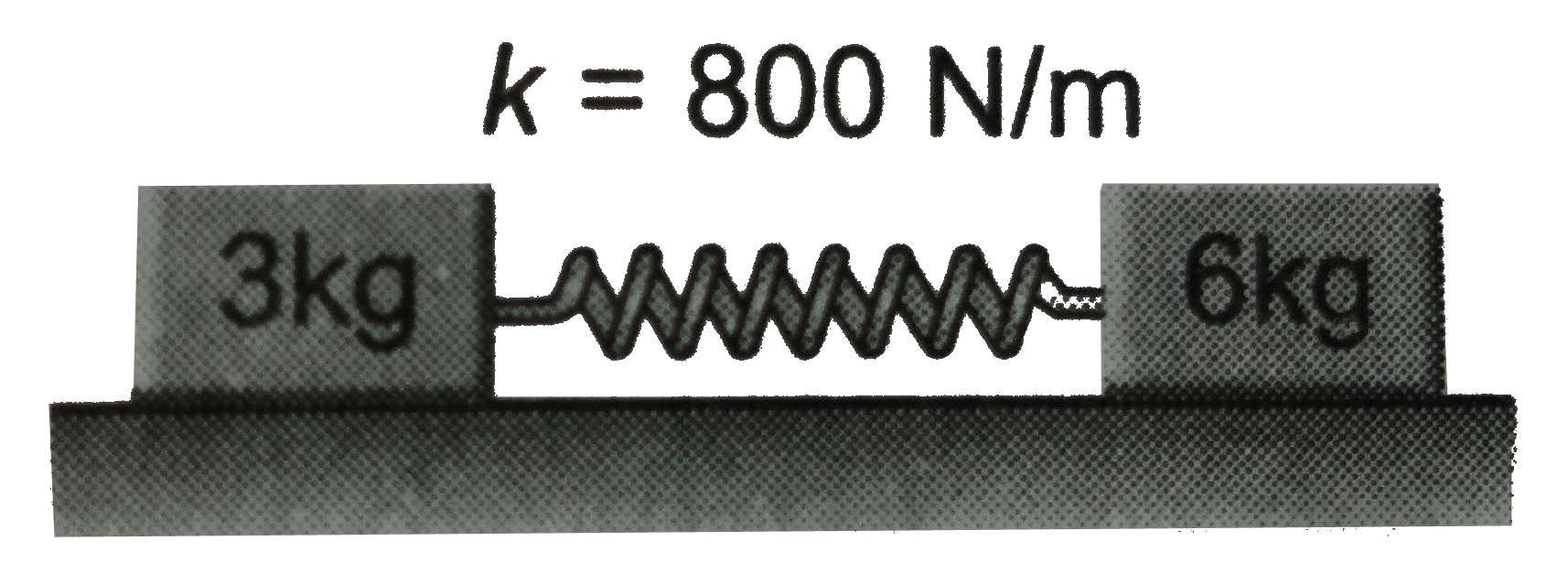
(a) The system performs, SHM with time period `(pi)/(10)s`
(b) The block of mass `3kg` perform SHM with amplitude `4 cm`
( c) The block of mass `6kg` will have maximum momentum of `2.40kg - m//s`
(d) The time periods of two blocks are in the ratio of `1:sqrt(2)`
View SolutionA simple pendulum of length 40 cm oscillates with an angular amplitude of 0.04 rad. Find a. the time period b. the linear amplitude of the bob, c. The speed of the bob when the strig makes 0.02 rad with the vertical and d. the angular acceleration when the bob is in moemntary rest. Take `g=10 ms^-2`.
View SolutionA ball is suspended by a thread of length l at the point O on an incline wall as shown. The inclination of the wall with the vertical is (a)the thread is displacement through a small angle away from the vertical and (b) the ball is released. Find the period of oscillation of pendulum. Consider both cases
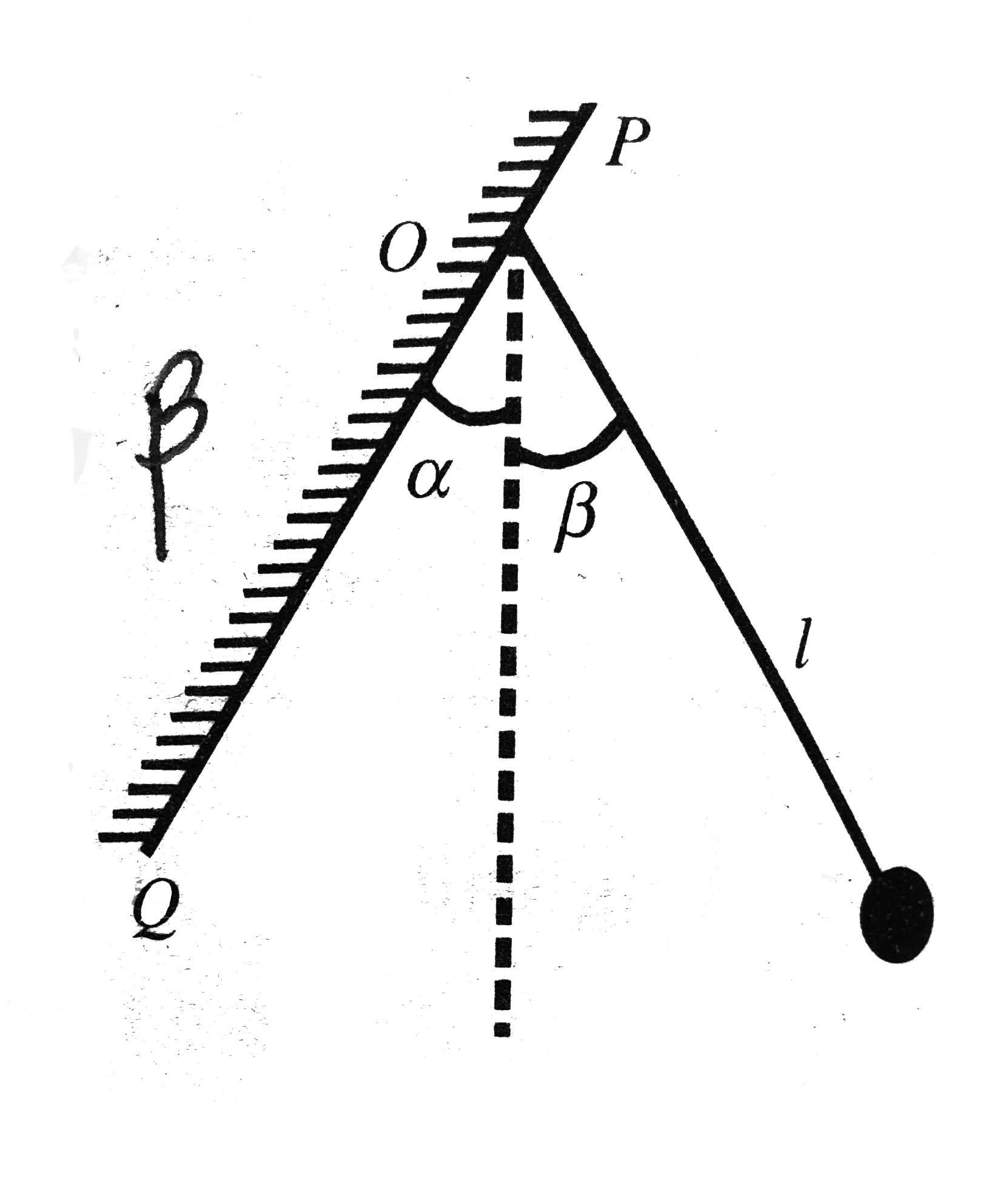
a. `alpha gt beta`
b. `alpha lt beta`
Assuming that any impact between the wall and the ball is elastic.
View Solutionfigure show the identical simple pendulums of length. One is tilled at an angle `alpha` and imparted an initial velocity `v_(1)` towards mean position and at a velocity `v_(2)` at an initial angular displacement `beta`. Find the phase difference in oscillations of these two pendulums
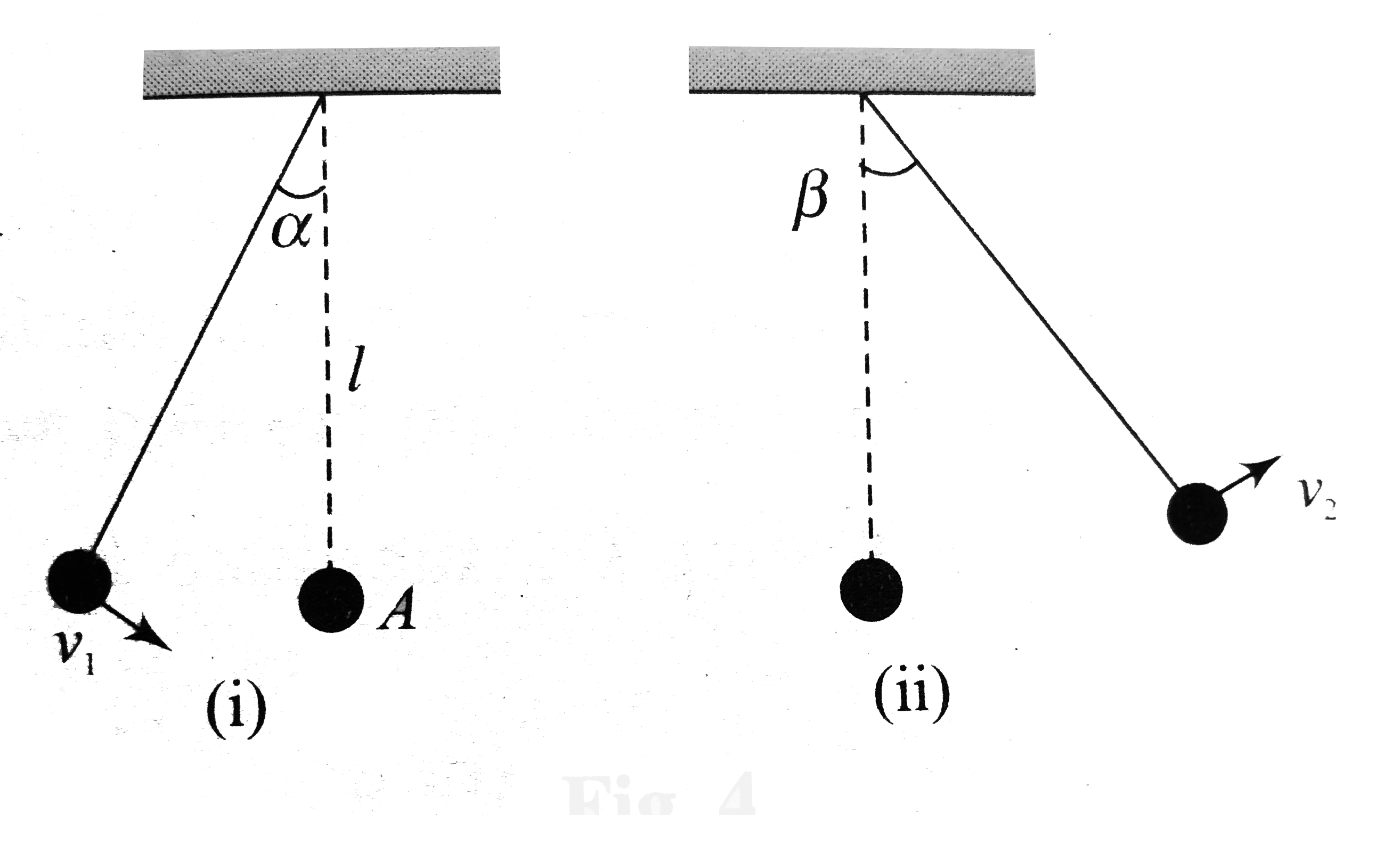
View SolutionIn previous question, if the second pendulum bob is thrown at velocity `v_(2)` at an angle `beta` from mean position, but on other side of mean position , find the phase diffrence in the two SHMs now as show in the figure.

View SolutionDerive an expression for the angular frequency of small oscillation of the bob of a simple pendulum when it is immersed in a liquid of density `rho`. Assume the density of the bob as `sigma` and length of the string as `l`.
View SolutionWhat is the period of a pendulum formed by pivoting a meter stick so that is free to rotate about a horizontal axis passing through the `75 cm` mark?
View SolutionA uniform of radius `5.0 cm` and mass `200 g` is fixed at its centre to a metal wire , the other end of which is fixed to a celling. The hanging disc is rotate about the wire through an angle and is released. If the disc makes torsional oscillations with time period `0.20 s` , find the torsional constant of the wire.

View SolutionAssume that a tunnel is dug across the earth (radius=R) passing through its centre. Find the time a particle takes to cover the length of the tunnel if (a) it is projected into the tunnel with a speed of `sqrt((gR)` (b) it is released from a height R above the tunnel (c ) it is thrown vertically upward along the length of tunnel with a speed of `sqrt(gR).`
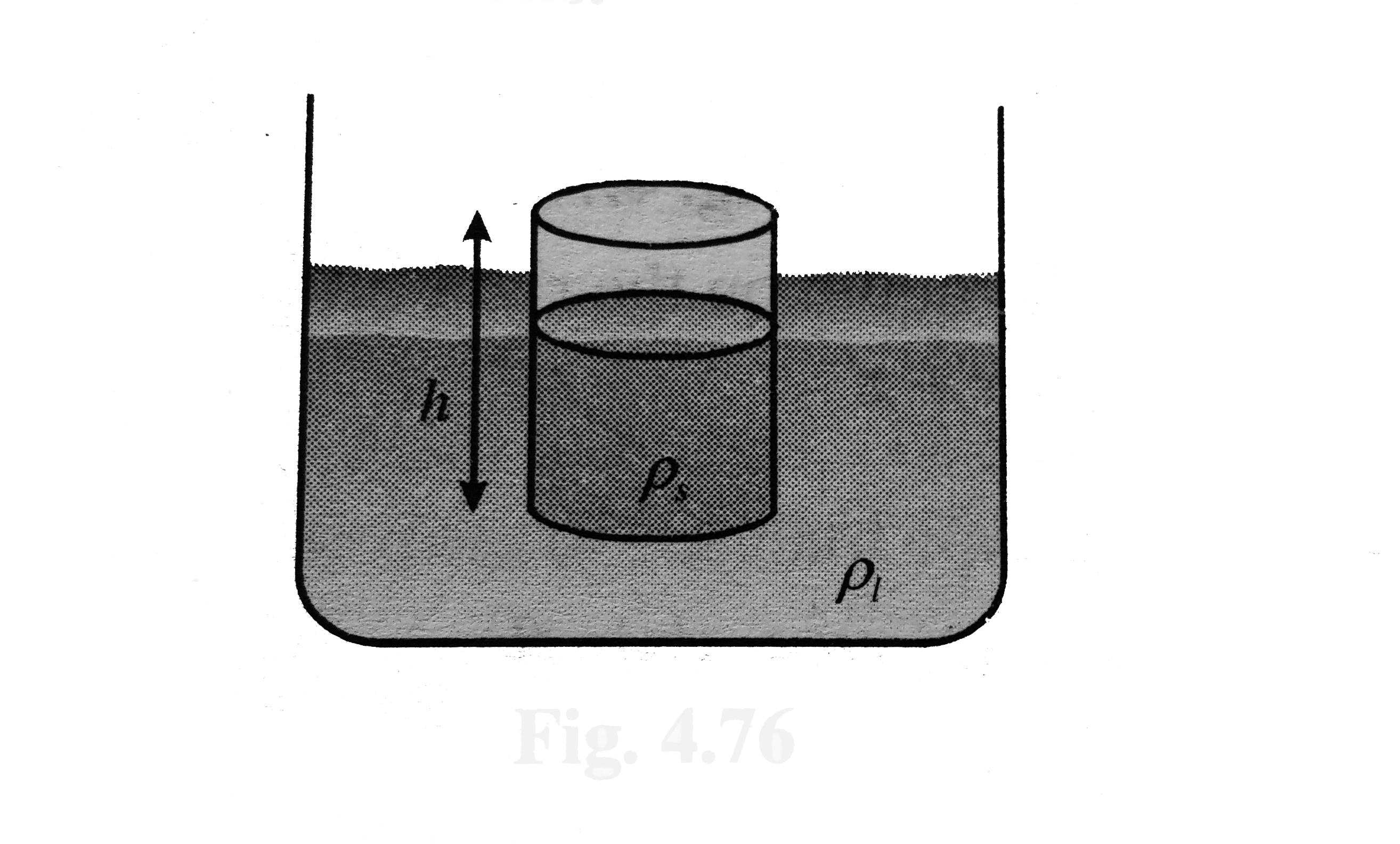
View SolutionConsider a solid cylinder of the density `rho_(s)` cross section area A and h ploating in a liquid of density `rho_(l)` as shown in figure `(rho_(l) gt rho_(s))` . It is depressed sligtly and allowed to oscillation.
View SolutionA V-shaped glass tube of uniform cross section is kept in a vertical plane as shown. A liquid is poured in the tube. In equilibrium the level of liquid in both limbs of tube are equal. Find the angular frequency of small oscillation of liquid.

View SolutionFind the amplitude and initial phase of a partical in SHM, whose motion equation is given as
`y = A sin omega t + B cos omega t`
View SolutionTwo simple harmonic motion are represent by the following equations
`y_(1) = 10 sin (pi//4) (12 t + 1)`
`y_(2) = 5 (sin 3 theta t + sqrt3 cos 3 theta t)`
Here `t` is in seconds.
Find out the ratio of their amplitudes.What are the time period of the two motion?
View Solution
Illustration questions and complete solutions for chapter LINEAR AND ANGULAR SIMPLE HARMONIC MOTION of CENGAGE PHYSICS (HINGLISH) of Class 11