Complete Solutions to Subjective of chapter LINEAR AND ANGULAR SIMPLE HARMONIC MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | LINEAR AND ANGULAR SIMPLE HARMONIC MOTION |
| CENGAGE PHYSICS | Subjective |
| Number Of Questions | 21 |
| Academic Year | 2023-24 |
A rigid rod of mass m with a ball of mass M attached to the free end is restrained to oscillate in a vertical plane as shown in the figure. Find the natural frequency of oscillation.
 09:06View Solution
09:06View SolutionA rectangular tank having base `15 cm xx 20 cm` is filled with water (density `rho = 1000 kg//m^(2))` up to `20 cm` and force constant `K = 280 N//m` is fixed to the bottom of the tank so that the spring remains vertical.
This system is in an elevator moving downwards with acceleration `a = 2 m//s^(2)`. A cubical block of side `l = 10 cm` and mass `m = 2 kg` is gently placed over the spring and released gradually, as shown in the figure.
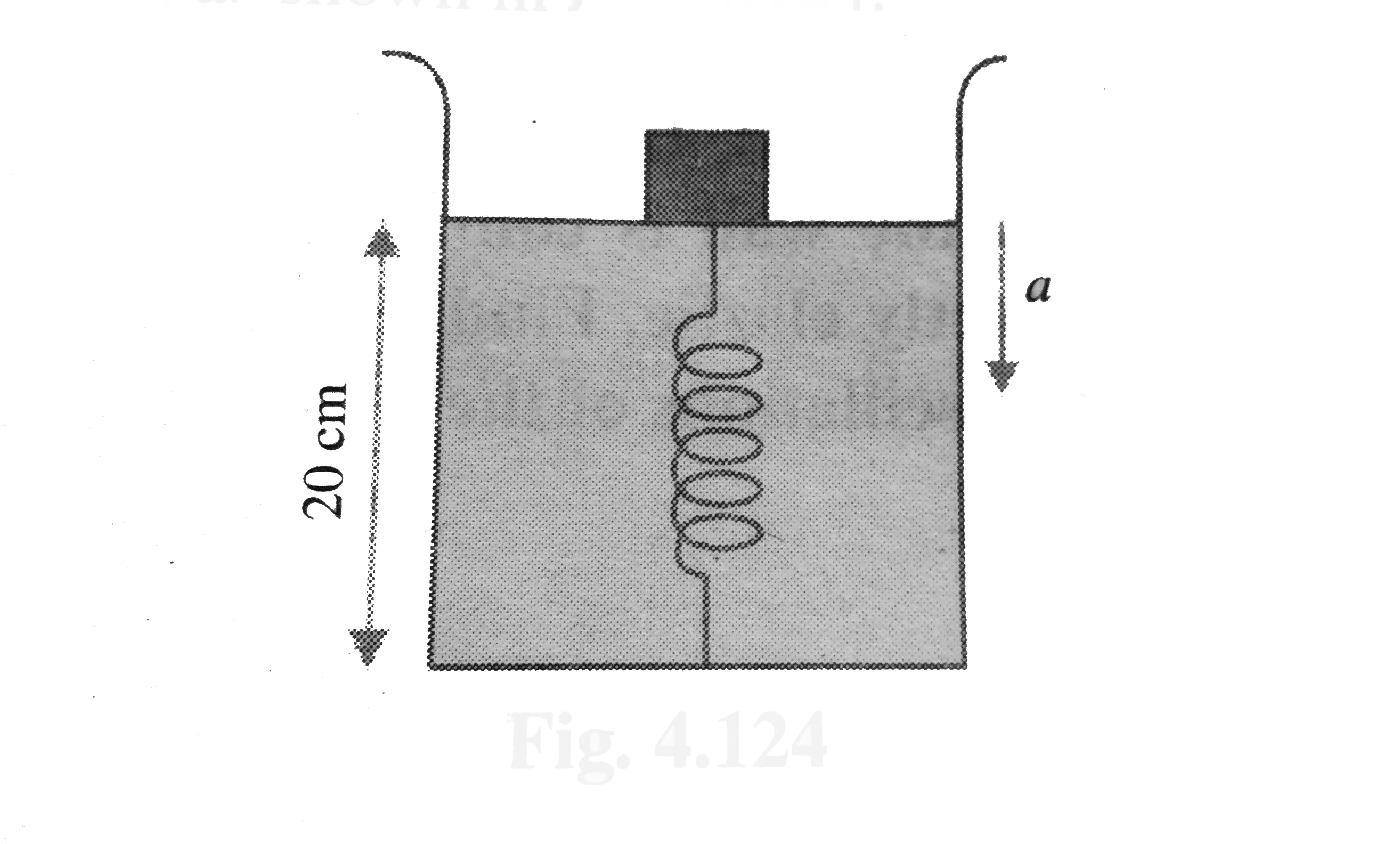
a. Calculate compression of the spring in equilibrium position.
b. If the block is slightly pushed down from equilibrium position and released, calculate frequency of its vertical oscillations.14:08View SolutionA body A mass `m_(1) = 1 kg` and body B of mass `m_(2) = 4.1 kg`. The body A perform free vertical harmonic oscillations with the amplitude `1.6 cm` and frequency `25 Hz`. Neglecting the mass of the spring , find the maximum and m inimum value of force that the system exerts on the hearing surface.
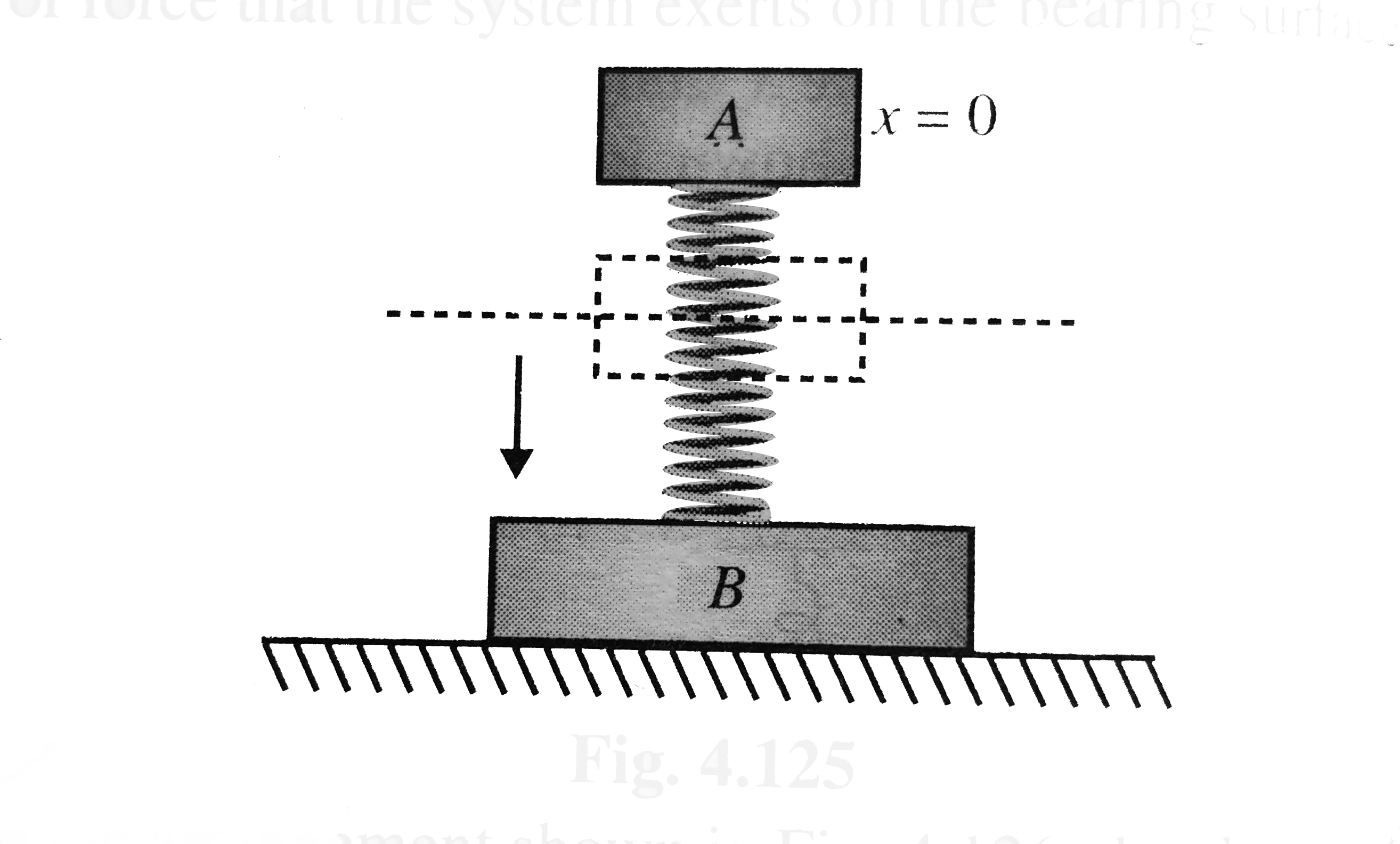 07:54View Solution
07:54View SolutionIn the arrangement shown in figure the sleeve `M` of mass `m=0.20kg ` is fixed between two identical springs whose combined stiffness is equal to `x=20 N//m`. The sleeve can slide without friction over a horizontal bar `AB`. The arrrangement rotates with a constant angular velocity `omega=4.4 rad//s` about a vertical axis passing through the middle of the bar . Find the period of small oscillations of the sleeve. At what values of `omega` will there be no oscillations of the sleeve ?
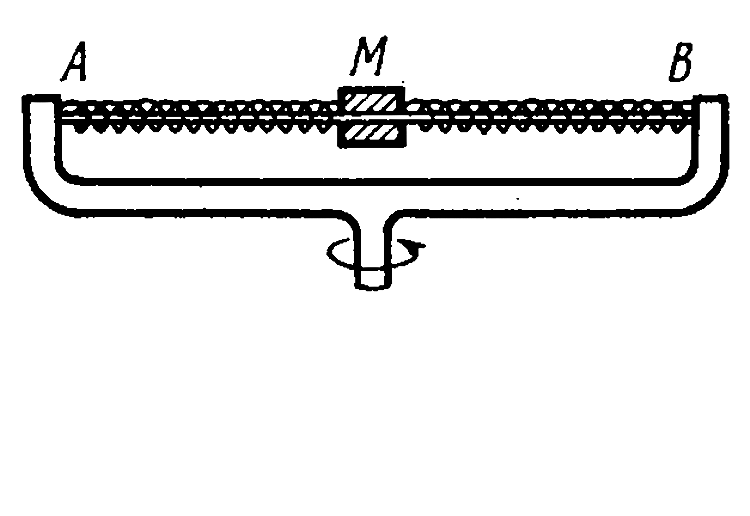 06:34View Solution
06:34View SolutionA vertical pole of length `l` , density `rho` , area of cross section A floats in two immiscible liquids of densities `rho_(1)`and `rho_(2)`. In equuilibrium possition the bottom end is at the interface of the liquids. When the cylinder is displaced vertically, find the time period of oscillation.
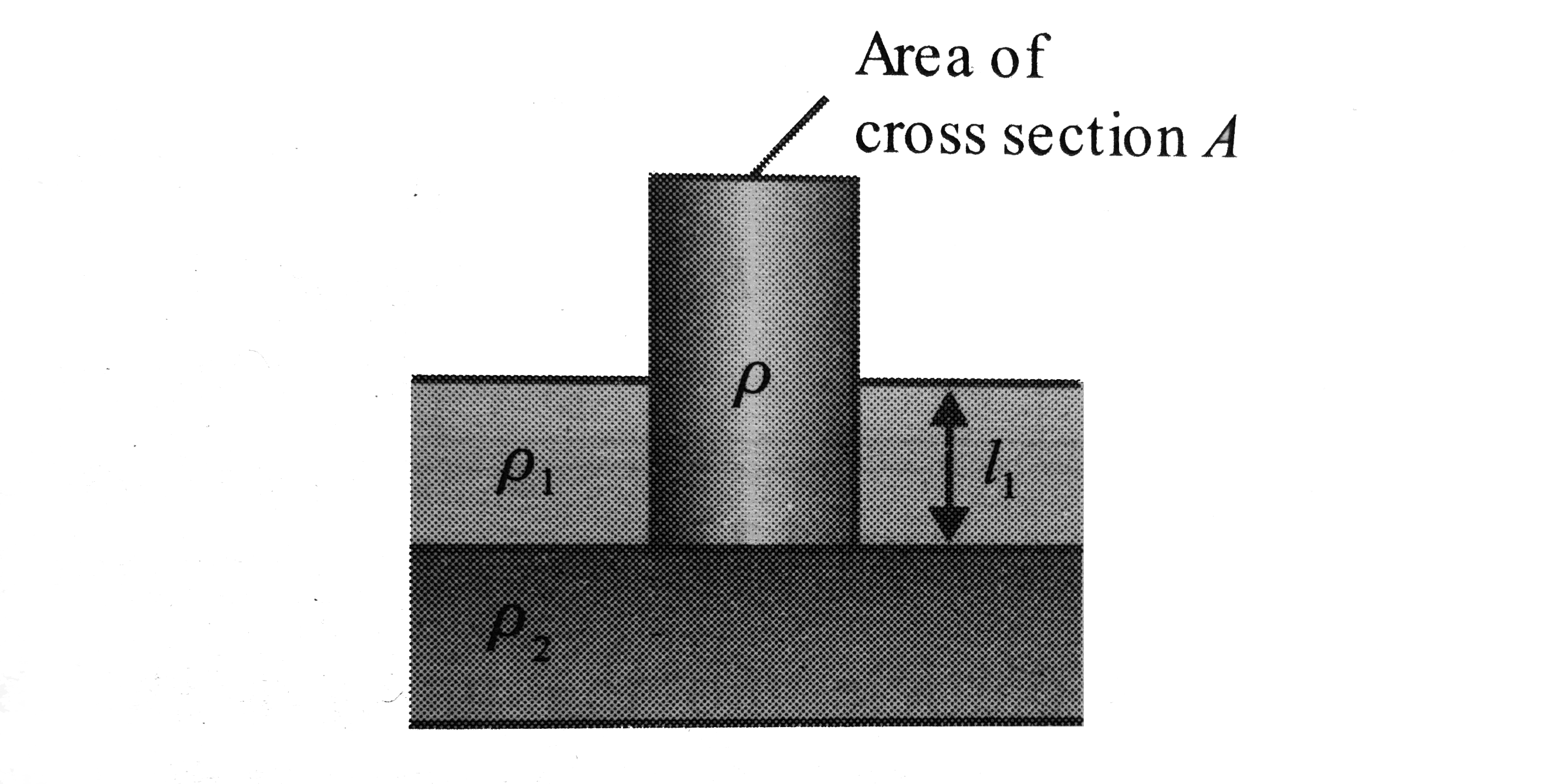 09:37View Solution
09:37View SolutionIn the shown arrangement, both the spring are in their natural lengths. The coefficient of friction between `m_(2)` and `m_(1)` is `mu`. There is no friction between `m_(1)` and the surface. If the blocks are displaced slightly, they together perform simple harmonic motion. Obtain
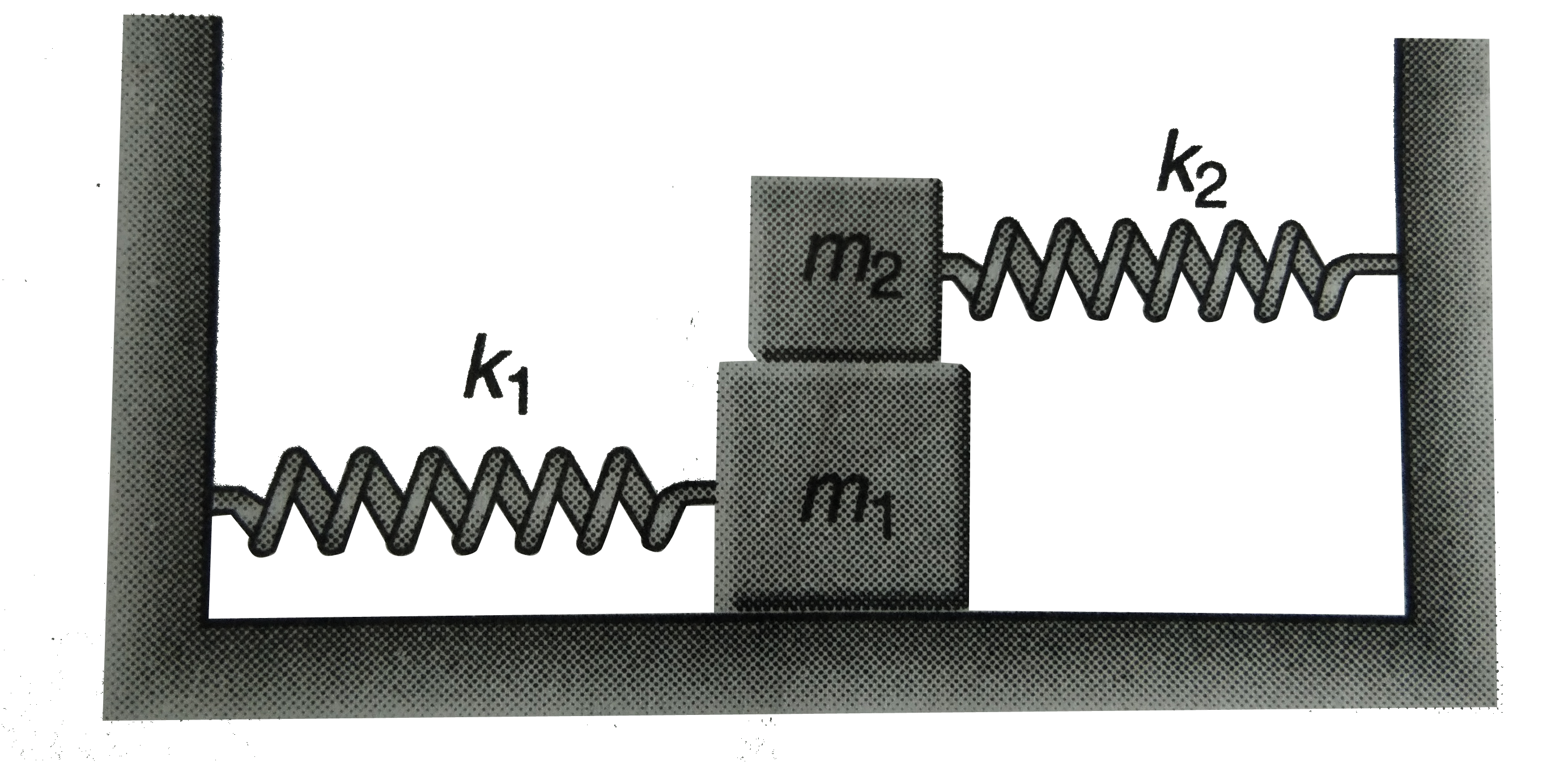
(a) Frequency of such oscillations.
(b) The condition if the friction force on clock `m_(2)` is to act in the direction of its displacement from mean position.
( c) If the condition obtained in (b) is met, what can be maximum of their oscillations ?08:04View SolutionA uniform dise of mass `m` and radius `R` is connected with two light springs `1` and `2`. The springs are connected at the highest point `M` and the `CM` 'N' of the dise. The other ends of the springs are rigidly attached with vertical walls. If we shift the `CM` in horizontalby a small distance , the discoscillates simple harmonically. Assuming a perfect rolling of the dise on the horizontal surface , find the angular frequency of oscillation.
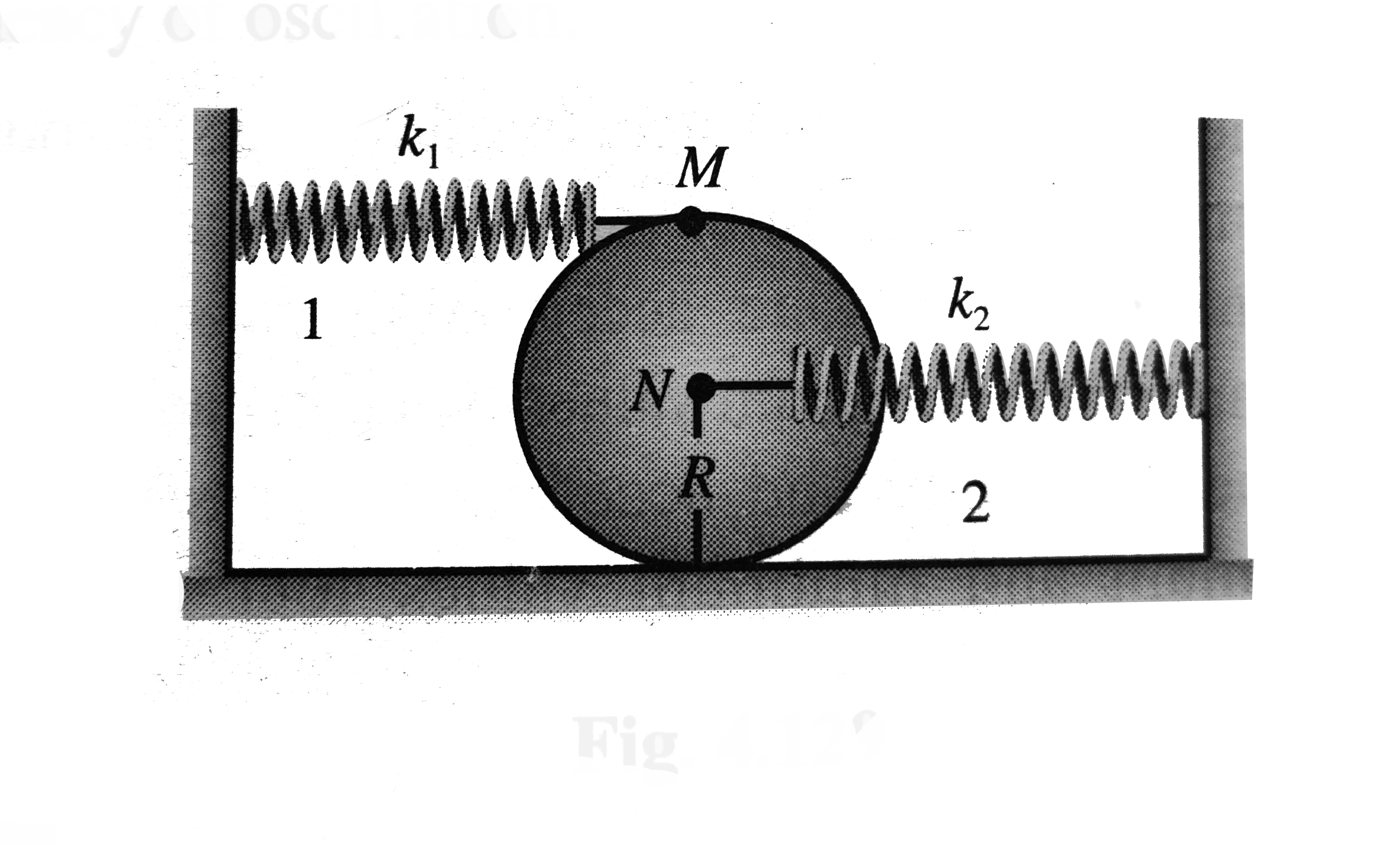 06:28View Solution
06:28View SolutionConsider a liquid which fills a uniform `U` - tube uniform `U`- tube, as shown in figure , up to a height `h`. Find angular the frequency of small oscillation of th eliquid in the `U` - tube.
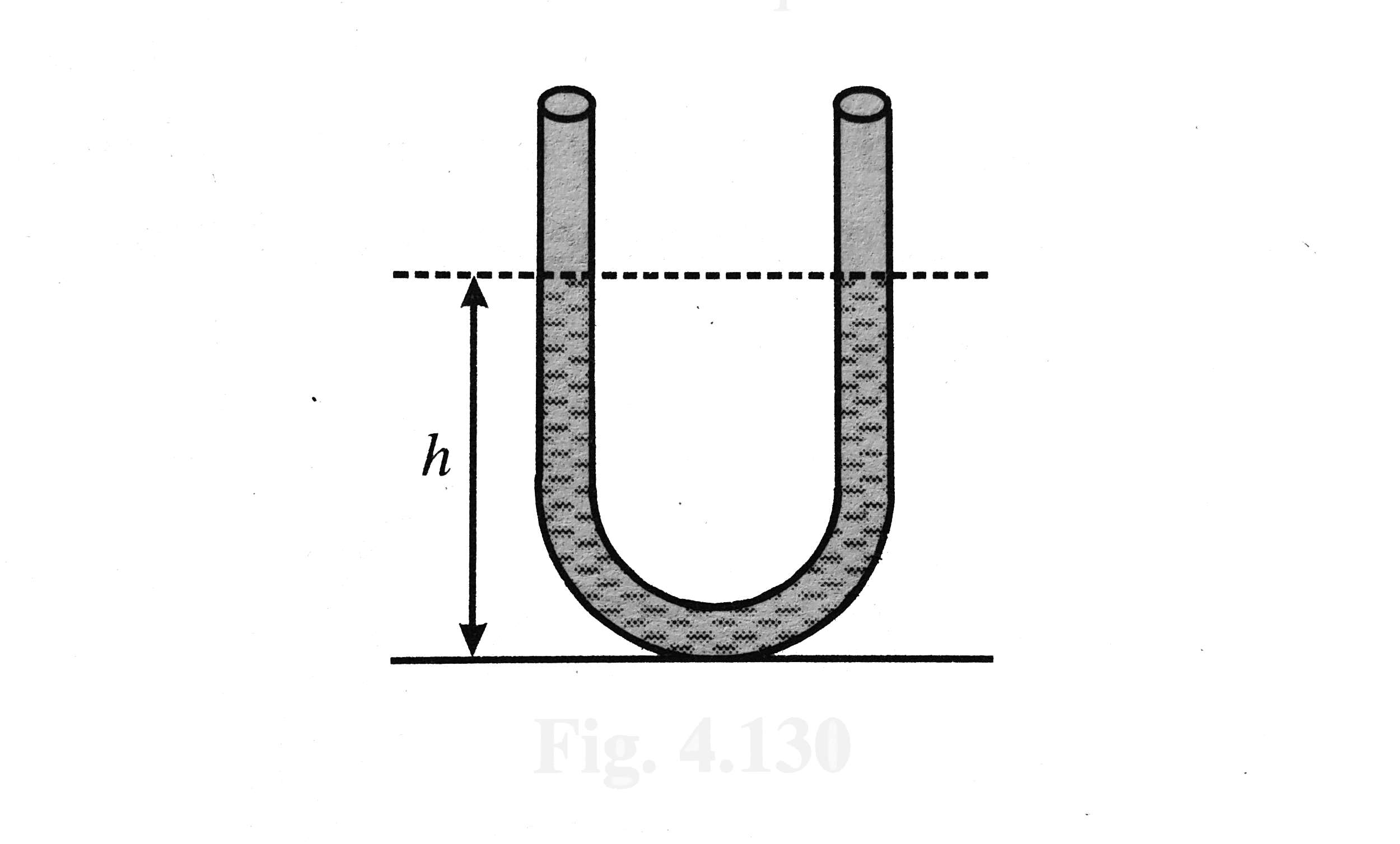 06:04View Solution
06:04View SolutionA particle of mass `m` is located in a unidimensional potential field where potential energy of the particle depends on the coordinates `x as U (x) = (A)/(x^(2)) - (B)/(x)` where `A` and `B` are positive constant.
Find the time period of small oscillation that the particle perform about equilibrium position.09:47View SolutionA particle of mass `2 kg` is moving of a straight line under the actin force `F = (8 - 2x)N`. It is released at rest from `x = 6m`.
a. Is the partical moving simple harmonically.
b.Find the equilibrium position of the particle.
c. Write the equation of motion of the partical.
d. Find the time period of SHM.04:23View SolutionA body of mass `m` hangs from a smooth fixed pulley `P_(1)` by the inextensible string fitted with the springs of stiffness `k_(1)` and `k_(2)` . The string passes over the smooth light pulley `P_(2)` which is connected with another ideal spring of stiffness `k_(2)`. Find the period of oscillation of the body.
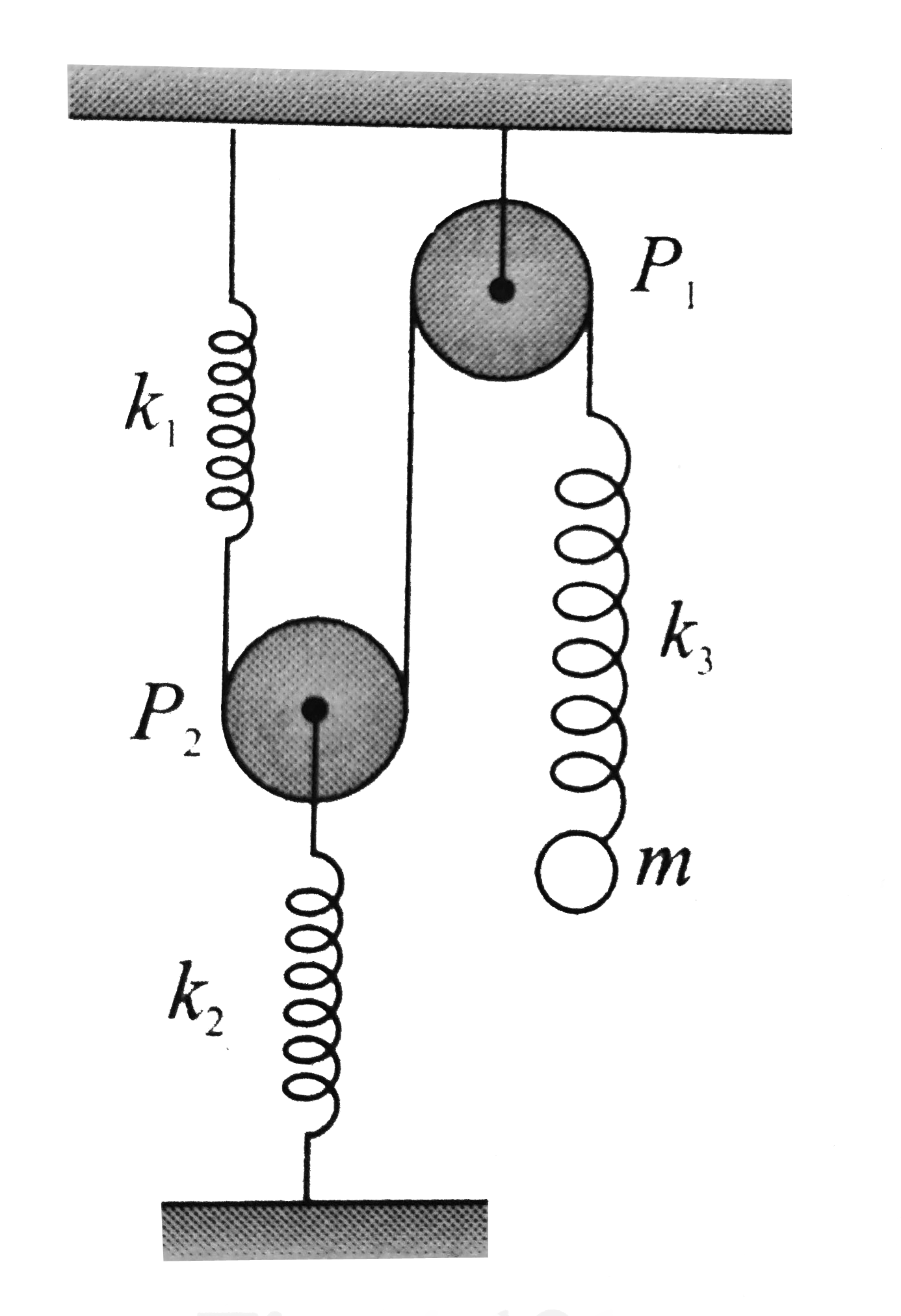 11:02View Solution
11:02View SolutionA block of mass `m` connected with a smooth prismatic wedge of mass m is released from rest when the spring is relaxed. Find the angular frequency of oscillation.
 14:18View Solution
14:18View SolutionA stepped pulley having mass `m` redius of gyration `k` is connected with two ideal springs of stiffnesws `k_(1)` and `k_(2)` as shown in figure. If the pulley shown in the figure . If the pulley rolls without sliding, find the angular frequency of its oscillation.
 08:24View Solution
08:24View SolutionA stepped dies of mass `M` and radius `R` is pivoted at its center `C` smoothly. An inextensible string connected with a light spring of stiffness `k` passes over the pulley. One end of the string is rigidly connected with the ground and the other end is attached to a body of mass `m` . If the string does not slide on the pulley, find the angularfrequency of oscillation of the syytem
 06:23View Solution
06:23View SolutionA disc of mass `m` hanged by a string is attached at `P` and a spring of stiffness `k` is attached at `O`. Find the frequency of small angular oscillation of the disc if the string does not slide over the pulley. Assume `l_(0) = Ml` of the disc about `O`.
 05:56View Solution
05:56View SolutionA uniform cylinder of length (L) and mass (M) having cross sectional area (A) is suspended, with its length vertical, from a fixed point by a massless spring, such that it is half - submerged in a liquid of density (rho) at equilibrium position. When the cylinder is given a small downward push and released it starts oscillating vertically with small amplitude. If the force constant of the spring is (k), the prequency of oscillation of the cylindcer is.
04:24View SolutionDisregading gravity, find the period of oscillation of the partical connected with four springs as shown in the figure.
`(given : theta = 45^(@). Beta = 30^(@))`
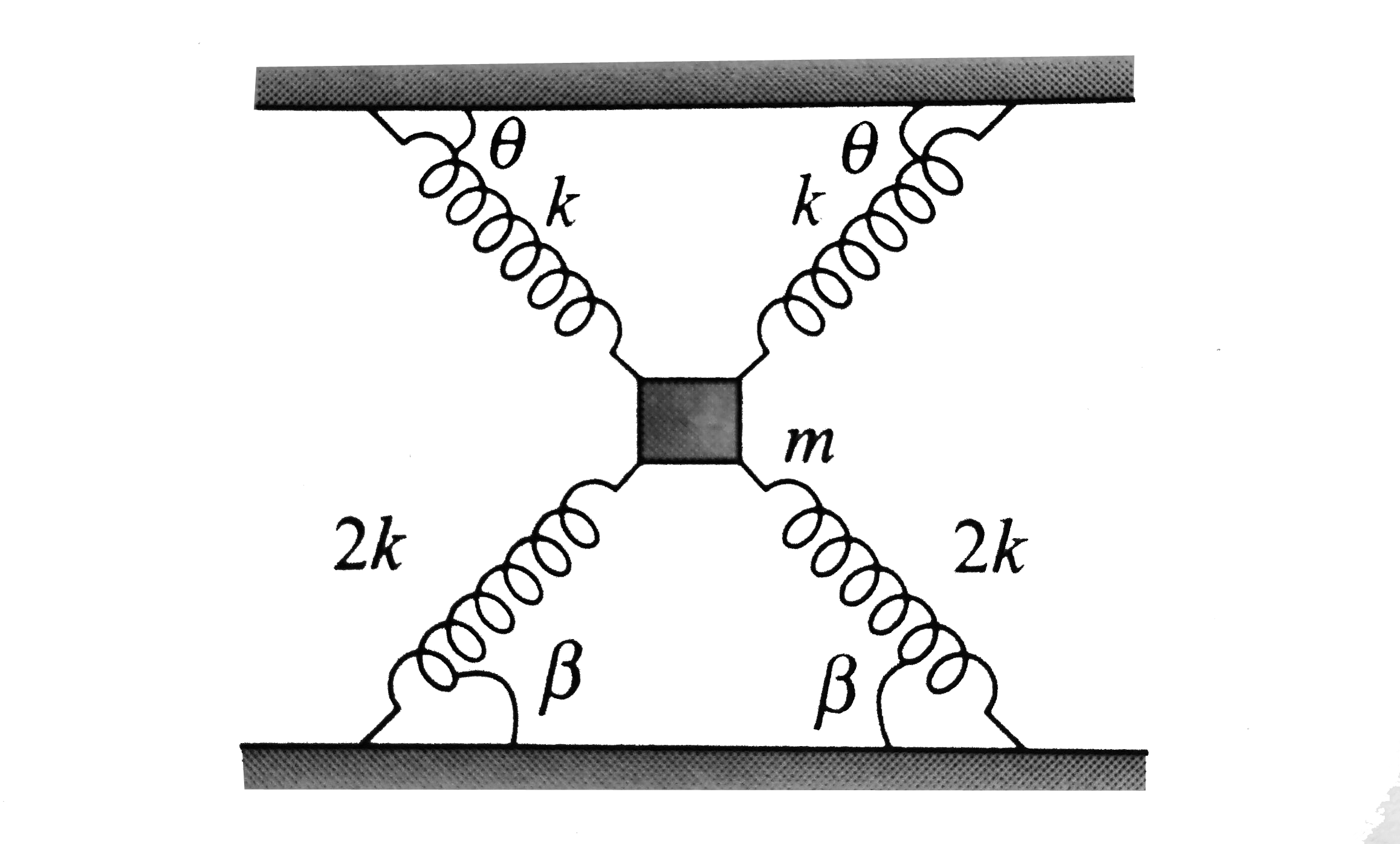 09:10View Solution
09:10View SolutionA smooth of mass `m_(1)` is lying on a rigid horizontal string A bob of mass `m_(2)` hangs from, the ring by an inextensible light string of length `l`. Find angular frequency of oscillation of the system.
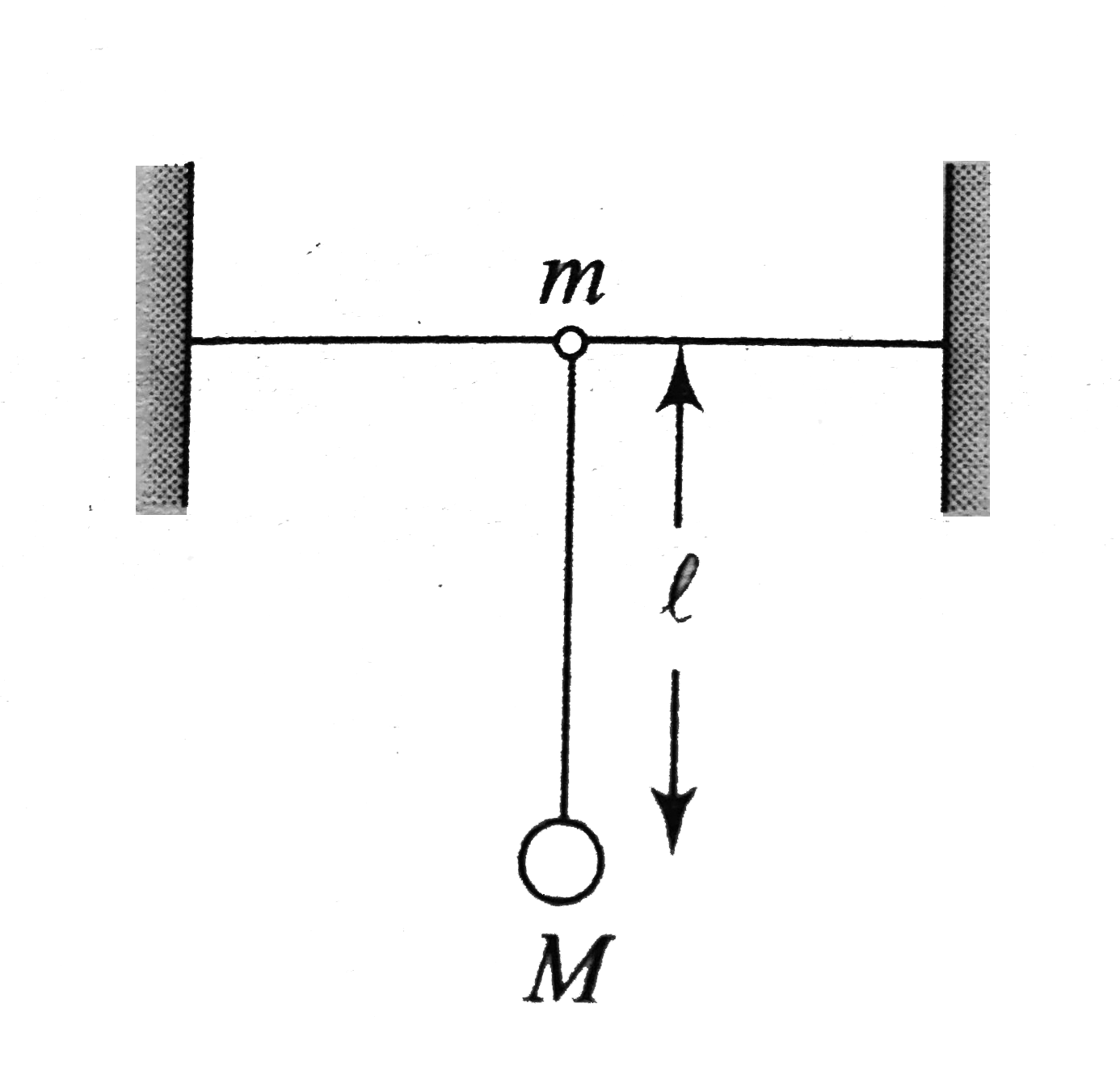 03:45View Solution
03:45View SolutionA smooth piston of mass `m` area of cross - section A is in equilibrium in a gas jar when the pressure of the gas is `P_(0)`. Find the angular frequency of oscillation of the piston, assuming adiabatic Change of state of the gas.
 07:12View Solution
07:12View SolutionIf velocity of a partical moving along a straight line changes sinusoidally with time as shown in given graph. Find the average speed over time interval `t = 0` to `t= 2 (2 n - 1)` second, `n` being any positive interget.
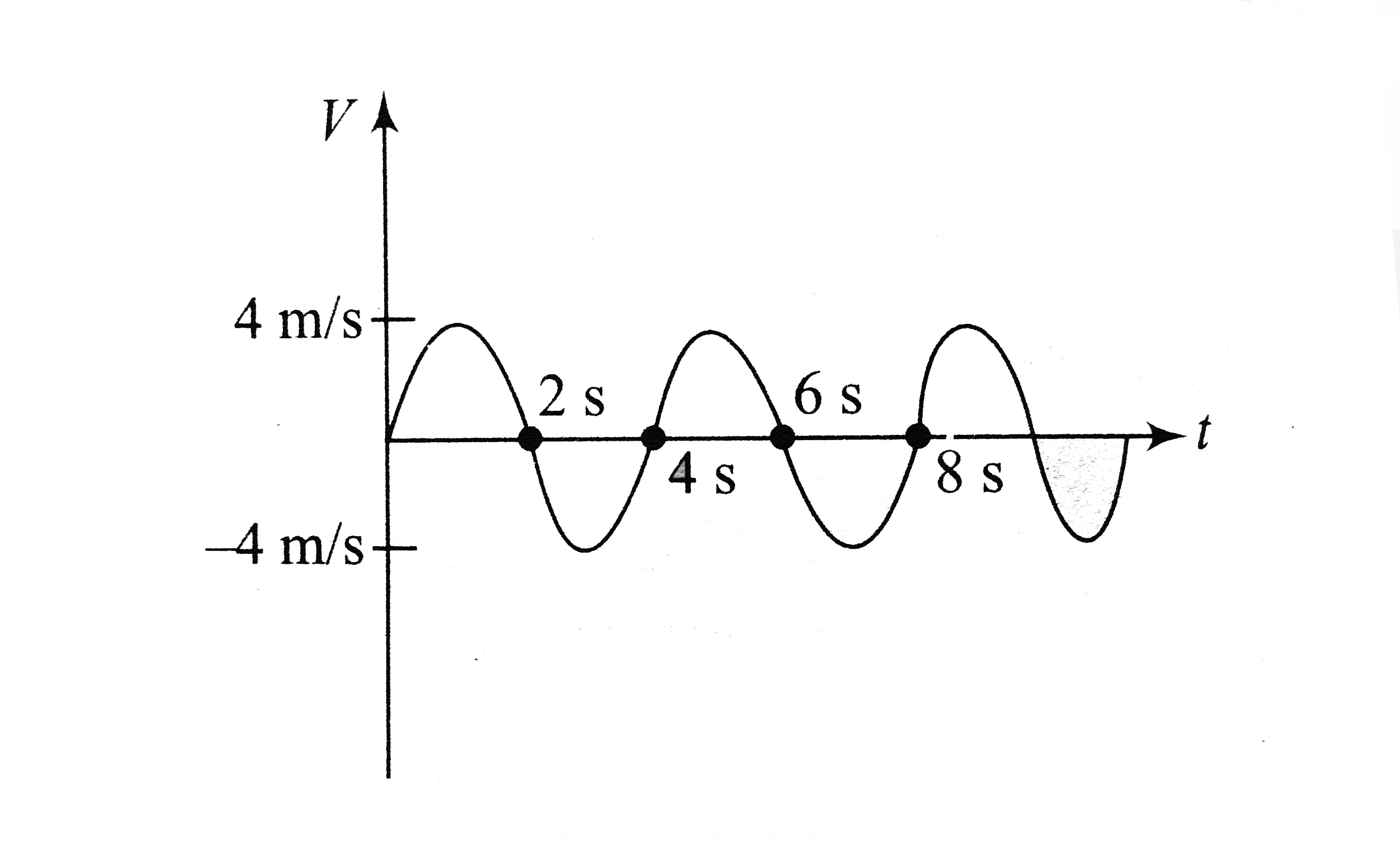 04:14View Solution
04:14View SolutionIn the figure shown, mass `2m` connected with a spring of force constant `k` is at rest and in equilibrium. A partical of mass `m` is released from height `4.5 mg//k` from `2m` . The partical stick to the block. Neglecting the duration of collision find time from the release of `m` to the moment when the spring has maximum compression.
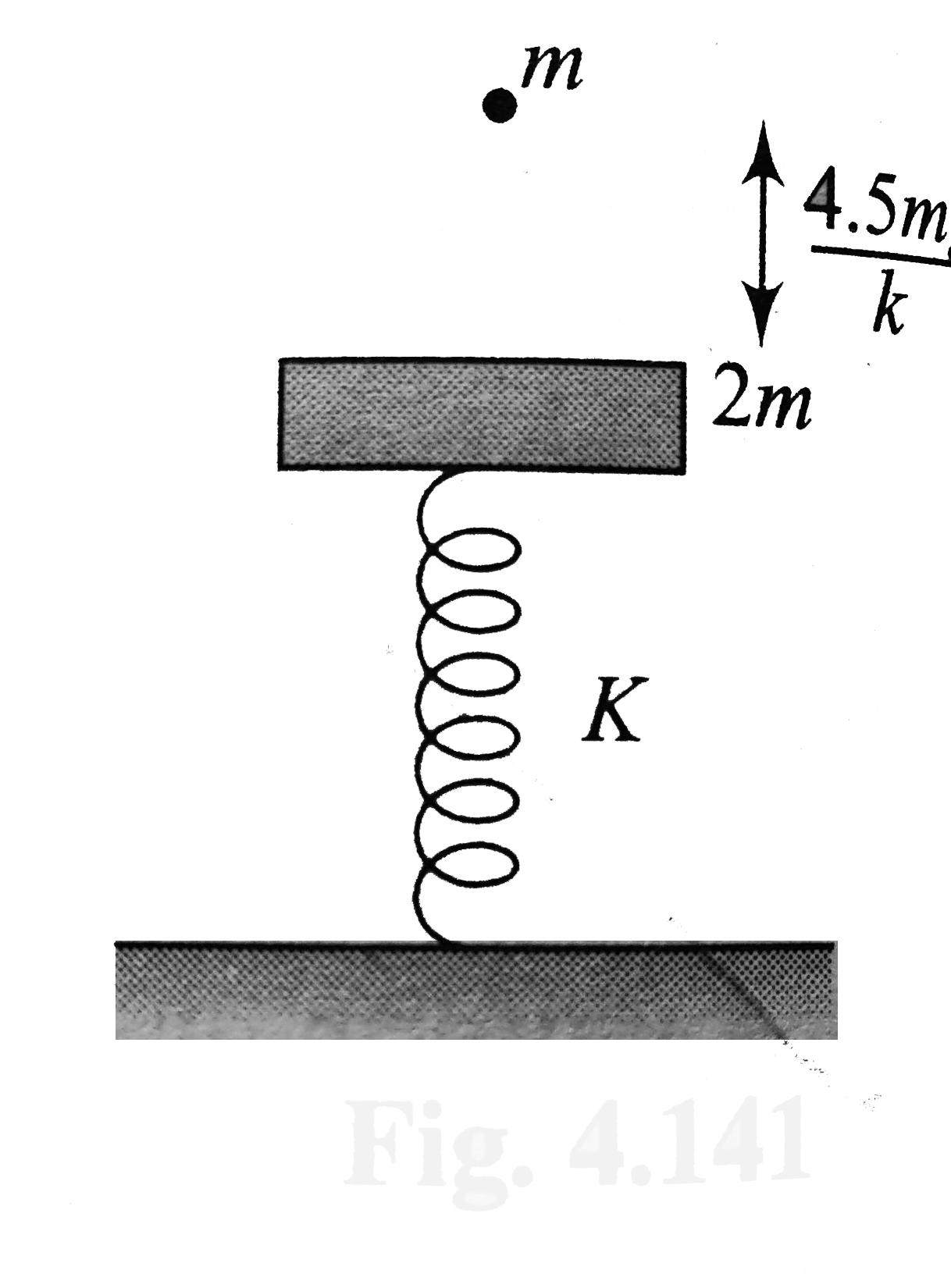 15:08View Solution
15:08View Solution
Subjective questions and complete solutions for chapter LINEAR AND ANGULAR SIMPLE HARMONIC MOTION of CENGAGE PHYSICS (HINGLISH) of Class 11
