Complete Solutions to Level -II(H.W) of chapter SYSTEM OF PARTICLES of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | SYSTEM OF PARTICLES |
| NARAYANA | Level -II(H.W) |
| Number Of Questions | 47 |
| Academic Year | 2023-24 |
Four particles, each of mass `1kg` are placed at the corners of a square of side `1m` in the `XY` plane. If the point of intersecting of the diagonals of the square is taken as the origin, the co-ordinates fo the centre of mass are
View SolutionThree identical particles each of mass `0.1 kg` are arranged at three corners of a square of side `sqrt(2) m`. The distance of the centre of mass from the fourth corners is
View SolutionA bomb of mass `m` at rest at the coordinate origin explodes into three equal pieces. At a certain instant one piece is on the x-axis at `x=60cm` and another is at `x=40cm, y=60cm`. The position of the third piece is
View SolutionMasses `1 kg, 1.5 kg,2 kg` and `Mkg` are situated at `(2,1,1),(1,2,1),(2,-2,1)` and `(-1,4,3)`. If their centre of mass is situated at `(1,1,3//2)`, the value of `M` is
View SolutionSix identical particles each of mass `m` are arranged at the corners of a regular hexagon of side length `L`. If the mass of one of the particle is doubled, the shift in the centre of mass is
View SolutionThree particles each of mass `m` are arranged at the corners of an equililateral triangle of side `L`. If one of masses is doubled. The shift in the centre of mass of the system
View SolutionFour identical particles each of mass `m` are arranged at the corners of a square of side length `l`. If the masses of the particles at the end of a side are doubled, the shift in the centre of mass of the system.
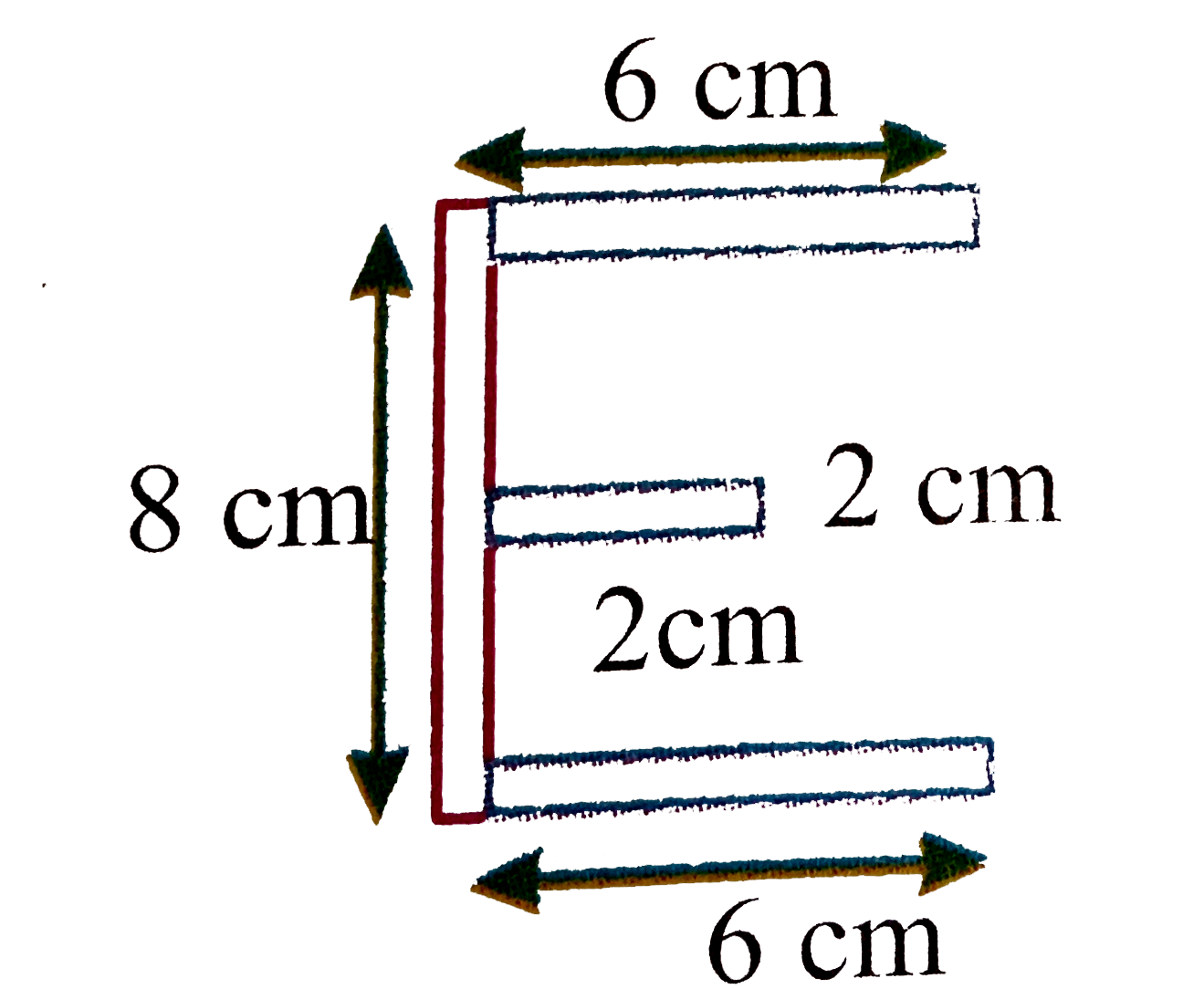
View SolutionThe co-ordinates of centre of mass of letter `E` which is cut from a uniform metal sheet are (take origin at bottom left corner and width of letter `2 cm` every where)
View SolutionTwo particles of equal masses have velocities `vec v_1 = 8 hat i` and `vec v_2 = 8 hat j`. First particle has an acceleration `vec a_1 = (5 hat i + 5 hat j) ms^(-2)` while the acceleration of the other particle is zero. The centre of mass of the two particles moves is a path of
View SolutionThe magnitude of two vectors which can be represented in the form `i+j(2x)k` is `sqrt(18)`. Then the unit vector that is perpendicular to these two vectors is
View SolutionA proton of velocity `(3hati+2hatj) ms^(-1)` enters a field of magnetic induction in the proton in (specific charge of proton `=0.96xx10^(8) Ckg^(-1)`) `[F=q(vec(v)xxvec(B))]`
View SolutionA vehicle starts from rest and moves at uniform acceleration such that its velocity increases by `3ms^(-1)` per every second. If diameter of wheel of that vehicle is `60 cm`, the angular acceleration of wheel is (in `rads^(-1)`)
View SolutionStarting from rest the fly wheel of a motor attains an angular velocity of `60 rad//sec` in `5` seconds. The angular acceleration obtained is
View SolutionA ceiling fan is rotating about its own axis with uniform angular velocity `omega`. The electric current is switched off then due to constant opposing torque is its angular velocity is reduced to `(2 omega)/3` as it completes `30` rotations. The number of rotations further it makes before coming to rest is
View SolutionA wheel has a speed of `1200` revolution per minute and is made to slow down at a rate of `4 rad//s^(2)`. The number of revolutions it makes before coming to rest is
View SolutionA particle of mass `1 kg` is projected with an initial velocity `10 ms^(-1)` at an angle of projection `45^(@)` with the horizontal. The average torque acting on the projectile and the time at which it strikes the ground about the point of projection in newton meter is
View SolutionA uniform meter scale of mass `1kg` is placed on table such that a part of the scale is beyond the edge. If a body of mass `0.25kg` is hung at the end of the scale then the minimum length of scale that should lie on the table so that it does not tilt is
View SolutionA heavy wheel of radius `20 cm` and weight `10 kg` is to be dragged over a step of height `10 cm`, by a horizontal force `F` applied at the centre of the wheel. The minimum value of `F` is
View SolutionTwo discs one of density `7.2 g//cm^(3)` and the other of density `8.9g//cm^(3)` are of same mass and thickness. Their moments of inertia are in the ratio
View SolutionThe mass of a circular ring is `M` and its radius is `R`. Its moment of inertia about an axis in the plane of ring at perpendicular distance `R//2` from centre of ring is
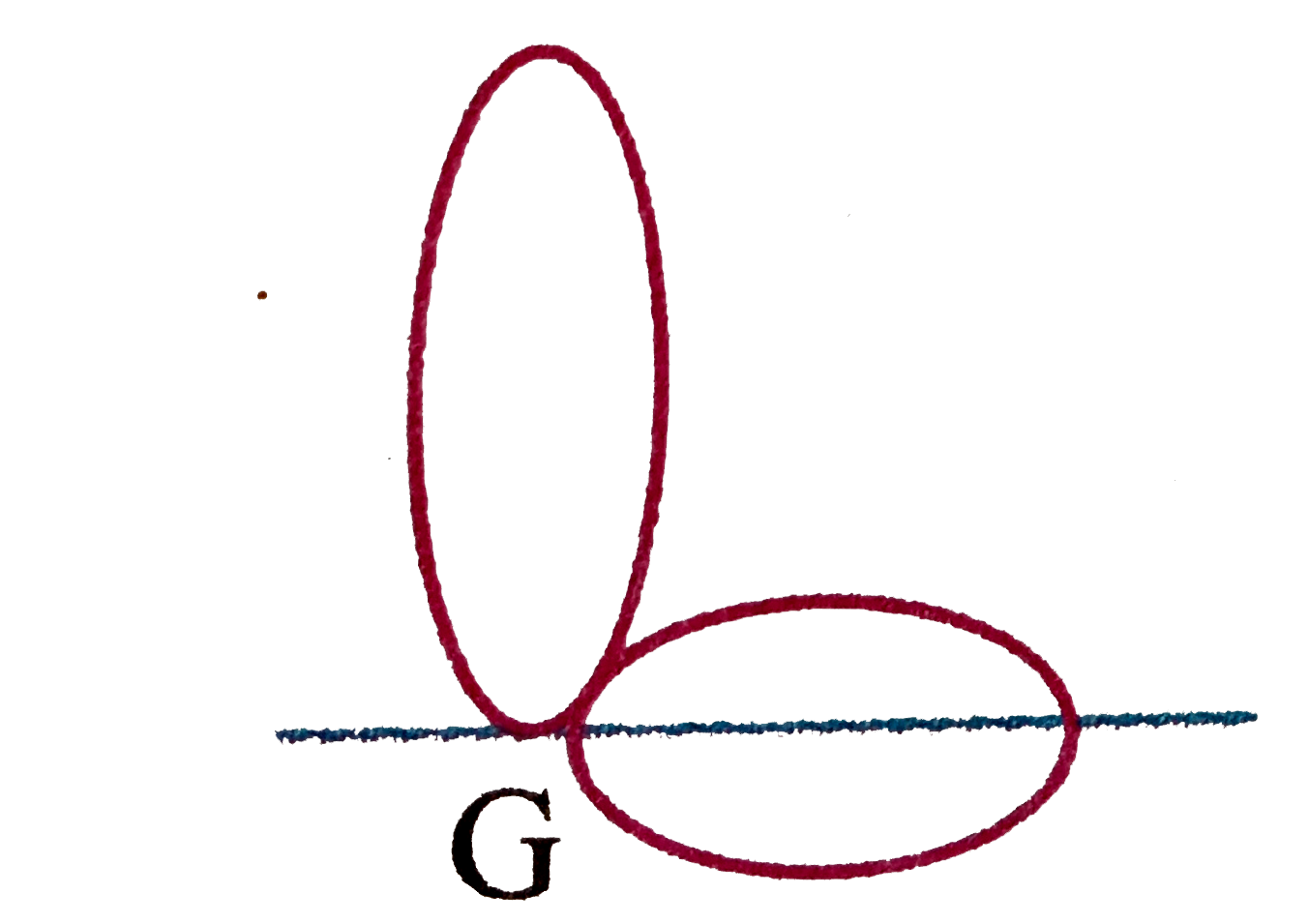
View SolutionTwo circular rings each of mass `M` and radius `R` are attached to each other at their rims and their planes perpendicular to each other as shown in figure. The moment of inertia of the system about a diameter of one of the rings and passing through the point of contanct is
View SolutionThe moment of inertia of a thin square plate of mass `1.2 kg ` is `0.2 kg m^(2)` when its is made to rotate about an axis perpendicular to plane of plate and through a corner of plate. The side length of plate is
View SolutionThree point masses `m_(1), m_(2)` and `m_(3)` are located at the vertices of an equilateral triangle of side `alpha`. What is the moment of inertia of the system about an axis along the altitude of the triangle passing through `m_(1)?`
View SolutionFrom a uniform wire, two circular loops are made (`i`) `P` of radius `r` and (`ii`) `Q` of radius `nr`. If the moment of inertia of `Q` about an axis passing through its center and perpendicular to tis plane is `8` times that of `P` about a similar axis, the value of `n` is (diameter of the wire is very much smaller than `r` or `nr`)
View SolutionThe moment of inertia of a uniform thin rod of length `L` and mass `M` about an axis passing through a point at a distance of `L//3` from one of its ends and perpendicular to the rod is
View SolutionTwo small spheres of mass `5kg` and `15 kg` are joined by a rod of length `0.5 m` and of negligible mass. The `M.I.` of the system about an axis passing through centre of rod and normal to it is
View SolutionRatio of densities of materials of two circular discs of same mass and thickness `5:6` the ratio of their `M.I.` about natural axes is
View Solution`M.I.` of a uniform horizontal solid cylinder of mass `M` about an axis passing through its edge and perpendicular to the axis of cylinder when its length is `6` times of its radius `R` is
View SolutionA circular disc of radius `R` and thickness `R//6` has moment of inertia `I` about an axis passing through its centre and perpendicular to its plane. It is melted and recast into a solid sphere. The `M.I` of the sphere about its diameter as axis of rotation is
View SolutionThe moment of inertia of ring about an axis passing through its diameter is `I`. Then moment of inertia of that ring about an axis passing through its centre and perpendicular to its plane is
View SolutionA thin rod of mass `6 m` and length `6L` is bent into regular hexagon. The `M.I.` of the hexagon about a normal axis to plane and through centre of system is
View SolutionIf `I_(1)` is moment of inertia of a thin rod about an axis perpendicular to its length and passing through its centre and `I_(2)` is its moment of inertia when it is bent into a shape of a ring then (Axis passing through its centre and perpendicular to its plane)
View SolutionThe moment of inertia of thin rod of linear density `lambda` and length `l` about an axis passing through one end and perpendicular to its length is
View SolutionMoment of inertia of a bar magnet of mass `M`,length `L` and breadth `B` is `I`. Then moment of inertia of another bar magnet with all these values doubled would be
View SolutionA circular disc is rotating without friction about its natural axis with an angular velocity `omega`. Another circular disc of same material and thickness but half the raduis is gently placed over it coaxially. The angular velocity of composite disc will be
View SolutionA ballot dancer is rotating about his own vertical axis on smooth horizontal floor with a time period `0.5 sec`. The dancer flods himself close to his axis of rotation due to which his radius of gyration decreases by `20%`, then his new time period is
View SolutionA particle of mass `1 kg` is moving alogn the line `y=x+2` with speed `2m//sec`. The magnitude of angular momentum of the particle about the origin is
View SolutionA uniform metal rod of length `L` and mass `M` is rotating about an axis passing throuth one of the ends perpendicular to the rod with angular speed `omega`. If the temperature increases by `t^@C` then the change in its angular velocity is proportional to which of the following ? (Coefficient of linear expansion of rod `=alpha`)
View SolutionA fly wheel of `M.I. 6xx10^(-2) kgm^(2)` is rotating with an angular velocity of `20 rad s^(-1)`. The torque required to bring it to rest in `4s` is
View SolutionWhen `200 J` of work is done on a fly wheel its frequency of rotation increase from `4Hz` to `9 Hz`. The `M.I.` of the wheel about the axis of rotations is nearly
View SolutionThe moment of inertia of a wheel of radius `20 cm` is `40 kgm^(2)` if a tangential force of `80 N` applied on the wheel, its rotational `K.E.` after `4s` is
View SolutionAn initial momentum is imparted to a homogenous cylinder, as a results of which it begins to roll without slipping up an inclined plane at a speed of `v_(0)=4m//sec` the plane make an angle `theta=30^(@)` with the horizontal. What height `h` will be the cylinder rise to? `(g=10 m//s^(2))`
View SolutionA solid cylinder starts rolling down on an inclined plane from its top and `V` is velocity of its centre of mass on reaching the bottom of inclined plane. If a block starts sliding down on an identical inclined plane but smooth, from its top, then the velocity of block on reaching the bottom of inlined plane is
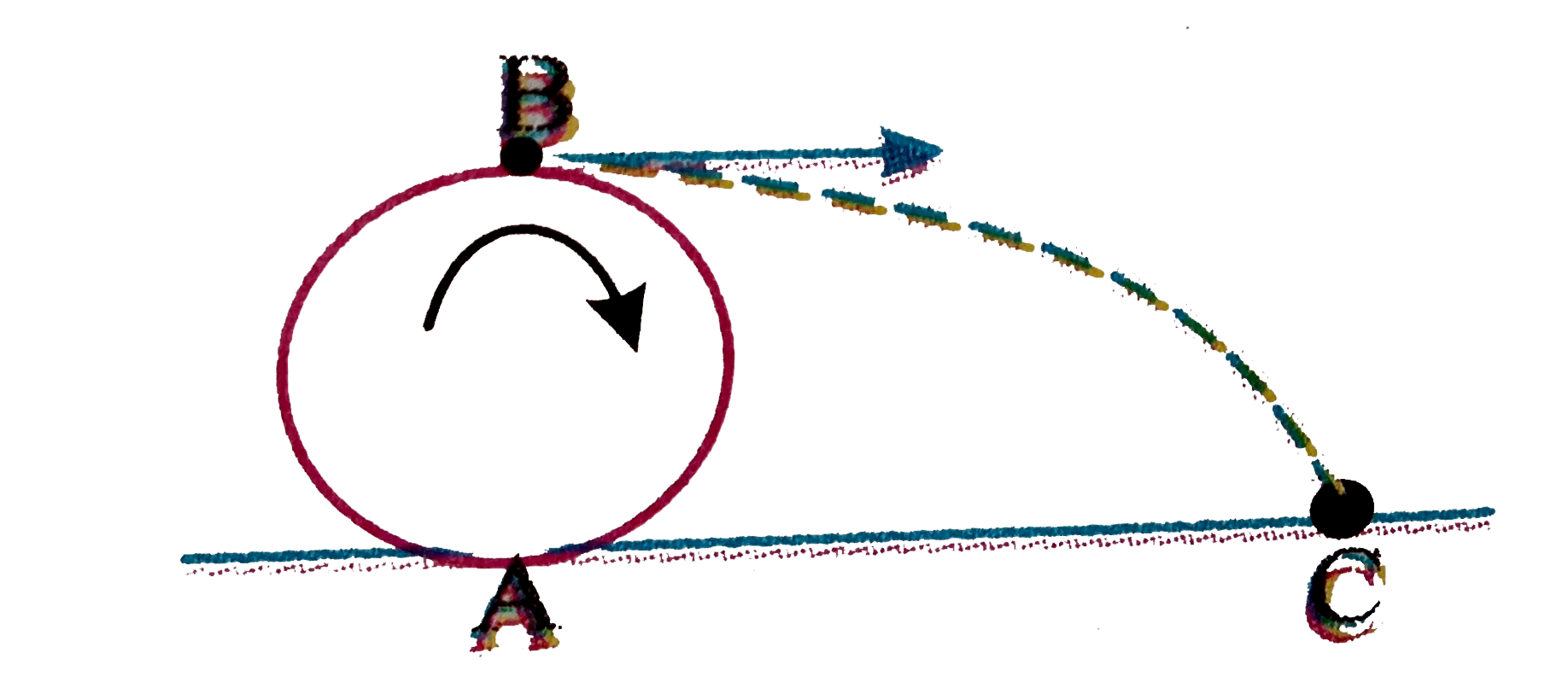
View SolutionA wheel of radius `0.2 m` rolls without slip ping with a speed `10 m//s` on ahorizontal road. When it is at a point `A` on the road, a small lump of mud separates from the wheel at its highest point `B` and drops at point `C` on the ground. The distance `AC` is
View SolutionA thin metal rod of length `0.5 m` is vertically straight on horizontal floor. This rod is falling freely to a side without slipping. The angular velocity of rod when its top end touches the floor is (nearly)
View SolutionWhat is the minimum value of coefficient of friction between the cylinder and inclined plane for rolling without slipping ?
View SolutionA thin metal disc of radius `0.25m` and mass `2kg` starts from rest and rolls down an inclined plane. If its rotational kinetic energy is `8J` at the foot of the inclined plane, then its linear velocity of centre of mass of disc is
View Solution
Level -II(H.W) questions and complete solutions for chapter SYSTEM OF PARTICLES of NARAYANA PHYSICS (HINGLISH) of Class 11