Complete Solutions to Level- II (C.W) of chapter WORK POWER AND ENERGY of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | WORK POWER AND ENERGY |
| NARAYANA | Level- II (C.W) |
| Number Of Questions | 68 |
| Academic Year | 2023-24 |
A body of mass `5 kg` is moved up over `10 m` along the line of greatest slope of a smooth inclined plane of inclination `30^@` with the horizontal. If `g = 10 m//s^(2)`, the work done will be
View SolutionA particle of mass `0.5 kg` is displaced from position `vec r_(1)(2,3,1)` to`vec r_(2)(4,3,2)` by applying a force of magnitude `30 N` which is acting along `(hati + hatj + hatk)`. The work done by the force is
View SolutionKinetic energy of a particle moving in a straight line varies with time `t` as `K = 4t^(2)`. The force acting on the particle
View SolutionA block of mass `5 kg` initially at rest at the origin is acted upon by a force along the positive `X-direction` represented by
`F=(20+5x) N`. Calculate the work done by the force during the displacement of the block from `x = 0` to `x = 4m`.
View SolutionA force `F` acting on a particle varies with the position `x` as shown in the graph. Find the work done by the force in displacing the particle from `x =-a` to `x = +2a`.
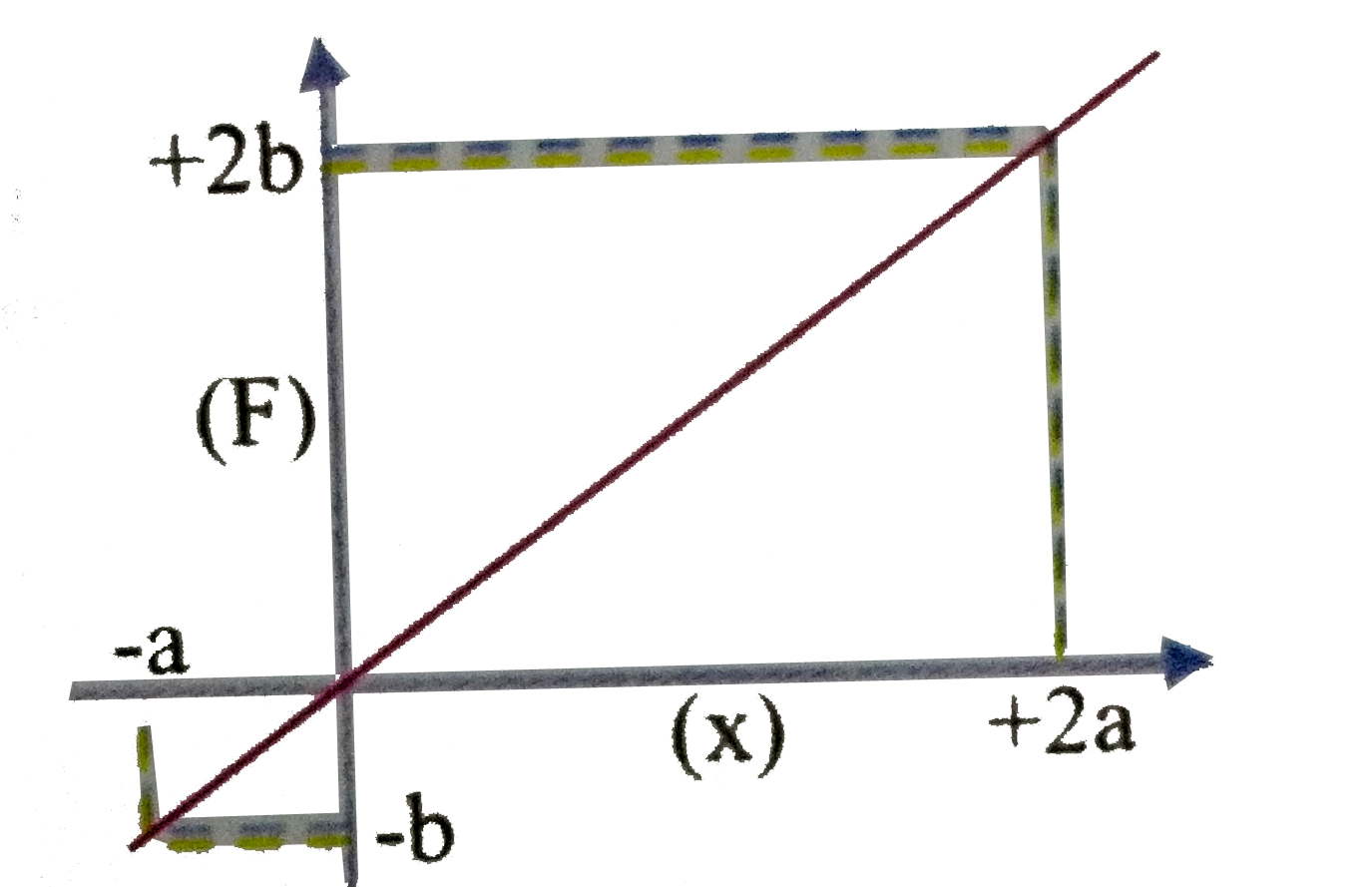 .
.
View SolutionA force `vec F=(2 hat i + 3hat j \- 4 hat k)N` acts on a particle moves `5 sqrt(2)m`, the work done by force in joule is
View SolutionTwo forces each of magnitude `10 N` act simultaneously on a body with their directions inclined to each other at an angle of `120^@`and displaces the body over `10 m` along the bisector of the angle between the two forces. Then the work done by force is
View Solutionn' identical cubes each of mass 'm' and edge 'L' are on a floor. If the cubes are to be arranged one over the other in a vertical stack, the work to be done is
View SolutionA uniform chain of mass m & length L is kept on a smooth horizontal table such that `(L)/(n) `portion of the chaing hangs from the table. The work dione required to slowly bringsthe chain completely on the table is
View SolutionA body is displaced from `(0,0)` to `(1 m, 1m)` along the path `x = y` by a force `F=(x^(2) hatj + y hati)N`. The work done by this force will be
View SolutionA particale moves under the effect of a force `F = Cs` from `x = 0` to `x = x_(1)`. The work down in the process is
View SolutionUnder the action of force `2 kg` body moves such that its position 'x' varies as a function of time `t` given by : `x = t^(2)//2`. The work done by the force in the first `5` seconds is
View SolutionA body of mass `5 kg` at rest under the action of a force which gives its velocity given by `v = 3 xx t m//s`, here 't' is time in seconds. The work done by the force in two seconds will be
View SolutionA body freely falls from a certain height onto the ground in a time 't'. During the first one third of the interval it gains a kinetic energy `Delta K_(1)` and during the last one third of the interval, it gains a kinetic energy `Delta K_(2)`. The ratio `Delta K_(1) : Delta K_(2)` is
View SolutionA man has twice the mass of a boy and has half the kinetic energy of the boy. The ratio of the speeds of the man and the boy must be
View SolutionThe speed of a car changes from `0` to `5 ms^(-1)` in the first phase and from `5 ms^(-1)` to `10 ms^(-1)` in the second phase and from `10 ms^(-1)` to `15 ms^(-1)` during the third phase. In which phase the increase in kinetic energy is more ?
View SolutionA rubber ball falling from a height of `5 m` rebounds from hard floor to a height of `3.5 m`. The `%` loss of energy during the impact is
View SolutionA long spring when stretched by `x cm`, has a potential energy `U`. On increasing the stretching to `nx cm,` the potential energy stored in spring will be
View SolutionAn elastic spring is compressed between two blocks of masses `1 kg` and `2 kg` resting on a smooth horizontal table as shown. If the spring has `12 J` of energy and suddenly released, the velocity with which the larger block of `2 kg` moves will be
 .
.
View SolutionA block of mass `2 kg` is on a smooth horizontal surface. A light of force constant `800 N//m` has one end rigidly attached to a vertical wall and lying on that horizontal surface. Now the block is moved towards the wall compressing the spring over a distance f `5 cm` and then suddenly released. By the time the spring regains its natural length and looses contact with the block, the velocity acquired by the block will be
View SolutionA bullet of mass `10 gm` is fired horizontally with a velocity `1000 ms^(-1)` from a riffle situated at a height `50 m` above the ground. If the bullet reached the ground with a velocity `500 ms^(-1)`, the work done against air resistance in the trajectory of the bullet is (in joule) `(g = 10 ms^(-2))`.
View SolutionA drop of mass `1.00 g` falling from a height `1.00 km`. It hits the ground with a speed of `50.0 ms^(-1)`. What is the work by the unknown resistive force ?
View SolutionA block of mass `5 kg` is initially at rest on a rough horizontal surface. A force of `45 N` acts over a distance of `2 m`. The force of friction acting on the block is `25 N`. The final kinetic energy of the block is
View SolutionA block of mass `2 kg` is initially at rest on a horizontal frictionless surface. A horizontal froce `overlineF = (9 -x^(2)) overlinei` newton acts on it, when the block is at `x = 0`. The maximum kinetic energy of the block between `x= 0 m` and `x = 3 m` in joule is
Conservation of mechanical energ
View SolutionA freely falling body takes `4s` to reach the ground. One second after release, the percentage of its potential energy, that is still retained is
View SolutionA vertically projected body attains the maximum height in `6s`. The ratio of kinetic energy at the end of `3^(rd)` second to decrease in kinetic energy in the next three seconds is
View SolutionTwo blocks A and B, each of mass m, are connected by a masslesss spring of natural length L and spring constant K. The blocks are initially resting on a smooth horizontal floor with the spring at its natural length, as shown in fig. A third identical block C, also of mass m, moves on the floor with a speed v along the line joining A and B, and collides elastically with A. Then
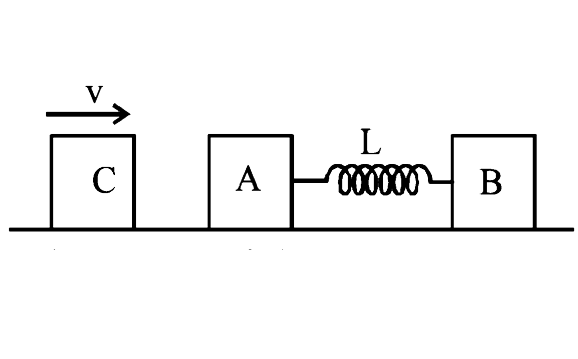
View SolutionA block of mass `m = 25 kg` on a smooth horizontal surface with a velocity `vec v = 3 ms^(-1)` meets the spring of spring constant `k = 100 N//m` fixed at one end as shown in figure. The maximum compression of the spring and velocity of block as it returns to the original position respectively are.
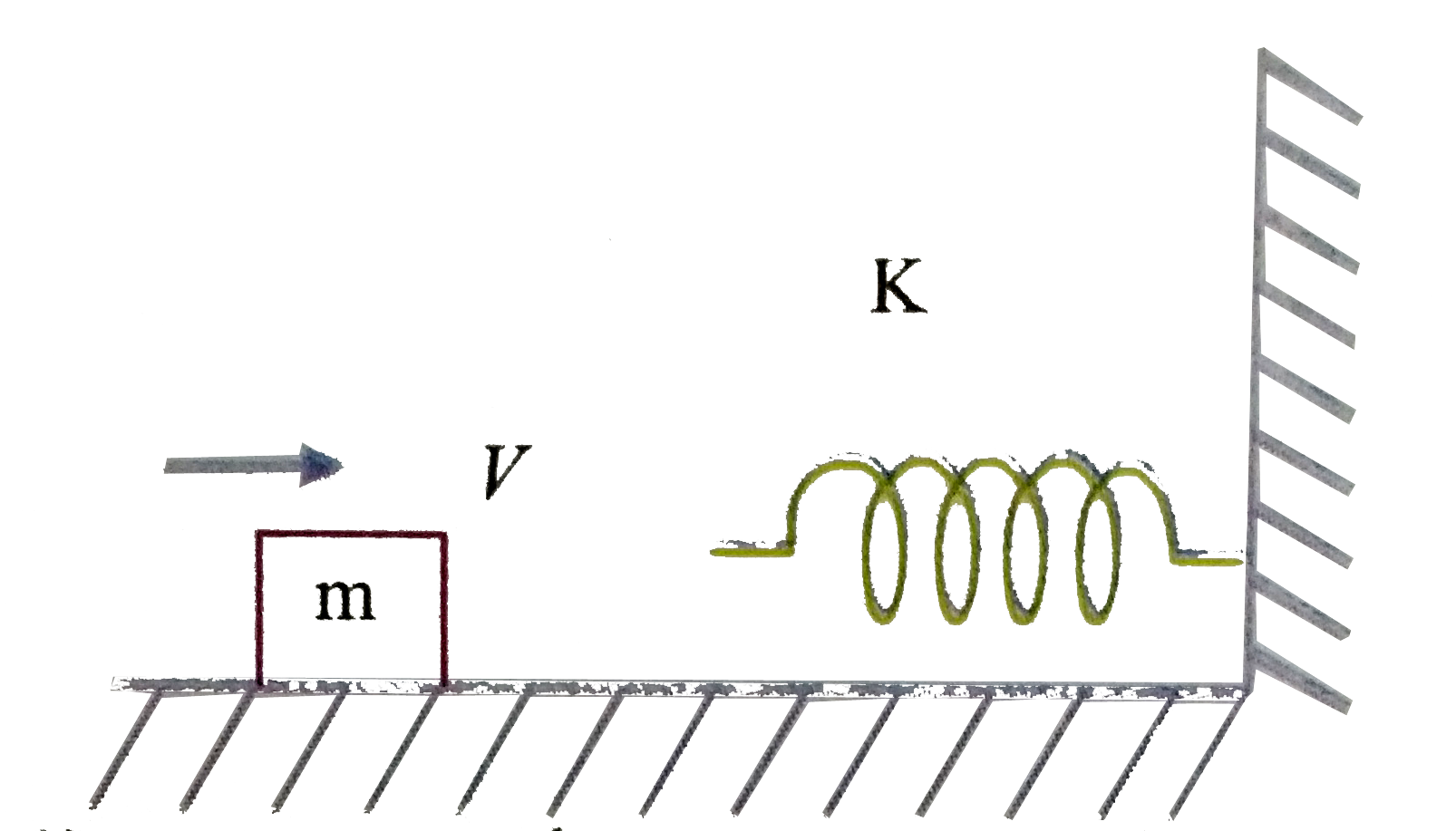 .
.
View SolutionA body is thrown vertically up with certain initial velocity, the potential and kinetic energies of the body are equal is thrown with double the velocity upwards, the ratio of potential and kinetic energies upwards, the ratio of potential and kinetic energies of the body when it crosses the same point, is
View SolutionA machine rated as `150 W`, changes the velocity of a `10 kg` mass from `4 ms^(-1)` to `10 ms^(-1)` in `4s`. The efficiency of the machine is nearly.
View SolutionA pump is required to lift `600 kg` of water per minute from a well `25 m` deep and to eject it with a speed of `50 ms^(-1)`. The power required to perform the above task is
View SolutionA tank on the roof of a `20 m` high building can hold `10 m^(3)` of water. The tank is to be filled form a pond on the ground in `20` minutes. If the pump has an efficiency of `60 %`, then the input power in `kW` is
View SolutionAn electric fan, with effective area of cross-section 'A', accelerates air of density 'rho' to a speed 'v'. What is the power needed for this process ?
View SolutionA point size mass `100 gm` is rotated in a vertical circle using a cord of length `20 cm`. When the string makes an angle `60^@` with the vertical, the speed of the mass is `1.5 m//s`. The tangential acceleration of the mass in that position is (in `ms^(-2)`).
View SolutionA vehicle is travelling along concave road then along convex road of same radius of curvatures at uniform speed. If the normal reactions on the vehicle as it crosses the lowest point of concave surface, highest point of concave surface, highest point of convex surface are `1.5 xx 10^(4) N, 3 xx 10^(3) N` respectively, then the mass of vehicle is `(g = 10 m//s^(-2))`.
View SolutionThe length of a simple pendulum is `1 m`. The bob is given a velocity `7 ms^(-1)` in horizontal direction from mean position. During upward motion of bob, if the string breaks when the bob is horizontal, then the maximum vertical height of ascent of bob from rest position is
View SolutionA body is allowed to slide down a frictionless track from rest position at its top under gravity. The track ends in a circular loop of diameter `D`. Then, the minimum height of the inclined track (in terms of `D`) so that it may complete successfully the loop is
View SolutionA body is mass `m` is rotating in a vertical circle of radius 'r' with critical speed. The difference in its `K.E` at the top and at the bottom is
View SolutionA simple pendulum of length 'l' carries a bob of mass 'm'. If the breaking strength of the string is `3 mg`, the maximum angular amplitude from the vertical can be
View SolutionA body of mass `4kg` moving with a speed of `3 ms^(-1)` collides head on with a body of mass `3 kg` moving in the opposite direction at a speed of `2 ms^(-1)`. The first body stops after the collision. The final velocity of the second body is
View SolutionThree identical particles with velocities `v_0hati`, `-3v_0hatj` and `5v_0hatk` collide successively with each other in such a way that they form a single particle. The velocity vector of resultant particle is
View SolutionFrom the top of a tower of height `100 m` a `10 gm` block is dropped freely and a `6 gm` bullet is fired vertically upwards from the foot of the tower with velocity `100 ms^(-1)` simultaneously. They collide and stick together. The common velocity after collision is `(g = 10 ms^(-2))`.
View SolutionA steel ball of radius `2 cm` is initially at rest. It is struck head on by another stell ball of radius `4 cm` travelling with a velocity of `81 cm//s`. If the collision is elastic their respective final velocities are
View SolutionA steel ball of radius `2 cm` is initially at rest. It is struck head on by another stell ball of radius `4 cm` travelling with a velocity of `81 cm//s`. The common velocity if it is perfectly inelastic collision.
View SolutionA tennis ball bounces down a flight of stairs, striking each step in turn and rebounding to half to height of the step. The coefficient of restitution is
View SolutionA ball hits the ground and loses `20 %` of its momentum. Coefficient of restitution is
View SolutionA plastic ball falling from a height `4.9 m` rebounds number of times. If total time for second collision is `2.4 sec`, then coefficient of restitution is
View SolutionA ball is dropped from a height 'h' on to a floor of coefficient of restitution 'e'. The total distance covered by the ball just before second hit is
View SolutionIn two separate collisions, the coefficient of restitutions `e_(1)` and `e_(2)` are in the ratio `3 : 1`. In the first collision the relative velocity of approach is twice the relative velocity of separation. Then, the ratio between relativevelocity of approach and relative velocity of separation in the second collision is
View SolutionA sphere of mass `m` moving with constant velocity `u`, collides with another stationary sphere of same mass. If `e` is the coefficient of restitution, the ratio of the final velocities of the first and second sphere is
View SolutionA canon shell fired breaks into two equal parts at its highest point. One part retraches the path to the canon with kinetic enegry `E_(1)` and the kinetic energy of the second part is `E_(2)`. Relation between `E_(1)` and `E_(2)` is
View SolutionA body of `200 g` begins to fall from a height where its potential energy is `80 J`. Its velocity at a point where its kinetic and potential energies are equal `(in m//s)`.
View SolutionThe work done by a force `overlineF = 2 overline i- overline j-overline k` in moving an object from origin to a point whose position vector is `overline r = 3 overline i + 2 overline j - 5 overline k`.
View SolutionA ball at rest is dropped from a height of `12 m`. If it looses `25 %` of its kinetic energy on striking the ground and bounces back to a height 'h'. The value of 'h' is equal to
View SolutionA mass of `2.9 kg` is suspended from a string of length `50 cm` and is at rest . Another body of mass `100 gm` which is moving horizontal with a velocity of `150 m//s` strikes it . After striking the two bodies combine together . Tension in the string , when it is at an angle of `60^(@)` with the velocity is : `g = 10 m//s^(2)`
View SolutionA body is thrown vertically upward from a point `A` 125 m above the ground. It goes up to a maximum height of 250 m above the ground and passes through `A` on its downward journey. The velocity of the body when it is at a height of 70 m above the ground is `(g=10 m//s^(2))`
View SolutionA body is mass `300 kg` is moved through `10 m` along a smooth inclined plane of inclination angle `30^@`. The work done in moving (in joules) is `(g = 9.8 ms^(-2))`.
View SolutionA ball of mass 'm' moving with a horizontal velocity 'v' strikes the bob of mass 'm' of a pendulum at rest. During this collision, the ball sticks with the bob of the pendulum. The height to which the combined mass raises is (g = acceleration due to gravity).
View SolutionThe velocity 'v' reached by a car of mass 'm' on moving a certain distance from the starting point when driven by a motor with constant power 'P' is such that
View SolutionA ball 'A' of mass 'm' moving along positive x-direction with kinetic energy `K` and linear momentum "p" undergoes elastic head on collision with a stationary ball 'B' of mass `M`. After the collision, the ball `A` moves along negative x-direction with kinetic energy `K//9`, the final linear momentum of the ball `B` is
View SolutionDisplacement of a body is `5 i+ 3 j - 4k m` due to the action of a force `6i+ 6j + 4k N` on it for `5 a`. The power in watt is
View SolutionA ball at rest is dropped freely from a height of `20 m`. It loses `30 %` of its energy on striking the ground and bounces back. The height to which it bounces back is
View SolutionA `3 kg` sphere makes an inelastic collision with another sphere at rest and they stick after the collision. If the composite mass moves with a speed of `(1^(th))/(4)` of the initial speed of `3 kg` sphere, the mass of second sphere is
View SolutionA ball is let to fall frm a height `h_(0)`. It makes 'n' collisions with the horizontal ground. If after 'n' collisions it rebounds with a velocity `v_(n)` and the ball rises to a height `h_(n)`, then the coefficient of restitution for the collision is
View SolutionA bullet is fired normally towards an immovable wooden block. If it loses `25 %` of its kinetic energy in penetrating through the block at thickness `x`, the further distance penetrated by the bullet into the block is
View SolutionA ball is falling freely from a certain height. When it reached `10 m` height from the ground its velocity is `v_(0)`. It collides with the horizontal ground and loses `50 %` of its energy and rises back to height of `10 m`. The value of velocity `v_(0)` is
View SolutionA motor of power `P_(0)` is used to deliver water at a certain rate through a given horizontal pipe. To increase the rate of flow of water through the same pipe 'n' times, the power of the motor is increased to `P_(1)`. The ratio of `P_(1)` to `P_(0)` is
View SolutionA body of mass `5 kg` makes an elastic collision with another body at rest and continues to move in the original direction after the collision with a velocity equal to `1//10^(th)` of its original velocity. The mass of the second body is
View Solution
Level- II (C.W) questions and complete solutions for chapter WORK POWER AND ENERGY of NARAYANA PHYSICS (HINGLISH) of Class 11