Complete Solutions to Solved Example of chapter WORK POWER AND ENERGY of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | WORK POWER AND ENERGY |
| NARAYANA | Solved Example |
| Number Of Questions | 55 |
| Academic Year | 2023-24 |
A body is displaced from `vec r_(A) =(2hat i + 4hatj -6hat k)` to `vecr_(B) = (6hat i -4hat j +3 hat k)` under a constant force `vec F = (2 hat i + 3 hat j - hat k)`. Find the work done.
View SolutionA force `vecF = 2 x hati + 2 hatj + 3z^(2)hatk N` is acting on a particle .Find the work done by this force in displacing the body from `(1, 2, 3) m` to `(3,6,1)m`
View SolutionThe force acting on an object varies with the distance travelled by the object as shown in the figure. Find the work done by the force in moving the object from `x = 0 m` to `x = 14 m`.
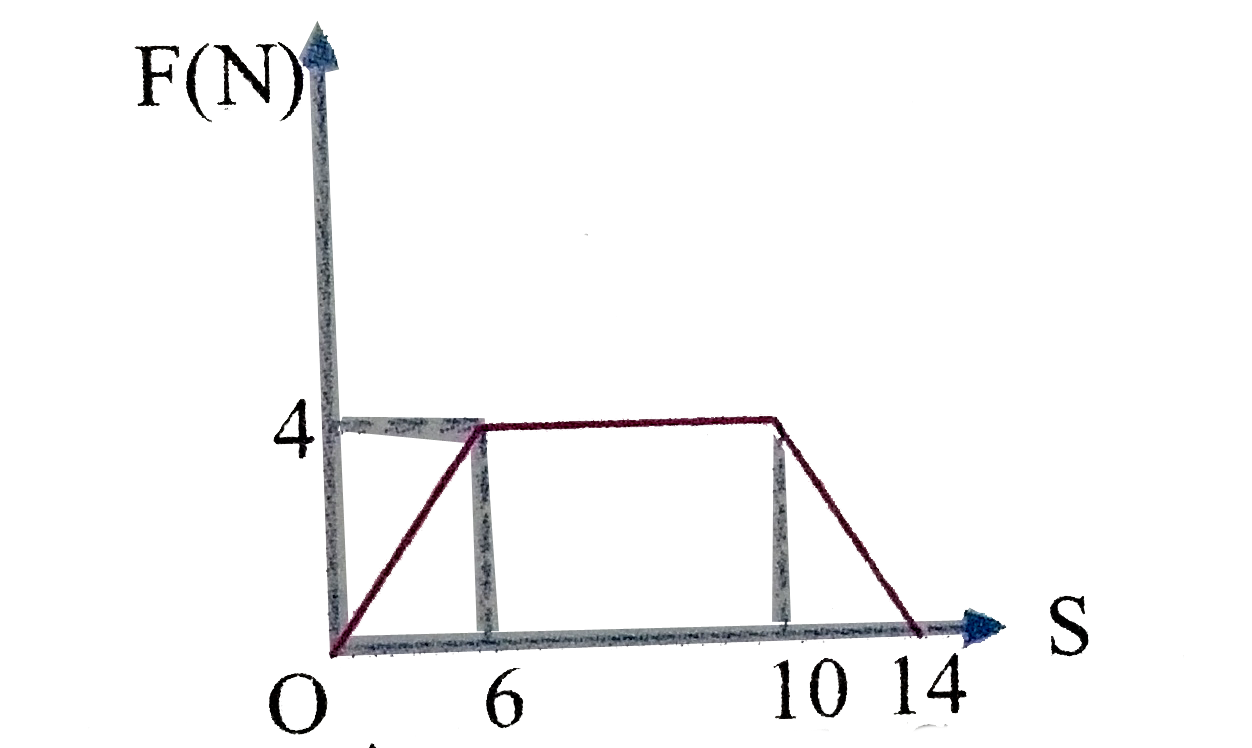 .
.
View SolutionWhen a rubber bandis streched by a distance `x` , if exerts resuring foprce of magnitube `F = ax + bx^(2)` where`a` and `b` are constant . The work in streached the unstreched rubber - band by `L` is
View SolutionA particle of mass `m` is projected at an angle `alpha` to the horizontal with an initial velocity `u`. The work done by gravity during the time it reaches its highest point is
View SolutionA `10 kg` block is pulled along a frictionless surface in the form of an arc of a circle of radius `10 m`. The applied force is `200 N`. Find the work done by (a) applied force and (b) gravitational force in displacing through an angle `60^@`.
 .
.
View SolutionA uniform chain of length `2m` is kept on a table such that a length of `60 cm` hangas freely from the adge of the table . The table . The total mass of the chain ia `4 kg` What is the work done in pulling the entire the chain the on the table ?
View SolutionFind the work done in lifting a body of mass `20 kg` and specific gravity `3.2` to a height of `8 m` in water ? `(g = 10 m//s^(2))`.
View SolutionA block of 'm' is lowered with the help of a rope of negligible mass through a distance 'd' with an acceleration of `g//3`. Find the work done by the rope on the block ?
View SolutionIf the system shown in released from rest. Find the net workdone by tension in first one second `(g = 10 m//s^(2))`.
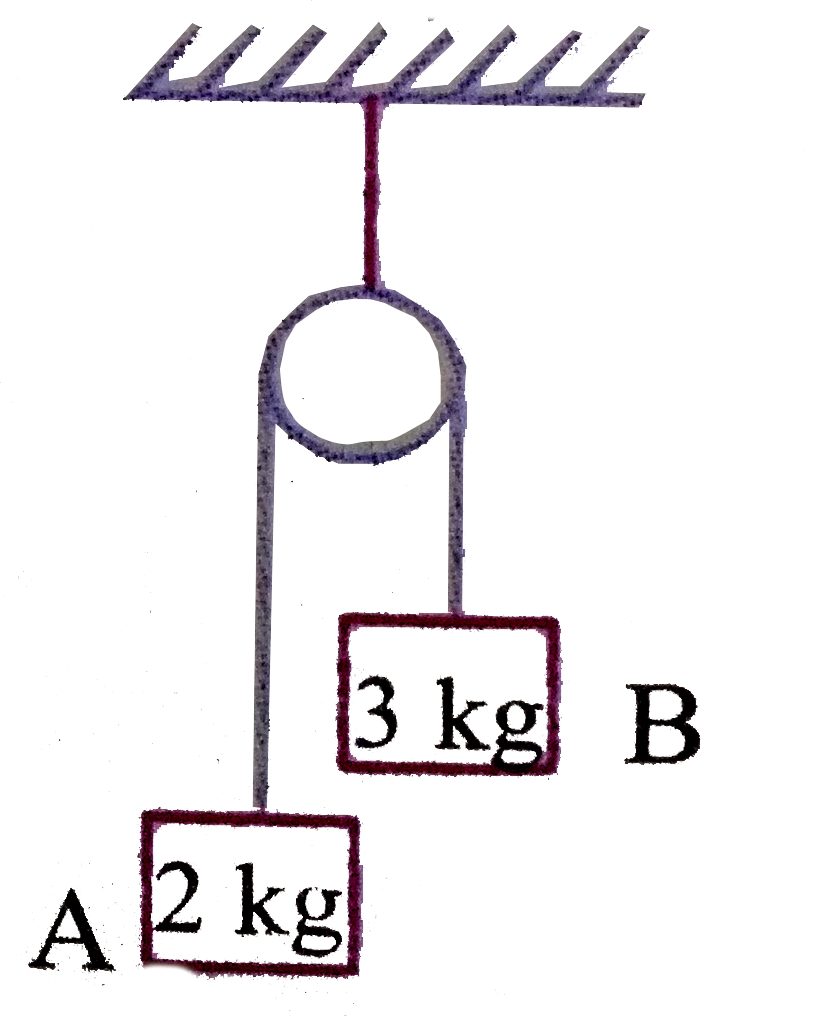 .
.
View SolutionA particle is projected at ` 60(@)` to the horizontal with a kinetic energy `K` . The kinetic energy at the highest point is
View SolutionAn athlete in the Olympic gamed covers a distance of `100 m` in `10 s`. His kinetic energy can be estimated to be in range.
(1) `200 J - 500 J`
(2) `2 xx 10^(5) J - 3 xx 10^(5) J`
(3) `20,000 J - 50,000 J`
(4) `2,000 J - 5,000 J`.
View SolutionThe kinetic energy of a particle moving along a circle of radius `R` depends on the distance covered `s` as `K=lambdas^(2)`, where `lambda` is a constant. Find the force acting on the particle as a function of `s`.
View SolutionA rectangular plank of mass `m_(1)` and height 'a' is on a horizontal surface. On the top of it another rectangular plank of mass `m_(2)` and height 'b' is placed. Find the potential energy of the system ?
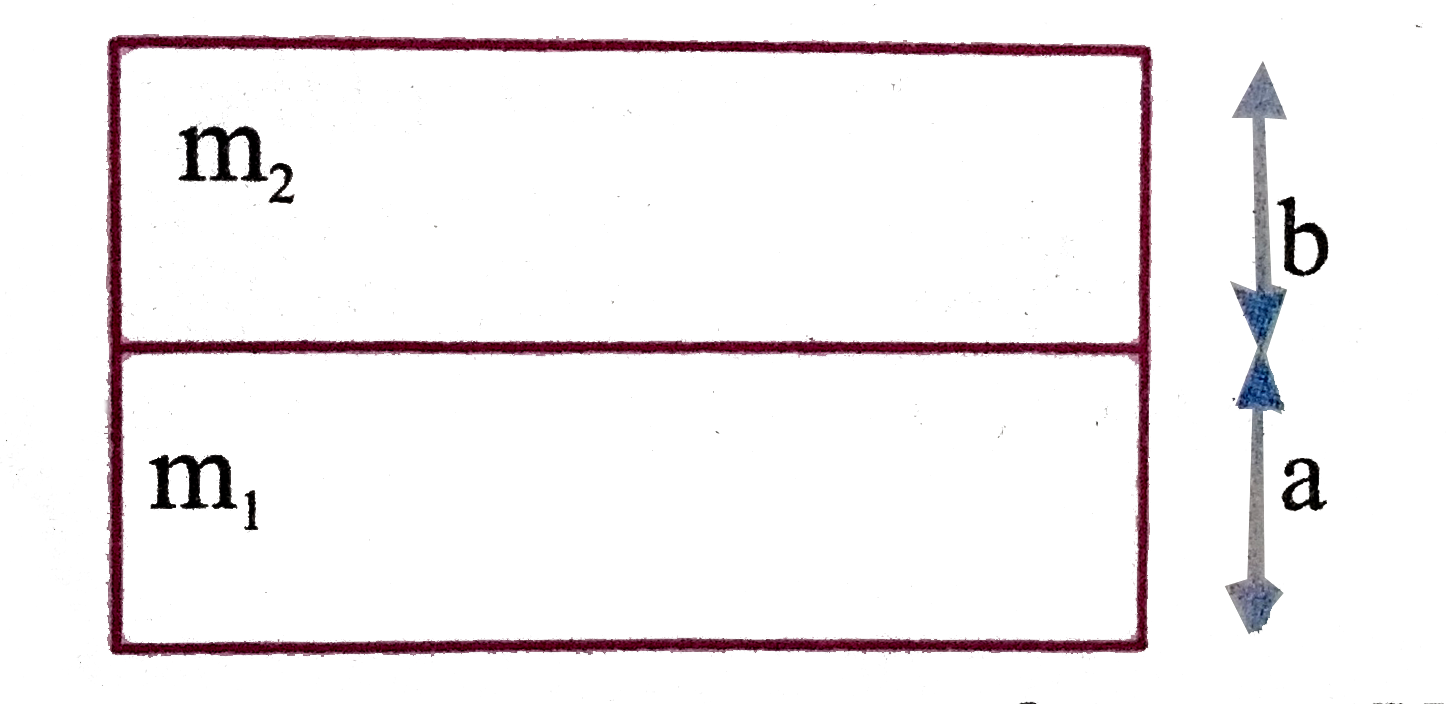 .
.
View SolutionA uniform rod of mass M and length L is held vertically upright on a horizontal surface as shown in figure. Assuming zero potential energy at the base of the rod, determine the potential energy of the rod.
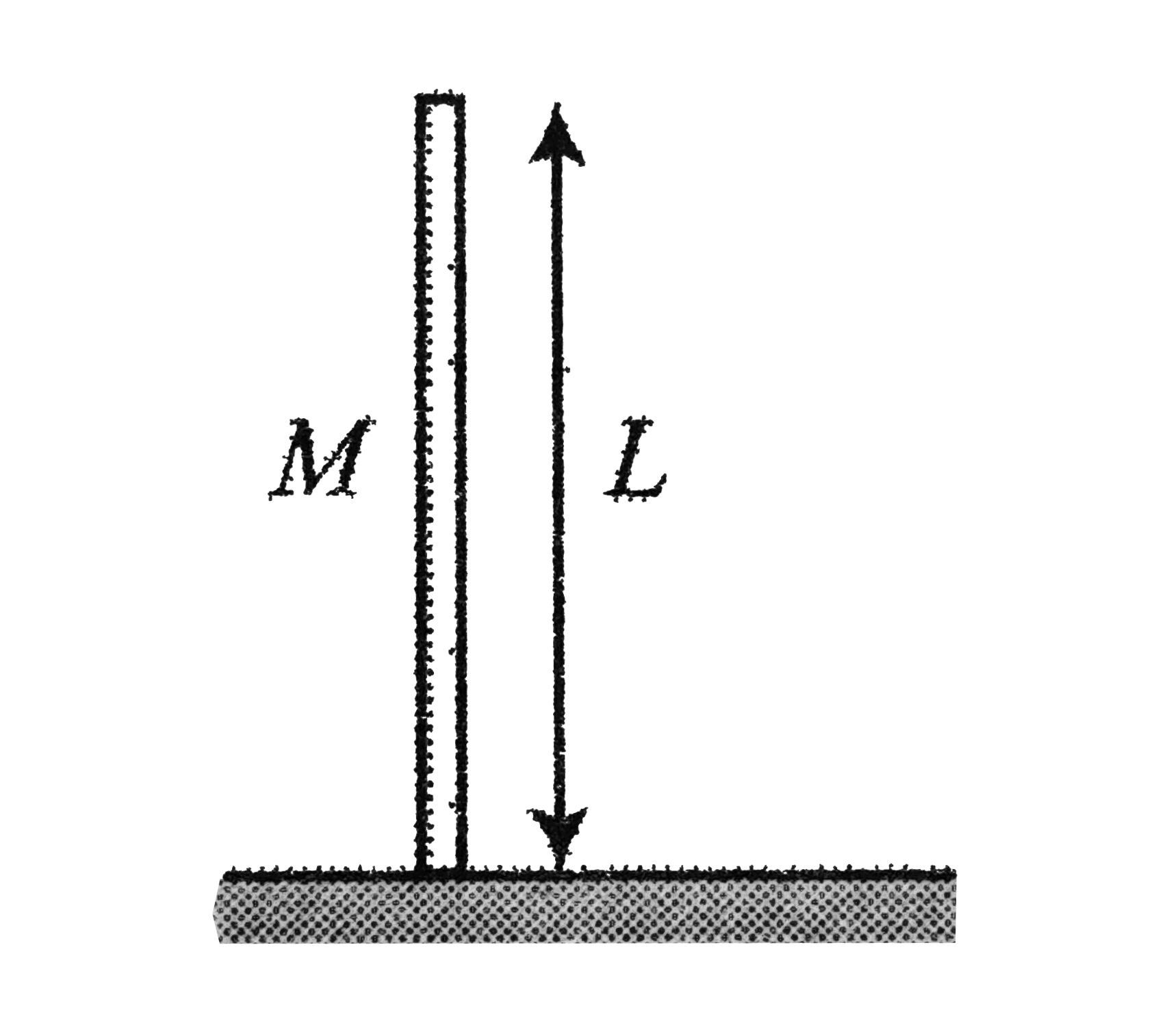
View SolutionA chain of length l and mass m lies of the surface of a smooth hemisphere of radius `Rgtl` with one end tied to the top of the hemisphere. Taking base of the hemisphere as reference line, find the gravitational potential energy of the chain.
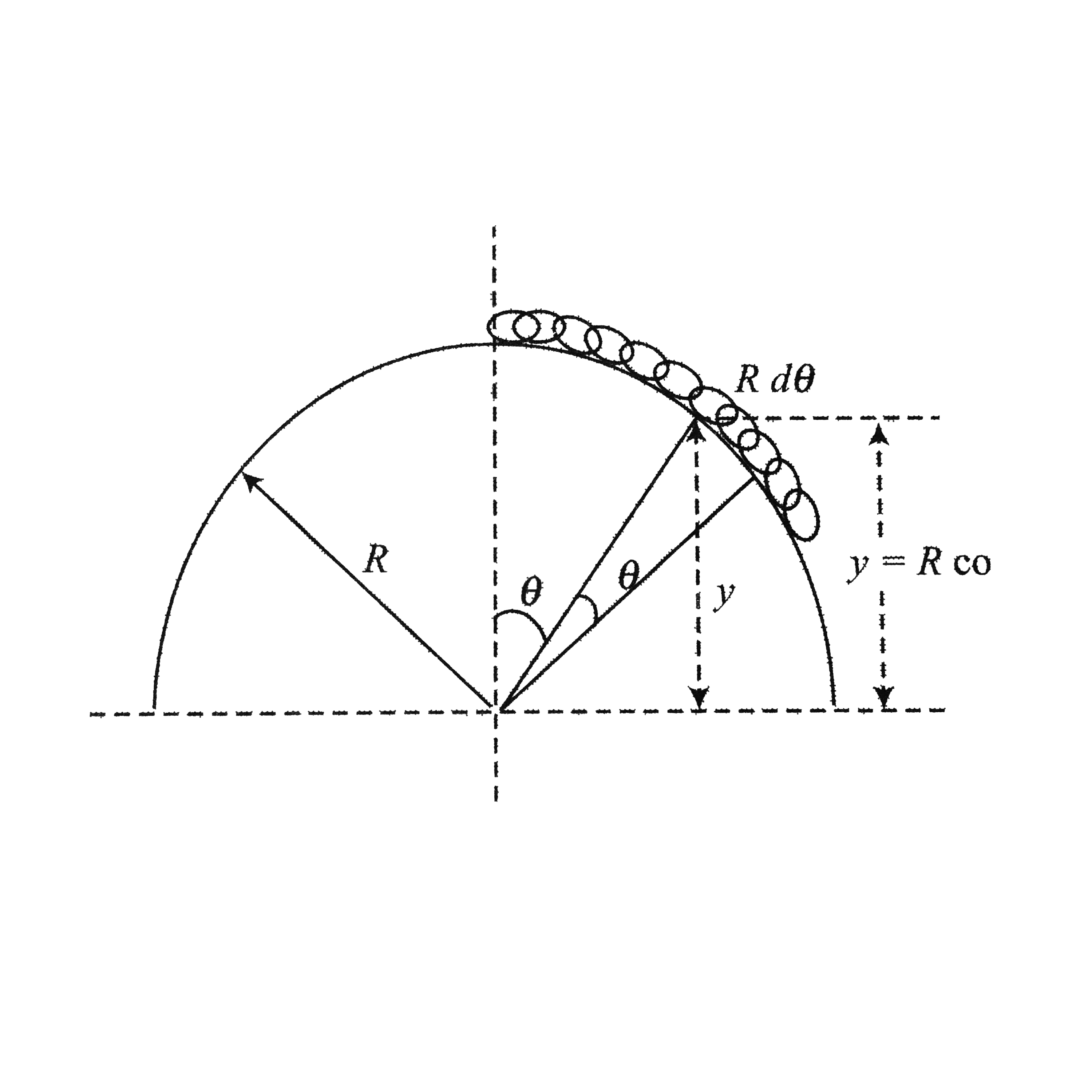
View SolutionAn elastic spring of unstretched length `L` and force constant `K` is stretched by amoun t `x` .It is further stretched by another length `y` The work done in the second streaching is
View SolutionUnder the action of a force, a `2 kg` body moves such that its position x as a function of time is given by `x =(t^(3))/(3)` where x is in metre and t in second. The work done by the force in the first two seconds is .
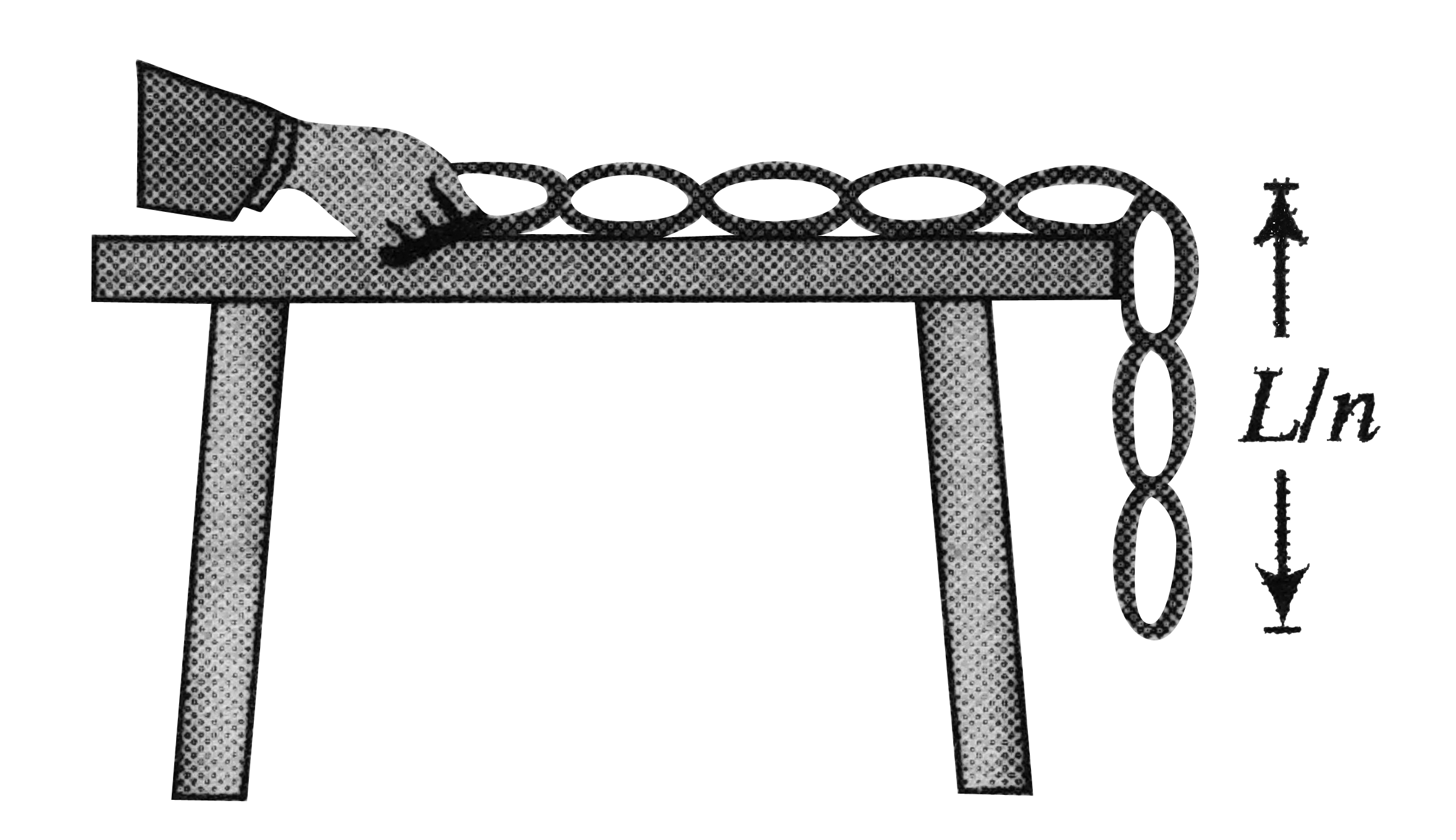
View SolutionA chain of length L and mass M is held on a frictionless table with `(1//n)th` of its length hanging over the edge. When the chain is released, find the velocity of chain while leaving the table.
View SolutionTwo blocks having masses `8kg` and `16kg` are connected to the two ends of a light spring. The system is placed on a smooth horizontal floor. An inextensible string also connects B with ceiling as shown in the figure at the initial moment. Initially the spring has its natural length. A constant horizontal force F is applied to the heavier block as shown. What is the maximum possible value of F so the lighter block doesn't loose contact with ground?

View SolutionA `2kg` block slides on a horizontal floor with the a speed of `4m//s` it strikes a uncompressed spring , and compresses it till the block is motionless . The kinetic friction force is compresses is `15 N` and spring constant is `10000 N//m` . The spring by
View SolutionIn the below figure, what constant force 'P' is requried to bring the `50 kg` body, which starts from rest to a velocity of `10 m//s` in moving `7 m` along the plane ? (Neglect friction).
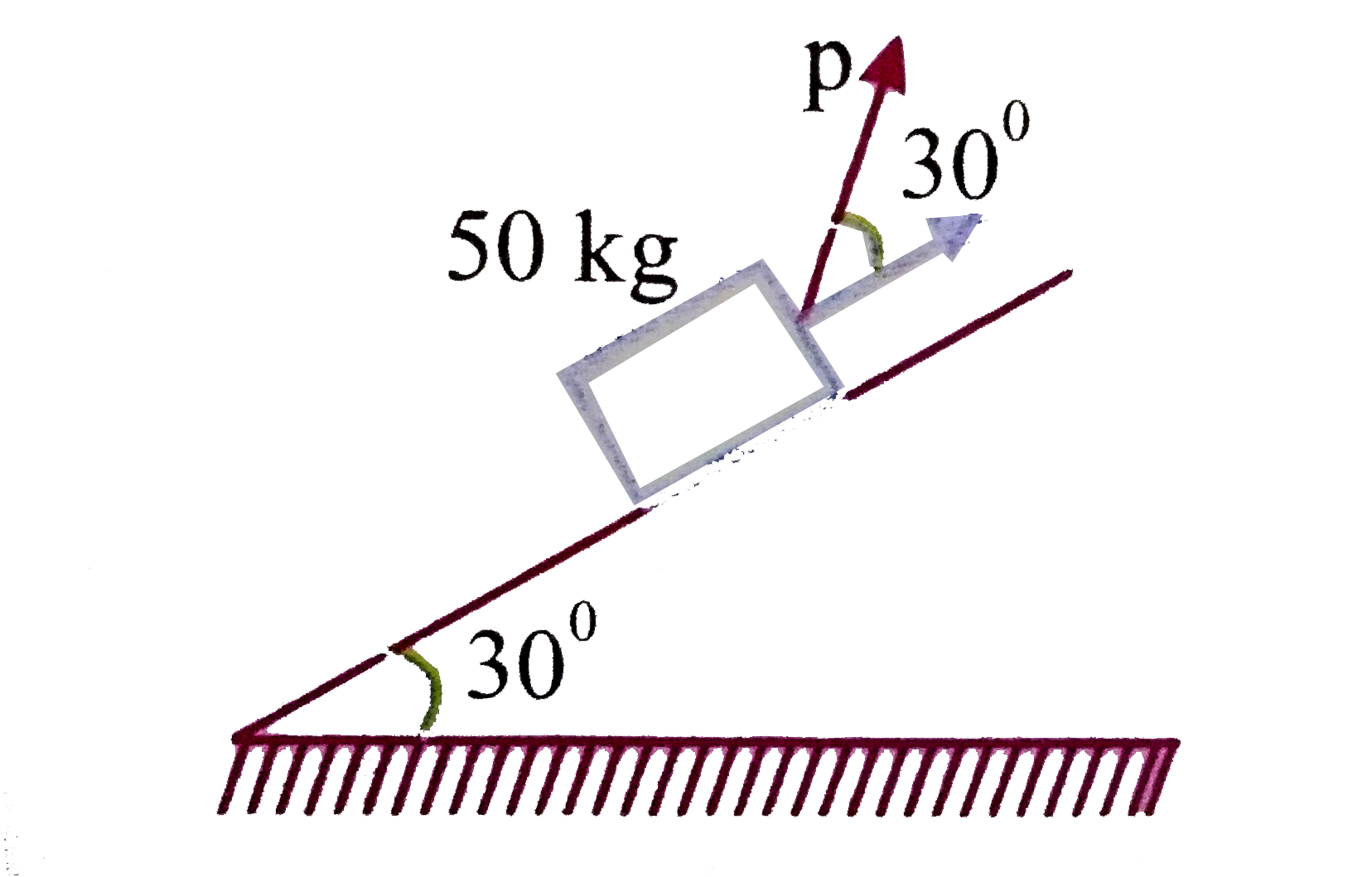 .
.
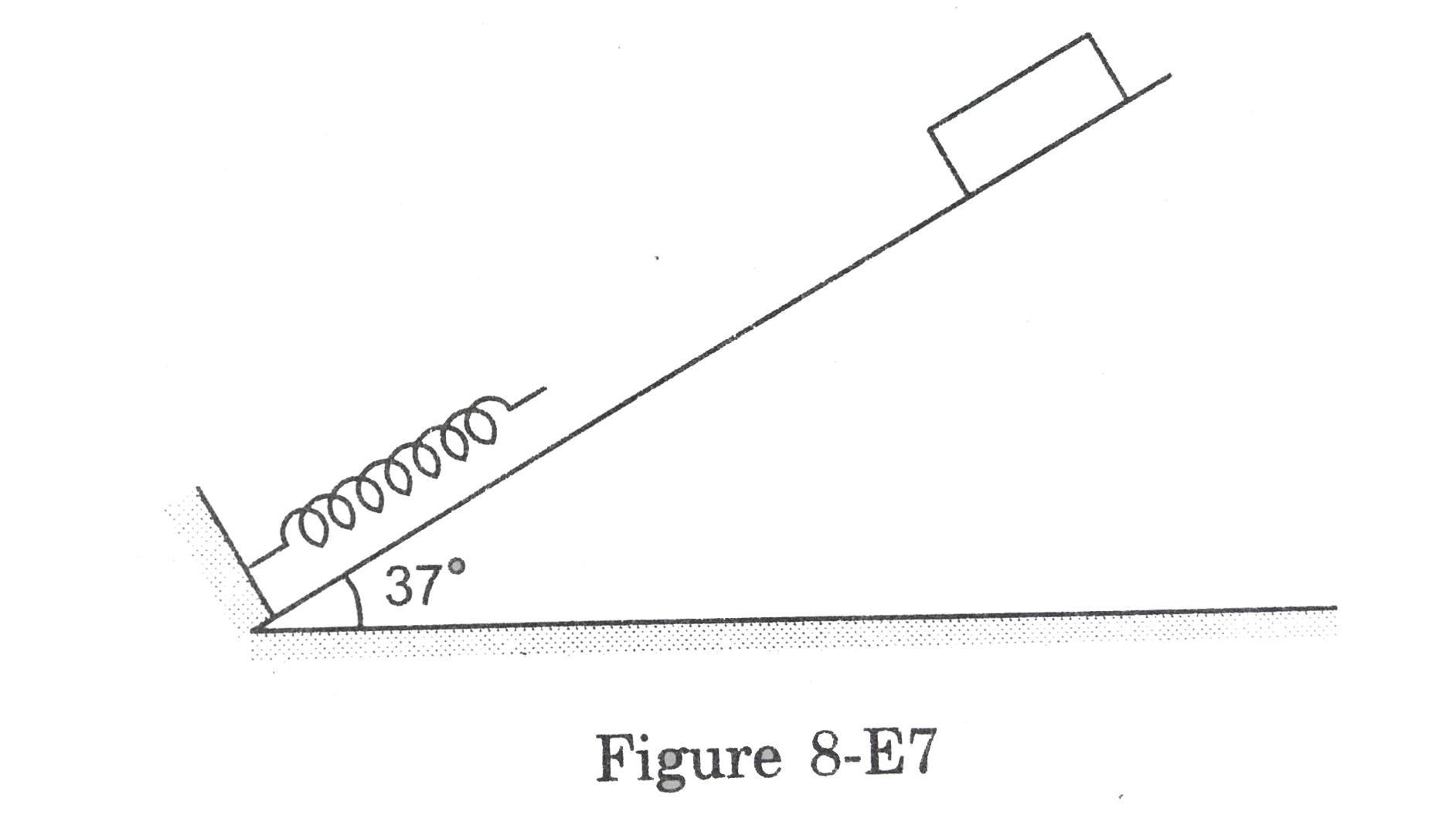
View SolutionFigure shows a spring fixed at the bottom end of an incline of inclination `37^0`. A small block of mass 2 kg starts slipping down the incline from a point 4.8 m away from the spring. The block compresses the spring by 20 cm, stops momentarily and then rebounds through a distance of 1 m up the incline. Find a. the frictioin coefficient between the plane and the block and b. the spring constant of the spring. Take `g=10 m/s^2`.
View SolutionIn a molecule, the potential energy between two atoms is given by `U (x) = (1)/(x^(12)) -(b)/(x^(6))`. Where 'a' and 'b' are positive constants and 'x' is the distance between atoms. Find the value of 'x' at which force is zero and minimim `P.E` at that point.
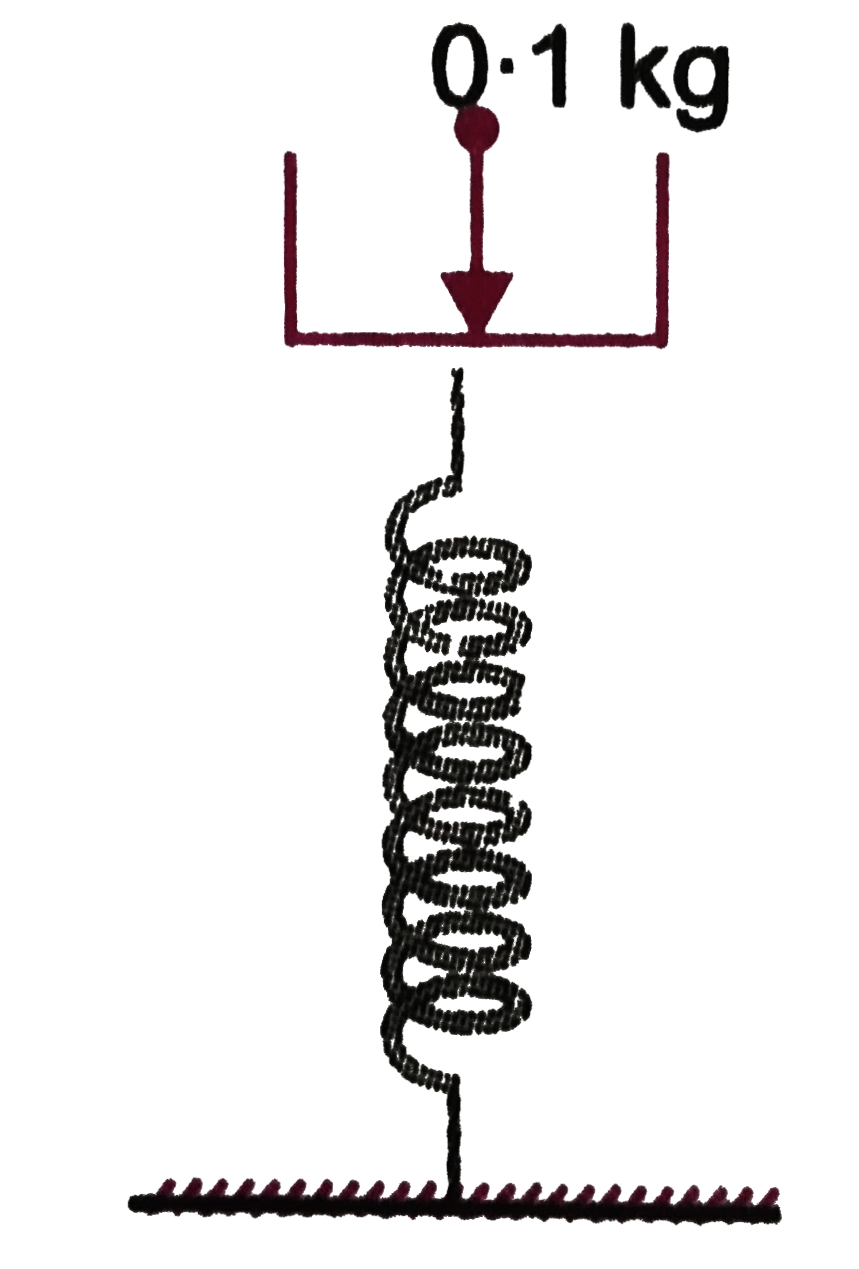
View SolutionA massless platform is kept on a light elastic spring as shown in figure. When a small stone of mass 0.1 kg is dropped on the pan from a height of 0.24 m, the spring compresses by 0.01m. From what height should the stone be droppped to cause a compression of 0.04m in the spring ?
View SolutionA small mass 'm' is sliding down on a smooth curved incline form a height 'h' and finally moves through a horizontal smooth surface. A light spring of force constant `K` is fixed with a vertical rigid stand on the horizontal surface, as shown in the figure. Find the value for the maximum compression in the spring if mass 'm' is released from rest from height 'h' and hits the spring in the horizontal surface.
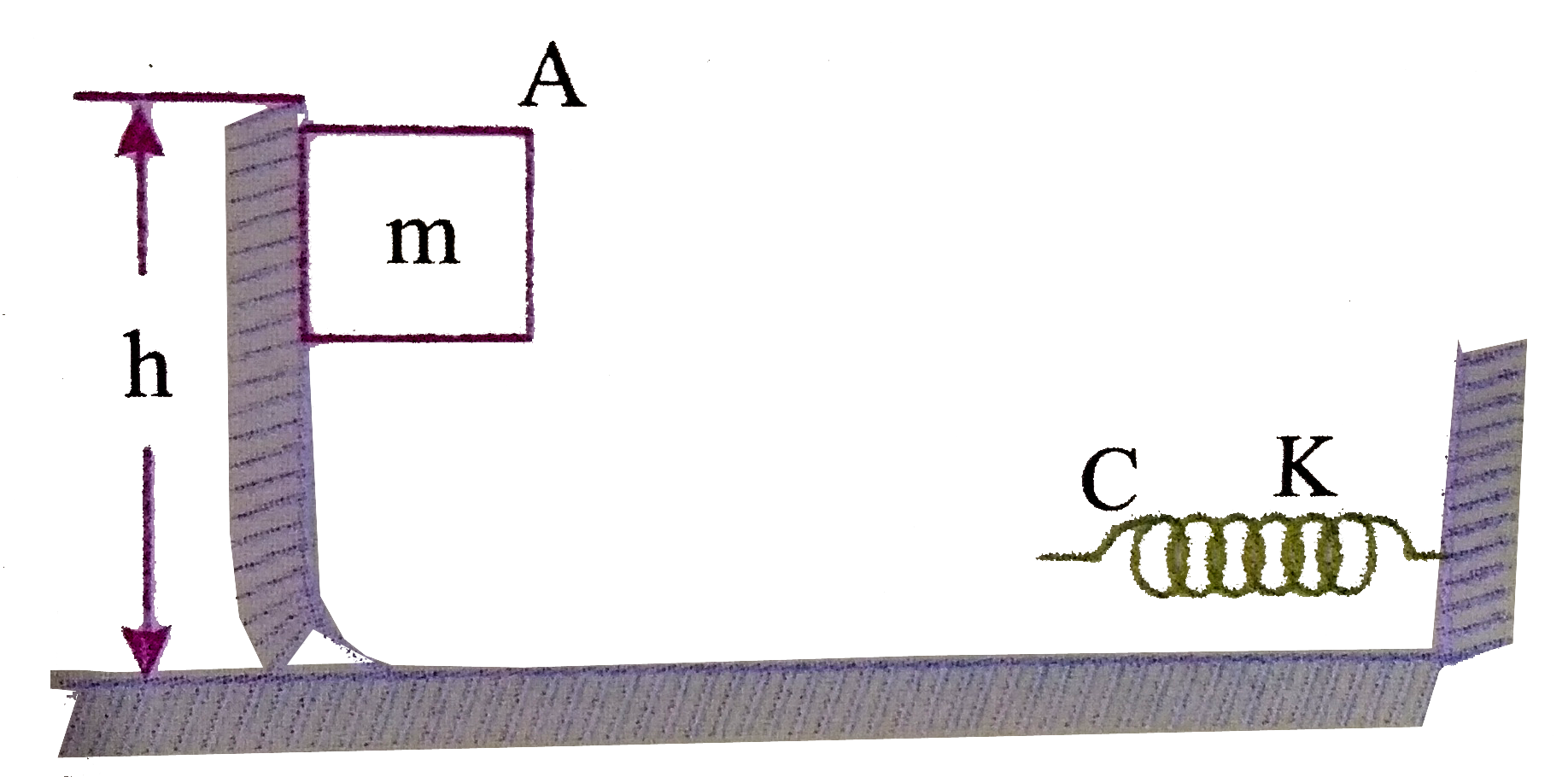 .
.
View SolutionA vehicle of mass `15` quintal climbs up a hill `200 m` high. It then moves on a level road with a speed of `30 ms^(-1)`. Calculate the potential energy gained by it and its total mechanical energy while running on the top of the hill.
View SolutionA particle is released from height `H`. At cartain height from the ground its kinetic energy is twice its gravitational potential energy. Find the height and speed of particle at that height.
View SolutionA uniform chain of length of length `pir` lies inside a smooth semicircular tube (AB) of radius r. Assuming a slight disturbance to start the chain in motion, the velocity with which it will emerge from the end `(B)` of the tube will be

View SolutionThe potential energy of a `1 kg` particle free to move along the x- axis is given by `V(x) = ((x^(4))/(4) - x^(2)/(2)) J`
The total mechainical energy of the particle is `2 J` . Then , the maximum speed (in m//s) is
View Solution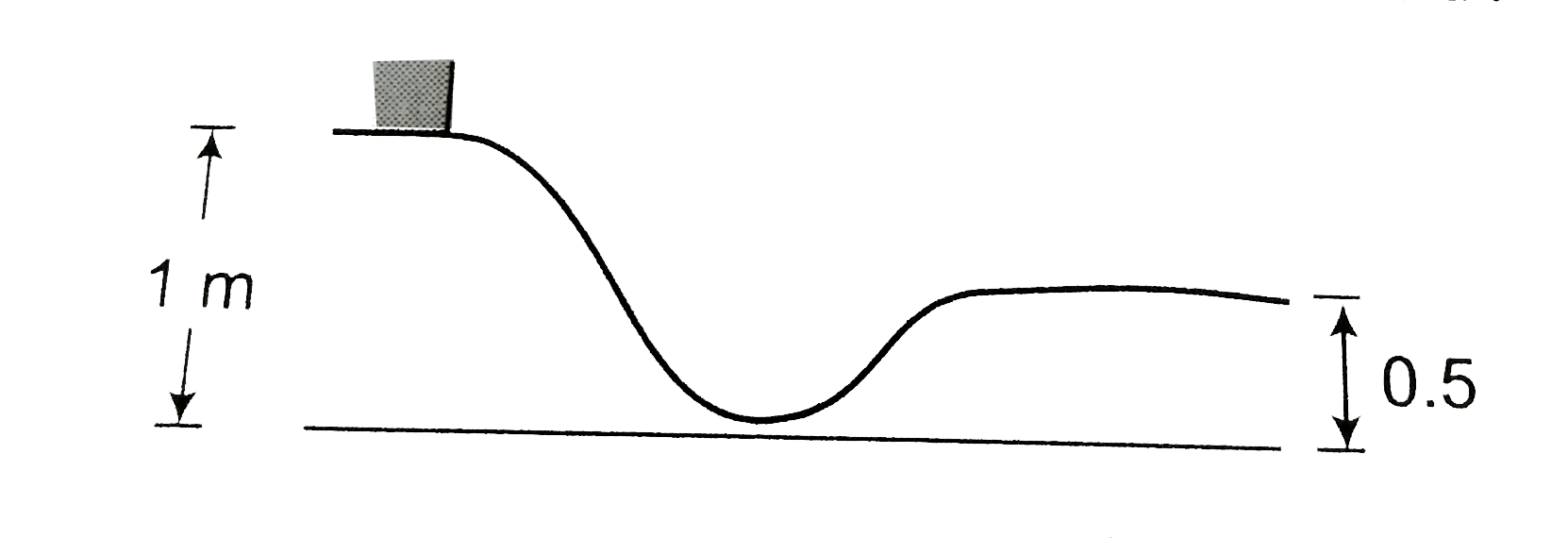
The figure shown a particle sliding on a frictionless track, which teminates in a straight horizontal section. If the particle starts slipping from the point `A`, how far away from the track will the particle hit the ground?
View SolutionAn automobile is moving at `100 kmph` and is exerting attractive force of `3920 N`. What horse power nust the engine develop, if `20 %` of the power developed is wasted ?
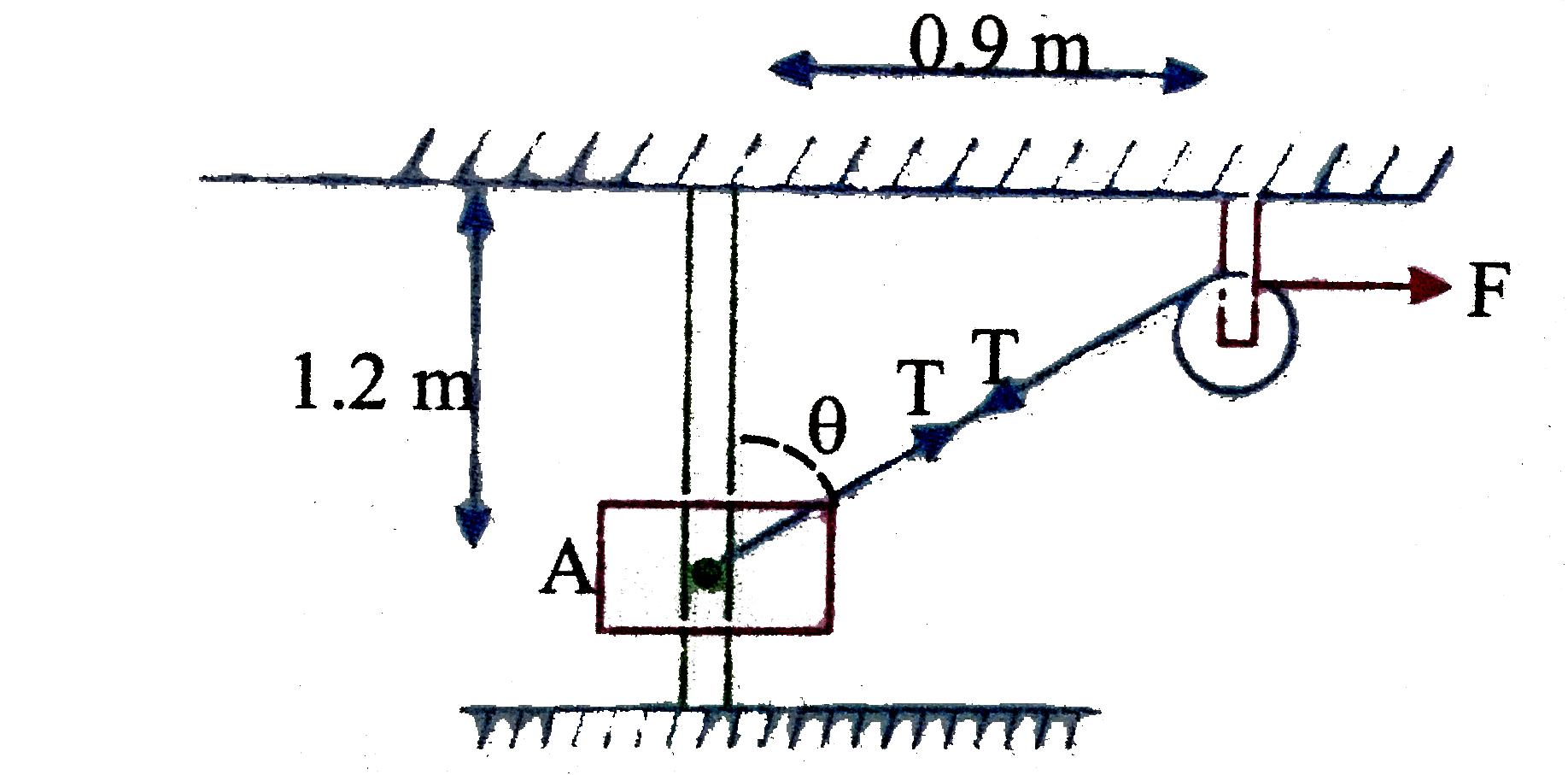
View SolutionThe `50 N` collar starts from rest at `A` and is lifted with a constant speed of `0.6 m//s` along the smooth rod. Determine the power developed by the force `F` at the instant shown.
 .
.
View SolutionA machine delivers power to a body which is directly proportional to velocity of the body. If the body starts with a velocity which is almost negligible, find the distance covered by the body in attaining a velocity `v`.
View SolutionFind the power of an engine which can draw a train of `400` metric ton up the inclined plane of `1` in `98` at the rate `10 ms^(-1)`. The resistance due to friction acting on the train is `10 N` per ton.
View SolutionA hose pipe has a diameter of `2.5 cm` and is required to direct a jet of water to a height of atleast `40 cm`. Find the minimum power of the pump needed for this hose.
View SolutionA body of mass `m` accelerates uniformly from rest to velocity `v_(0)` in time `t_(0)`, find the instantaneous power delivered to body when velocity is `(v_(0))/(2)`.
View SolutionA nail is located at a certain distance vertically below the point of suspension of a simple pendulum. The pendulum bob is released from a position where the string makes an angle of `60^(@)` with the vertical. Calculate the distance of nail from the point of suspension such that the bob will just perform revolutions with the nail as centre. Assume the length of the pendulum to be one meter.
View SolutionA body slides without friction from a height `H = 60 cm` and then loops the loop of radius `R = 20 cm` at the bottom of an incline. Find the ratio of forces exerted on the body by the track at the positions `A,B` and `C (g = 10 ms^(-2))`.
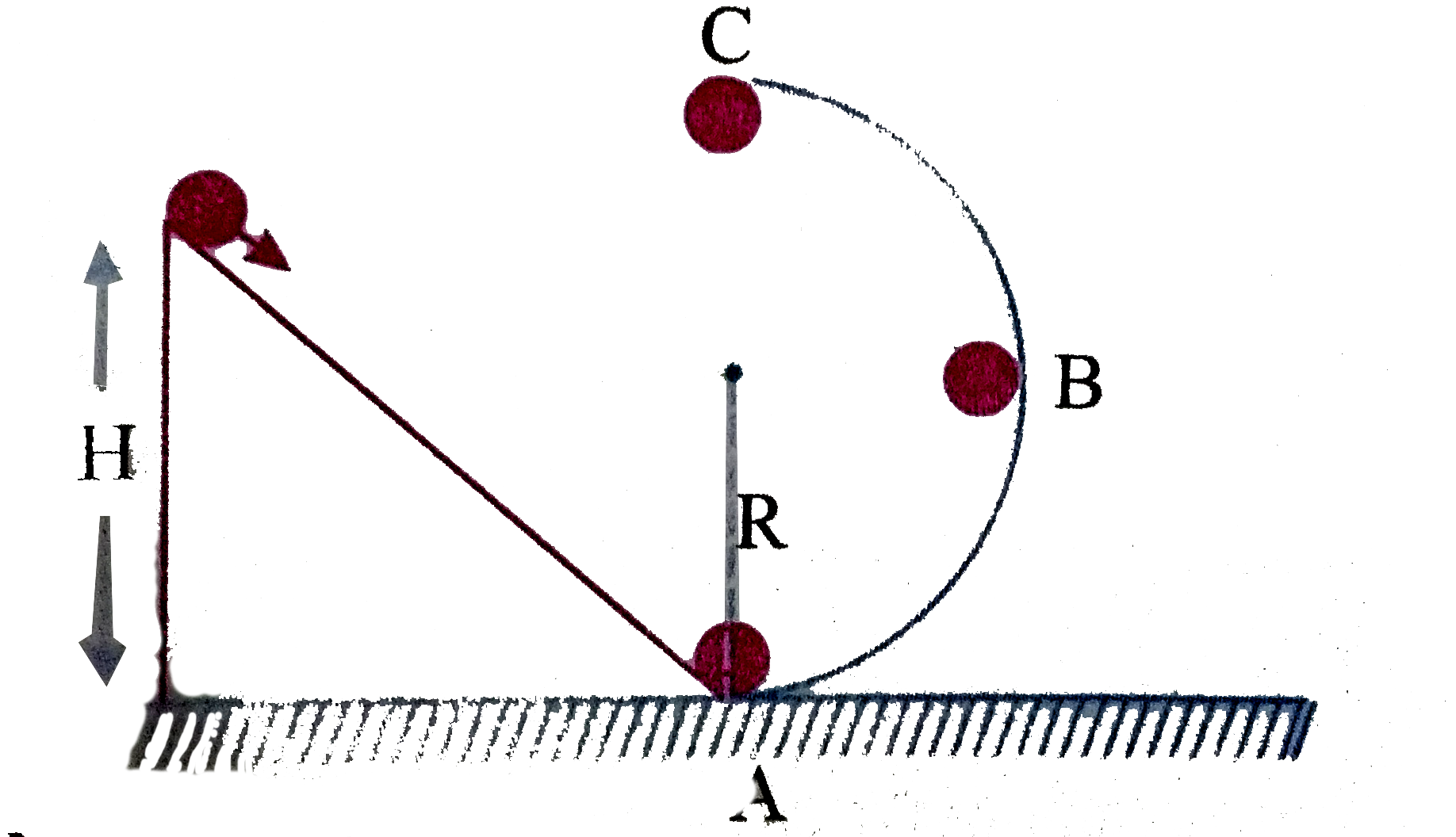 .
.
View SolutionA heavy particle hanging from a fixed point by a light inextensible string of length `l` is projected horizonally with speed `sqrt(gl)` . Find the speed of the particle and the inclination of the string to the vertical at the instant of the motion when the tension in the string is equal to the weight of the particle.
View SolutionA bullet of mass m moving at a speed v hits a ball of mass M kept at rest. A small part having mass m\' breaks from the ball and sticks to the bullet. The remaining ball is found to move at a speed `v_1` in the direction of the bullet. Find the velocity of the bullet after the collision.
View SolutionTwo bodies of masses `m_(1)` and `m_(2)` are moving with velocities `1 ms^(-1)` and `3ms^(-1)` respectively in opposite directions. If the bodies undergo one dimensional elastic collision, the body of mass `m_(1)` comes to rest. Find the ration of `m_(1)` and `m_(2)`.
View SolutionTwo identical balls `A and B` arereleased from the positions shown in figure. They collide elasticallly on horizontal position `MN` . The ratio of the heightsattaned by `A and B` after collisuion will be (neglect friction):
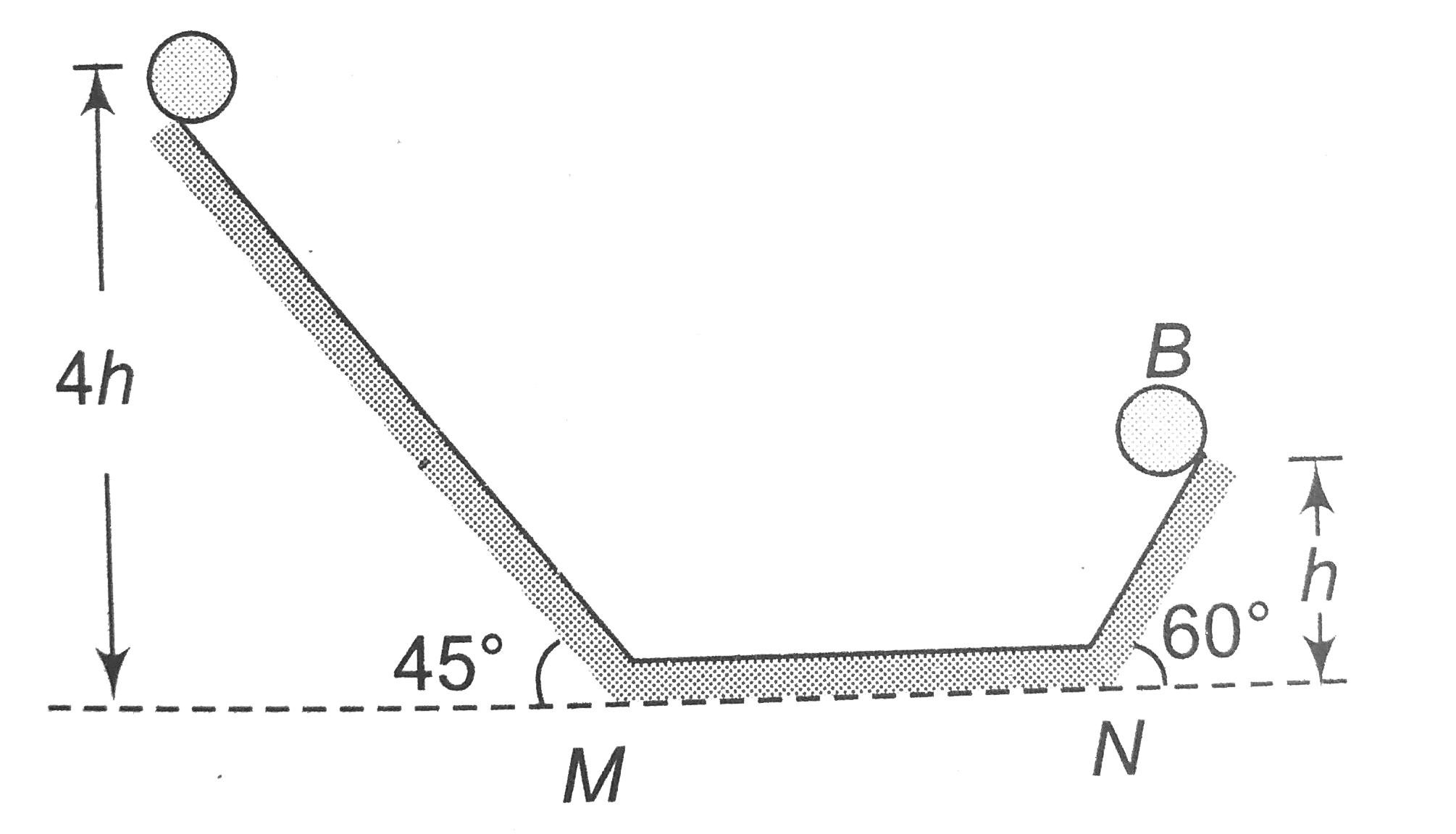
View Solution`n` elastic balls are placed at rest on a smooth horizontal plane which is circular at the ends with radius `r` as shown in the figure. The masses of the balls are `m`, `m/2`, `m/2^2`, …………….`(m)/(2^(n-1))` respectively. What is the minimum velocity which should be imparted to the first ball of mass `m` such that `n^th` ball completes the vertical circle
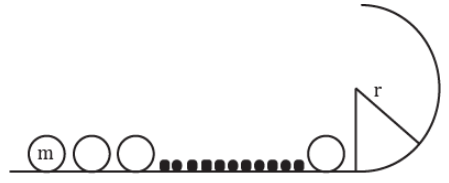
View SolutionBall 1 collides directly with another identical ball 2 at rest. Velocity of second ball becomes two times that of 1 after collison. Find the coefficient of restitution between the two balls?
View SolutionA body `X` with a momentum `p` with another identical stationary body `Y` one dimensionally. During the collision `Y` gives an impulse `J` to body `X`. Then coefficient of restitution is:
View SolutionA ball of mass `m` collides with the ground at an angle. With the vertical. If the collision lasts for time `t`, the average force exerted by the ground on the ball is : (e = coefficient of restitution between the ball and the ground)
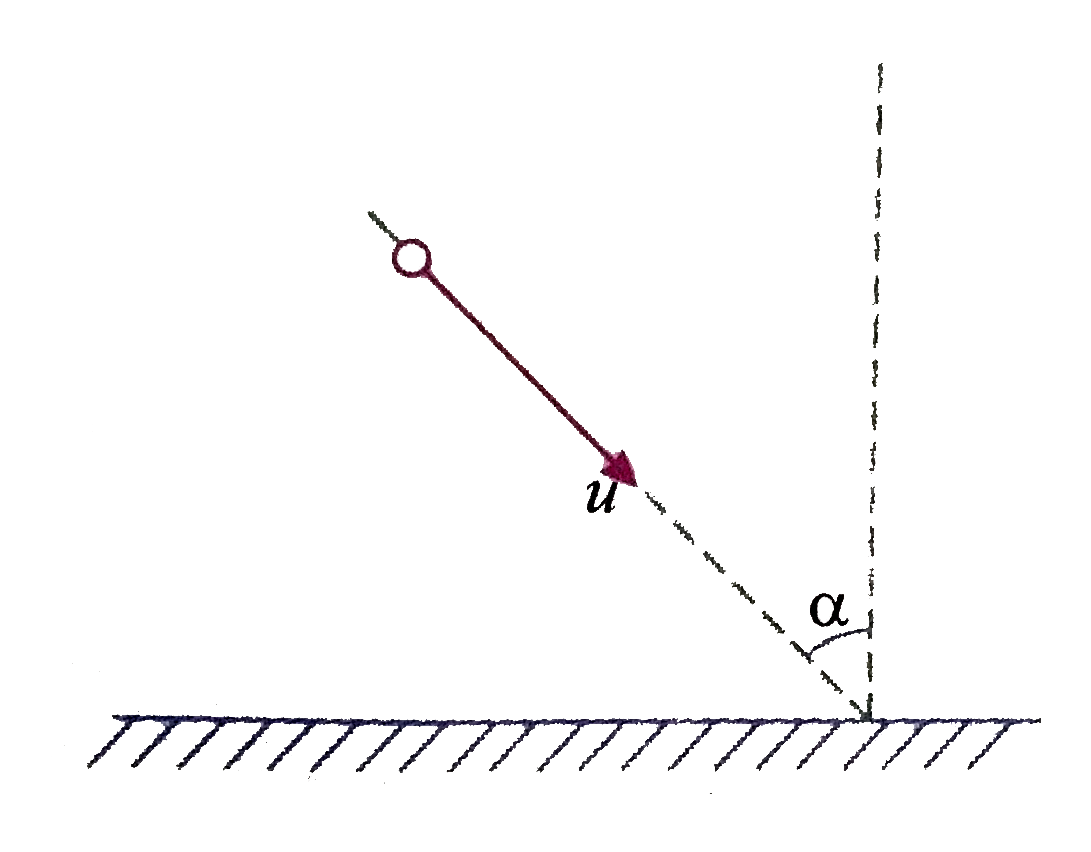 .
.
View SolutionA ball strickes a horizontal floor at an angle `theta = 45^@` with the normal to floor. The coefficient of restitution between the ball and the floor is `e = 1//2`. The function of its kinetic energy lost in the collision is.
View SolutionTwo equal sphere `A and b` lie on a smooth horizontal circle groove at opposite ends of a diameter. At time `t= 0,A` is projected along the groove and tis first implings on`B` at time `t = T_(1) and again at time `t = T_(2)`. If `e` is the coefficient of restitution, the ratio `T_(2)//T_(1)` is
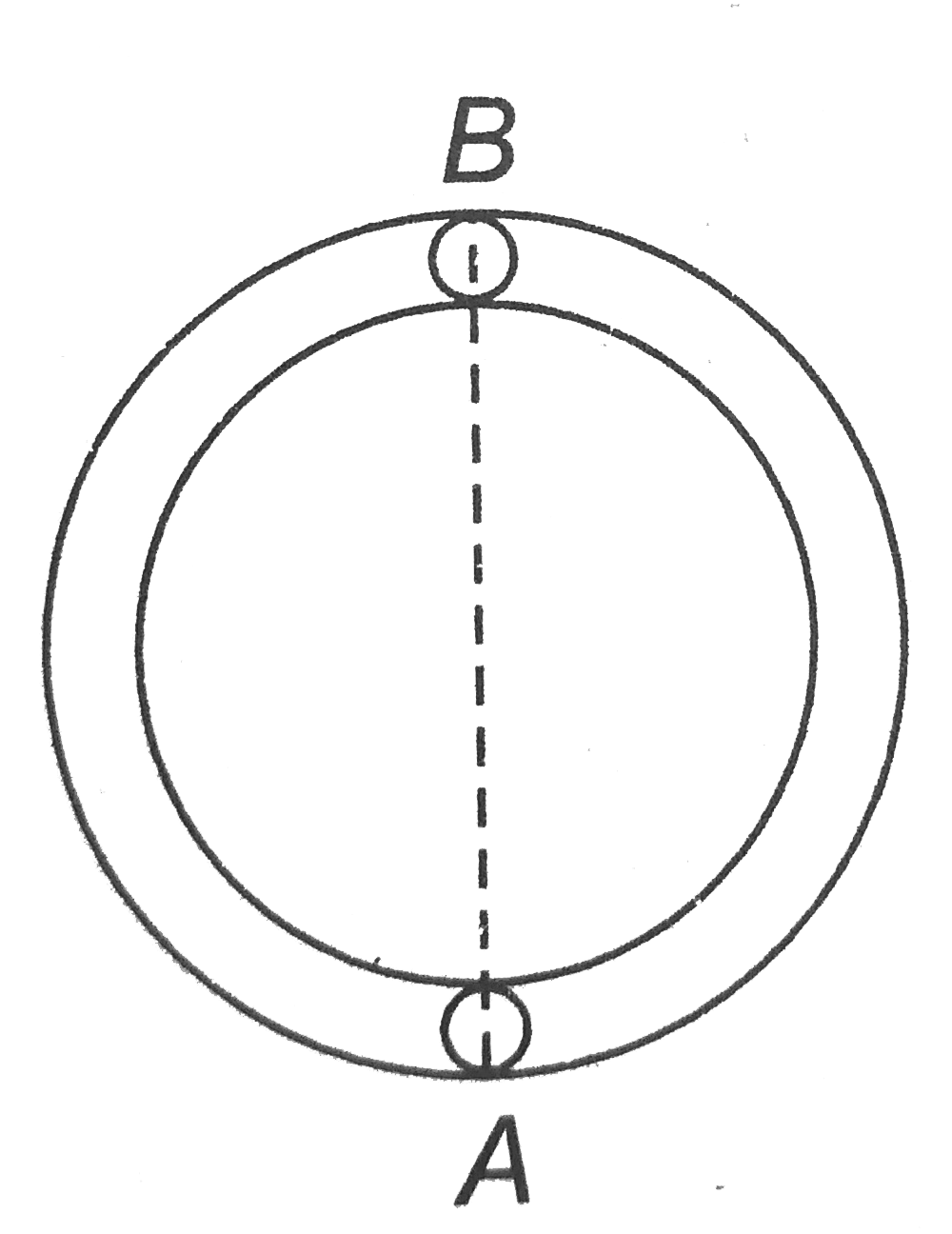
View SolutionAfter perfectly inelastic collision between two identical balls moving with same speed in different directions, the speed of the combined mass becomes half the initial speed. Find the angle between the two before collision.
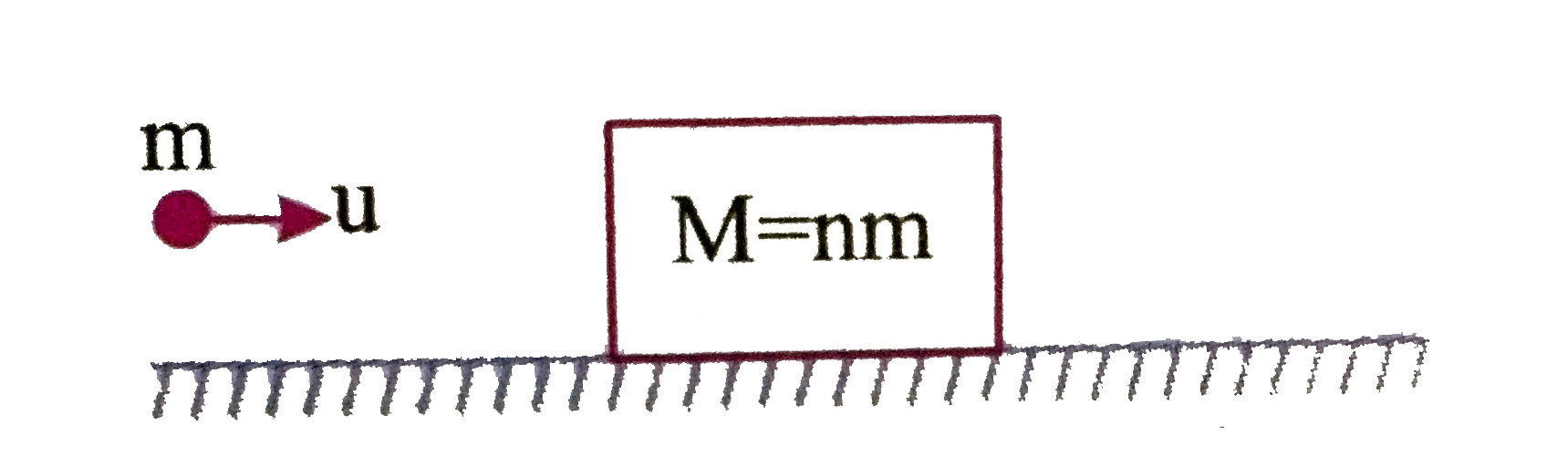
View SolutionA bullet of mass 'm' moving with velocity 'u' passes through a wooden block of mass `M = nm` as shown in figure. The block is resting on a smooth horizontal floor. After passing through the block, velocity relative to the block is
 .
.
View SolutionA block of mass `0.50kg` is moving with a speed of `2.00m//s` on a smooth surface. It strikes another mass of `1 kg` at rest and they move as a single body. The energy loss during the collision is
View SolutionConsider a rubber ball freely falling from a height ` h = 4.9 m` onto a horizontally elastic plate. Assume that the duration of collision is negligible and the collisions with the plate is totally elastic .
Then the velocity as a function of time and the height as a function of time will be :
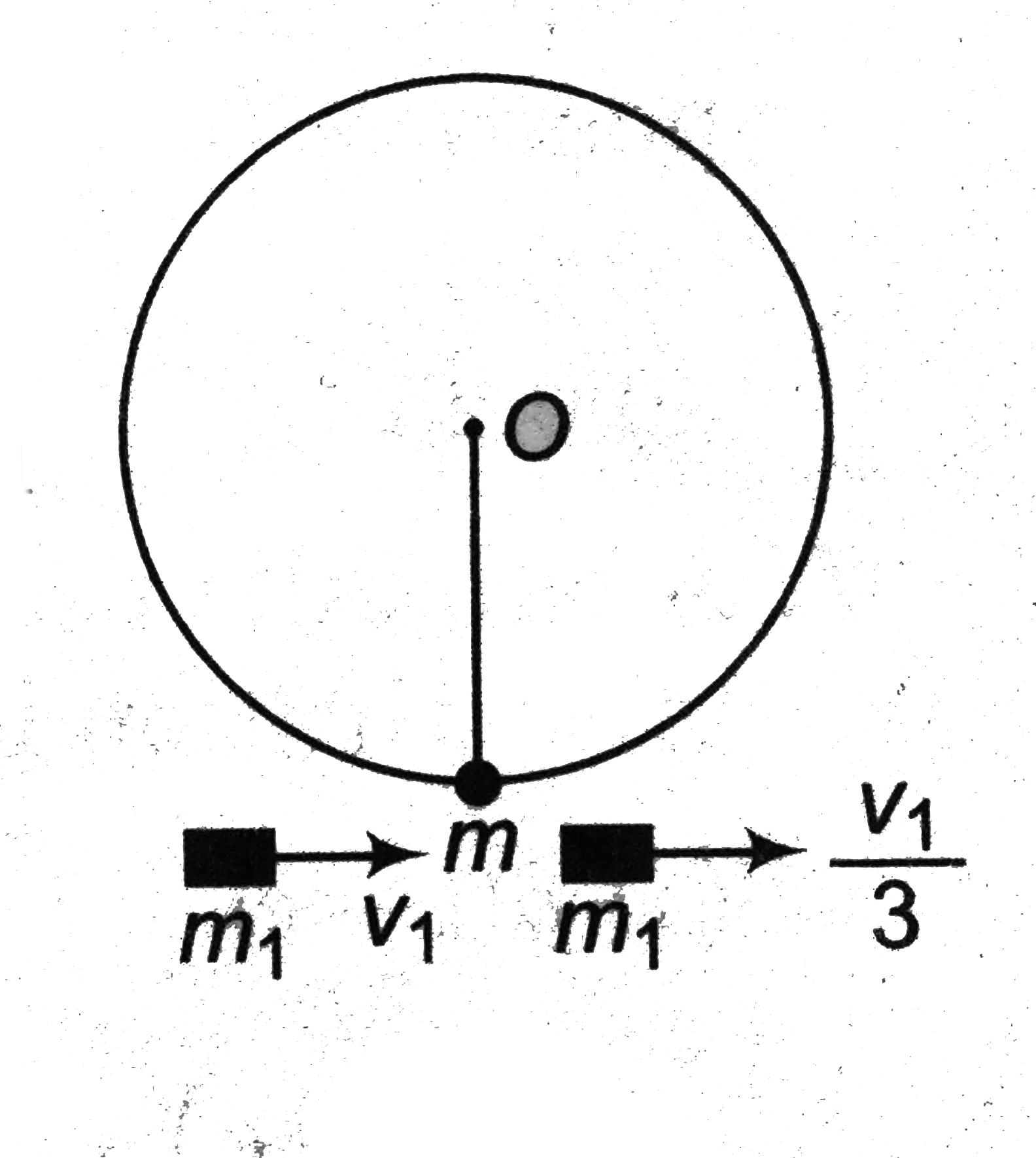
View SolutionA pendulum consists of a wooden bob of mass `m` and length `l`. A bullet of mass `m_1` is fired towards the pendulum with a speed `v_1` and it emerges from the bob with speed `v_1/3`. The bob just completes motion along a vertical circle. Then `v_1` is
View SolutionTwo billiard balls of same size and mass are in contact on a billiard table. A third ball of same mass and size strikes them symmetrically and remains at rest after the impact. Find the coefficient of restitution between the balls?
View Solution
Solved Example questions and complete solutions for chapter WORK POWER AND ENERGY of NARAYANA PHYSICS (HINGLISH) of Class 11