Complete Solutions to EXAMPLE of chapter KINEMATICS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | KINEMATICS |
| TAMILNADU GOVT | EXAMPLE |
| Number Of Questions | 45 |
| Academic Year | 2023-24 |
Two vectors `vecA` and `vecB` of magnitude 5 units and 7 units respectively make an angle `60^(@)` with each other as shown below. Find the magnitude of the resultant vector and its direction with respect to the vector G `vecA`.
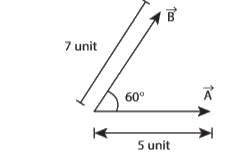
View SolutionTwo vectors `vecA` and `vecB` of magnitude 5 units and 7 units make an angle `60^(@)` with each other. Find the magnitude of the difference vector `vecA-vecB` and its direction with respect to the vector `vecA`.
View SolutionWhat are the unit vectors along the negative x–direction, negative y–direction, and negative z– direction?
View SolutionTwo vectors `vecA` and `vecB` are given in the component form as `vecA=5hati+7hatj-4hatk` and `vecB=6hati+3hatj+2hatk`. Find `vecA+vecB,vecB+vecA,vecA-vecB,vecB-vecA`
View SolutionGiven the vector `vecA=2hati+3hatj`, what is `3vecA`?
View SolutionA vector `vecA` is is given as in the following Figure. Find `4vecA` and `-4vecA`
View SolutionGiven two vectors `vecA=2hati+4hatj+5hatk` and `vecB=hati+3hatj+6hatk`, Find the product `vecA.vecB`, and the magnitudes of `vecA` and `vecB`. What is the angle between them?
View SolutionCheck whether the following vectors are orthogonal.
(i) `vecA=2hati+3hatj` and `vecB=4hati-5hatj`
(ii) `vecC=5hati+2hatj` and `vecD=2hati-5hatj`
View SolutionTwo vectors are given as `vecr=2hati+3hatj+5hatk` and `vecF=3hati-2hatj+4hatk`. Find the resultant vector `vectau=vecrxxvecF`
View SolutionCompare the components for the following vector equations
`vecF=mveca` Here m is positive number
(b) `vecP=0`
View SolutionDetermine the value of the T from the given vector equation.
`5hatj-Thatj=6hatj+3Thatj`
View SolutionCompare the components of vector equation `vecF_(1)+vecF_(2)+vecF_(3)=vecF_(4)`
View SolutionDetermine the position vectors for the following particles which are located at points P, Q, R, S.
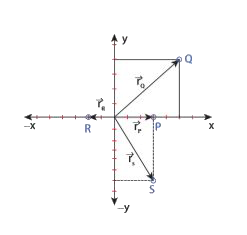
View SolutionA person initially at rest starts to walk 2 m towards north, then 1 m towards east, then 5 m towards south and then 3 m towards west. What is the position vector of the person at the end of the trip?
View SolutionAssume your school is located 2 km away from your home. In the morning you are going to school and in the evening you come back home. In this entire trip what is the distance travelled and the displacement covered?
View SolutionAn athlete covers 3 rounds on a circular track of radius 50 m. Calculate the total distance and displacement travelled by him.
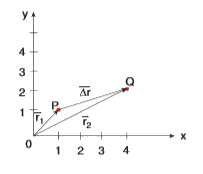
View SolutionCalculate the displacement vector for a particle moving from a point P to Q as shown below. Calculate the magnitude of displacement.
View SolutionConsider the function `y=x^(2)`. Calculate the derivative `(dy)/(dx)` using the concept of limit.
View SolutionFind the derivative with respect to t, of the function `x=A_(0)+A_(1)t+A_(2)t^(2)` where `A_(0),A_(1)` and `A_(2)` are constants.
View SolutionConsider an object travelling in a semi- circular path from point O to point P in 5 second, as is shown in the Figure. Calculate the average velocity and average speed.

View SolutionThe position vector of a particle is given `vecr=2thati+3hatj-5hatk`.
Calculate the velocity and speed of the particle at any instant t
View SolutionThe position vector of a particle is given `vecr=2thati+3hatj-5hatk`.
Calculate the velocity and speed of the particle at time t = 2 s
View SolutionThe velocity of three particles A, B, C are given below. Which particle travels at the greatest speed?
`vec(v_(A))=3hati-5hatj+2hatk`
`vec(v_(B))=hati+2hatj+3hatk`
`vec(v_(C))=5hati+3hatj+4hatk`
View SolutionTwo cars are travelling with respective velocities `vecv_(1)=10ms^(-1)` along east and `vecv_(2)=10ms^(-1)` along west. What are the speeds of the cars?
View SolutionConsider two masses of 10 g and 1 kg moving with the same speed `10ms^(-1)`. Calculate the magnitude of the momentum.
View SolutionA particle moves along the x-axis in such a way that its coordinates x varies with time 't' according to the equation `x=2-5t+6t^(2)`. What is the initial velocity of the particle?
View SolutionSuppose two cars A and B are moving with uniform velocities with respect to ground along parallel tracks and in the same direction. Let the velocities of A and B be `35kmh^(−1)` due east and `40kmh^(−1)` due east respectively. What is the relative velocity of car B with respect to A?
View SolutionSuppose two trains A and B are moving with uniform velocities along parallel tracks but in opposite directions. Let the velocity of train A be `40kmh^(−1)` due east and that of train B be `40kmh^(−1)` due west. Calculate the relative velocities of the trains
View SolutionConsider two trains A and B moving along parallel tracks with the same velocity in the same direction. Let the velocity of each train be `50kmh^(−1)` due east. Calculate the relative velocities of the trains.
View SolutionHow long will a boy sitting near the window of a train travelling at `36kmh^(−1)` see a train passing by in the opposite direction with a speed of `18kmh^(−1)`. The length of the slow- moving train is 90 m.
View SolutionA swimmer’s speed in the direction of flow of a river is `12kmh^(−1)`. Against the direction of flow of the river the swimmer’s speed is `6kmh^(−1)`. Calculate the swimmer’s speed in still water and the velocity of the river flow.
View SolutionA velocity–time graph is given for a particle moving in x direction, as below
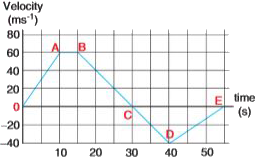
Describe the motion qualitatively in the interval 0 to 55 s .
View SolutionA velocity–time graph is given for a particle moving in x direction, as below
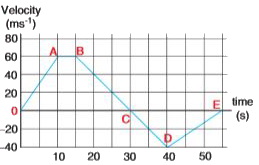
Find the distance and displacement travelled from 0 s to 40 s .
View SolutionA velocity–time graph is given for a particle moving in x direction, as below
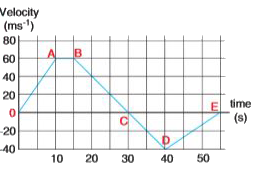
Find the acceleration at t = 5 s and at t = 20 s
View SolutionIf the position vector of the particle is given by `vecr=3t^(2)hati+5thatj+4hatk`, Find the
The velocity of the particle at t = 3 s
View SolutionIf the position vector of the particle is given by `vecr=3t^(2)hati+5thatj+4hatk`, Find the
Speed of the particle at t = 3 s
View SolutionIf the position vector of the particle is given by `vecr=3t^(2)hati+5thatj+4hatk`, Find the
acceleration of the particle at time t = 3 s
View SolutionAn object is thrown vertically downward. What is the acceleration experienced by the object?
View SolutionAn iron ball and a feather are both falling from a height of 10 m.
What are the time taken by the iron ball and feather to reach the ground?
b) What are the velocities of iron ball and feather when they reach the ground? (Ignore air resistance and take `g=10ms^(-2)` )
View SolutionA train was moving at the rate of `54kmh^(−1)` when brakes were applied. It came to rest within a distance of 225 m. Calculate the retardation produced in the train.
View SolutionSuppose an object is thrown with initial speed `10 ms^(-1)` at an angle `pi//4` with the horizontal, what is the range covered? Suppose the same object is thrown similarly in the Moon, will there be any change in the range? If yes, what is the change? (The acceleration due to gravity in the Moon `g_("moon")=(1)/(6)g` )
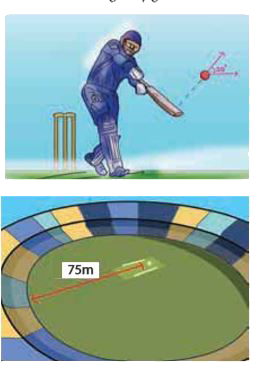
View SolutionIn the cricket game, a batsman strikes the ball such that it moves with the speed `30ms^(-1)` at an angle `30^(@)` with the horizontal as shown in the figure. The boundary line of the cricket ground is located at a distance of 75 m from the batsman? Will the ball go for a six? (Neglect the air resistance and take acceleration due to gravity `g=10ms^(-2)` ).
View SolutionCalculate the angle `theta` subtended by the two adjacent wooden spokes of a bullock cart wheel is shown in the figure. Express the angle in both radian and degree.

View SolutionA particle moves in a circle of radius 10 m. Its linear speed is given by vt 3 where t is in second and v is in `ms^(-1)`.
(a) Find the centripetal and tangential acceleration at t = 2 s.
(b) Calculate the angle between the resultant acceleration and the radius vector.
View SolutionA particle is in circular motion with an acceleration `alpha=0.2rads^(-2)`.
(a) What is the angular displacement made by the particle after 5 s?
(b) What is the angular velocity at t = 5 s?. Assume the initial angular velocity is zero.
View Solution
EXAMPLE questions and complete solutions for chapter KINEMATICS of FULL MARKS PHYSICS(TAMIL) of Class 11