Complete Solutions to Exercise of chapter VECTOR ALGEBRA of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | VECTOR ALGEBRA |
| KC SINHA | Exercise |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
Classify the following measures as scalars and vector:` 5 seconds`.
View SolutionClassify the following measures as scalars and vector:` 3 km/hr
View SolutionClassify the following measures as scalars and vector:`(10 gm)/(cm^3)`
View SolutionClassify the following measures as scalars and vector: 10 Newton
View SolutionClassify the following measures as scalars and vector:`20m/sec towards north`
View SolutionClassify the following measures as scalars and vector: `1000 cm^3`
View SolutionClasify the following quantities as scalars and vector: 10 kg
View SolutionClasify the following quantities as scalars and vector: `20 cm/sec^3`
View SolutionClasify the following quantities as scalars and vector: ` 50 m/second`
View SolutionClasify the following quantities as scalars and vector: `20 m/sec` towards west
View SolutionClasify the following quantities as scalars and vector: `50 kg weight
View SolutionClasify the following quantities as scalars and vector: `100^0C`
View SolutionClasify the following quantities as scalars and vector: 100 kg weight
View SolutionClasify the following quantities as scalars and vector: `30^0`
View SolutionClasify the following quantities as scalars and vector: charge
View SolutionClasify the following quantities as scalars and vector: energy
View SolutionClasify the following quantities as scalars and vector: potential
View SolutionClasify the following quantities as scalars and vector: displacement
View SolutionRepresent graphically a displacement of 50 km, `50^o`west of south.
View SolutionRepresent graphically: A displacement of 20 m, north east.
View SolutionRepresent graphically: A displacement of 50 m, `60^0` south of east
View SolutionRepresent the following graphically: A displacement of 40 km, `30^0` east of north A displacement of 50 km south east A displacement of 70 km, `40^0` north of west
View SolutionRepresent graphically: a displacement of `40 km, 20^0` east of south
View SolutionRepresent graphically a displacement of : `20 km` south west
View SolutionRepresent graphically a displacement of : `60 km ,`40^o` north of west
View SolutionIn the adjoining figure which of the vector are: collinear
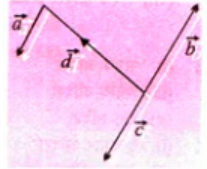
View SolutionIn the adjoining figure which of the vector are: cointial

View SolutionIn the adjoining figure which of the vector are: equal

View SolutionIn the adjoining figure ABCD is a rectangle . Examine which of the vector are: equal
View SolutionIn the adjoining figure ABCD is a rectangle . Examine which of the vector are: collinear
View SolutionIn the adjoining figure ABCD is a rectangle . Examine which of the vector are: coinitial
View SolutionIn the adjoining figure ABCD is a rectangle . Examine which of the vector are: collinear but not equal
View SolutionIn the given figure ABCDEF is a regular hexagon. Examine which vector are, equal
View SolutionIn the given figure ABCDEF is a regular hexagon. Examine which vector are, collinear
View SolutionIn the given figure ABCDEF is a regular hexagon. Examine which vector are, Cointial
View SolutionIn the given figure ABCDEF is a regular hexagon. Examine which vector are, Collinear but not equal
View SolutionThe position vector of foru points A,B,C,D are `veca, vecb, 2veca+3vecb and veca-2vecb respectively. Expess the vectors `vec(AC), vec(DB), vec(BC) and vec(CA)` in terms of `veca and vecb`.
View SolutionIf `AD, BE and CF` be the median of a `/_\ABC`, prove that `vec(AD)+vec(BE)+vec(CF)`=0
View SolutionIf G is the centroid of `/_\ABC, `prove that `vec(GA)+vec(GB)+vec(GC)`=0. Further if `G_1` bet eh centroid of another `/_\PQR`, show that `vec(AP)+vec(BQ)+vec(CR)=3vec(GG_1)`
View SolutionFive forces ` vec A B` , ` vec A C` , ` vec A D` , ` vec A E` and ` vec A F` act at the vertex of a regular hexagon `A B C D E Fdot` Prove that the resultant is `6 vec A O` , where `O` is the centre of hexagon.
View SolutionIf ABCDEF is a regular hexagon, prove that `vec(AC)+vec(AD)+vec(EA)+vec(FA)=3vec(AB)`
View SolutionABCD is a parallelogram E and F are the middle points of AD and CD respectively. Express `vec(BE) and vec(BF)` in terms of `veca and vecb , where vec(BA)=vecaand vec(BC)=vecb`.
View SolutionIf D and E are the mid-points of sides AB and AC of a triangle `A B C` respectively, show that ` vec B E+ vec D C=3/2 vec B Cdot`
View SolutionIn trapezium PQRS, given that `QR|| PS and 2QR=PS. If ``vec(PQ)=veca,vec(QR)=vecb and vec(RS)=vecc`, express `veca` in terms `vecb and vecc`
View SolutionOX, OY and OZ are three edges of a cube andn P,Q,R are the vertices of rectangle OXPY, OXQZ and OYSZ respectively. If `vec(OX)=vecalpha, vec(OY)=vecbeta and vec(OZ)=vecgamma express vec(OP), vec(OQ), vec(OR) and vec(OS) in erms of vecalpha, vecbeta and vecgamma.
View SolutionIf `veca+2vecb+3vecc, 2veca+8vecb+3vecc, 2veca+5vecb-vecc and veca-vecb-vecc` be the positions vectors A,B,C and D respectively, prove that `vec(AB) and vec(CD)` are parallel. Is ABCD a parallelogram?
View SolutionIf `A B C D` is quadrilateral and `Ea n dF` are the mid-points of `A Ca n dB D` respectively, prove that ` vec A B+ vec A D` +` vec C B` +` vec C D` =4 ` vec E Fdot`
View Solution`A B C D` is parallelogram and `P` is the point of intersection of its diagonals. If `O` is the origin of reference, show that ` vec O A+ vec O B+ vec O C+ vec O D=4 vec O Pdot`
View Solution`veca,vecb,vecc` are the position vectors of vertices A, B, C respectively of a paralleloram, ABCD, ifnd the position vector of D.
View SolutionFind the sum of the vectors `vec=hati-2hatj+hatk, vecb=-2hati+4 hatj+5hatk and vecc=hati-6hatj-7hatk`
View SolutionFind the scalar and vector components of the vector with initial point `A(2,1)` and terminal point `B\ (-5,7)dot`
View SolutionIf the position vectors of A and B respectively `hati+3hatj-7hatk and 5 hati-2hatj+4hatk`, then find
View SolutionFind the vector joining the points `P(2, 3, 0)`and `Q( 1, 2, 4)`directed from P to Q.
View SolutionFind the values of x for which `x(hati+hatj+hatk)` is a unit vector
View SolutionFind unit vector in the direction of vector ` -> a=2 hat i+3 hat j+ hat k`.
View SolutionFind a unit vector in the direction of the vector ` vec a=3 hat i-2 hat j+6 hat kdot`
View SolutionFind the direction cosines of the vector: `hati+2hatj+6hatk`
View SolutionFindthe vector in the directionof the vector `-hati+2hatj+2hatk` that has magnitude 7.
View SolutionFind a vector in the direction of vector `veca= hat i-2 hat j` `that has magnitude 7 units.
View SolutionIf `vec(OP)=2hati+3hatj-hatk and vec(OQ)=5hati+4hatj-3hatk`. Find `vec(PQ)` and the direction cosines of `vec(PQ)`.
View SolutionThe position vectors of two points A and B are `hati+hatj+hatk` and `5hati-3hatj+hatk`. Find a unit vector in direction of `vec(AB)`, and also find the direction cosines of `vec(AB)`. What angles does `vec(AB)` make with the three axes?
View SolutionWrite the direction ratios of the vector ` -> a= hat i+ hat j-2 hat k` and hence calculate its direction cosines.
View SolutionFind the unit vector in the direction of vector ` -> P Q` , where P and Q are the points (1, 2, 3) and (4, 5, 6), respectively.
View SolutionIf `P-=(1,5,4) and Q-=(4,1,-2)` find the direction ratios of `vec(PQ)`
View SolutionIf `veca=hati+hatj+hatk, vecb=2hati-hatj+3hatk and vecc=hati-2hatj+hatk` find a unit vector parallel to ther vector `2veca-vecb+3c`.
View SolutionIf`veca=hati+2hatj-hatk and vecb=3hati+hatj-hatk` find a unit vector int direction of `veca-vecb` .
View SolutionThe position vectors of four points P,Q,R annd S are 2a+4c,5a+`3sqrt(3)b+4c,-2sqrt(3)b+c and 2a+c` respectively, prove that PQ is parallel to RS.
View SolutionFind the lengths of the sides of the triangle whose vertices are `A(2,4,-1),(4,5,1),C(3,6,-3)` and show that the triangle is right angled.
View SolutionThe vectors `2hati-hatj+hatk,hati-3hatj-5hatk` and `3hati-4hatj-4hatk` forms a/an
View SolutionIf position vectors of P,Q,R,S be respectively `2hati+4hatk, 5hati+4hatj+4hatk, -4hati-8hatj+hatk, 2hati+hatk`, prove that RS is parallel to PQ and is twice of PQ.
View SolutionThe position vectors of the points P,Q,R,S are `hati+hatj+hatk, 2hati+5hatj,3hati+2hatj-3hatk and hati-6hatj-hatk.` Prove that the lines PQ and RS are prallel and find the ratio of their lengths.
View SolutionProve that the three points whose positions vectors are `3hati-hatj+2hatk, hati-hatj-3hatk and 4hati-3hatj+hatk` form an isosceles tirangle.
View SolutionProve that the vecotos `3hati+5hatj+2hatk, 2hati-3hatj-5hatk and 5hati+2hatj-3hatk` form the sides of an equlateral triangle.
View SolutionProve that the points `hati-hatj, 4hati-3hatj+ hatk and 2hati-4hatj+5hatk` are the vertices of a right angled triangle.
View SolutionUsing dot product of vectors show that the vectors `2hati-hatj+hatk, hati-3hatj-5hatk and 3hati-4hatj-4hatk` form a righat angled triangle
View SolutionFind as unit vector paralel to the sum of the vectors `2hati+3hatj-5hatk and hati+2hatj+3hatk`
View SolutionThe two adjacent sides of a parallelogram are `2 hat i-4 hat j+5 hat k` and ` hat i-2 hat j-3 hat k` . Find the unit vector parallel to one of its diagonals. Also, find its area.
View SolutionFind the unit vector in the direction of the sum of the vectors `veca=2hati+2hatj-5hatk and vecb=2hati+hatj+3hatk`.
View SolutionFind a vector magnitude 5 units, and parallel to the resultant of the vectors ` vec a=2 hat i+3 hat j- hat k` and ` vec b= hat i-2 hat j+ hat kdot`
View SolutionLet `veca=hati+2hatj and vecb=2hati+hatj. Is |veca|=|vecb|` Are the vectors `veca and vecb ` equal?.
View SolutionFind the values of x,y and z so that the vectors `veca=xhati+2hatj+zhatk and vecb=2hati+yhatj+hatk` are equal.
View SolutionIf `veca and vecb` are non-collinear vectors and `vecA= (p+4q)veca+ (2p+q+1)vecb and vecB= (-2p+q+2)veca+ (2p-3q-1)vecb`, and if `3vecA= 2vecB`, then determine `p and q`.
View SolutionFind the all the values of lamda such that `(x,y,z)!=(0,0,0)`and `x(hati+hatj+3hatk)+y(3hati-3hatj+hatk)+z(-4hati+5hatj)=lamda(xhati+yhatj+zhatk)`
View SolutionCheck whether the following sets of three points are collinear: `-2veca+3vecb+5vecc, veca+2vecb+3vecc, 6veca-vecc`
View SolutionProve th the following sets of three points are collinear: `2hati+hatj-hatk, 3hati-2hatj+hatk and hati+4hatj-3hatk`
View SolutionThe points with position vectors `60hati+3hatj,40hati-8hatj, ahati-52hatj` are collinear if (A) `a=-40` (B) `a=40` (C) `a=20` (D) none of these
View SolutionProve that the ponts `A(1,2,3),B(3,4,7),C(-3 ,-2, -5)` are collinear and find the ratio in which B divides AC.
View SolutionVectors `veca and vecb` are non-collinear. Find for what value of x vectors `vecc=(x-2)veca+vecb and vecd=(2x+1)veca-vecb` are collinear ?
View SolutionIf `veca,vecb,vecc` are non zero and non coplanar vectors show that the following vector are coplanar: `2veca-3vecb+4vecc, -veca+3vecb-5vecc,-veca+2vecb-3vecc`
View SolutionIf `veca,vecb,vecc` are non zero and non coplanar vectors show that the following vector are coplanar: `5veca+6vecb+7vecc,7veca-8vecb+9vecc, 3veca+20vecb+5vecc`
View SolutionIf `veca,vecb,vecc` are non zero and non coplanar vectors show that the following vector are coplanar: `4veca+5vecb+vecc, -vecb-vecc, 5veca+9vecb+4vecc`
View SolutionIf `veca,vecb,vecc` are non coplanar vectors, check whether the following points are coplanar: `6veca+2vecb-vecc, 2veca+vecb+3vecc, -veca+2vecb-4vecc, -12veca-vecb-3vecc`
View SolutionIf `veca,vecb,vecc` are non coplanar vectors, prove that the following points are coplanar: `6veca-4vecb+10vecc, -5veca+3vecb-10vecc, 4veca-6vecb-10vecc,2vecb+10vecc`
View SolutionIf the vectors `2hati-hatj+hatk,hati+2hatj-3hatk and 3hati+ahatj+5hatk` are coplanar, the prove that a=4.
View SolutionIf `veca, vecb, vecc`, be three on zero non coplanar vectors estabish a linear relation between the vectors: `4veca+5vecb+vecc,-vecb-vecc, 3veca+9vecb+4vecc,-4veca+4vecb+4vecc`
View SolutionIf `veca, vecb, vecc`, be three on zero non coplanar vectors estabish a linear relation between the vectors: ` 8vecb+6vecc, veca+vecb+vecc, 2veca-vecb+vecc, veca-vecb-vecc`
View SolutionExamine whather followig vectors are coplanar or not: `5veca +6vecb + 7vecc, 7veca - 8vecb + 9vecc, 3veca + 20 vecb + 5vecc`
View SolutionExamine whether the following vectors from a linearly dependent or independent set of vector: `hati+3hatj+5hatk, 2hati+6hatj+10hatk`
View SolutionExamine whether the following vectors from a linearly dependent or independent set of vector: `veca=(1,-2,30),vecb=(-2,3,-4),vecc=(1,-1,5)``
View SolutionExamine whether the following vectors from a linearly dependent or independent set of vector: ` veca-3vecb+2vecc, veca-9vecb-vecc,3veca+2vecb-vecc where veca,vecb,vecc` are non zero non coplanar vectors
View Solution
Exercise questions and complete solutions for chapter VECTOR ALGEBRA of KC SINHA ENGLISH of Class 12