Complete Solutions to CONCEPT APPLICATION of chapter GEOMETRY of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | GEOMETRY |
| PEARSON IIT JEE FOUNDATION | CONCEPT APPLICATION |
| Number Of Questions | 61 |
| Academic Year | 2023-24 |
In the adjoining figure, `DeltaABC` is right angled at `C` and `M` is the mid-point of hypotenuse `AB`, If `AC = 32 cm` and `BC = 60 cm,` then find the length of `CM.`

View SolutionA cyclic polygon has n sides such that each of its interior angle measures `144^(@)`. What is the measures of the angle subtended by each of its side at the geometrical center of the polygon.
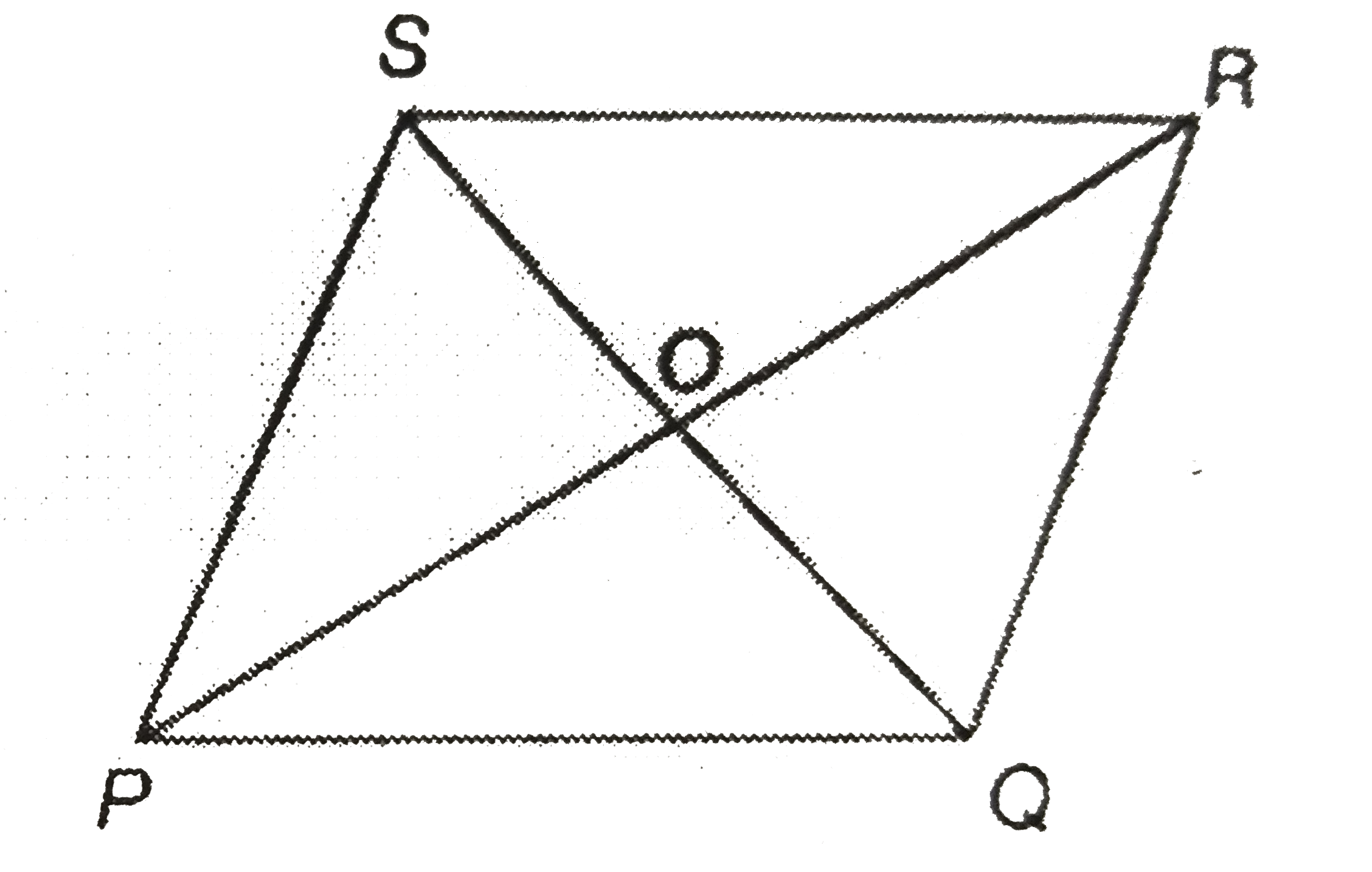
View SolutionIn the following, `PQRS` is a rhombus, `SQ` and `PR` are the diagonals of the rhombus intersecting at `O`. If angle `OPQ = 35^(@)`, then find the value of angle ORS + angle OQP.
View SolutionIn the following, CDEF is a cyclic quadrilateral. `bar(CG)` and `bar(DH)` are the angle bisectors of `angleC` and `angleD` respectively. If `angleE=100^(@)` and `angleF=110^(@)`, then find `angleCPD`.

View SolutionIn the following figure, `ABC` is an equilateral triangle. `DE` is parallel to `BC` and equal to half the lengths of BC. If `AD+ EC+ CB=24 cm`, then What is the perimeter of triangle `ADE`?

View SolutionIn `trianglePQR`, M and N are points on `PQ` and `PR`, respectively, such that `bar(MN) || bar(QR)`, If PM =`x`, PR `=x+9, PQ=x+13` and `PN=x-2`, then find `x`.
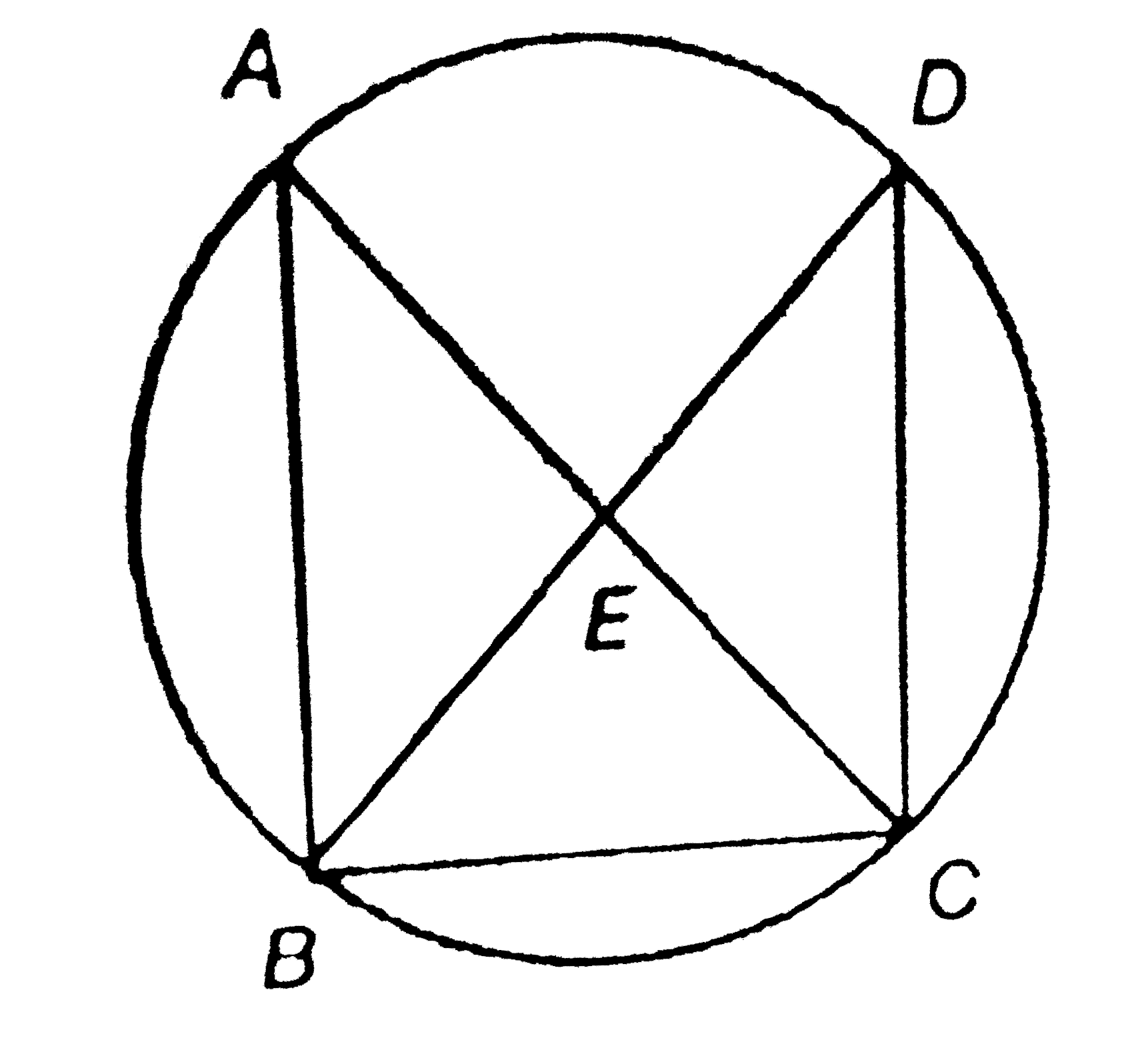
View SolutionIn the following figure (not to scale), the chords AC and BD intersects at E and `angleBAE=angleECD + 20^(@)`. If `angleCDE=60^(@)`, find `angleABE`.
View SolutionIn the following figure, `bar(DE)` and `bar(FG)` are equal chords of the circle subtending `angleDHE` and `angleFHG` at the point H on the circle. If `angleDHE=23 (1/2)^(2)`, then find `angleFHG`.

View SolutionThe bisector of two adjacent angles in a parallelogram meet at a point `P` inside the parallelogram. The angle made by these bisectors at a point is ……………………
View SolutionIf `x^(@)` is the measure of an angle which is equal to its complement and `y^(@)` is the measure of an angle which is equal to its supplement, then `x^(@)/y^(@)` is……………………
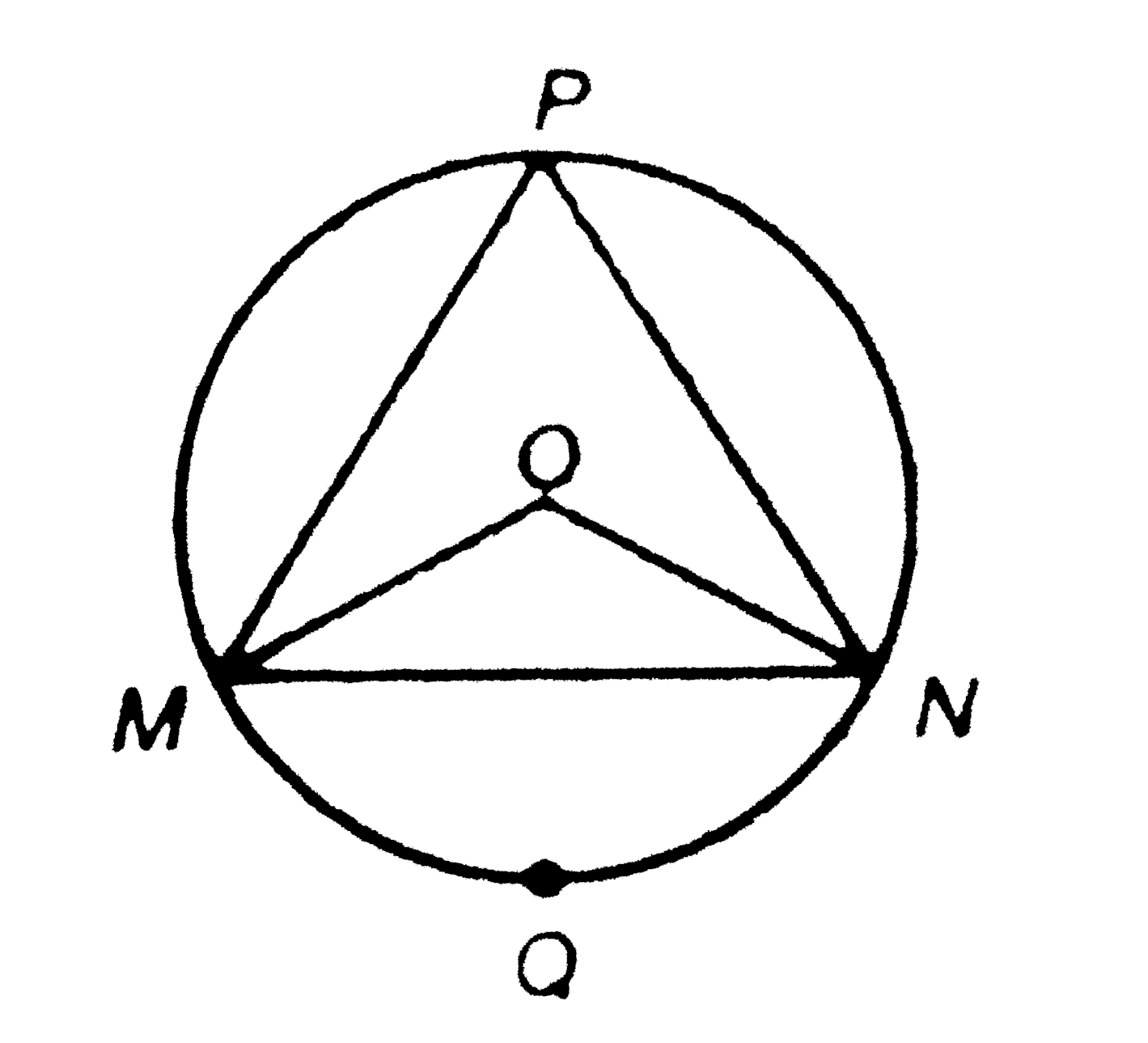
View SolutionIn the following figure, O is the center of the circle. If `angleMPN = 55^(@)`, then find the value of `angleMON+angleOMN +1/2angleMNO`.
View SolutionIn the following figure, `trianglePQR` is right-angled at `R` and `S` is the mid-point of hypotenuse `PQ`. If `RS=25 cm` and `PR=48 cm,` then find `QR.`
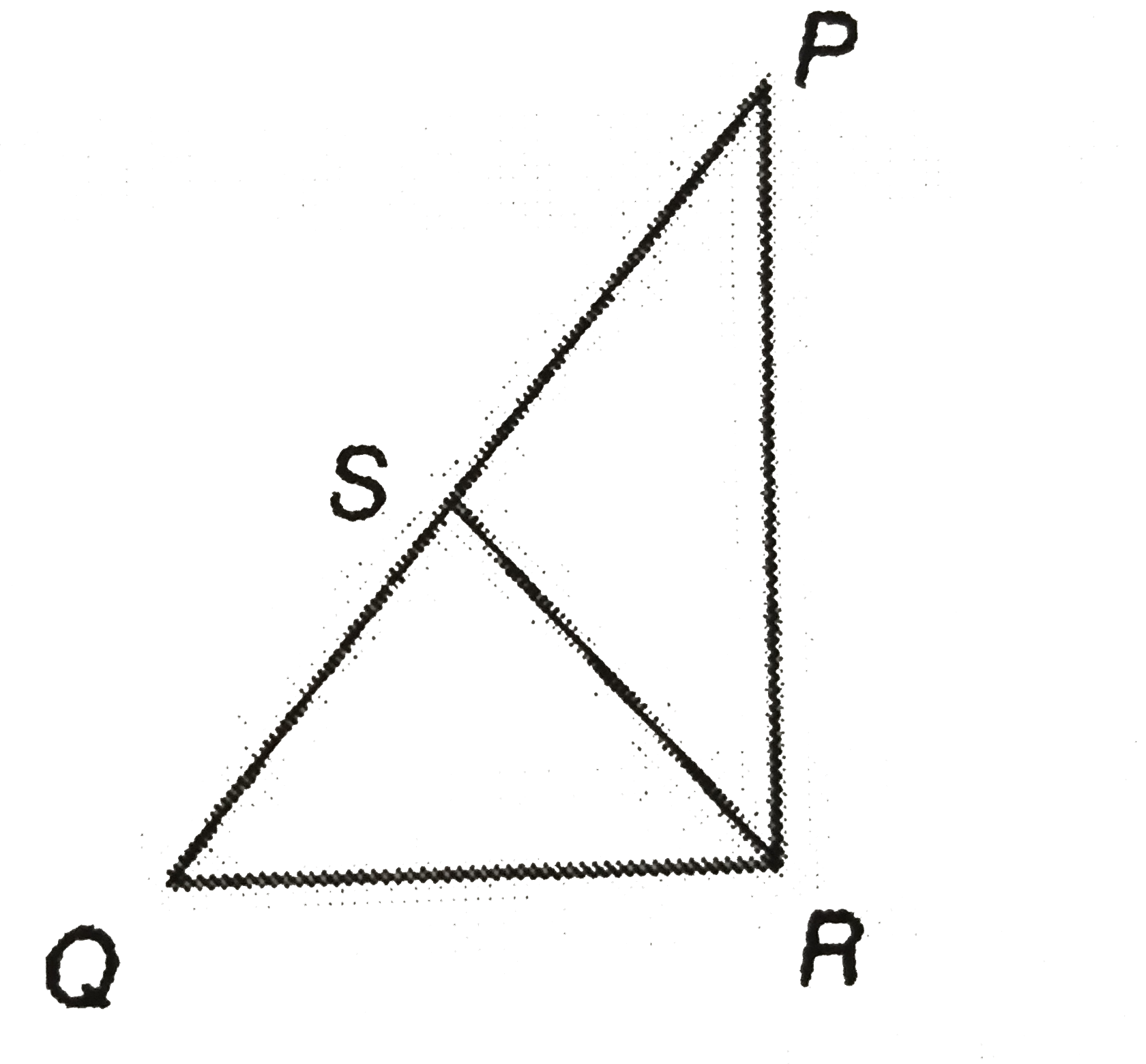
View SolutionIn a cyclic quadrilateral `PQRS, PS=PQ ,RS=RQ` and `anglePSQ = 2angleQSR`, Find `angleQSR`.
View SolutionIn the following figure, two isosceles right angled triangles, DEF and HGI are on the same base `bar(DH)` and `bar(DH)` is parallel to `bar(FI)`. If DE=GH=9 cm and DH=20 cm, then the area of the quadrilateral `FEGI` is………………….
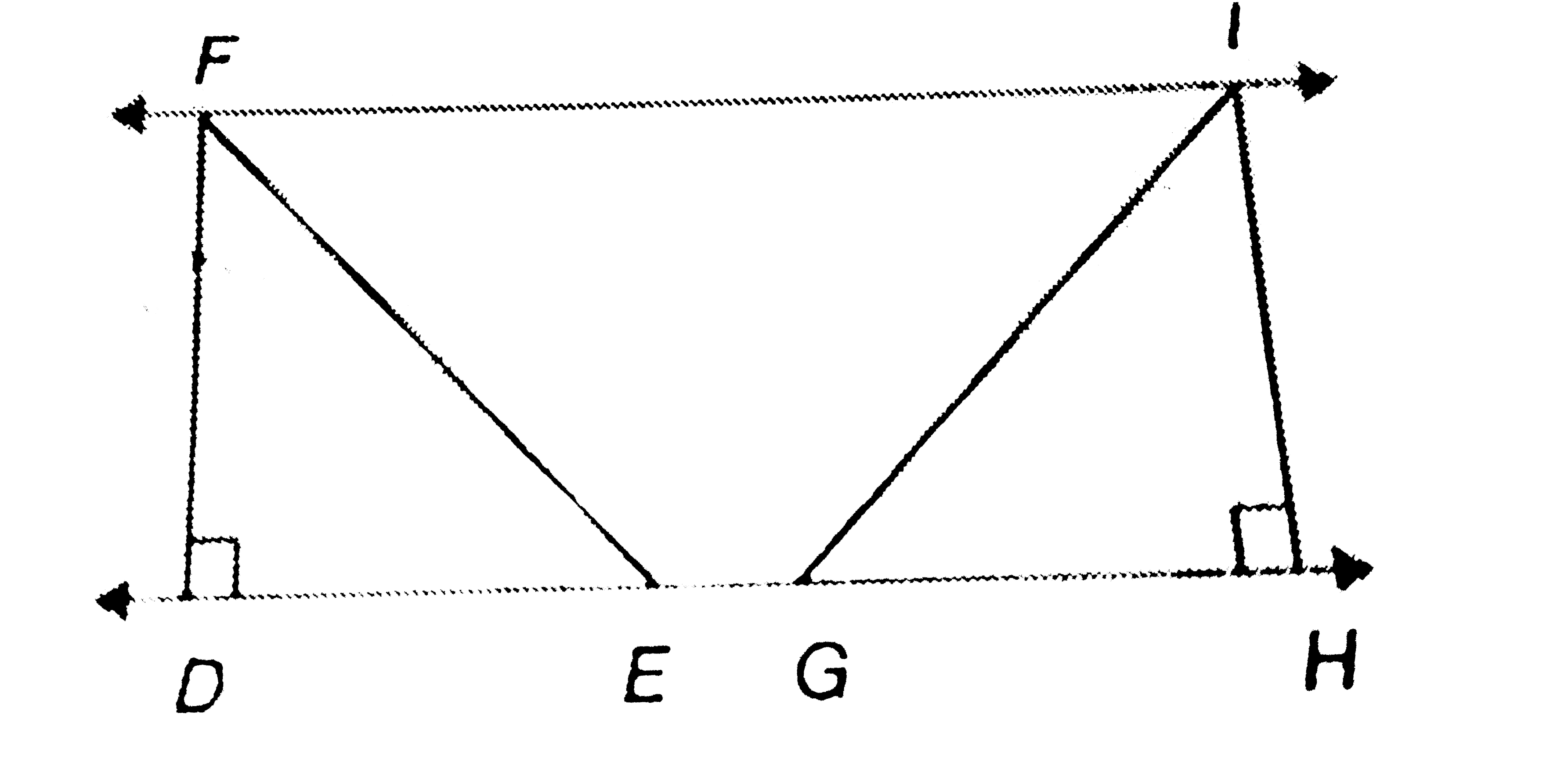
View SolutionA pole of height `14 m` casts a `10 m` long shadow on the ground. At the same time, a tower casts a `70 m` long shadow on the ground. Find the height of the tower.
View SolutionThe angle subtended by a minor arc in its alternate segment is…………….
View SolutionThe number of diagonals of a regular polygon is 27. Then, each of the interior angles of the polygon is…………………
View SolutionABC is a triangle inscribed in a circle, AC being the diameter of the circle. The length of AC is as much more than the length of BC as the length of BC is more than the length of AB. Find AC:AB.
View Solution`frown(MN)` is the arc of the circle with centers O. If `angleMOR = 100^(@)` and `angleNOR=135^(@)`, then `1/2angleORN+1/4angleORM` is………………

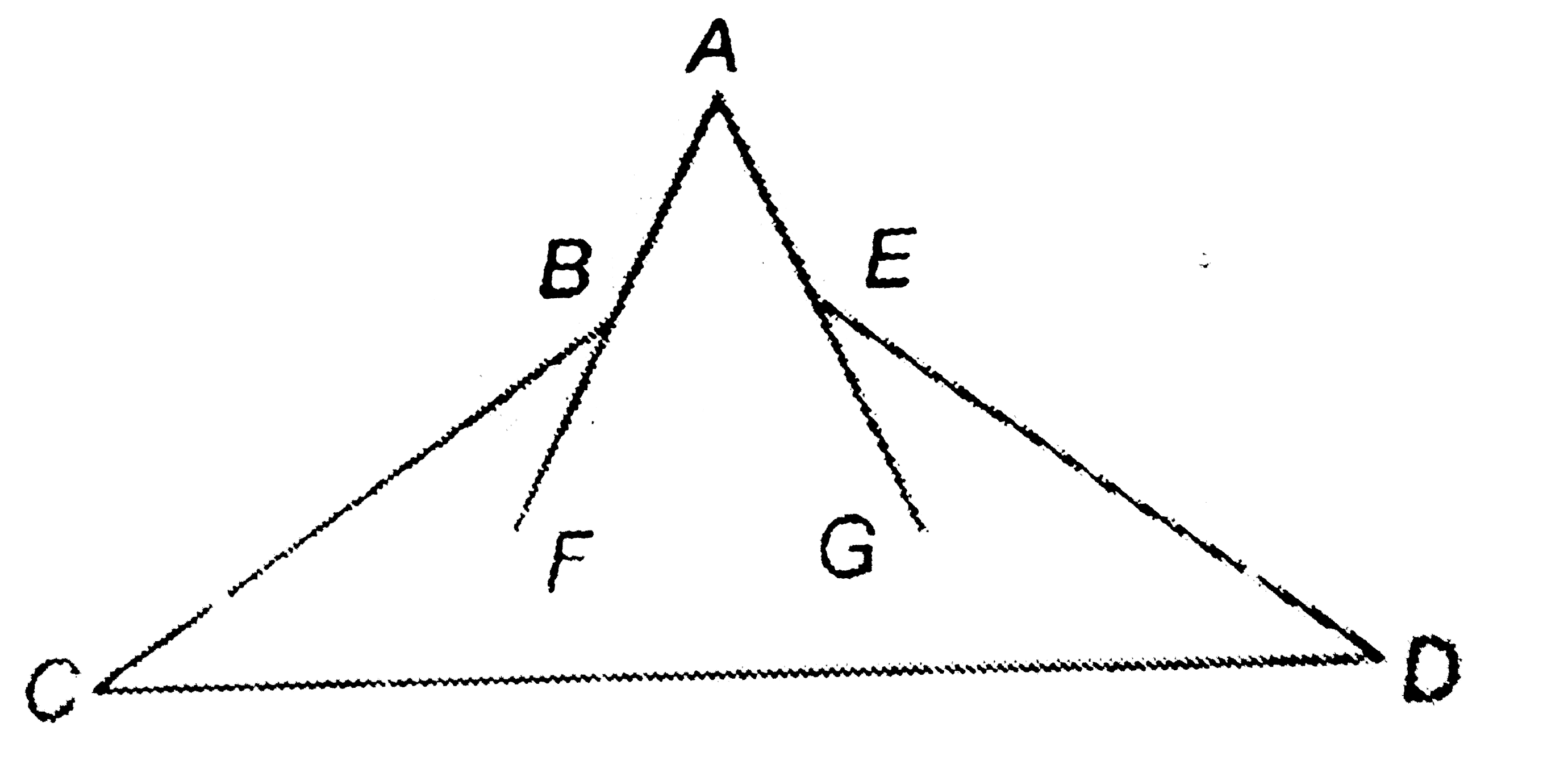
View SolutionIn the following figure (not be scale), `angleBCD=40^(@)`, `angleEDC=35^(@)`, `angleCBF=30^(@)` and `angleDEG=40^(@)`, Find `angleBAE`.
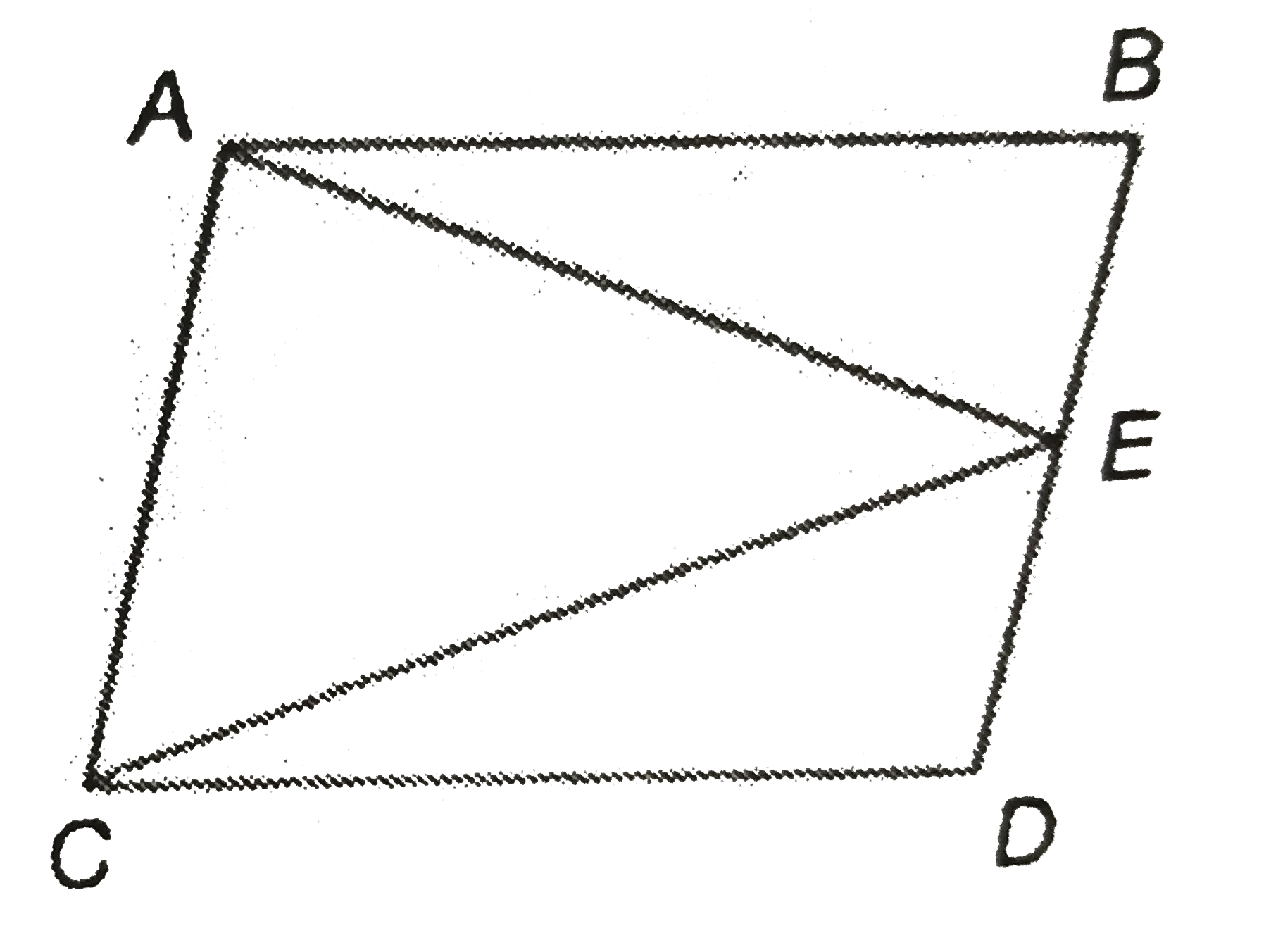
View SolutionIn the following figure (not to scale), `bar(AB) || bar(CD)`. If `angleBAE=25^(@)` and `angleDCE=30^(@)`, then find `angleAEC`.
View SolutionA tower of height 60 m casts a 40 m long shadow on the ground. At the same time, a needle of height 12 m casts a `x` cm long shadow the ground. Find `x`
View SolutionIn the given figure, AC is the diameter, AB and AD are equal chords. If `angle AED=110^(@)`, then find `angleBAD`.

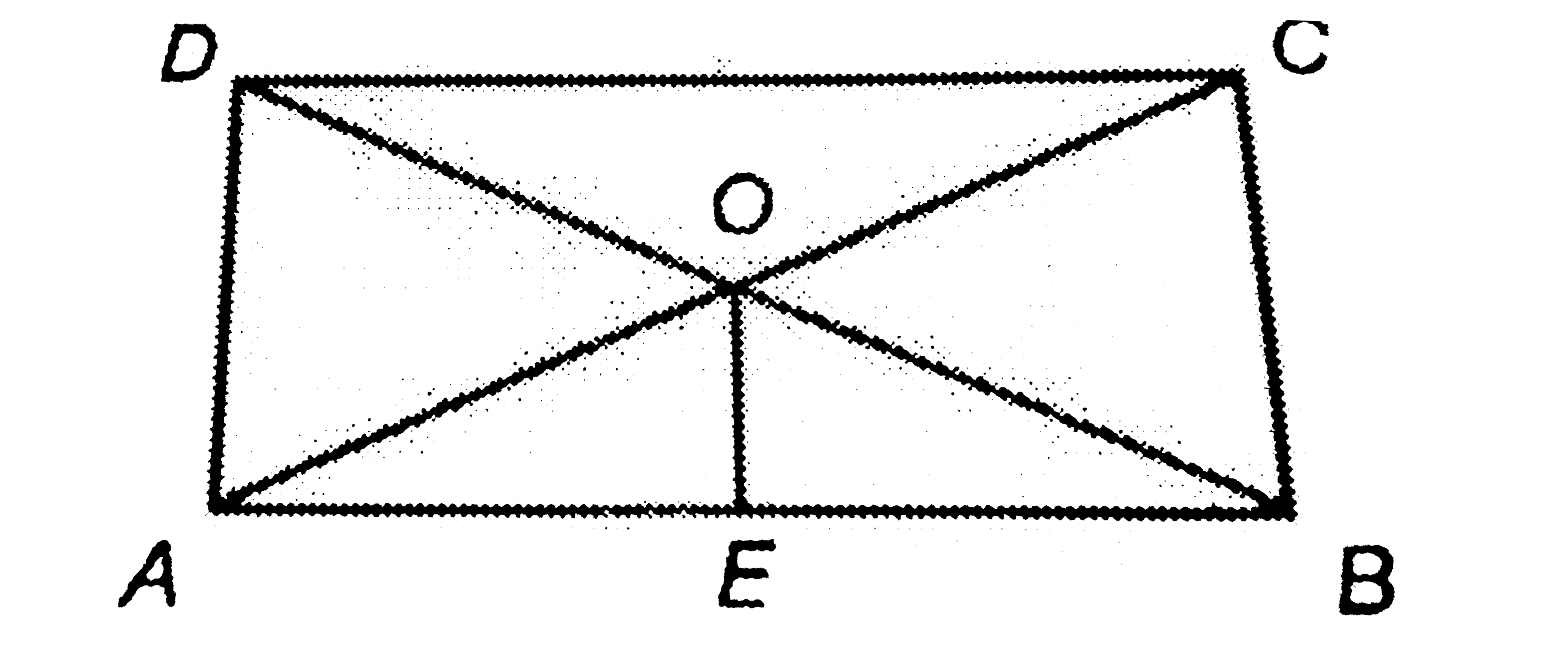
View SolutionIn the given rectangle ABCD, the sum of the lengths of two diagonals is equal to 52 cm and E is a point in AB, such that `bar(OE)` is perpendicular to `bar(AB)`. Find the lengths of the sides of the rectangle, if OE=5 cm.
View SolutionIn the following figure (not to scale), AD bisects `angleBAC`. If `angleBAD=45^(@)` is inscribed in a circle, then which of the following is the longest?
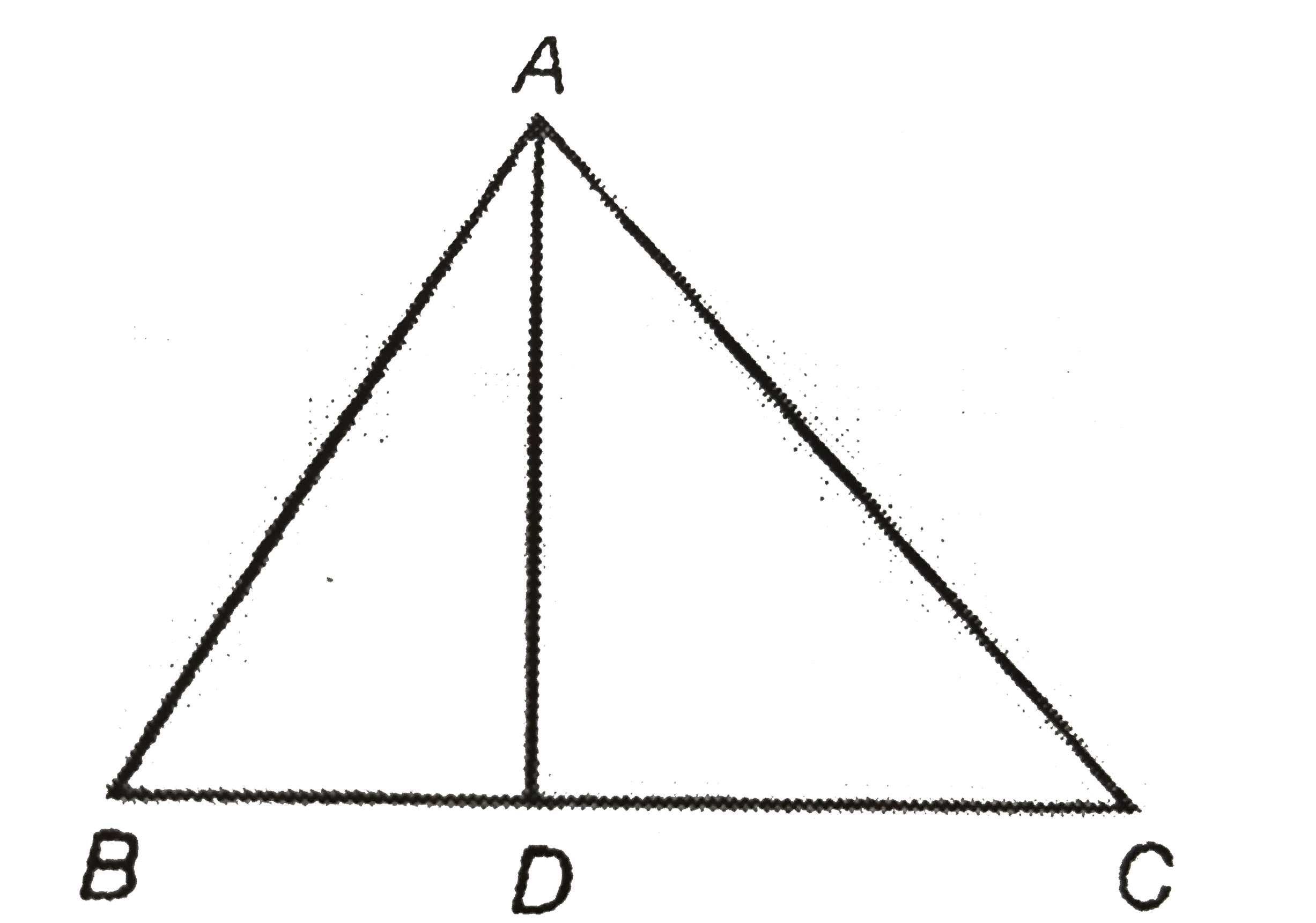
View SolutionIn the given figure, `bar(AB) || bar(DE)` and area of the parallelogram ABFD is 24 `cm^(2)`. Find the areas of `triangleAFB, triangleAGB` and `triangleAEB`.

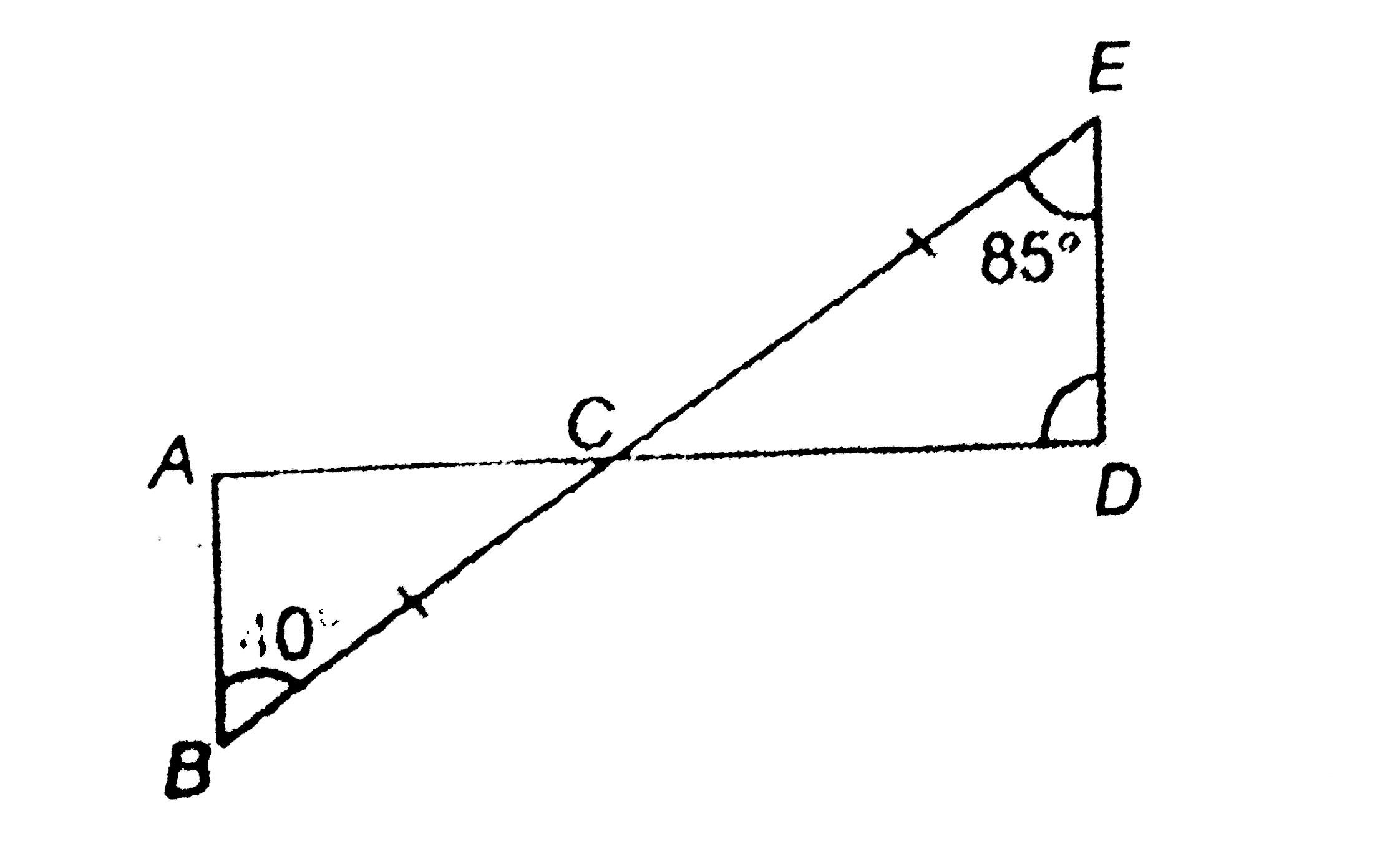
View SolutionIn the given figure, `bar(AD)` and `bar(BE)` intersect at C, such that BC = CE, `angleABC=40^(@)` and `angleDEC=85^(@)`. Find `angleBAC-angleCDE`.
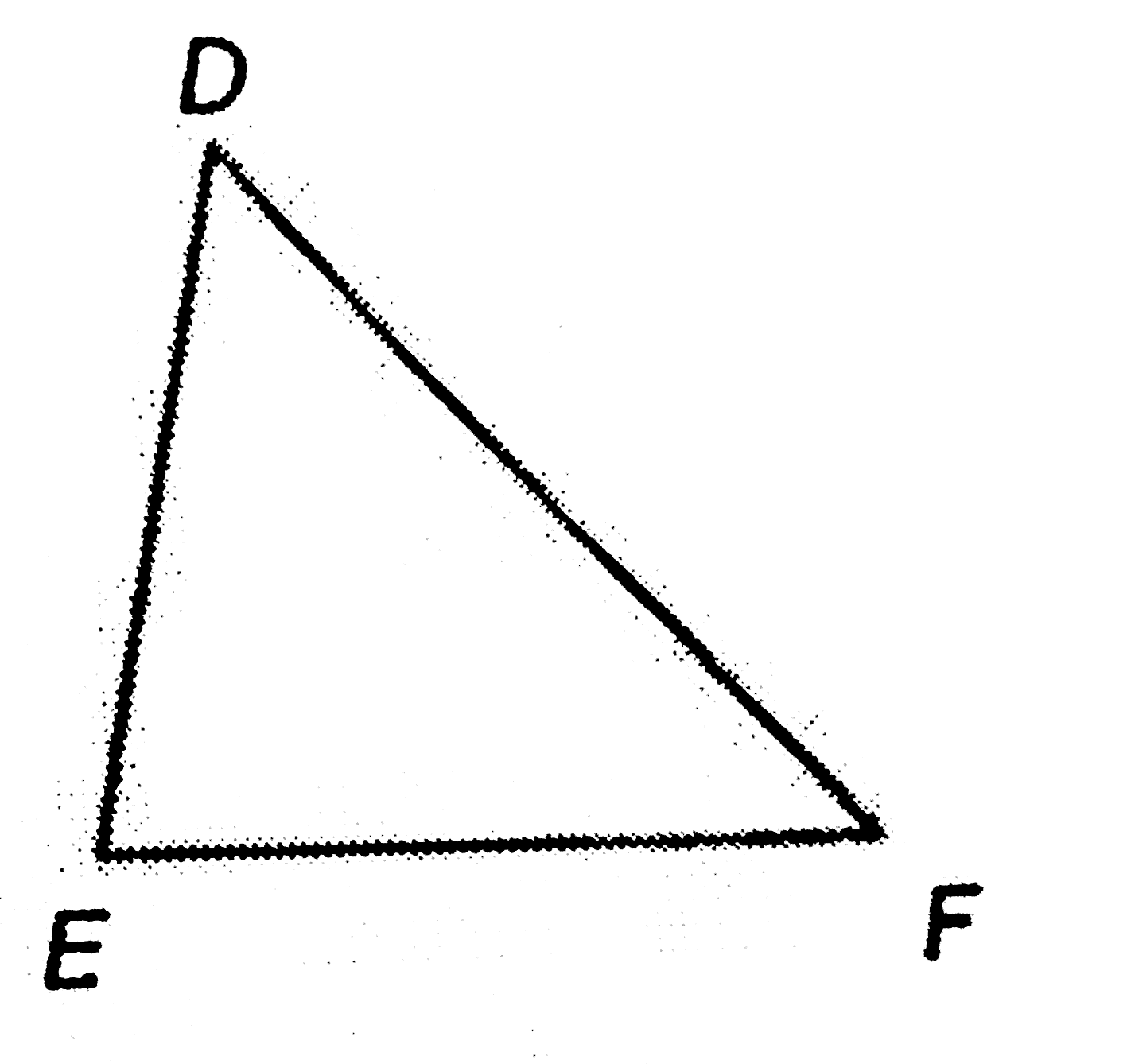
View SolutionIn the given figure, DEF is a triangle. If DF is the longest side and EF is the shortest side, then which of the following is true?
View SolutionThe ratio between the exterior angle and the interior angle of a regular polygon is `1:3`. Find the number of the sides of the polygon.
View SolutionFind each interior and exterior angle of a regular polygon having 30 sides.
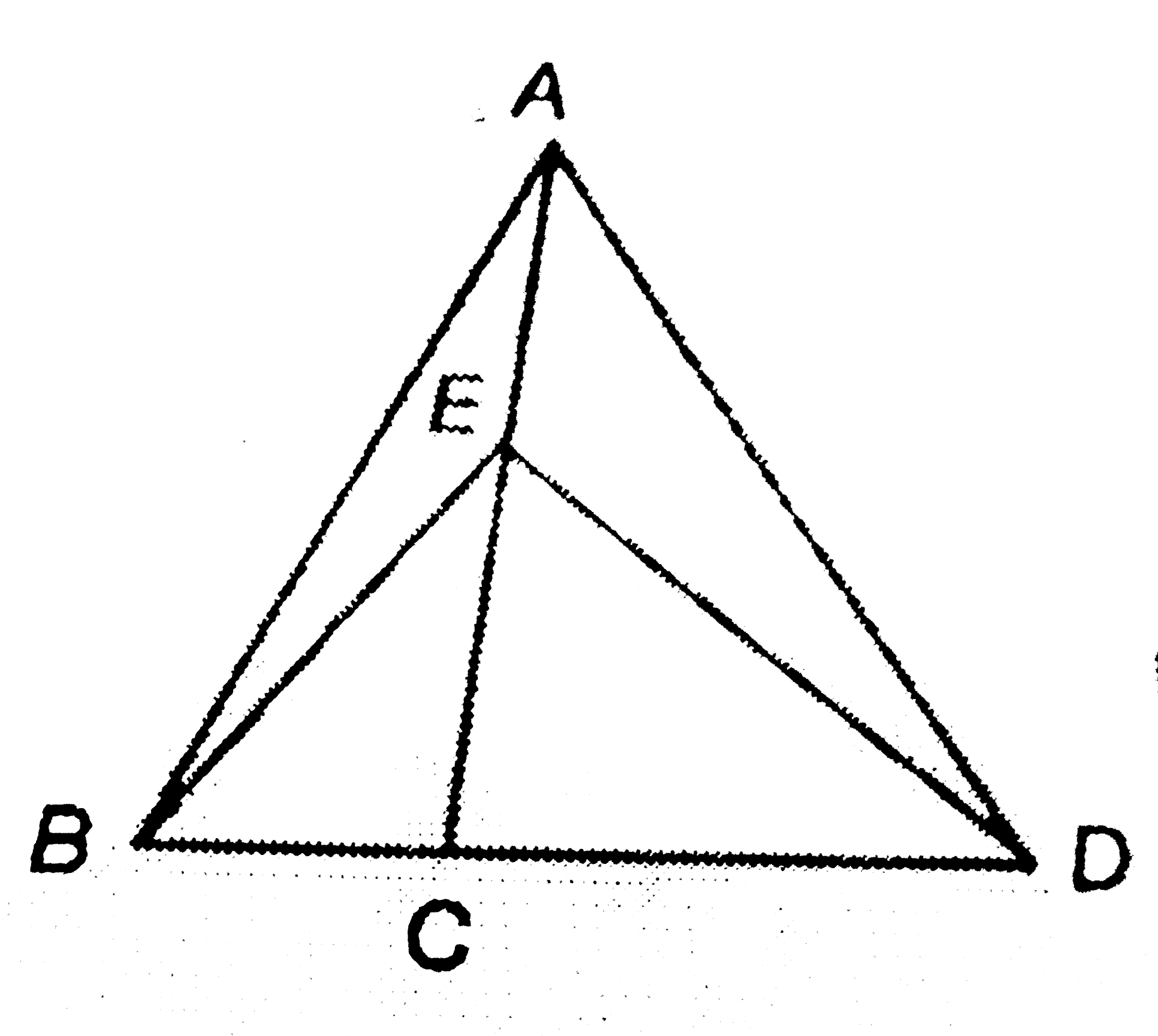
View SolutionIf `BC:CD=2:2`, `AE:EC=2:3`, then find the ratio of the area of the `triangleECD` to the area of `triangleAEB`.
View SolutionIn the given figure (not to scale), O is the center of the circle. If PB=PC, `anglePBO-25^(@)` and `angleBOC-130^(@)`, then find `angleABP+angleDCP`.
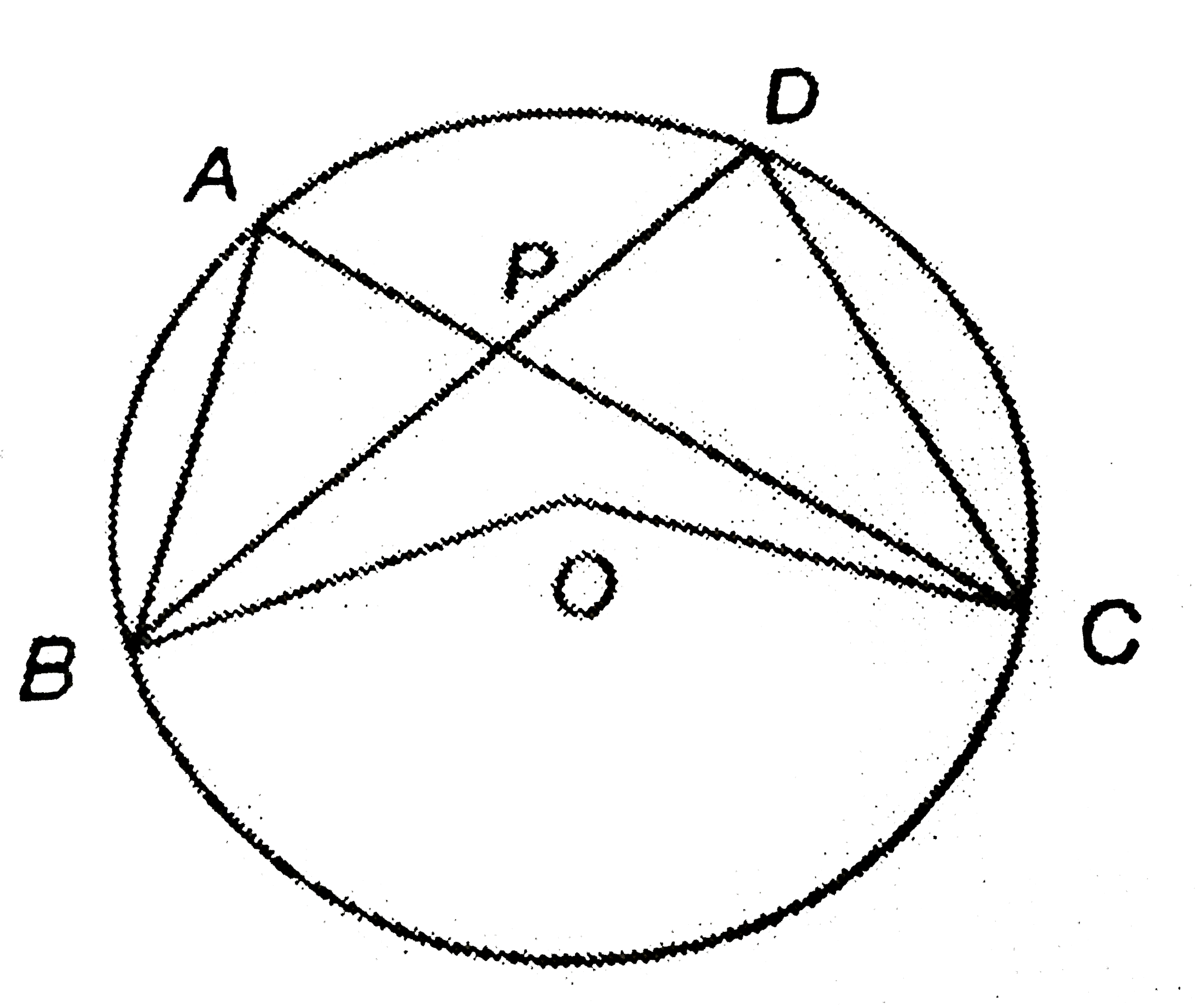
View SolutionIn a polygon, the greatest angle is `110^(@)` and all the angles are distinct in integral measures (in degrees). Find the maximum number of sides it can have.
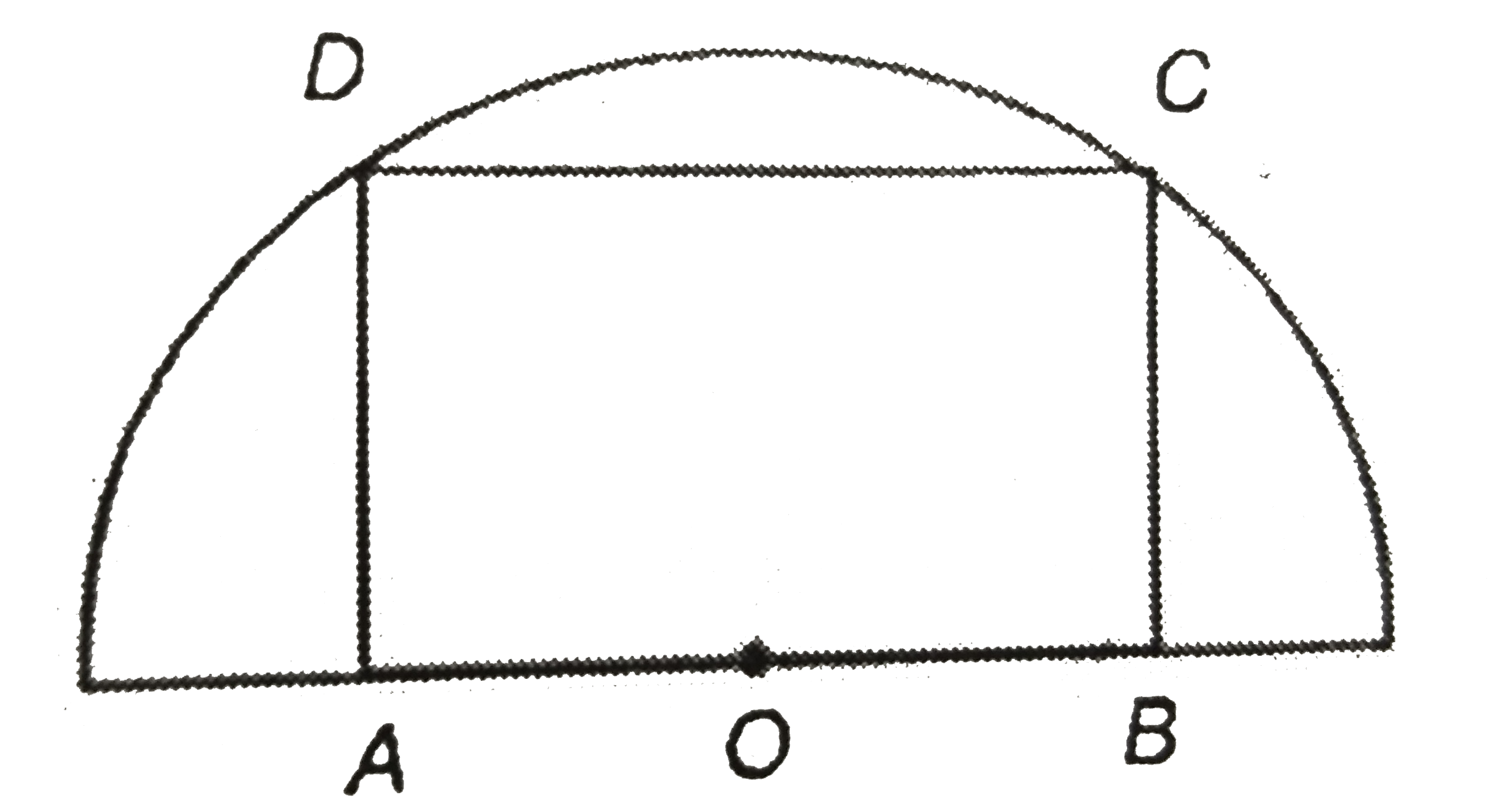
View SolutionIn the given figure, ABCD is a rectangle inscribed in a semi-circle. If the length and the bredth of the rectangle are in the ratio `2:1`. What is the ratio of the perimeter of the rectangle to the diameter of the semicircle?
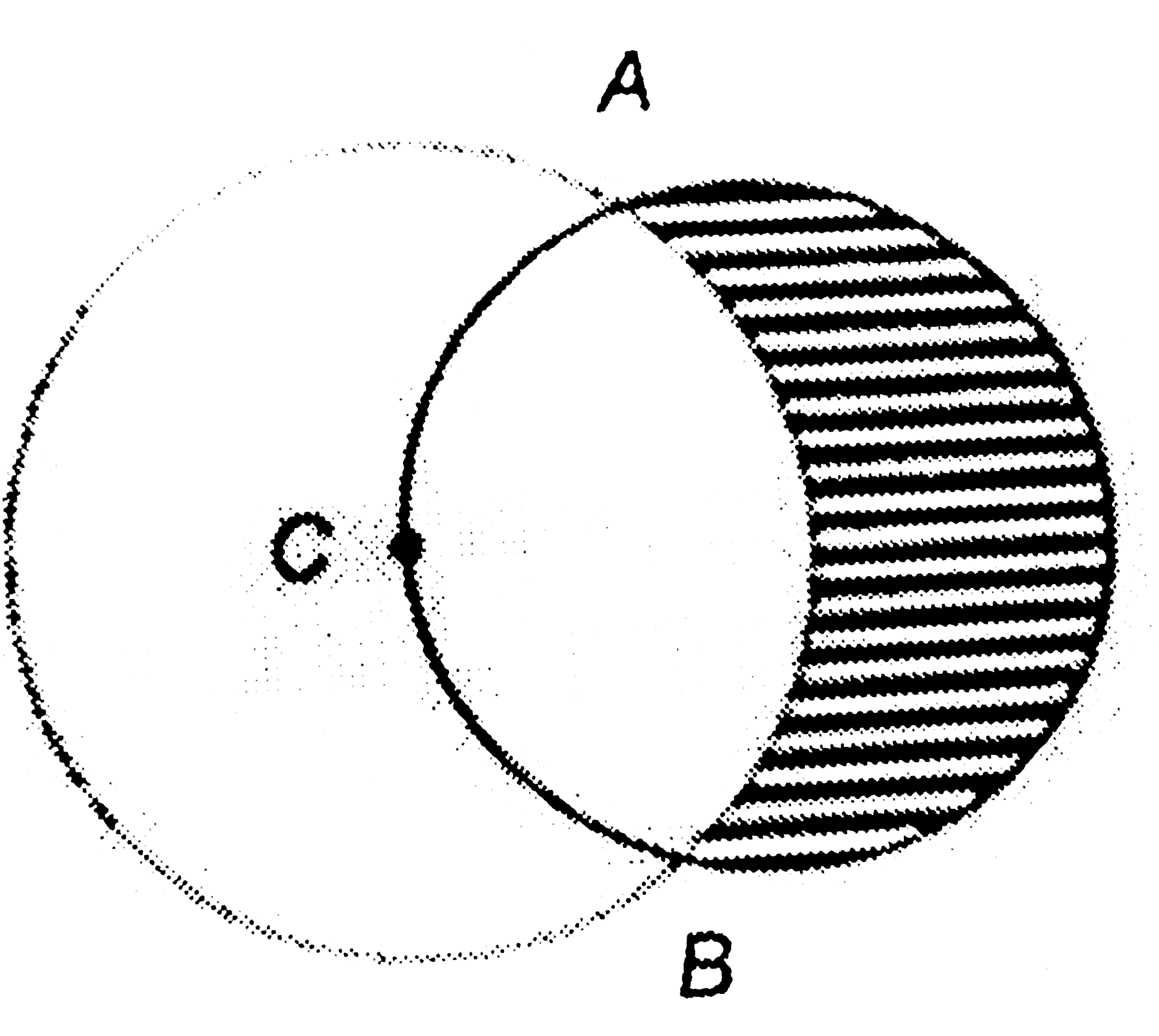
View SolutionIn the given figure, `bar(AB)` is the diameter of the circle with area `pi` sq. units. Another circle is drawn with C as center, which is on the given circle and passing through A and B. Find the area of the shaded region.
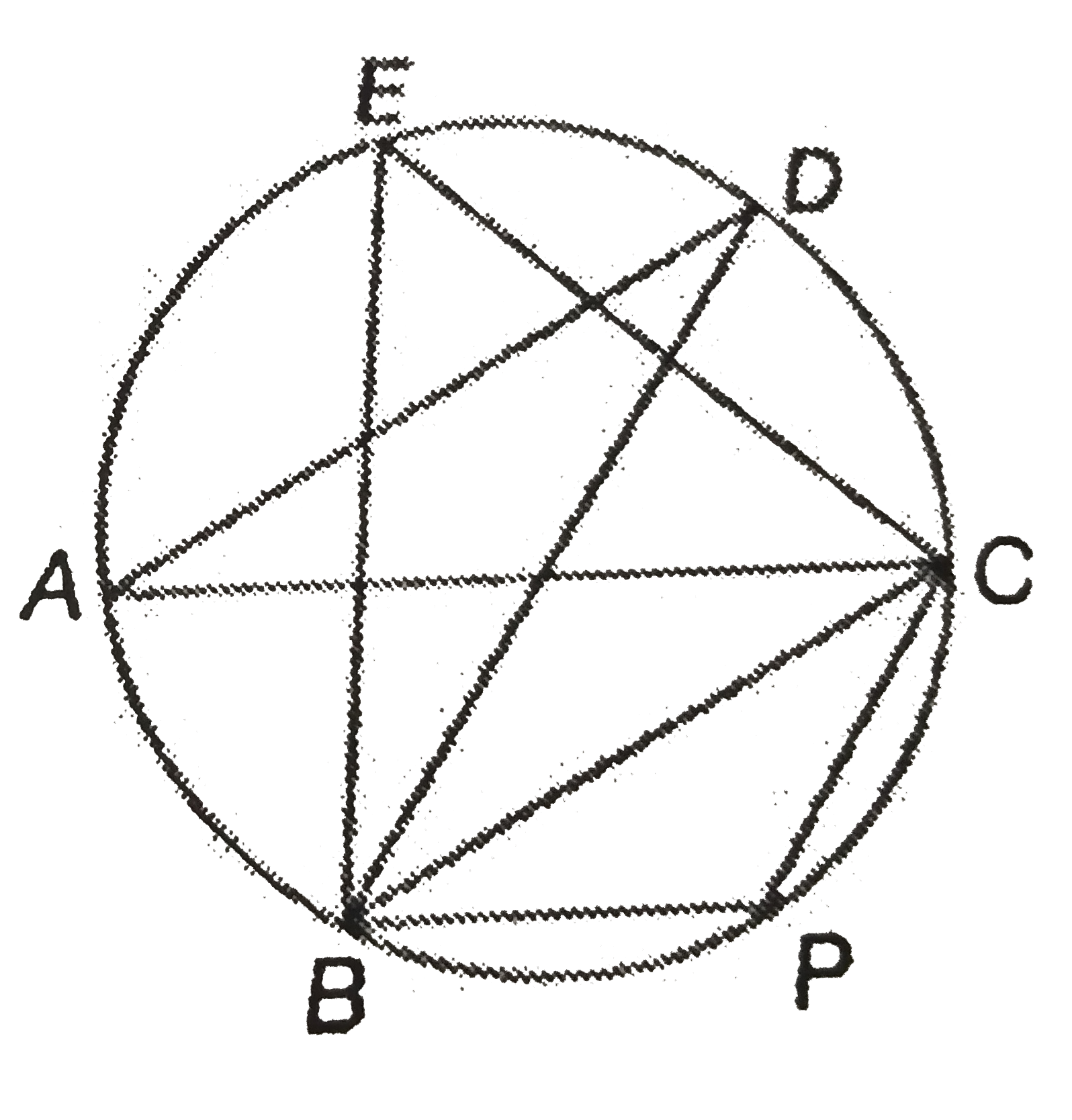
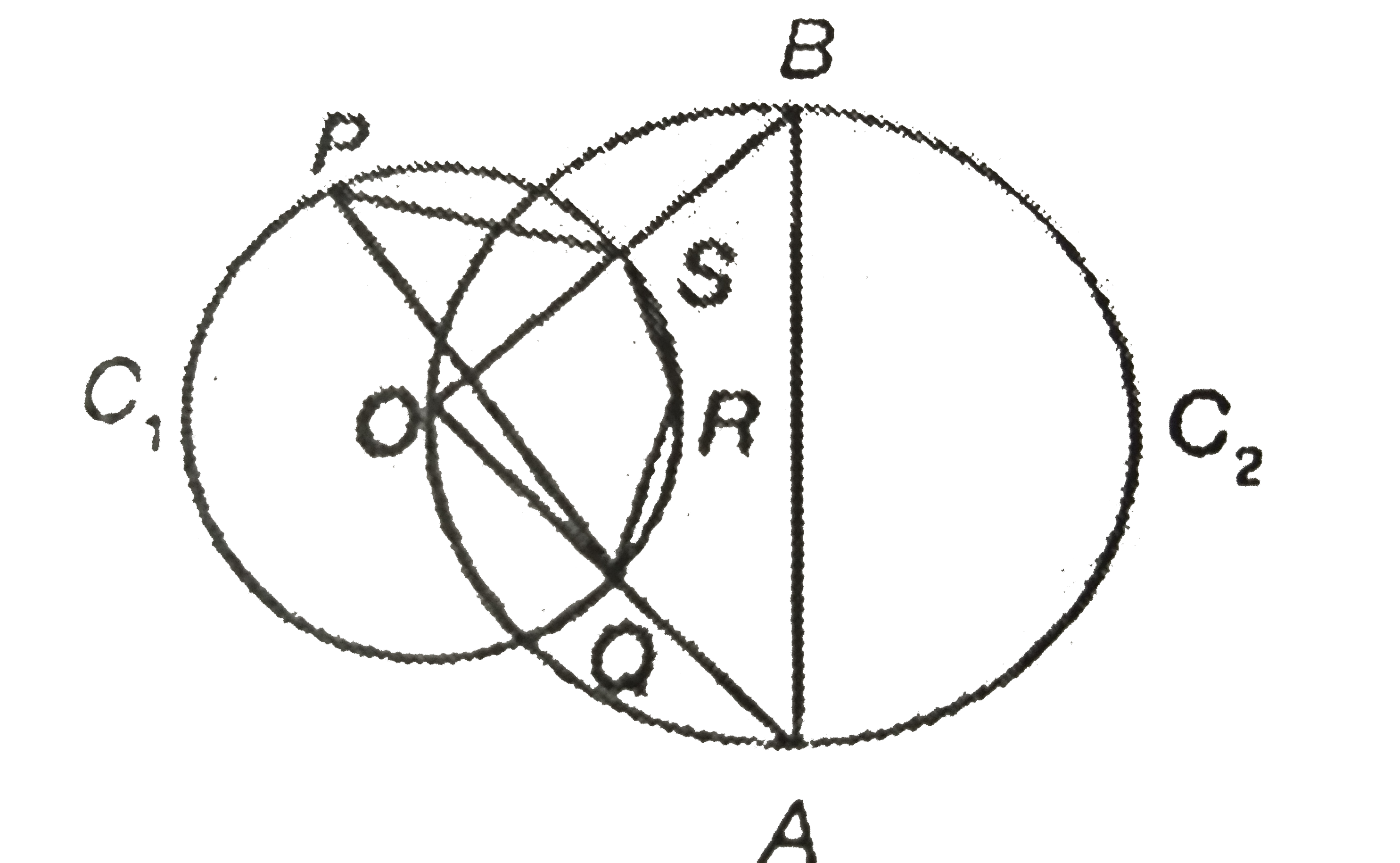
View SolutionIn the following figure (not to scale), `C_(1)` and `C_(2)` are two congruent circles with centers `O_(1)` and `O_(2)`. Respectively. Each circle passes through the center of the other circle. If the circumference of each circle is 2cm, the perimeter of the shaded region is................ cm.

View SolutionIn the given figure, (not to scale), the points M,R, N,S and Q are concylic. Find `anglePQR+angleOPR + angleNMS + angleOSN`, if O is the center of the circle.

View SolutionIn the given figure (not to scale), AC is the diameter of the circle and `angleADB=20^(@)`, then find `angleBPC`.
View SolutionIn the following figure, O is the center of the circle and CD=EF=GF. If `angleCOD=40^(@)`, then find reflex `angleCOG`.
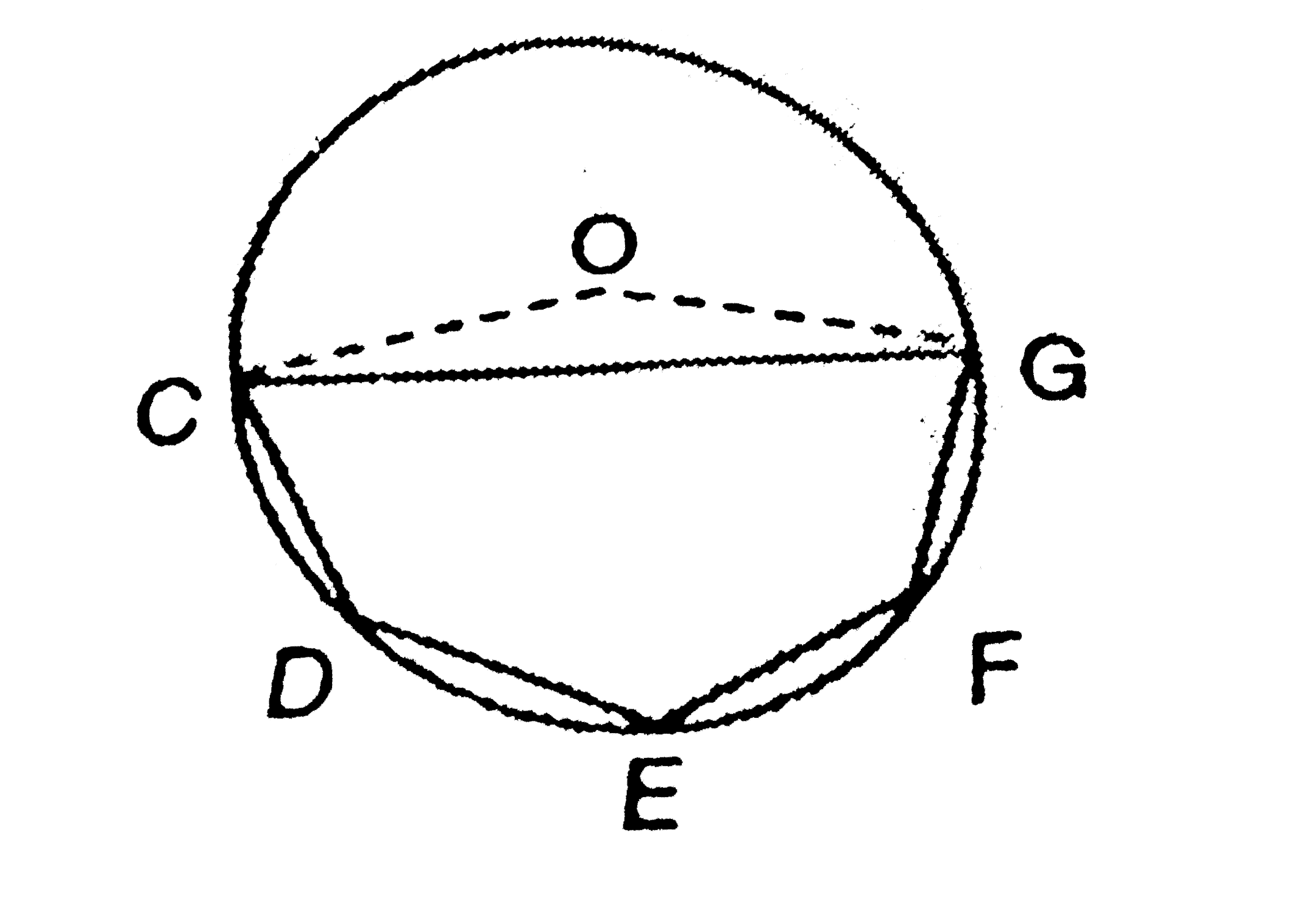
View SolutionIn the given figure (not to scale), AC is the median as well as altitude to BD. In `triangleACE`, AD is the median to CE. Which of the following is true?
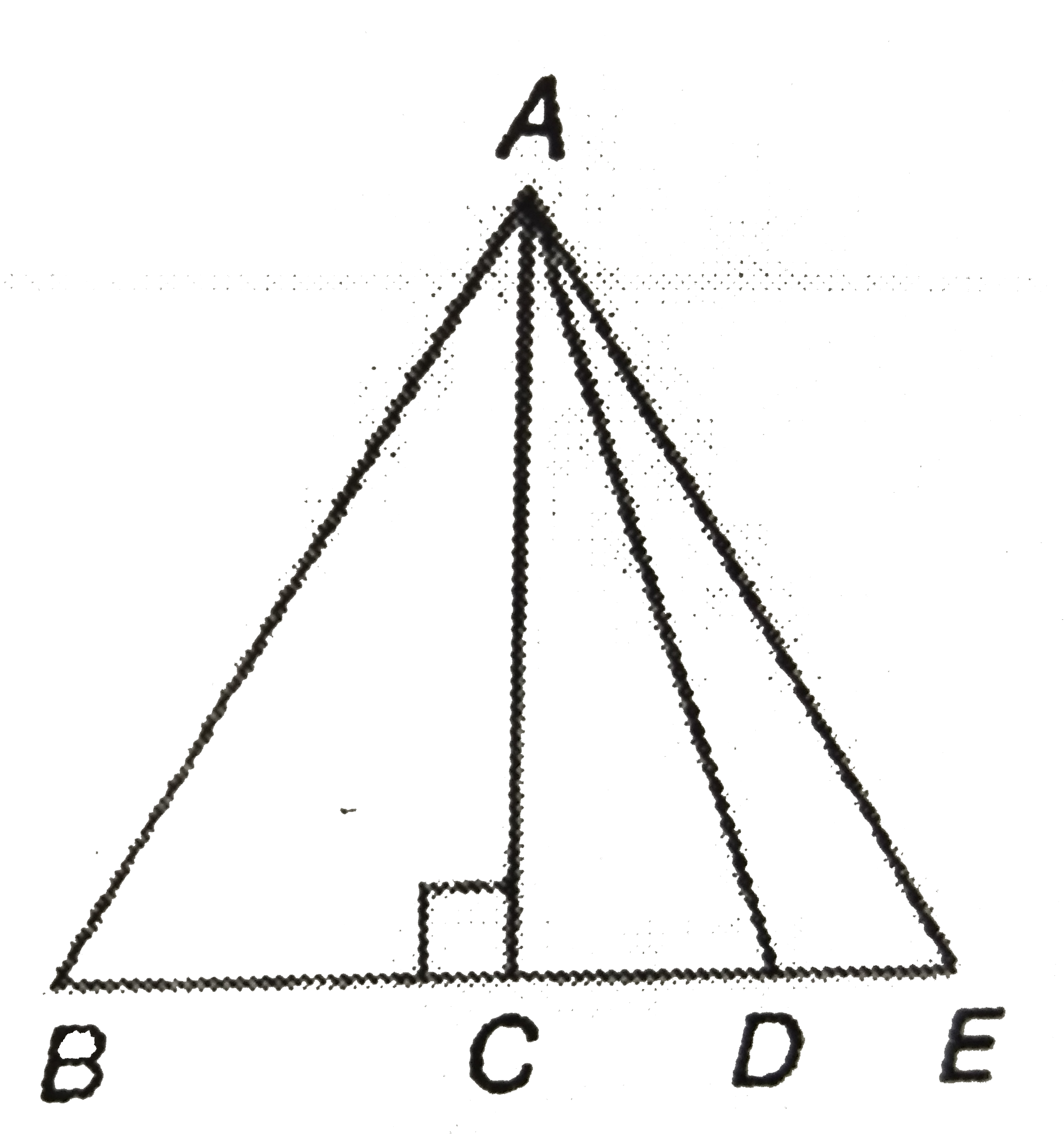
View SolutionIn the given figure, (not to scale), rectangle ABCD and triangle ABE are inscribed in the circle O. If `angleAEB=40^(@)`,then find `angleBOC`.
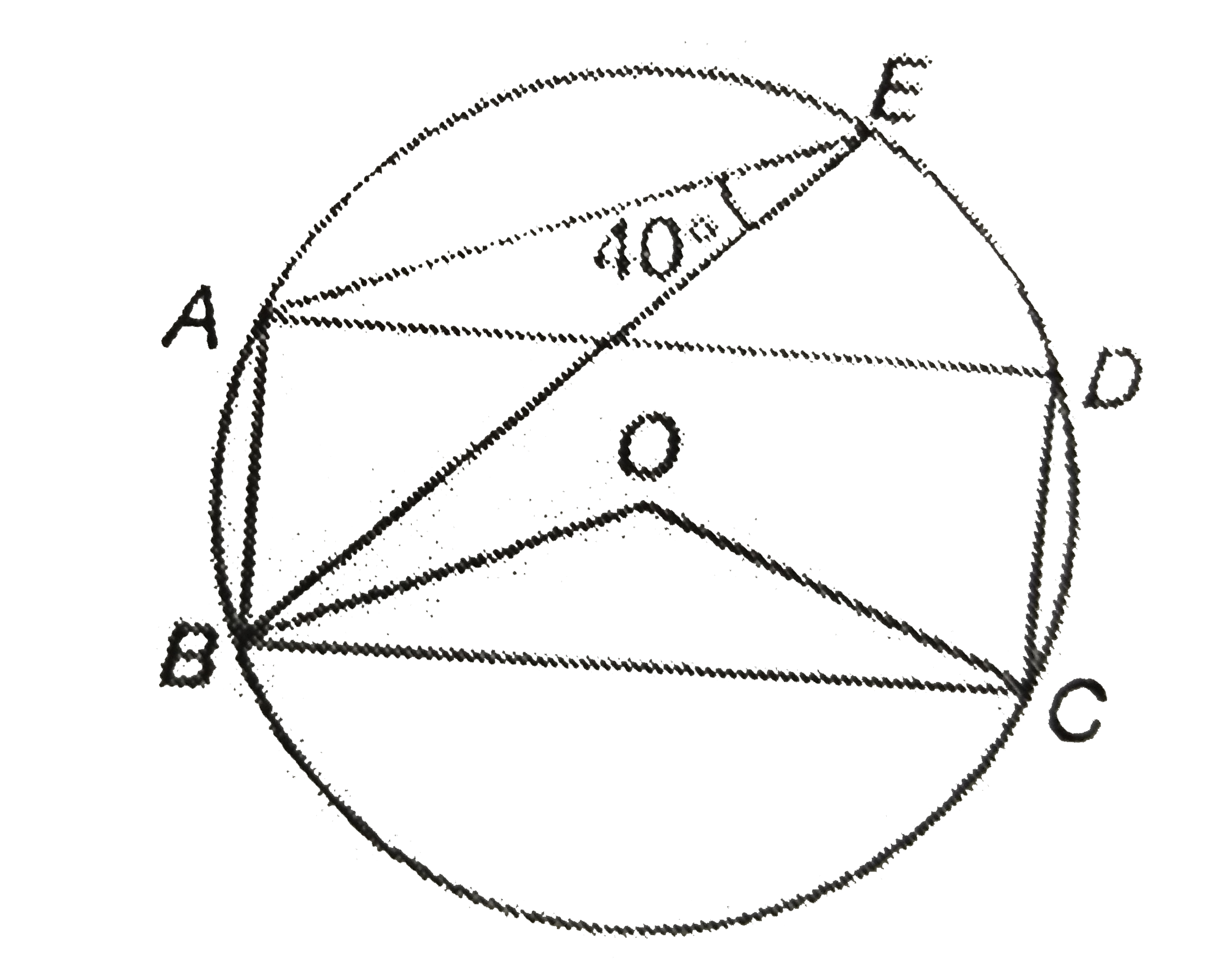
View SolutionIn the given figure (not to scale), O is the center of the circle, BC and CD are equal chords. If `angleOBC=55^(@)`,then find `angleBAC`.
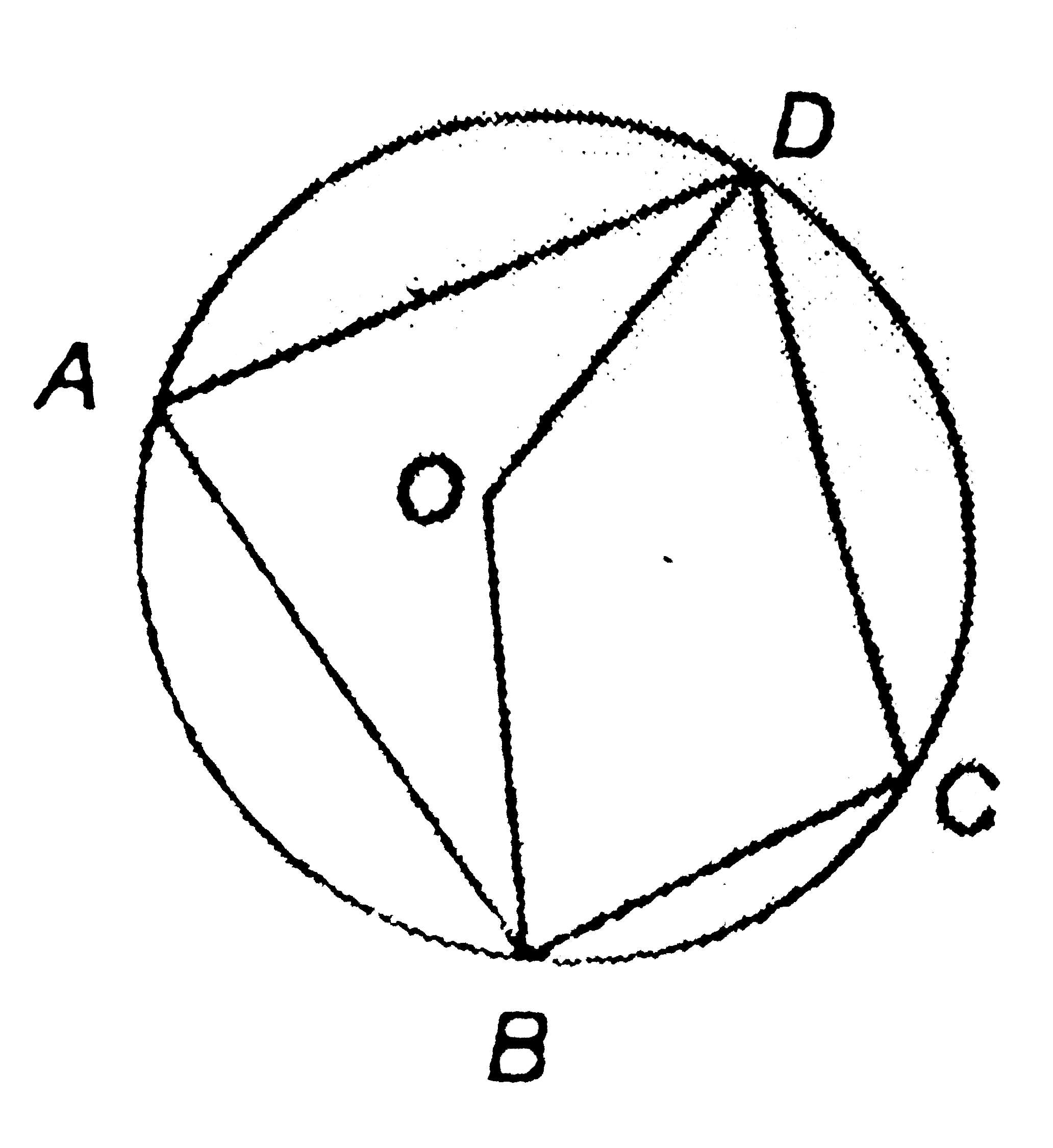
View SolutionIn the given figure (not to scale), O is the center of the circle `C_(1)` and AB is the diameter of the circle `C_(2)`. Quadrilateral PQRS is inscribed in the circle with center O. Find `angleQRS`.
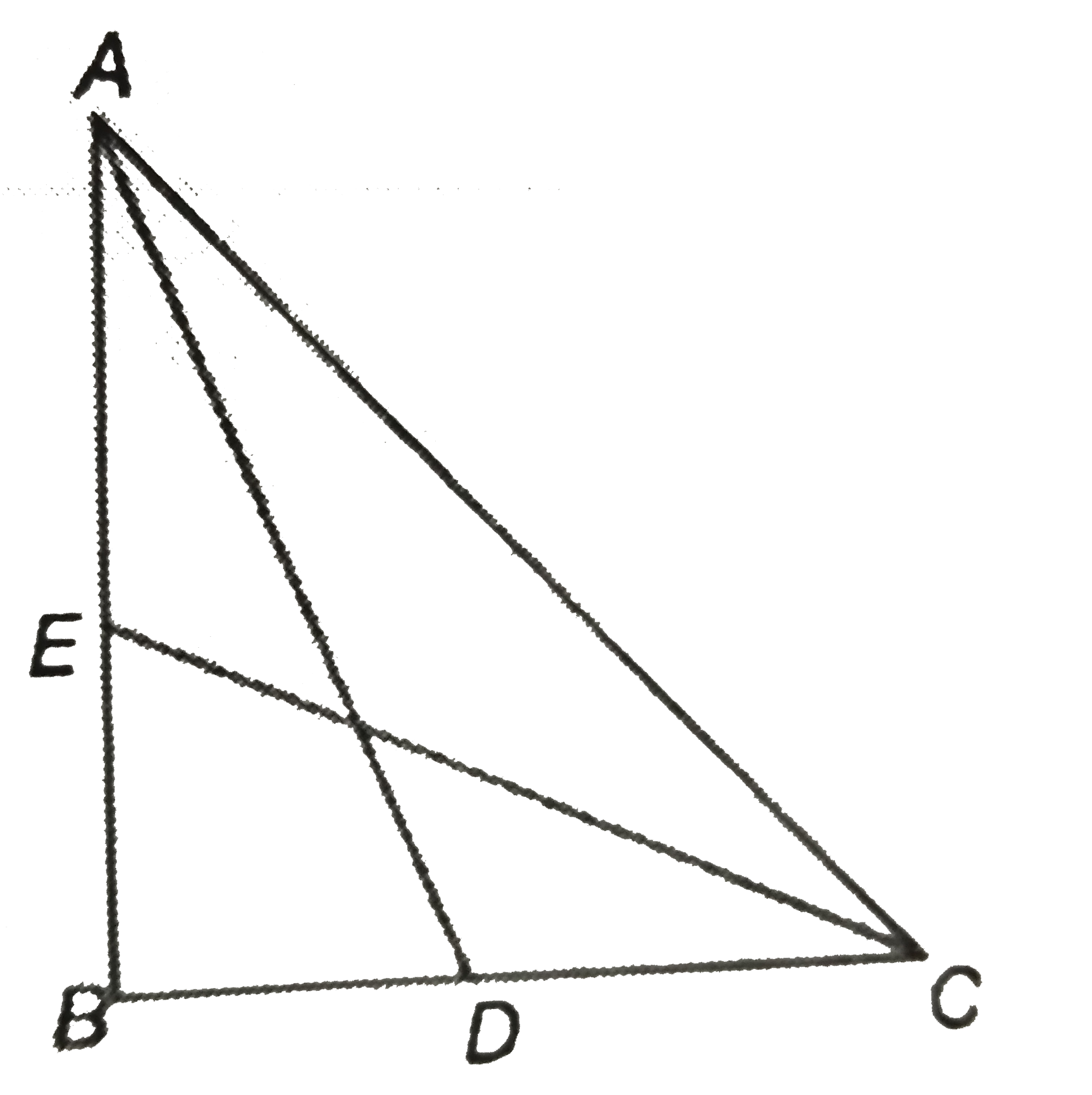
View SolutionIn the given figure, (not be scale), E and D are the mid-points of AB and BC respectively. Also, `angleB=90^(@), AD=sqrt(292)` cm and `CM=sqrt(208)` cm. Find the `AC`.
View SolutionIn `triangleABC`, P is the mid-point of BC and Q is the mid-point of AP. Find the ratio of the area of `triangleABQ` and the area of `angleABC`. The following are the steps involved in solving the above problem.
A) We know that a a median of a triangle divides a triangle into two triangles of equal area.
B) `rArr Ar(triangleABP) =1/2[Ar(triangleABC)]`
C) `Ar(triangleABQ)=1/2[Ar(triangleABP)]=1/4[Ar(triangleABC)]`
D) `rArr(triangleABQ):Ar(triangleABC)=1:4`
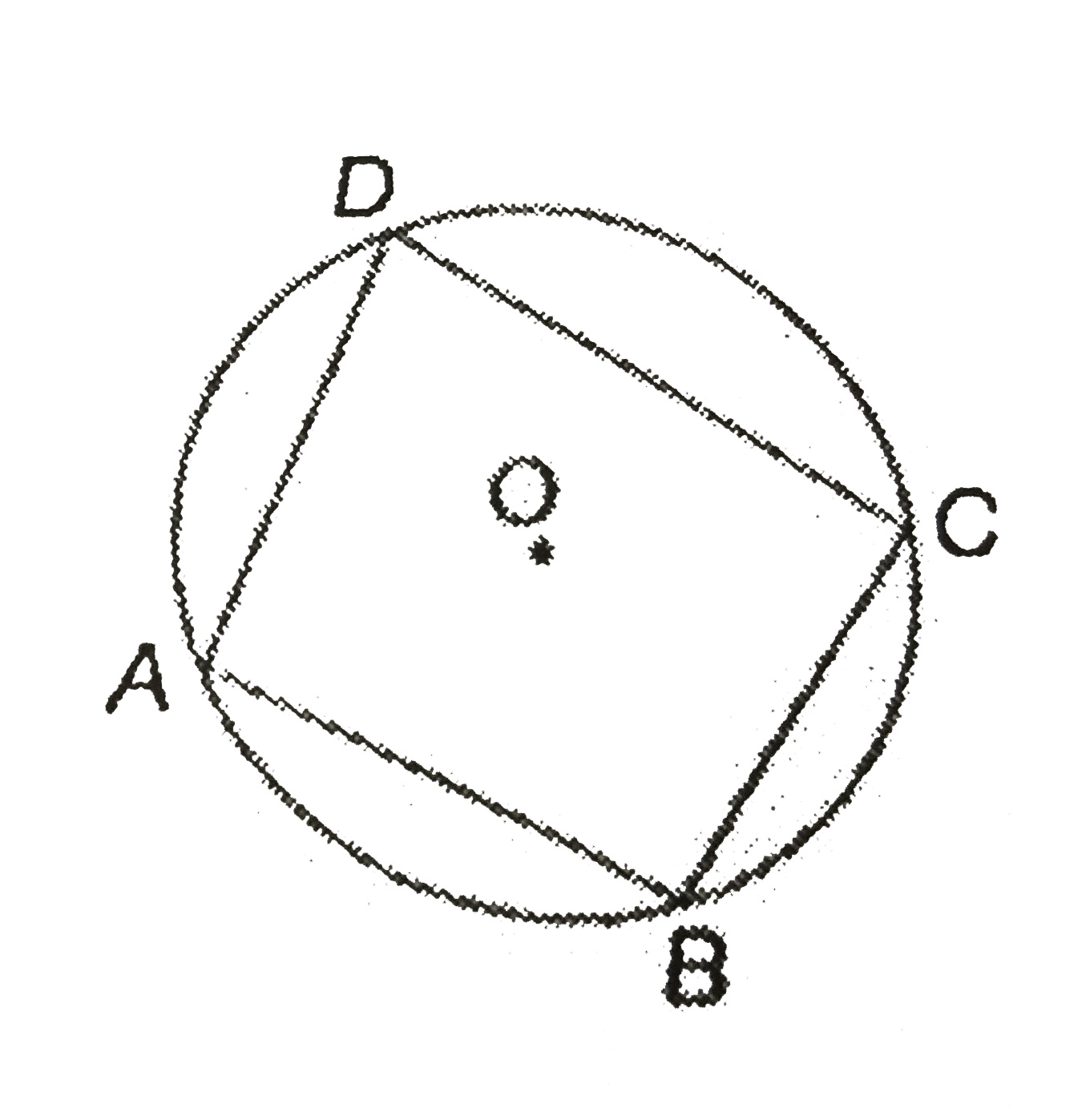
View SolutionABCD is a cyclic quadrilateral, ABC is a minor arc and O is the center of the circle. If `angleAOC=160^(@)`, then find `angleABC`.
The following are the steps involved in solving the above problem. Arrange them in sequential order:
A) We have, `angleABC+angleADC=180^(@)`
B) `angleABC +1/2angleAOC=180^(@)( therefore angleADC=1/2 angleAOC)`
C) `angleABC=180^(@)-80^(@)`
D) `angleABC+160^(@)/2=180^(@)`
E) `therefore angleABC=100^(@)`.
View SolutionShow that each diagonal of a parallelogram divide it into two congruent triangles.
The following are the steps involved in showing the above result. Arrange them in sequential order.
A) In `triangleABC` and `triangleCDA`, AB=DC and BC=AD `(therefore` opposite angles of parallelogram) AC=AC (common side).
B) Let ABCD be a parallelogram. Join AC.
C) By SSS congruence property, `triangleABC ~=triangleCDA`.
D) Similarly, BD divides the triangle into two congruent triangles.
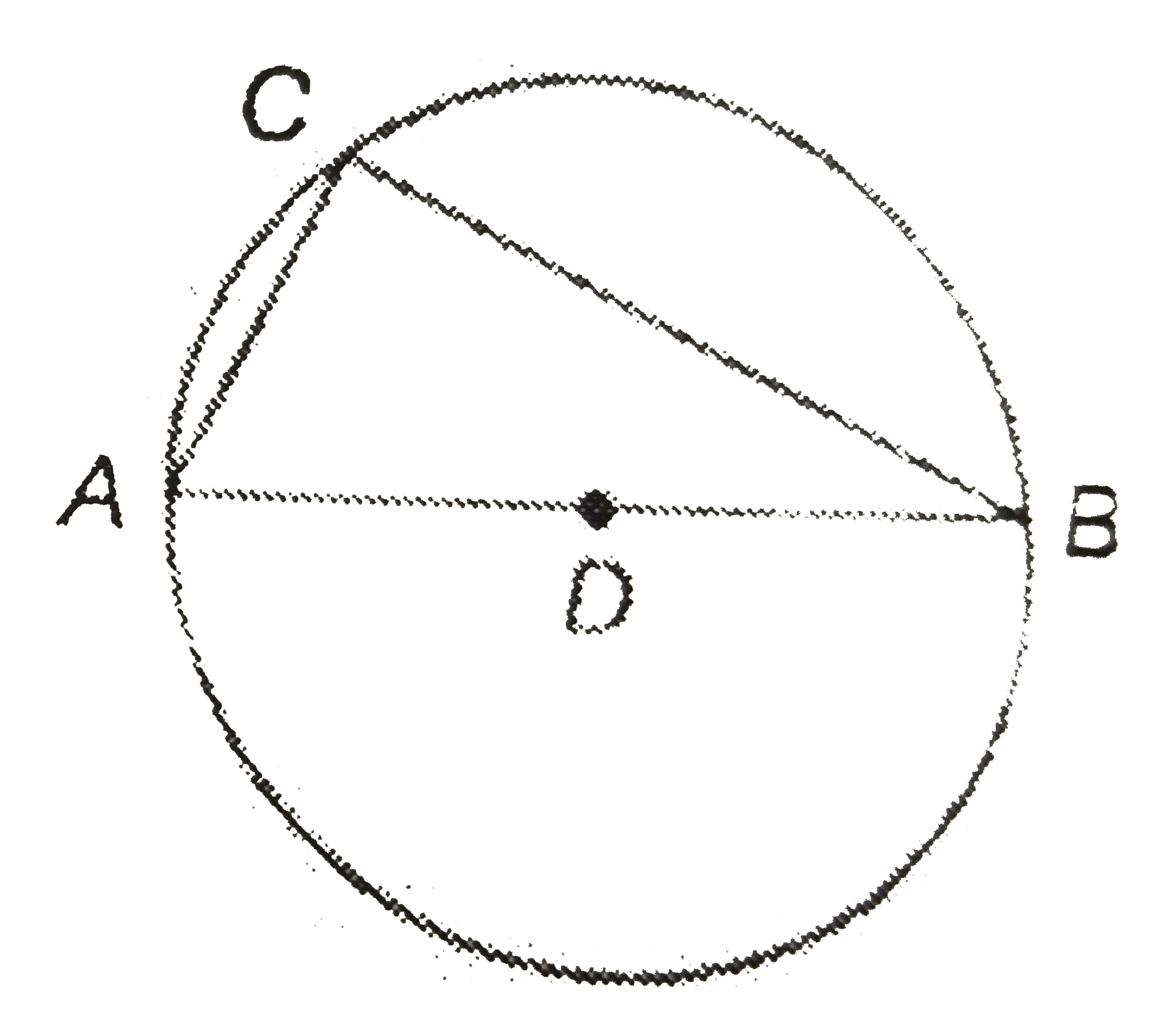
View SolutionShow that any angle in a semi-circle is a right angle.
The following arc the steps involved in showing the above result. Arrange them in sequential order.
A) `therefore angleACB=180^(@)/2=90^(@)`
B) The angle subtended by an arc at the center is double of the angle subtended by the same arc at any point on the remaining part of the circle.
c) Let AB be a diameter of a circle with center D and C be any point on the circle. Join AC and BC.
D) `therefore angleAD = 2 xx angleACB`
`180^(@)=2angleACB(therefore angleADB=180^(@))`
View SolutionA,B,C and D are concylic, AC bisects BD. If AB =9 cm, BC=8 cm, and CD=6 cm, then find the measure of AD.
View SolutionIn the given figure, PQRS is a parallelogram. A and B are the mid-point of `bar(PQ)` and `bar(SR)` respectively. If PS=BR, then the quadrilateral ADBC is a…………………… ,
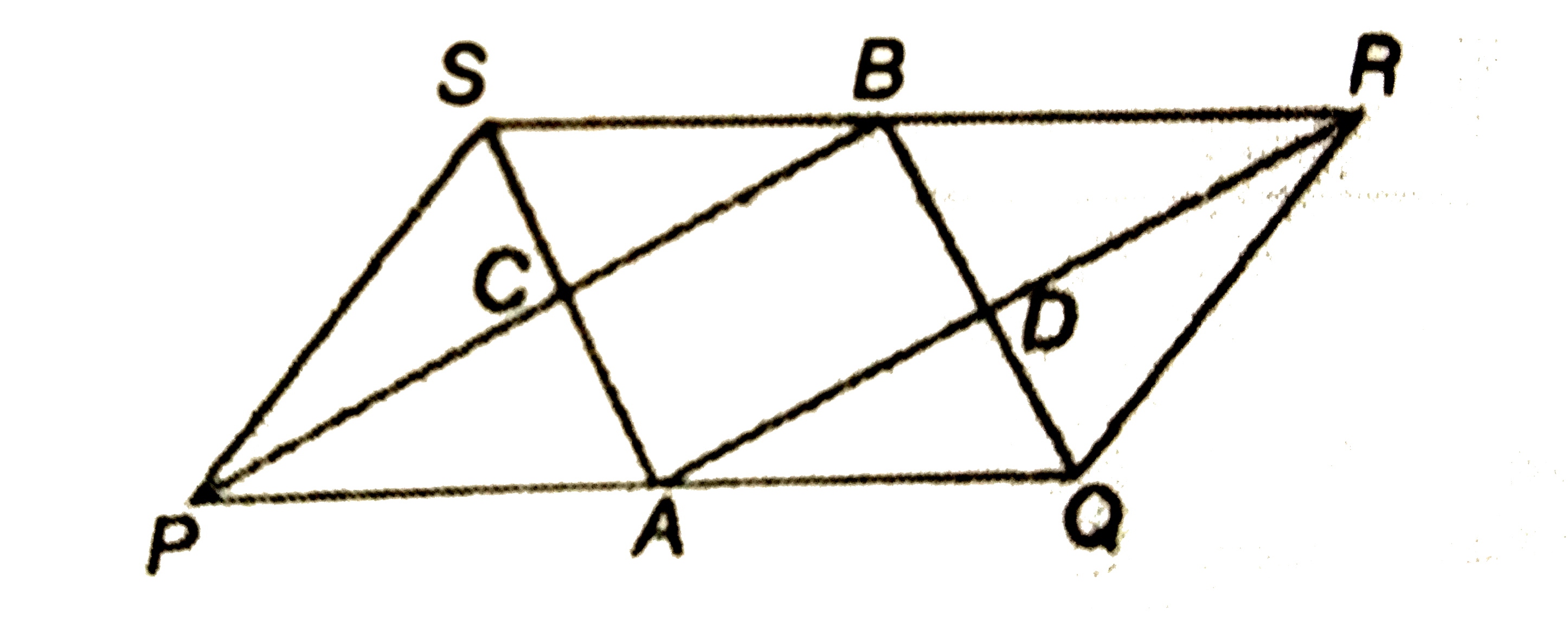
View SolutionThe sides of a triangle are 2006 cm, 6002 cm and m cm, where m is a positive integer. Find the number of such possible triangles.
View SolutionIf a,b and c are the lengths of the sides of a right triangle ABC with `c=2a` and `b^(2)-3a^(2)=0`, then `angleABC=……………………`
View SolutionIn `triangleABC, AC=BC`, S is the circum-center and `angleASB=150^(@)`. Find `angleCAB`.

View SolutionIn the given figure, `P,Q,R` and `S` are concylic points, and `O` is the mid-point of the diameter `QS`. If `angleQPR=25^(@)`, then find `angleSQR`.

View SolutionIn `triangleABC, angleB=90^(@)`, P,Q and R are the mid-points of `bar(AB), bar(BC)` and `bar(AC)` respectively. Then which of the following is true?
View SolutionIf `p, q` and `r` are the lengths of the sides of a right triangle, `PQR`, and the hypotenuse `r=sqrt(2pq)`, then `angleQPR`=……………………
View SolutionIn a triangle PQR, PQ=QR, A and B are the mid-points of `bar(QR)` and `bar(PR)` respectively. A circle passes through P,Q, A and B. Then which of the following is necessarily true?
View SolutionIn the figure given below (not to scale), D is a point on the circle with center A and C is a point on the circle with centers B, `bar(AD) bot bar(BD)` and `bar(BC) bot bar(CA)`. Then which of the following is true?

View SolutionIn the given figure, the angles `angleADE` and `angleABC` differ by `15^(@)`. Find `angleCAE`.
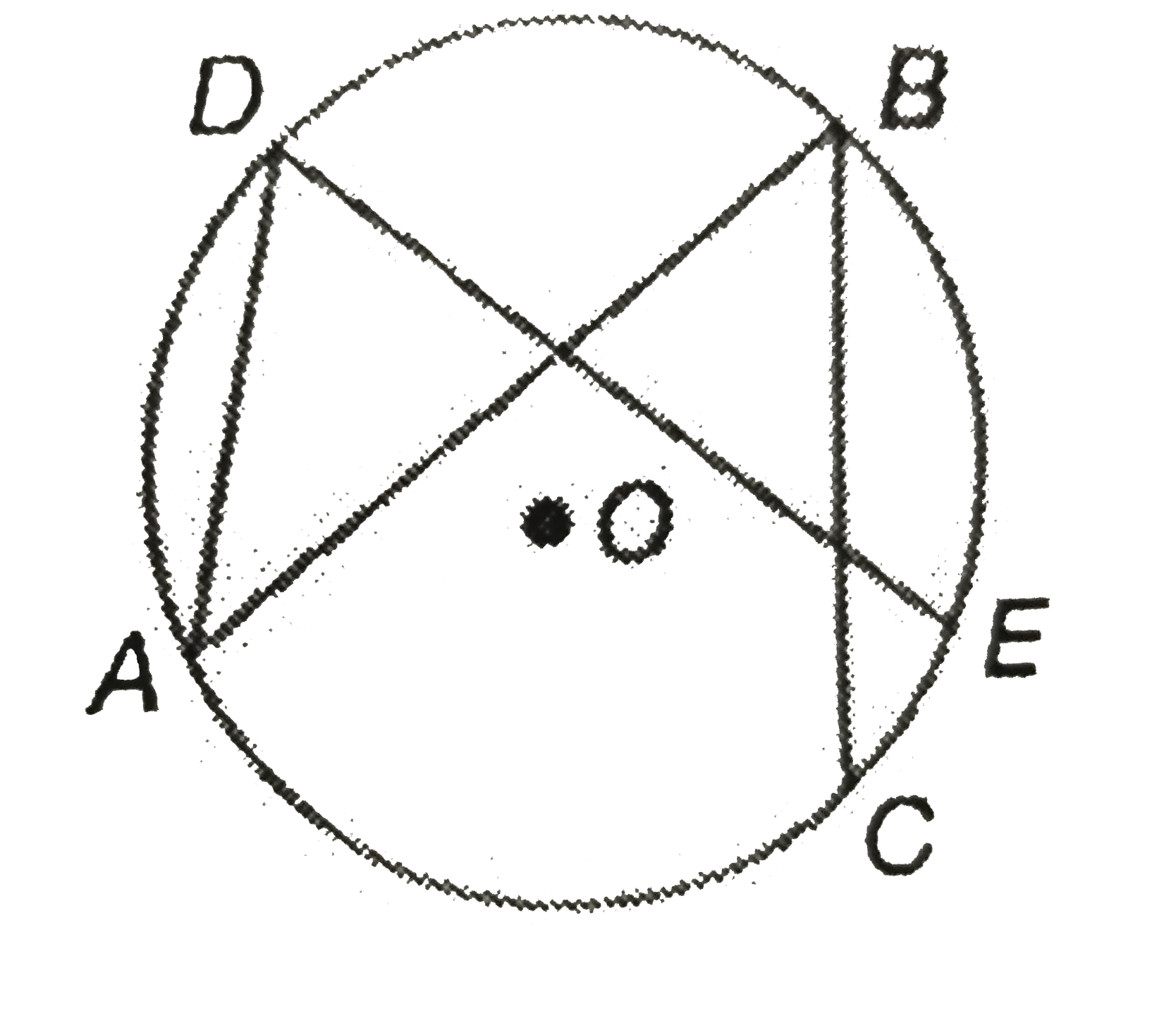
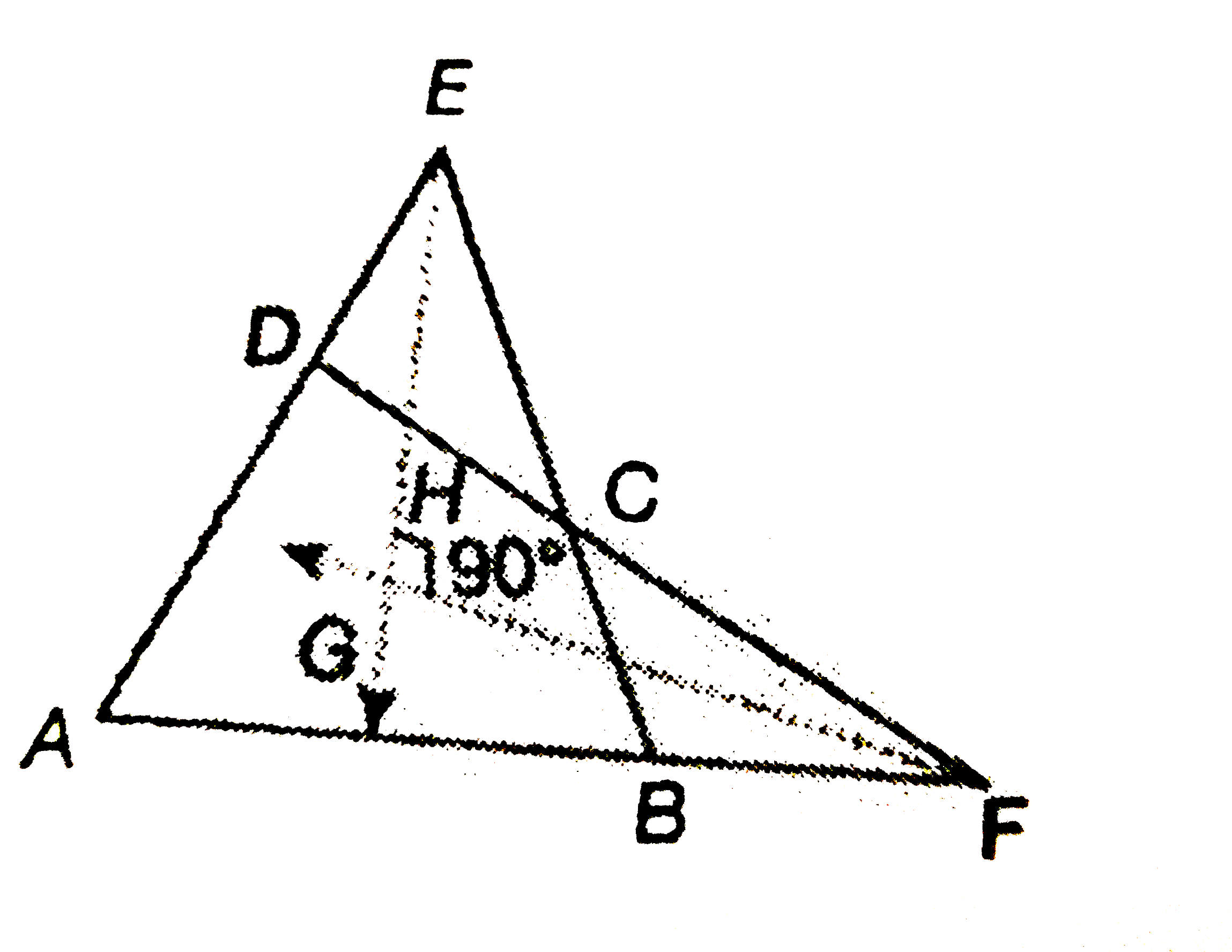
View SolutionIn the given figure, ABCD is a cyclic quadrilateral, `angleABC=70^(@), bar(FG)` bisects `angleCFA, bar(EG)` bisects `angleDEB, angleDCE = 60^(@)` and `angleEGF=90^(@)`. Find `angleHEC`.
View SolutionIn the given figure, `A,D,B,E` and `C` are concylic. If `angleACB=60^(@)` and `angleAED=50^(@)`, then find `angleDEB`.
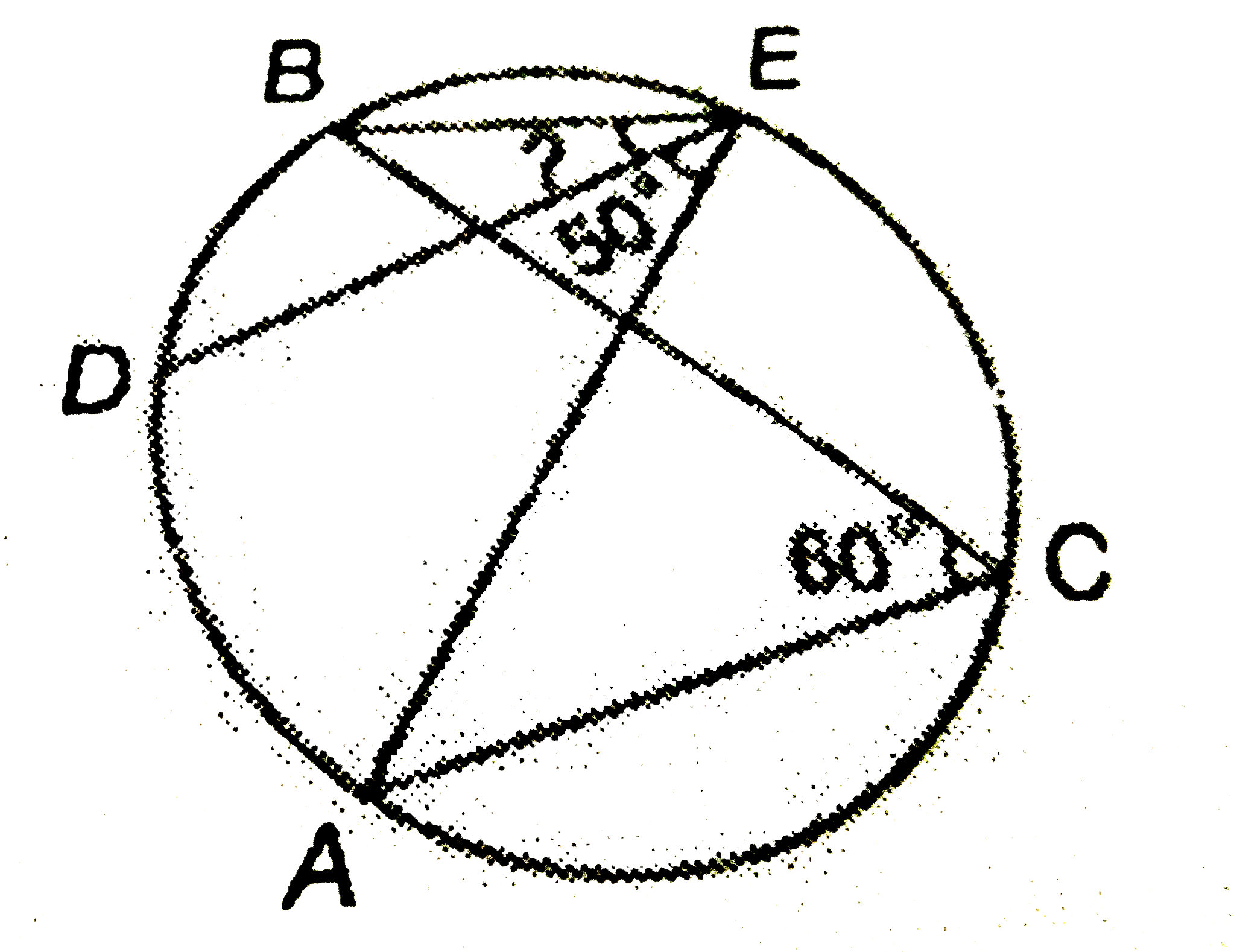
View Solution
CONCEPT APPLICATION questions and complete solutions for chapter GEOMETRY of PEARSON IIT JEE FOUNDATION of Class 9