A small body is thrown at an angle to the horizontal with the initial velocity v0. Neglecting the air drag, find:
(a) the displacement of the body as a function of time r(t),
(b) the mean velocity vector <<v>> averaged over the first t seconds and over the total time of motion.
The correct Answer is:(a) r=v0t+gt2/2; (b) ⟨v⟩t=v0+gt/2, ⟨v⟩=v0−g(v0g)/g2.
As the body is under gravity of constant accelerating →g, it's velocity vector and displacement vectors are:
→v=→v0+→gt (1)
and Δ→r=→r=→v0t+12gt2 (→r=0 at t=0) (2)
So, <→v> over the first t seconds
<→v>=Δ→rΔt=→rt=→v0+→gt2 (3)
Hence from Eq. (3), <→v> over the first t seonds
<→v>=→v0+→g2τ (4)
For evaluating t, take
→v⋅→v=(→v0+→gt)⋅(→v0+→gt)=v20+2(→v0⋅→g)t+g2t2
or, v2=v20+(→v0⋅→g)t+g2t2
But we have v=v0 at t=0 and
Also at t=τ (Figure). (also from energy conservation)
Hence using this property in Eq. (5)
v20−v20+2(→v0⋅→g)τ+g2τ2
As τ≠0, so, τ=−2(→vo⋅→g)g2
Putting this value of τ in Eq. (4), the average velocity over the time of flight
<→v≥→v0−→g(→v0⋅→g)g2

Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - The Fundamental Equation Of Dynamics59videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Laws Of Conservation Of Energy, Momentum And Angular Momentum82videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation36videos
OSCILLATIONS AND WAVES
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena38videos
THERMODYNAMICS AND MOLECULAR PHYSICS
Similar Questions
A body of mass m is thrown at an angle to the horizontal with the initial velocity v0. Assuming the air drag to be negligible, find:
(a) the momentum increment Δp that the body acquires over the first t seconds of motion,
(b) the modulus of the momentum increment Δp during the total time of motion.View SolutionA body of mass m is thrown at an angle α to the horizontal with the initial velocity v0. Find the mean power developed by gravity over the wholetime of motion of the body, and the instantaneous power of gravity as a function of time.
View Solution
Knowledge Check
- Question 1 - Select One
A body of mass m is thrown upwards at an angle θ with the horizontal with velocity v. While rising up the velocity of the mass after t second will be
A√(vcosθ)2+(vsinθ)2B√(vcosθ−vsonθ2)−gtC√v2+g2t2−(2vsinθ)gtD√v2+g2t2−(2vcosθ)gt
A body of mass m=1kg is thrown at an angle θ=45∘ to the horizontal with the initial velocity u=10m/s. Find the mean power delivered by gravity over the whole time of motion of the body.
View SolutionA body of mass m is thrown at an angle α to the horizontal with the initial velocity v0 Find (a) instantaneous power of gravity as a function of time and
(b) mean power developed by gravity in time (i) t=0 to T,
(ii) t=0 to T2, (iii) t=T2 to t=T, where T is the time of flight.View SolutionA body is thrown from the surface of the Earth at an angle α to the horizontal with the initial velocity v0. Assuming the air drag to be negligible, find:
(a) the time of motion,
(b) the maximum height of ascent and the horizontal range, at what value of the angle α they will be equal to each other,
(c) the equation of trajectory y(x), where y and x are displacements of the body along the vertical and the horizontal respectively,
(d) the curvature radii of trajectory at its initial point and at its peak.View SolutionA body is thrown at an angle αto the horizontal with initial velocity v0.Assuming air drag to be negligible , find (i)the time of motion, (ii)maximum height of ascent and the horizontal range, (iii)the angle of projection at which they are equal , (iv)the angle of projection at which the horizontal range is maximum and the angle at which the vertical range is maximum.
View SolutionA man traversed half the distance with a velocity v0. The remaining part of the distance was covered with velocity V1. For half the time and with velocity v2 for the other half of the time . Find the average speed of the man over the whole time of motion.
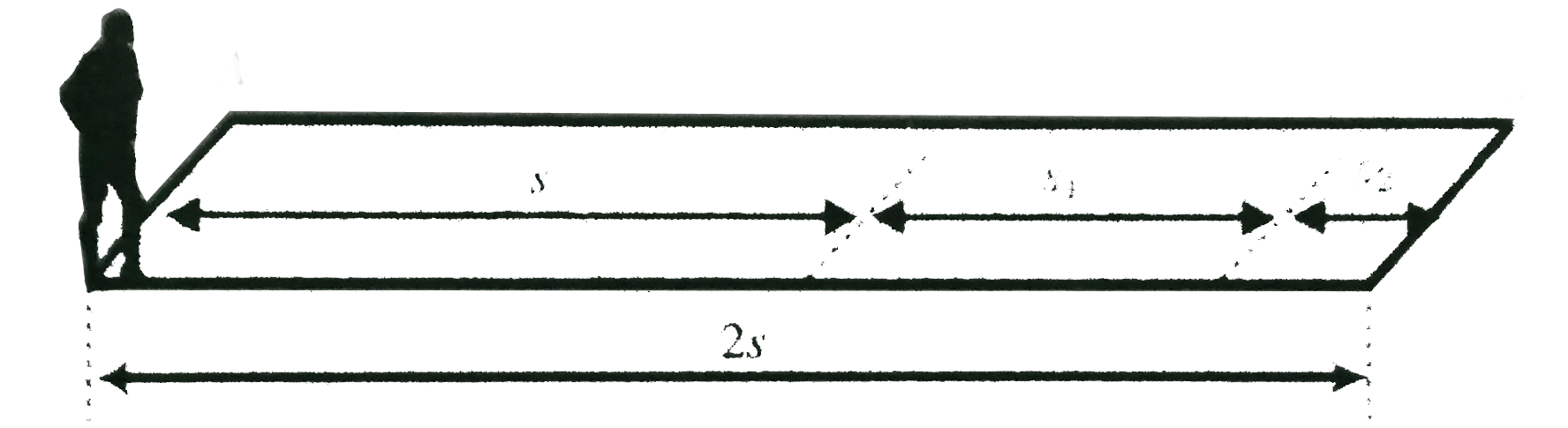 . View Solution
. View SolutionA point traversed half the distance with a velocity v0. The remaining part of the distance was covered with velocity v1 for half the time, and with velocity v2 for the other half of the time. Find the mean velocity of the point averaged over the whole time of motion.
View SolutionA radius vector of a point A relative to the origin varies with time t as r=ati−bt2j, where a and b are positive constants, and I and j are the unit vectors of the x and y axes. Find:
(a) the equation of the point's trajectory y(x), plot this function,
(b) the time dependence of the velocity v and acceleration w vectors, as well as of the moduli of these quantities,
(c) the time dependence of the angle α between the vectors w and v,
(d) the mean velocity vector averaged over the first t seconds of motion, and the modulus of this vector.View Solution

