D is any point on side AC of a ΔABC with AB=AC then
A
CD<BD
B
CD=BD
C
CD>BD
D
NONE
Video Solution
Text Solution
The correct Answer is:A
Given in ΔABC,D is any Point on side AC such that AB=AC.
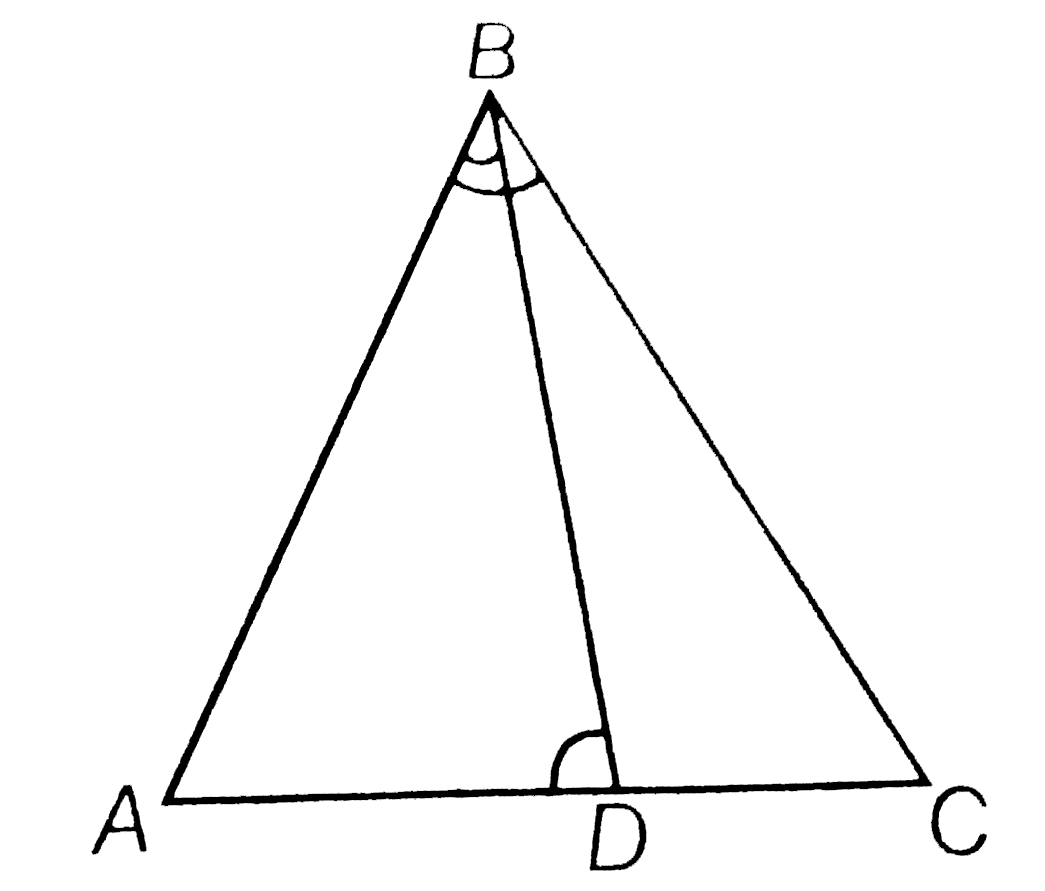
Proof in ΔABC AC=AB[given]
⇒ ∠ABC=∠ACB (i)
[angles opposites to equal sides are equal sides are equal]
InΔABCandΔDBC ∠ABC>∠DBC [since,∠DBCis a internal angle of ∠B]
⇒ ∠ACB>∠DBC [from Eq.(i)]
⇒BD>CD [side opposite to greater angle is longer]
or CD<BD
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
P is any point on the side BC of a △ABC. P is joined to a. If D and E are the midpoints of the sides AB and AC respectively and M and N are the midpoints of BP and CP respectively then quadrilateral DENM is
Aa trapeziumBa parallelogramCa rectangleDa rhombusIn ΔABC, AB=AC and D is a point on side AC such that BD = BC, If AB = 12.5 cm and BC = 5 cm, then what is the measure of DC ?
A2 cmB2.5 cmC3 cmD1.8 cmIf D is such a point on the side BC of △ABC that AB/AC=BD/CD, then AD must be a/an :
Aaltitude of △ABCBmedian of △ABCCangle bisector of △ABCDperpendicular bisector of △ABC
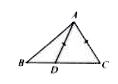
D is a point on side BC of triangle ABC such that AD = AC (see figure). Show that AB>AD.
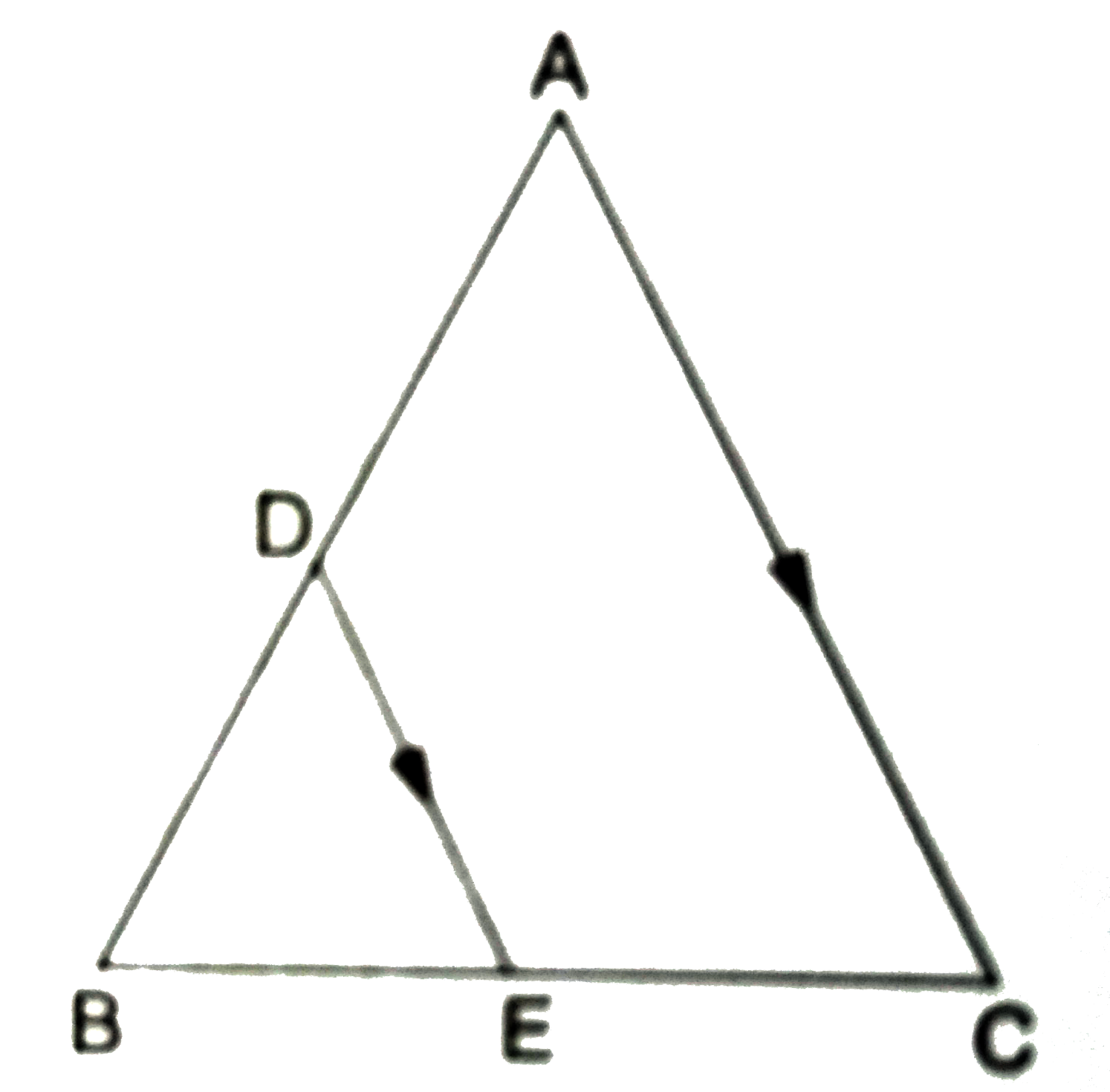
View SolutionIf D is a point on the side AB of ΔABC such that AD:BD=3:2andE is a point on BC such that DE∣∣AC, find the ratio of the areas of ΔABCandΔDBE
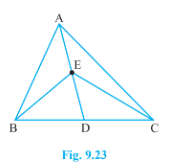
View SolutionIn Fig.9.23, E is any point on median AD of a ΔABC. Show that ar (ABE) = ar (ACE).
View SolutionD is a point on the circumcircle of ΔABC in which AB=AC such that B and D are on opposite sides of line AC. If CD is produced to a point E such that CE=BD, prove that AD=AE.
View SolutionD is a point on the circumcircle of ΔABC in which AB=AC such that B and D are on the opposite sides of AC. If CD is produced to a point E such that CE=BD. Prove that AD=AE.
View Solution
