Find the area of the region bounded by the curve y2=4x and x2=4y.
A
157 sq units
B
167 sq units
C
163 sq units
D
16 sq units
Video Solution
Text Solution
The correct Answer is:C
Given wquation of curve are
y2=4x
and x2=4y
⇒(x24)=4x
⇒x44.4=4x
⇒x4=64x
⇒x4−64x=0
⇒x(x3−43)=0
⇒x=4,0
Area of shaded region,A=∫40(√4x−x24)dx
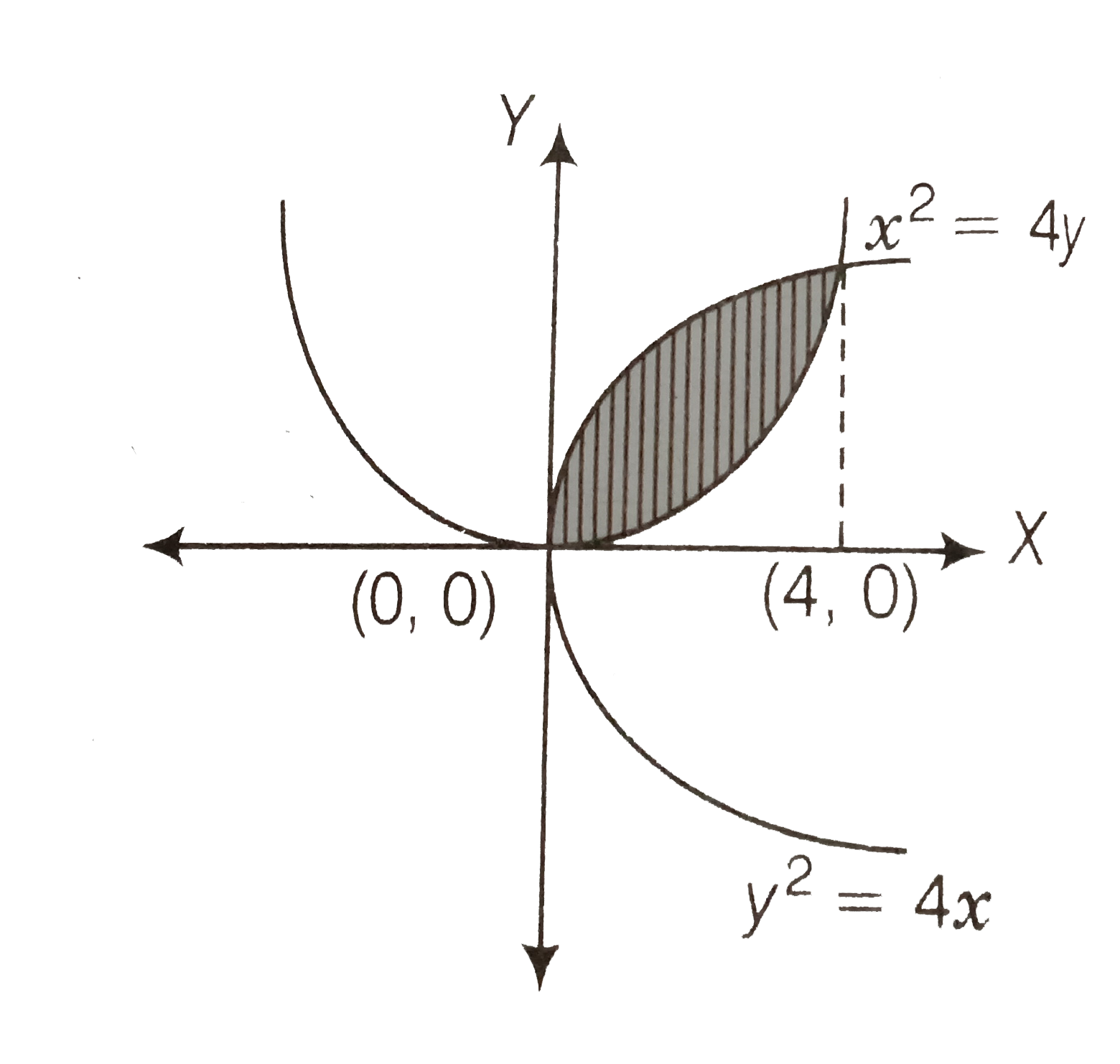
=∫40(2√x−x24)dx=[x3/2.23−14.x33]40
=2.23.8−14.643−0=323−163=163 sq units
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The area of the region bounded by the curves y=x2 and x=y2 is
A13B12C14D3Find the area of the region bounded by the curves y2=x+1 and y2=−x+1.
A53 sq. units.B73 sq. units.C83 sq. units.D103 sq. units.
Find the area of the region bounded by the curve y2=2x and x2+y2=4x.
View SolutionFind the area of the region bounded by the curve y2=2x and x2+y2=4x.
View SolutionFind the area of the region bounded by the curves y2=4ax and x2=4ay.
View SolutionFind the area of the region bounded by the curve y2=4x and the line x=3.
View SolutionFind the area of the region bounded by the curves x2+y2=4 and (x−2)2+y2=4.
View SolutionDraw a rough sketch and find the area of the region bounded by the parabolas y2=4xandx2=4y, using the method of integration.
View Solution