Find the area of the region included between y2=9x and y=x.
The correct Answer is:N/a
We have, y2=9x
⇒x2=9x
⇒x2−9x=0
⇒x(x−9)=0
⇒x=0,9
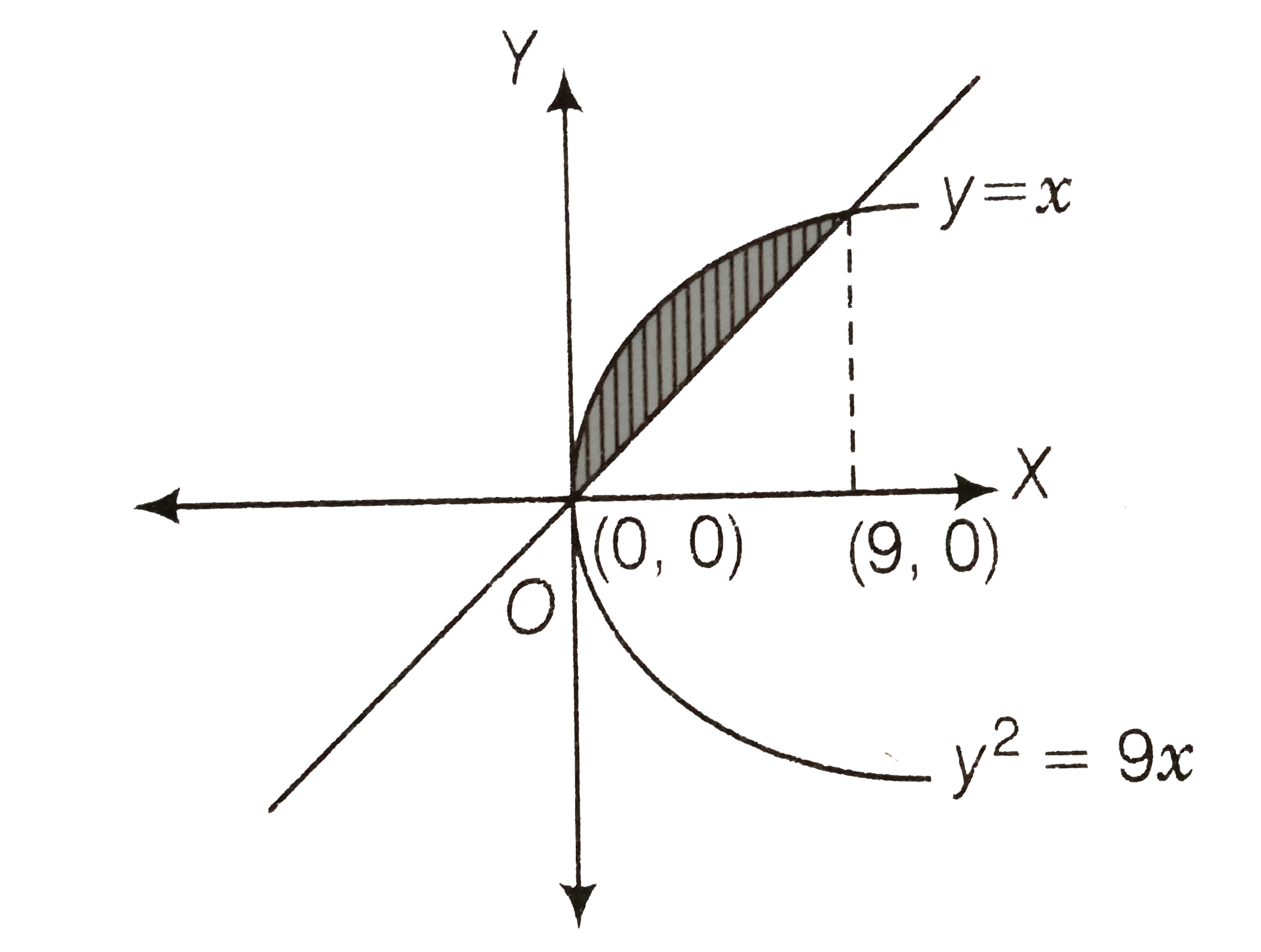
∴ Area opf shaded region,A=∫90(√9x−x)dx=∫903x1/2dx−∫90xdx
=[3.x3/23.2]90−[x22]90
=⎡⎣3.323×23.2−0⎤⎦[812−0]
=54−812=108−812=272 sq units
Topper's Solved these Questions
Similar Questions
Find the area of the region included between y=x2+5 and the line y=x+7
View SolutionFind the area of the region bounded by the curve y2=9x and y=3x.
View SolutionFind the area of the region bounded by the curve y2=9x and y=3x.
View SolutionFind the area of the region included between the parabola y2=x and the line x+y=2 .
View SolutionFind the area of the region bounded by the curve y2=2x and x2+y2=4x.
View SolutionFind the area of the region bounded by the curve y2=2x and x2+y2=4x.
View SolutionFind the area of the region included between the parabolas y2=4axandx2=4ay,wherea>0.
View SolutionFind the are of the region included between the parabola y2=3x and the circle x2+y2−6x=0 lying in the first quadrant
View SolutionFind the area of the region enclosed between curves y=|x−1| and y=3−|x|.
View SolutionFind the area of the region defied by x2+y2≤64 and y2≥12x
View Solution
