Question

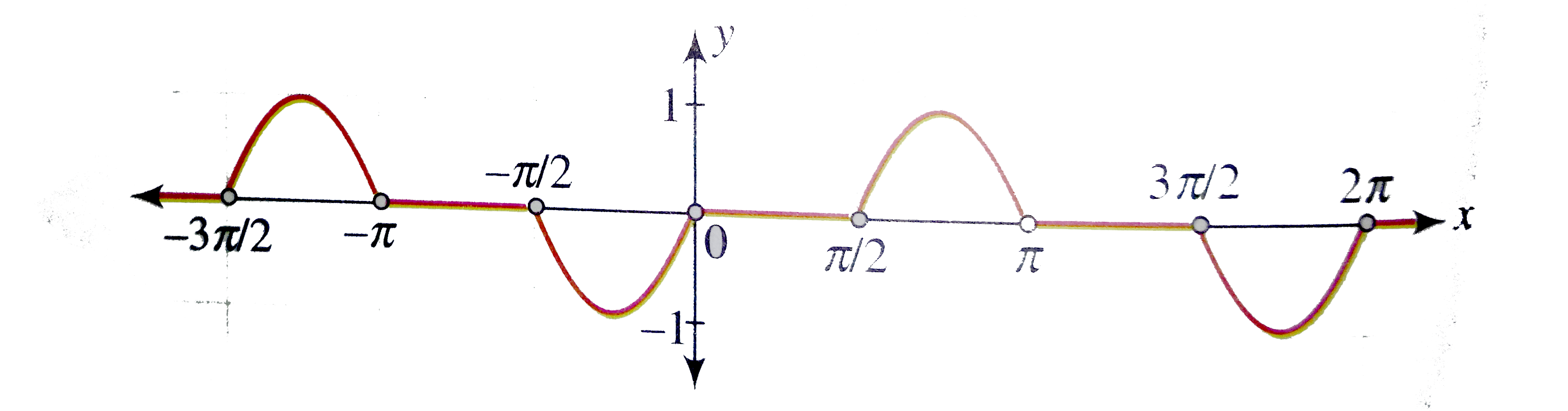
Draw the graph of $f\left(x\right)=\frac{\sin x}{\sqrt{1+{\tan }^{2}x}}-\frac{\cos x}{\sqrt{1+{\cot }^{2}x}}$. Then find the range of f(x).

Explanation

`f(x) = (sin x)/(|sec x|) - (cos x)/(|"cosec "x|)`
`= sin x *| cos x| - cos x * |sin x|`
`= {{:(0",", x in (0", "pi//2)),(-sin 2x, x in (pi//2, pi)),(0",", x in (pi, 3pi//2)),(sin 2x",", x in (3pi//2, 2pi)):}`
Clearly the domain of f(x) is `R - {(npi)/(2), n in Z}` and the period of f(x) is `2pi`.
So the range of f(x) is (-1, 1).