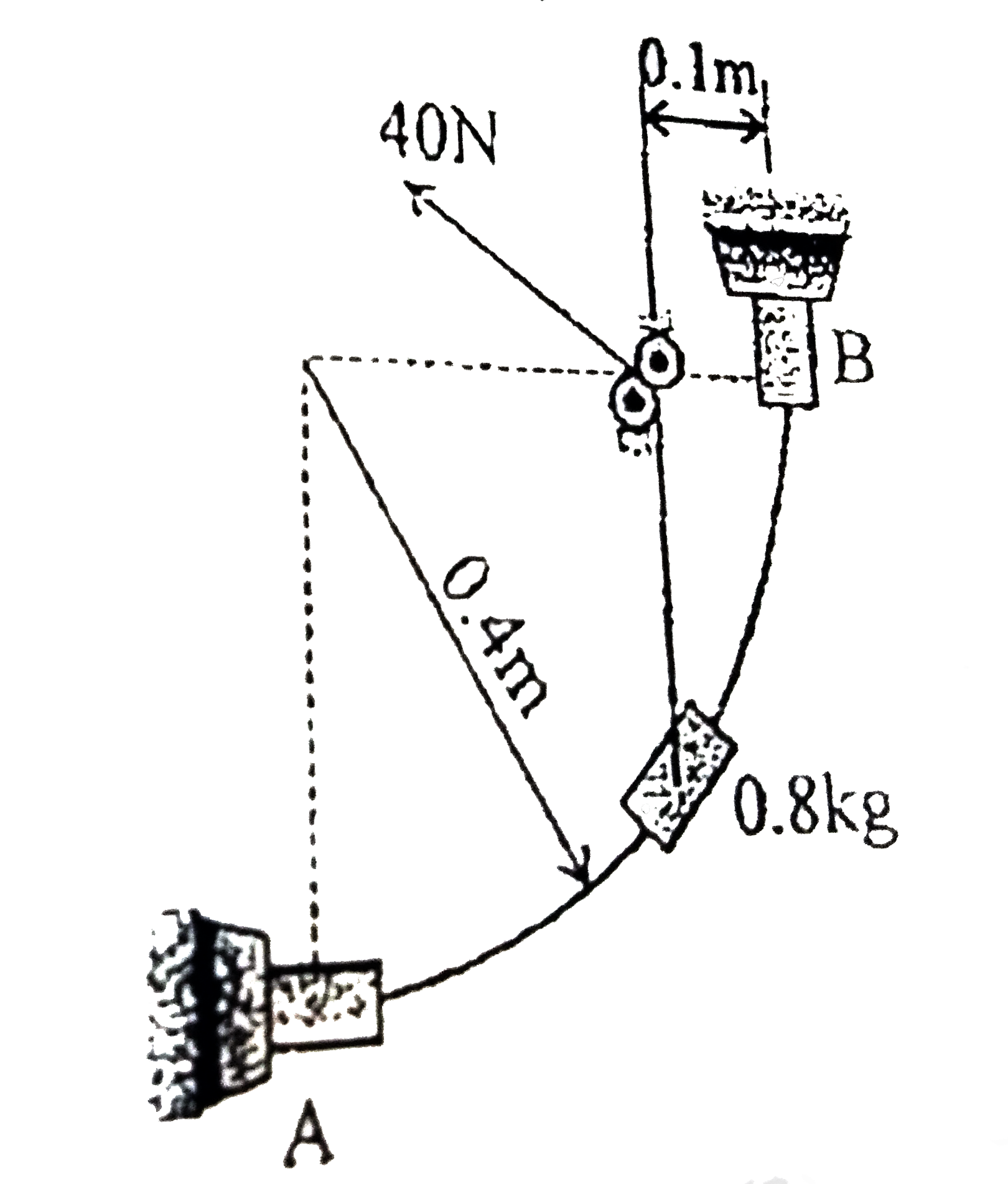
The 0.8 kg collar slides freely on the fixed vertical circular rod. Calculate the velocity v of the collar as it hits the stop at B if it elevated from rest at A by the actionof the constant 40N force in the cord. The cord is guided by the small fixed pulleys.
40m/s
4√2m/s
32m/s
8m/s
WT+Wg=ΔKE
40×(0.5−0.1)−0.8×10×0.4
=1/2mv2−0
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (A)
32 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (B)
3 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART- C
4 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
A 10 kg collar P slides with negligible friction on the fixed vertical shaft. When the collar is released from rest at the bottom position shown, it moves up the shaft under the action of the constant force F = 200 N applied to the cable. The position of the small pulley at B is fixed. Find the spring constant k (in k−N/m) which the spring must have if its maximum compression is to be limited to 0.4 m.

View SolutionA 10 kg collar P slides with negligible friction on the fixed vertical shaft. When the collar is released from rest at the bottom position shown, it moves up the shaft under the action of the constant force F = 200 N applied to the cable. The position of the small pulley at B is fixed. Find the spring constant k (in k−N/m) which the spring must have if its maximum compression is to be limited to 0.4 m.

View Solution
Knowledge Check
A small particle of mass m is given an initial high velocity in the horizontal plane and winds its cord around the fixed vertical shaft of radius a. All motion occurs essentially in horizontal plane. If the angular velocity of the cord is when the distance from the particle to the tangency point is r0, then the angular velocity of the cord after it has turned through an angle is.
 Aω=ω0Bω=ar0ω0Cω=ω01−ar0θDω=ω0θ
Aω=ω0Bω=ar0ω0Cω=ω01−ar0θDω=ω0θConsider a quarter circular smooth rod AB fixed AB fixed in a vertical plane with centre of the circle at O. A bead of mass m slides without friction with the help of string starting from point A under the action of a constant force F=2gm as shown in figure.

Work done by force F up till the moment when bead reaches at position B is : (Take m=√2kg )A100JB200JC400JD50J
A small collar of mass m = 100 g slides over the surface of a horizontal circular rod of radius R = 0.3 m. The coefficient of friction between the rod and the collar is μ=0.8. Find the angle made with vertical by the force applied by the rod on the collar when speed of the collar is V = 2 m/s.
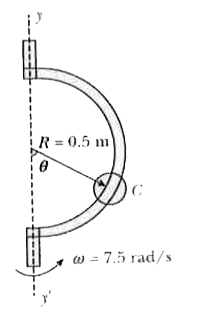
View SolutionA small 0.25 kg collar C many slide on a semicircular rod which is made to rotate about the vertical axis yy' at a constant of 7.5 rad/s. Determine the value of θ for which the collar will not slide on the rod, assuming no friction between the collar and the rod.
View SolutionA pair of parallel horizontal conducting rails of negligible resistance shorted at one end is fixed on a table. The distance between the rails is L. A conducting massless rod of resistance R can slide on the rails frictionlessly. The rod is tied to a massless string which passes over a pulley fixed to the edge of the table. A mass m tied to the other end of the string hangs vertically. A constant magnetic field B exists perpendicular to the table. If the system is released from rest, calculate
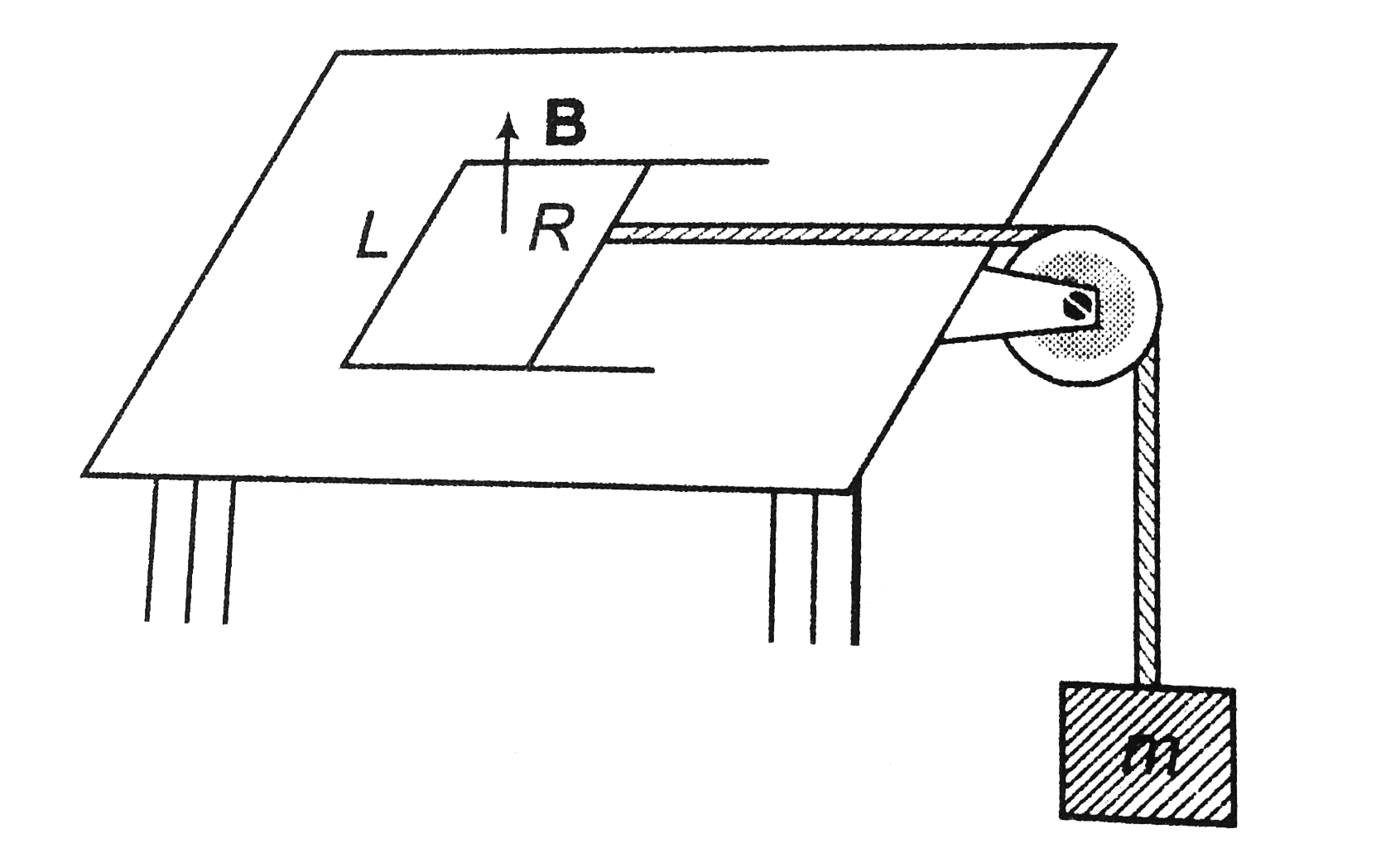
a. the terminal velocity achieved by the rod and
b. The acceleration of the mass of the instant when the velocity of the rod is half the terminal velocity.
View SolutionA pair of parallel horizontal conducting rails of negligible resistance shorted at one end is fixed on a table. The distance between the rails is L. A conducting massless rod of resistance R can slide on the rails frictionlessly. The rod is tied to a massless string which passes over a pulley fixed to the edge of the table. A mass m tied to the other end of the string hangs vertically. A constant magnetic field B exists perpendicular to the table. If the system is released from rest, calculate

a. the terminal velocity achieved by the rod and
b. The acceleration of the mass of the instant when the velocity of the rod is half the terminal velocity.
View SolutionA point charge +q is fixed at point A on the circumference of a fixed smooth non-conducting circular wire track of radius R in a gravity free space. A small ring of mass m and charge + which can slide freely on the wire track is given tangential velocity at point B which is diametrically opposite to A. If the distance of closest approach between the fixed charge and the ring is R then force applied by the wire track on the ring just after the release will be
View SolutionA ring of mass m = 0.3kg can slide freely on smooth vertical rod. A light and inextensible string is connected to the ring and passes over a smooth and fixed pulley as shown in the figure. The distance of the pulley from the rod is 0.8m and the other end of the string is connected to a block of mass M = 0.5kg. The ring is held in level with pulley and then released from rest.
The distance by which the ring moves down before coming to rest for the first time is 0.75K. Find K.
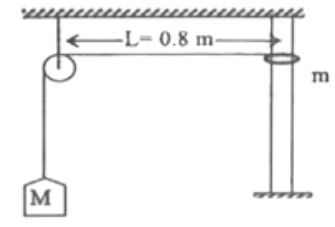
View Solution
