Find the area enclosed the curve y=sin x and the X-axis between $x = 0 and x = π$ .

A

$\frac{7}{2}$ sq. units

B

$\frac{7}{4} + \sqrt{3}$ sq. units

C

$\frac{7 \sqrt{3}}{4}$ sq. units

D

$7 - \frac{\sqrt{3}}{4}$ sq. units

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

We have $y = sin 2 x, y = \sqrt{3} sin x$
Solving, we get $sin 2 x = \sqrt{3} sin x$
$∴ 2 sin x cos x = \sqrt{3} sin x$
$∴ sin x = 0 or cos x = \sqrt{3} / 2$
$∴ x = 0, π or x = π / 6$

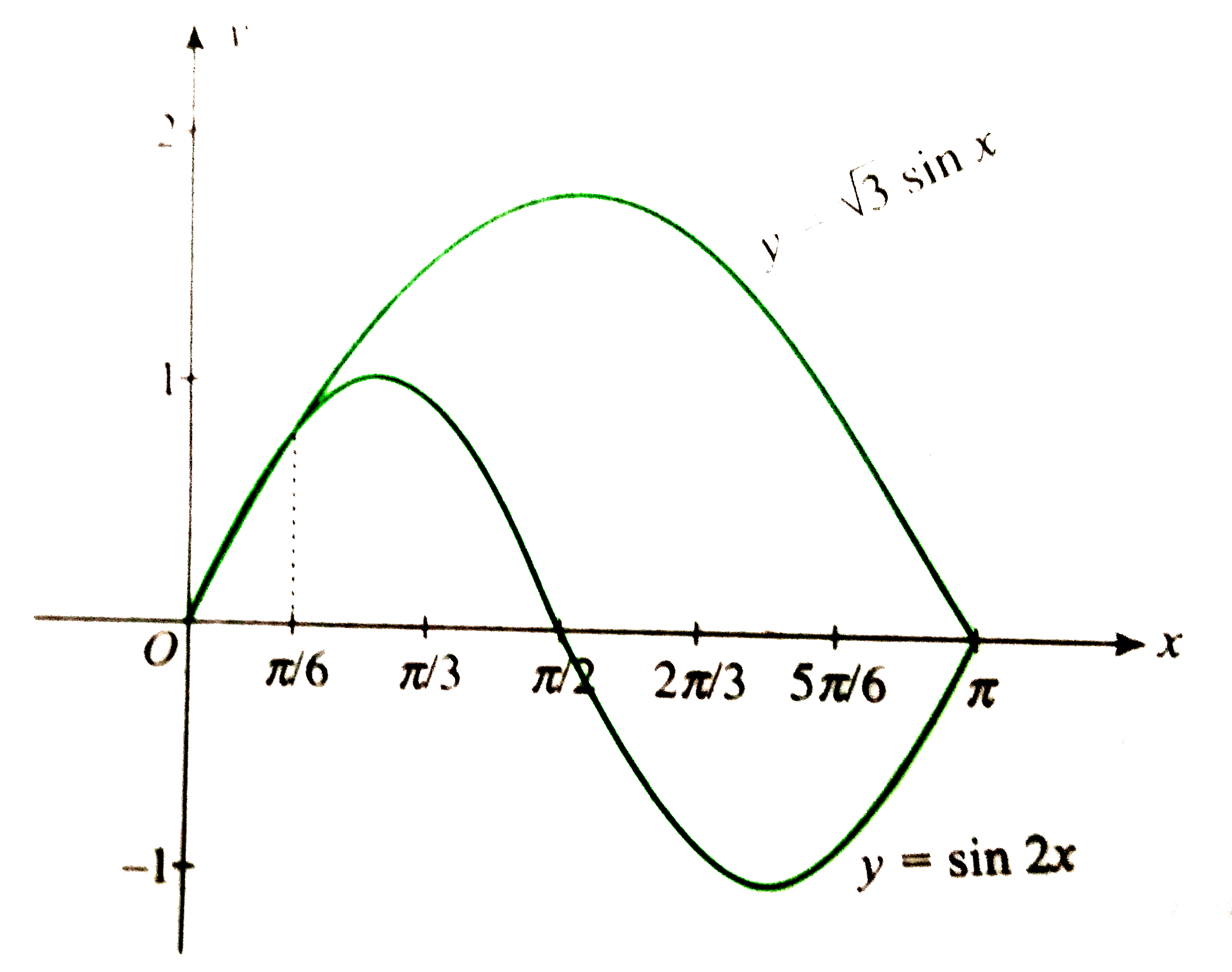
$∴ Required area$
$= π / 6 \int 0 (sin 2 x - \sqrt{3} sin x) d x + π \int π / 6 (\sqrt{3} sin x - sin 2 x) d x$
$= {[- \frac{cos 2 x}{2} + \sqrt{3} cos x]}_{0}^{π / 6} + {[- \sqrt{3} cos x + \frac{cos 2 x}{2} +]}_{π / 6}^{π}$
$= [\frac{7}{4} - \sqrt{3}] + [\sqrt{3} + \frac{7}{4}]$
$= \frac{7}{2}$

|

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Area bounded by the curves $y = x sin x and$ x-axis between $x = 0 and x = 2 π$ is
A $2 π$
B $3 π$
C $4 π$
DNone of these
Question 2 - Select One
If A is the area lying between the curve $y = sin x and$ x-axis between x=0 $and x = π / 2$ . Area of the region between the curve $y = sin 2 x and x$ -axis in the same interval is given by
A $A / 2$
BA
C $2 A$
D $3 / 2 A$
Question 3 - Select One
Area bounded by the curves $y = x sin x and$ x-axis between $x = 0 and x = 2 π$ is
A $2 π$
B $3 π$
C $4 π$
DNone

CENGAGE-AREA-Exercise (Single)

Home
Ask Doubt
Profile