The area enclosed by y=x2+cosx and its normal at x=π2 in the first quadrant is
A
π532−π464+π332+1
B
π516−π432+π324−1
C
π532−π432+π316
D
π532−π432+π324+1
Video Solution
Text Solution
The correct Answer is:D
f(x)=x2+cosx
⇒ f'(x)=2x−sinx
⇒ f'(π4)=π−1
⇒ Equation of normal at x=π2 is
(y−π44)=11−π(x−π2)
It meets x-axis at x=(π−1)π24+π2
Also f'(x)=2x−sinx>0 for x>0and<0 for x<0
So f(x) increases for x∈(0,∞)and decreases for x∈(−∞,0)
Graph of the function is as shown in the followin figure.
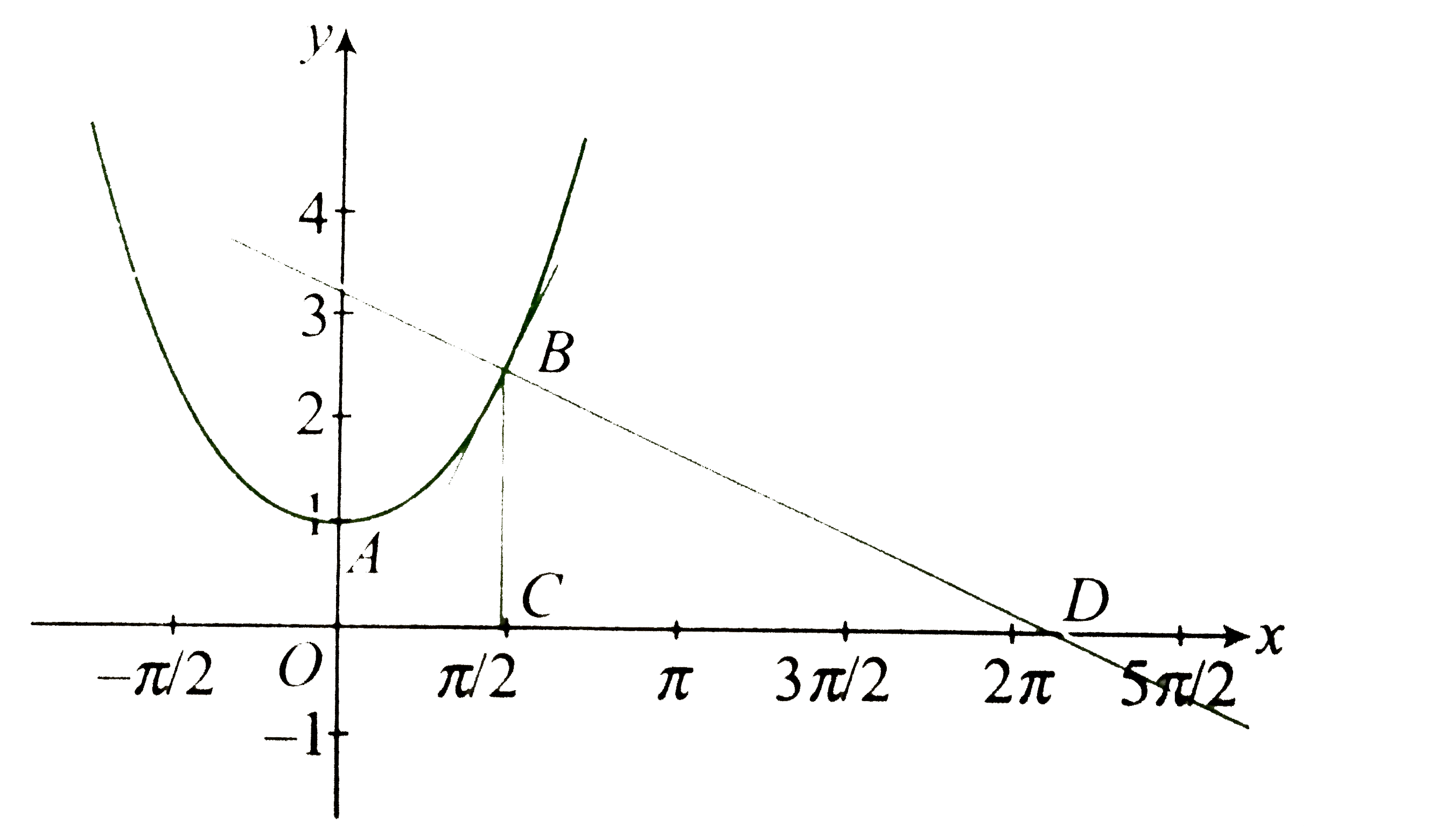
Required area
=Area OABCO+Area of ΔBCD
=π/2∫0(x2+cosx)dx+12[(π−1)π24+π2−π2]×π24
=[x33+sinx]π/20+(π−1)π28
=π324+1+π432(π−1)
=π532−π432+π324+1
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Multiple)10videos
AREA
- BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Comprehension)21videos
AREA
- BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.37videos
AREA
- BOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Question Bank29videos
APPLICATIONS OF DERIVATIVES
- BOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank20videos
AREA UNDER CURVES
Similar Questions
Knowledge Check
- Question 1 - Select One
The area of the region bounded by y2=4x,x=0,x=4 and the X-axis in the first quadrant is
A16B163C32D323 - Question 2 - Select One
The area of the region bounded by x2=y−2,y=4,y=6 and the Y-axis in the first quadrant is
A23B23(8−√2)C23(8−2√2)D32(8−√2)
Find the area of the region bounded by y2=8x,x=2,x=4 and the x-axis in the first quadrant.
View SolutionThe area bounded by the circle x2+y2=a2 and the line x+y=a in the first quadrant is
View SolutionThe area enclosed by y=x3, its normal at (1,1) and x - axis is λ, then find the value of 4λ=?
View SolutionThe area bounded by the curve y2=9x and the lines x=1,x=4andy=0, in the first quadrant,is
View SolutionFind the area of the region bounded by y2=4x,x=1,x=4 and the x-axis in the first quadrant.
View SolutionIf the area enclosed by y=x3, its normal at P(1,1) and x -axis is A8 then А equals
View Solution

