Question

Consider two curves $C_1:y^2=4\left[\sqrt{y}\right]xand{C}_2:x^2=4\left[\sqrt{x}\right]y,$
where [.] denotes the greatest integer function. Then the area of region
enclosed by these two curves within the square formed by the lines $x=1,y=1,x=4,y=4$
is
$\frac{8}{3}sq\stackrel{.}{u}nits$
(b) $\frac{10}{3}sq\stackrel{.}{u}nits$

$\frac{11}{3}sq\stackrel{.}{u}nits$
(d) $\frac{11}{4}sq\stackrel{.}{u}nits$

Options

A. `8//3` sq. units
B. `10//3` sq. units
C. `11//3` sq. units
D. `11//4` sq. units

Answer

C

Explanation

`y^(2)=4[sqrt(y)]x`
`"For "y in [1,4),[sqrt(y)]=1 or y^(2)=4x.`
`"Similarly, for "x in [1,4),[sqrt(x)]=1` and
`x^(2)=4[sqrt(x)]y" would transform into "x^(2)=4y`

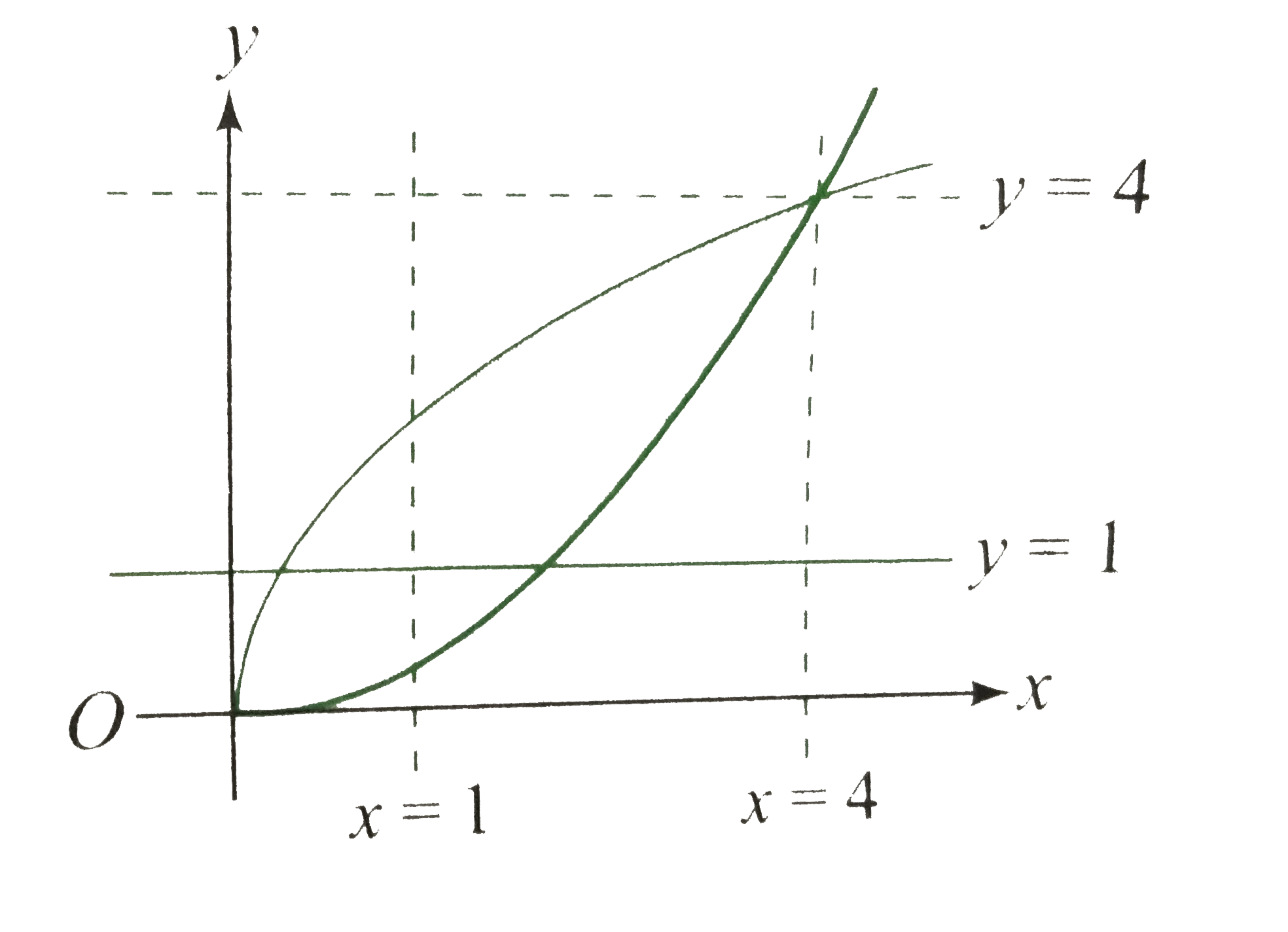
The required area is the shaded region.
`A=overset(2)underset(0)int(2sqrt(x)-1)dx+overset(4)underset(2)int(2sqrt(x)-(x^(2))/(4))dx`
`=((4)/(3)x^(3//2)-x)_(1)^(2)+((4)/(3)x^(3//2)-(x^(3))/(12))_(2)^(4)=(11)/(3)` sq. units