The area of the region containing the points (x, y) satisfy-ing 4≤x2+y2≤2(|x|+|y|) is

A

8 sq. units

B

2 sq. units

C

4π sq. units

D

2π sq. units

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:A

The points in the required region satisfy
4≤x2+y2≤2(|x|+|y|) …(1)
Since the curve (1) is symmetrical about both the axes, the required area is 4 times the area of the region in the first quadrant. Therefore, it is sufficient to sketch the region and to find the area in the first quadrant.
In the first quadrant, the curve (1) consist of two curves
x2+y2≥4 (C1)
and x2+y2−2x−2y≥0 (C2)
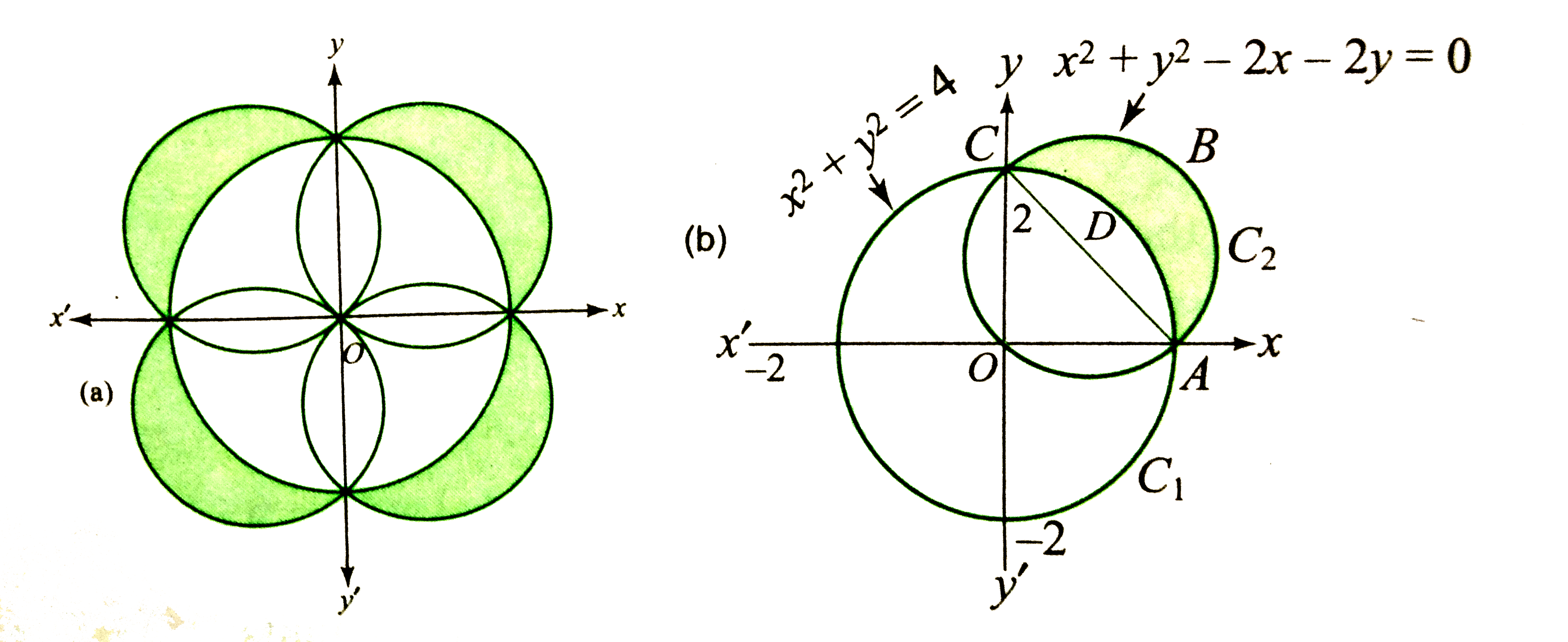
ocr_image
∴ Required area =4 (area ABCDA)
=4(area of semi-cirlce ABCA)-(area of sector ADCBA)
=4(area of semi-circle ABCA)-(area of sector OADCO-area of triangle OAC)
=4{π−(π−2}=8 sq. units.

Updated on:21/07/2023
|
Class 12MATHSAPPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Let P(x,y) be a moving point in the x-y plane such that [x].[y] = 2, where [.] denotes the greatest inte- ger function, then area of the region containing the points P(x,y) is equal to :

    A1 sq. units
    B2 sq. units
    C4 sq. units
    DNone of these

  • Area bounded by the region consisting of points (x,y) satisfying y≤√2−x2,y2≥x,√y≥−x is

    Aπ2
    Bπ
    C2π
    Dπ/4

  • Let R be the region containing the point (x, y) on the X-Y plane, satisfying 2≤|x+3y|+|x−y|≤4. Then the area of this region is

    A5 sq. units
    B6 sq. units
    C7 sq. units
    D8 sq. units