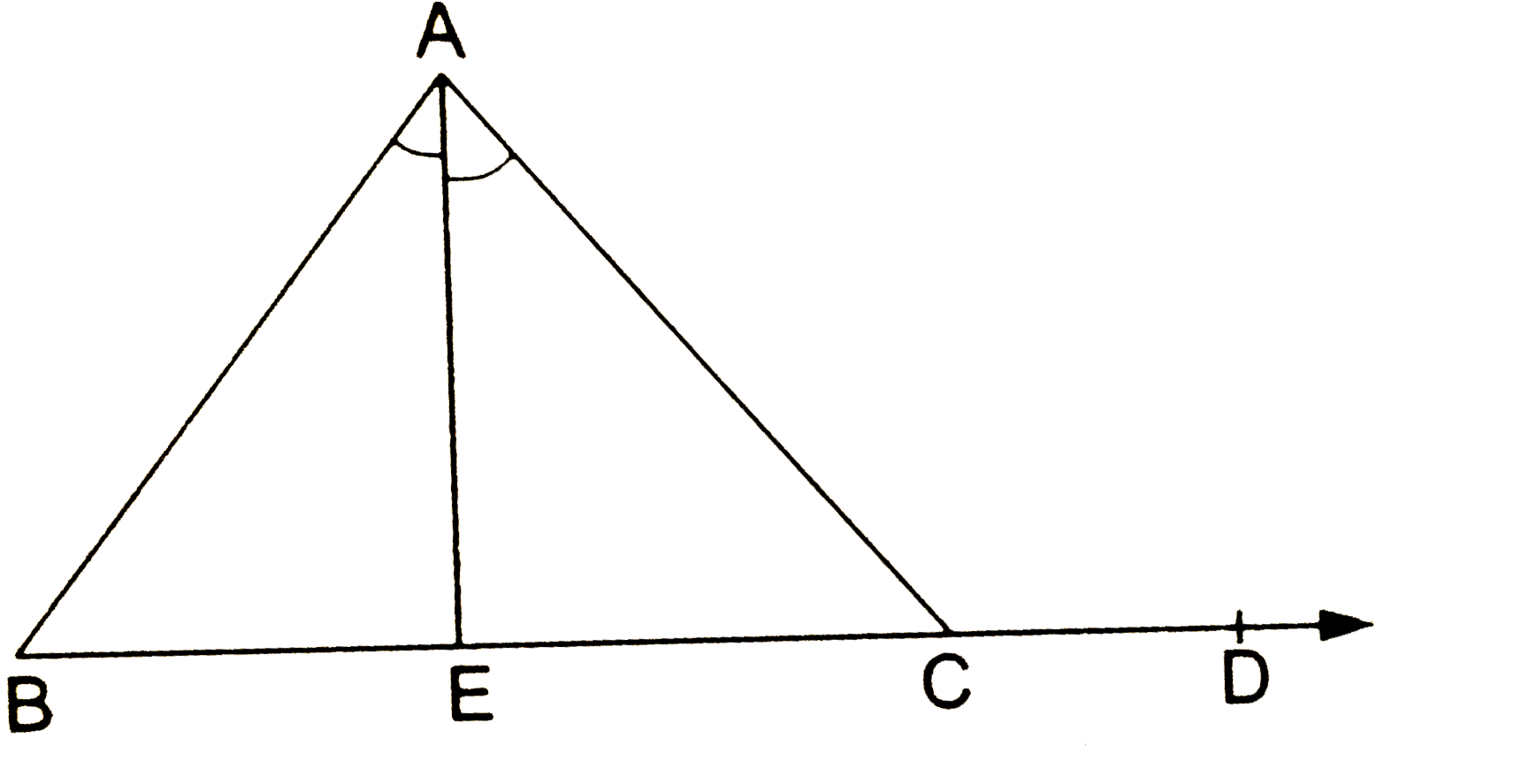
The side BC of $Δ A B C$ is produced ot D. The bisector of $∠ A$ meets BC at E. Prove that $∠ A B C + ∠ A C D = 2 ∠ A E C$ .

Video Solution

Text Solution

Verified by Experts

Side BE of $Δ A B E$ has been produced to C.
$∴ ∠ A E C = ∠ A B E + ∠ B A E$
$\Rightarrow ∠ A E C = ∠ A B C + \frac{1}{2} ∠ A$
$\Rightarrow 2 ∠ A E C = 2 ∠ A B C + ∠ A ... . (i)$
Again, side BC of $Δ A B C$ has been produced to D.
$∴ ∠ A C D = ∠ A C D + ∠ A . ... . (i i)$
On subtracting (ii) from (i), we get
$2 ∠ A E C - ∠ A C D = ∠ A B C$
$∴ ∠ A B C + ∠ A C D = ∠ A C D = 2 ∠ A E C$ .

|

Updated on:21/07/2023

Class 9 MATHS TRIANGLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The side BC of $Δ$ ABC is produced to D. If $∠ A C D = 108^{\circ}$ and $∠ B = \frac{1}{2} ∠ A$ then $∠ A$ is
A $36^{\circ}$
B $72^{\circ}$
C $108^{\circ}$
D $59^{\circ}$
Question 2 - Select One
In $△ A B C$ , $∠ B = 72^{\circ}$ and, $∠ C = 44^{\circ}$ . Side BC is produced to D. The bisectors of $∠ B$ and $∠ A C D$ meet at E. What is the measure of $∠ B E C$
त्रिभुज ABC में, $∠ B = 72^{\circ}$ तथा $∠ C = 44^{\circ}$ है | भुजा BC को D तक बढ़ाया जाता है | $∠ B$ तथा $∠ A C D$ के समद्विभाजक Eपर मिलते हैं | कोण BEC का मान क्या होगा ?
A $36^{\circ}$
B $32^{\circ}$
C $46^{\circ}$
D $58^{\circ}$
Question 3 - Select One
The side BC of $Δ A B C$ is produced to D. If $∠ A C D = 114^{\circ}$ and $∠ A B C = (\frac{1}{2}) ∠ B A C$ , what is the value (in degrees) of $∠ B A C$ ?
A36
B48
C76
D84

RS AGGARWAL-TRIANGLES-EXAMPLE

Home
Ask Doubt
Profile