Verticle Circular Motion
To solve the problem of determining the minimum velocity required for a particle to complete vertical circular motion, we can follow these steps:
1. Identify the Points in Motion:
- Let's denote point A as the top of the circular path, point C as the lowest point of the circular path, and the radius of the circle as R.
2. Apply Conservation of Energy:
- At point A, the particle has kinetic energy and potential energy. At point C, it has kinetic energy and potential energy as well. We can express this as:
Energy at A=Energy at C
- The total energy at point A is:
EA=12mu20+mg(0)=12mu20
- The total energy at point C is:
EC=12mv2+mg(−2R)=12mv2−2mgR
3. Set the Energies Equal:
- Setting the energies equal gives us:
12mu20=12mv2−2mgR
4. Simplify the Equation:
- We can cancel m from both sides (assuming m≠0):
12u20=12v2−2gR
- Rearranging gives:
u20=v2−4gR
5. Determine the Minimum Velocity at Point C:
- At point C, for the particle to just complete the circular motion, the tension in the string can be considered zero. Thus, the gravitational force provides the necessary centripetal force:
mg=mv2R
- Simplifying gives:
v2=gR
6. Substituting Back:
- Substitute v2 back into the energy equation:
u20=gR−4gR=5gR
7. Final Expression for Minimum Velocity:
- Taking the square root gives:
u0=√5gR
Conclusion:
The minimum velocity u0 required at point A for the particle to complete the vertical circular motion is:
u0=√5gR
- Let's denote point A as the top of the circular path, point C as the lowest point of the circular path, and the radius of the circle as R.
- At point A, the particle has kinetic energy and potential energy. At point C, it has kinetic energy and potential energy as well. We can express this as:
Energy at A=Energy at C
- The total energy at point A is:
EA=12mu20+mg(0)=12mu20
- The total energy at point C is:
EC=12mv2+mg(−2R)=12mv2−2mgR
- Setting the energies equal gives us:
12mu20=12mv2−2mgR
- We can cancel m from both sides (assuming m≠0):
12u20=12v2−2gR
- Rearranging gives:
u20=v2−4gR
- At point C, for the particle to just complete the circular motion, the tension in the string can be considered zero. Thus, the gravitational force provides the necessary centripetal force:
mg=mv2R
- Simplifying gives:
v2=gR
- Substitute v2 back into the energy equation:
u20=gR−4gR=5gR
- Taking the square root gives:
u0=√5gR
The minimum velocity u0 required at point A for the particle to complete the vertical circular motion is:
u0=√5gR
Similar Questions
A bob is tied up with a string AB of length l. End A of the string is fixed. There is a obstacle C vertically below A so that length AC=2l3. Bob is released from a height 'h' from its lowermost possible position so that it is just able to comlete verticle circular motion about C. Find 'h'.

View SolutionA stone tied to a string is rotated a vertical circle. The minimum speed of the stone during a complete vertical circular motion.
View SolutionAssertion In circular motion, average speed and average velocity are never equal.
Reason In any curvilinear path, these two are never equal.
View SolutionAssertion In circular motion, dot product of v and ω is always zero.
Reason ω is always perpendicular to the plane of the circular motion.
View SolutionA particle in a circular motion covers a distance of 0.5 πr where r is the radius of the circular path. What is the angular displacement ?
View SolutionThe position vector of a particle in a circular motion about the origin sweeps out equal areal in equal time. Its
View SolutionA particle of mass m is doing horizontal circular motion with the help of a string (conical pendulum) as shown in the figure. If speed of the particle is constant then,
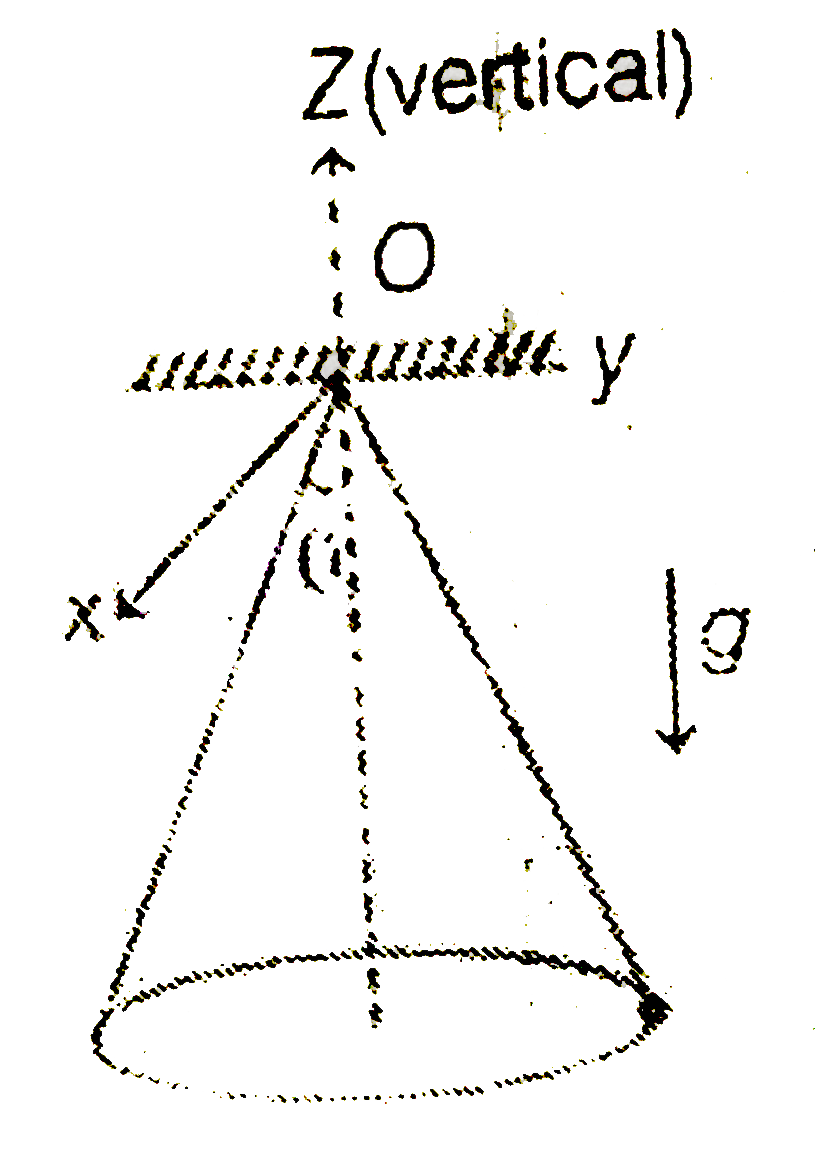
View SolutionHow does rotatory motion differ from circular motion?
View SolutionWhat is meant by circular motion? Give one example.
View SolutionAssertion A charged particle is rotating in a circular path in uniform magnetic field. Then, plane of circle is perpendicular to the magnetic field.
Reason Circular motion is a two-dimensional motion.
View Solution
