Centre of Mass
To solve the problem of finding the center of mass for a system of masses, we can break it down into a step-by-step process.
1. Understanding the Concept of Center of Mass:
- The center of mass (CM) is a point that represents the average position of all the mass in a system. For a system of discrete particles, the CM is the point where the total mass can be considered to be concentrated.
2. Formula for Discrete Mass System:
- For a system of discrete particles, the center of mass →RCM is given by the formula:
→RCM=m1→r1+m2→r2+m3→r3+…+mn→rnm1+m2+m3+…+mn
- Here, mi is the mass of the ith particle and →ri is the position vector of the ith particle.
3. Example Calculation:
- Suppose we have two masses, m1=2kg located at →r1=(1,0) m and m2=3kg located at →r2=(4,0) m.
- We can calculate the center of mass as follows:
→RCM=m1→r1+m2→r2m1+m2
→RCM=(2kg⋅(1,0)m)+(3kg⋅(4,0)m)2kg+3kg
→RCM=(2,0)+(12,0)5=(14,0)5=(2.8,0)m
4. Continuous Mass System:
- For a continuous mass distribution, we use integration to find the center of mass. The formula becomes:
→RCM=∫xdmM
- Here, dm is an infinitesimal mass element and M is the total mass.
5. Example of a Uniform Rod:
- For a uniform rod of length L, the center of mass is located at L2 from one end.
- The center of mass (CM) is a point that represents the average position of all the mass in a system. For a system of discrete particles, the CM is the point where the total mass can be considered to be concentrated.
- For a system of discrete particles, the center of mass →RCM is given by the formula:
→RCM=m1→r1+m2→r2+m3→r3+…+mn→rnm1+m2+m3+…+mn
- Here, mi is the mass of the ith particle and →ri is the position vector of the ith particle.
- Suppose we have two masses, m1=2kg located at →r1=(1,0) m and m2=3kg located at →r2=(4,0) m.
- We can calculate the center of mass as follows:
→RCM=m1→r1+m2→r2m1+m2
→RCM=(2kg⋅(1,0)m)+(3kg⋅(4,0)m)2kg+3kg
→RCM=(2,0)+(12,0)5=(14,0)5=(2.8,0)m
- For a continuous mass distribution, we use integration to find the center of mass. The formula becomes:
→RCM=∫xdmM
- Here, dm is an infinitesimal mass element and M is the total mass.
- For a uniform rod of length L, the center of mass is located at L2 from one end.
Similar Questions
Statement-1 : The centre of mass and centre of gravity of a body are two different positions in general.
and
Statement-2 : The centre of mass and centre of gravity of a body coincide if graviational field is unifrom.
View SolutionConsidering a system having two masses m1 and m2 in which first mass is pushed towards centre of mass by a distance a, the distance required to be moved for second mass to keep centre of mass at same position is :-

View Solution
Knowledge Check
As the temperature is increased, the period of a pendulum a) Increases as its effective length increases even through its centre of mass still remains at the centre of the bob. b) decreases as its effective length increases even though its centre of mass still remains at the centre of the bob. c) Increases as its effective length increases due to shifting of centre of mass below the centre of the bob. d) decreases as its effective length remains same but the centre of mass shifts above the centre of the bob.
AIncreases as its effective length increases even through its centre of mass still remains at the centre of the bob.Bdecreases as its effective length increases even though its centre of mass still remains at the centre of thed bob.CIncreases as its effective length increases due to shifting of centre of mass below the centre of the bob.Ddecreases as its effective length remains same but the centre of mass shifts above the centre of the bob.
If net force on a system of particles is zero. Then
Column IColumn II(A)Acceleration of centre of mass(p)Constant(B)Velocity of centre of mass(q)Zero(C)Momentum of centre of mass(r)May be zero(D)Velocity of an individual particle of the system(s)May be constant
View SolutionThe mass of a body cannot be considered to be concentrated at the centre of mass of the body for the purpose of computing its moment of inertia.
For then the moment of inertia of every body about an axis passing through its centre of mass would be zero.
View SolutionA uniform circular disc of mass M and radius R is pivoted at distance x above the centre of mass of the disc, such that the time period of the disc in the vertical plane is infinite. What is the distance between the pivoted point and centre of mass of the disc ?
View SolutionSTATEMENT-1 : The relation →τ=d→Ldt is applicable in centre of mass frame, even though the centre of mass is accelerating.
and
STATEMENT-2 : The relation →τ=d→Ldt can be directly applied in a non-inertial frame.
View SolutionAssertion: A constant force F is applied on two blocks and one spring system as shown in figure. Velocity of centre of mass increases linearly with time.

Reason: Acceleration of centre of mass is constant.
View SolutionIn a double star system one of mass m1 and another of mass m2 with a separation d rotate about their common centre of mass. Then rate of sweeps of area of star of mass m1 to star of mass m2 about their common centre of mass is
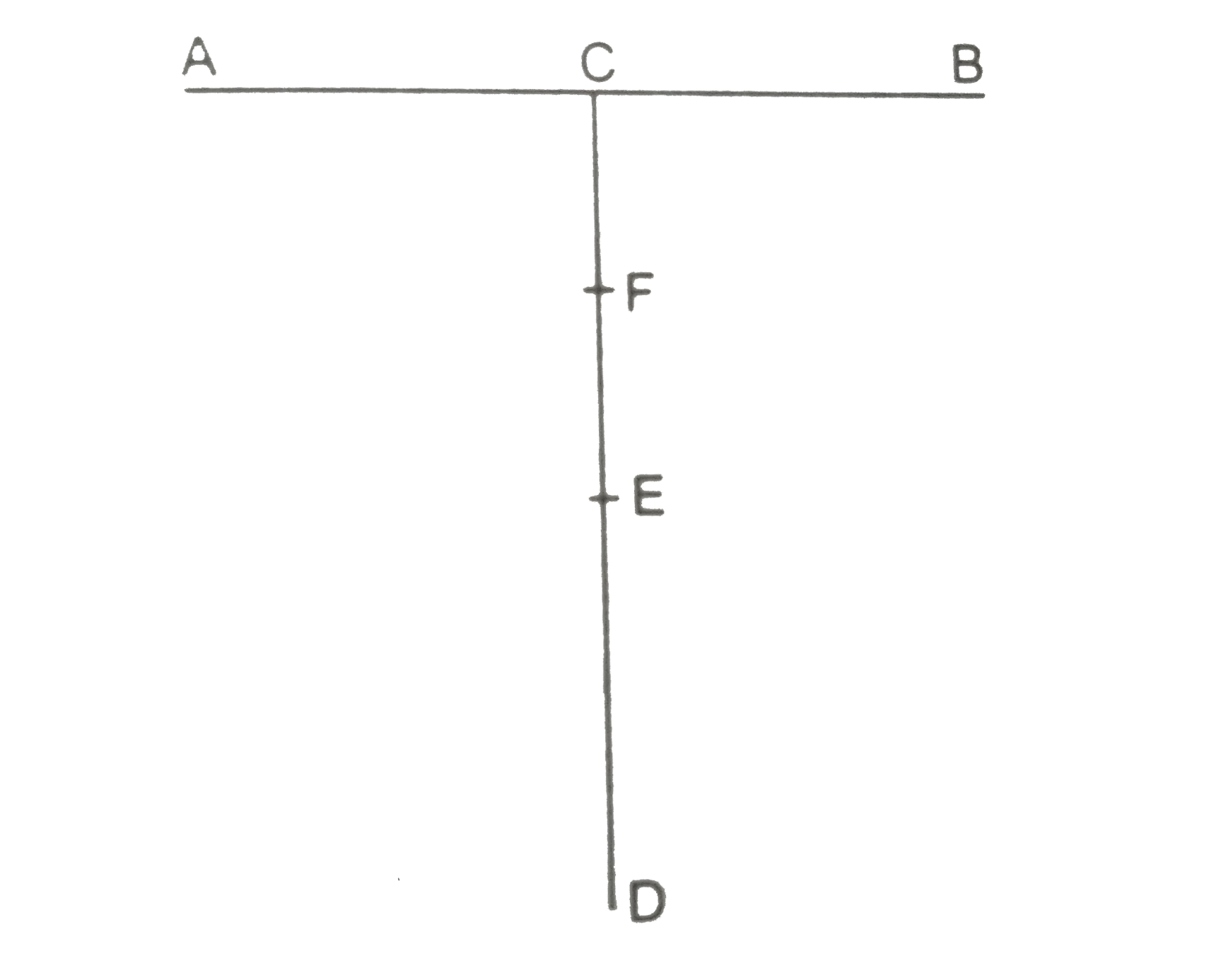
View SolutionTwo identical uniform rods AB and CD, each of length L are jointed to form a T-shaped frame as shown in figure. Locate the centre of mass of the frame. The centre of mass of a uniform rod is at the middle point of the rod.
View Solution
