In Figure, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD=2 cm , find the area of the (i) quadrant OACB (ii) shaded region.
To solve the problem step by step, we will find the area of the quadrant OACB and the area of the shaded region.
Step 1: Find the area of the quadrant OACB
The area of a quadrant of a circle can be calculated using the formula:
Area of Quadrant=14×πr2
where r is the radius of the circle.
Given:
- Radius r=3.5cm
Substituting the value of r:
Area of Quadrant=14×π×(3.5)2
Calculating (3.5)2:
(3.5)2=12.25
Now substituting this back:
Area of Quadrant=14×π×12.25
Using π≈227:
Area of Quadrant=14×227×12.25
Calculating:
Area of Quadrant=22×12.254×7=271.528≈9.625cm2
Step 2: Find the area of the shaded region
The shaded region is the area of the quadrant minus the area of triangle OBD.
Step 2.1: Find the area of triangle OBD
The area of a triangle can be calculated using the formula:
Area of Triangle=12×base×height
In triangle OBD:
- Base OB=3.5cm (radius)
- Height OD=2cm
Substituting these values:
Area of Triangle=12×OB×OD=12×3.5×2
Calculating:
Area of Triangle=12×7=3.5cm2
Step 2.2: Calculate the area of the shaded region
Now, we can find the area of the shaded region:
Area of Shaded Region=Area of Quadrant−Area of Triangle
Substituting the values:
Area of Shaded Region=9.625−3.5=6.125cm2
Final Answers:
(i) Area of the quadrant OACB=9.625cm2
(ii) Area of the shaded region = 6.125cm2
---
Area of Quadrant=14×πr2
where r is the radius of the circle.
- Radius r=3.5cm
Area of Quadrant=14×π×(3.5)2
(3.5)2=12.25
Area of Quadrant=14×π×12.25
Area of Quadrant=14×227×12.25
Area of Quadrant=22×12.254×7=271.528≈9.625cm2
Area of Triangle=12×base×height
- Base OB=3.5cm (radius)
- Height OD=2cm
Area of Triangle=12×OB×OD=12×3.5×2
Area of Triangle=12×7=3.5cm2
Area of Shaded Region=Area of Quadrant−Area of Triangle
Substituting the values:
Area of Shaded Region=9.625−3.5=6.125cm2
(i) Area of the quadrant OACB=9.625cm2
Topper's Solved these Questions
Similar Questions
In Fig. 12.30, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD=2cm, find the area of the
(i) quadrant OACB,
(ii) shaded region.
View SolutionIn Figure, OCDE is a rectangle inscribed in a quadrant of a circle of radius 10cm. If OE=2√5cm , find the area of the rectangle.
View SolutionIn Figure, O is the centre of the circle. Find ∠BAC
View SolutionIn a circle with centre O and radius 5cm, AB is a chord of length 5√3cm . Find the area of sector AOB.
View SolutionIn Figure, O is the centre of the circle. Find ∠CBD
View SolutionIn the figure, AB is a diameter of the circle with centre O and OA = 7cm. Find the area of the shaded region
View SolutionIn Figure, ABCD is a cyclic quadrilateral; O is the centre of the circle. If ∠BOD=1600, find the measure of ∠BPD
View SolutionIn Fig. 15.99, a square OABC is inscribed in a quadrant OPBQ of a circle. If OA=21 cm , find the area of the shaded region. (FIGURE)
View SolutionIn the given figure, AB and PQ are perpendicular diameters of the circle whose centre is O and radius OA = 7 cm. Find the area of the shade region.

View SolutionIn Figure , a square OPQR is inscribed in a quadrant OAQB of a circle. If the radius of circle is 6√2 cm, find the area of the shaded region
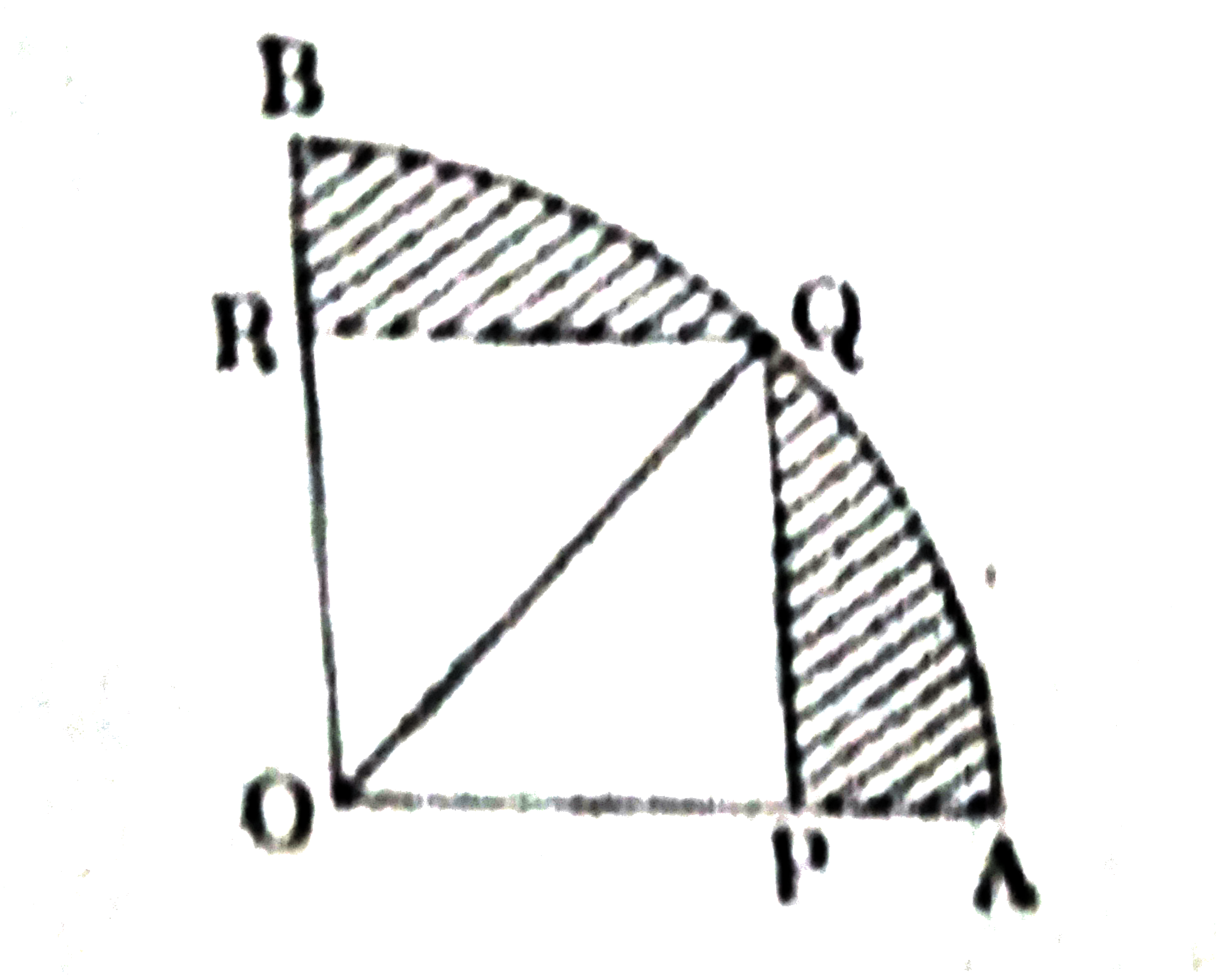
View Solution
