k transparent slabs are arranged one over another. The refractive indices of the slabs are g„ ...Ilk and the thicknesses are t1,t2,t3.............,tk . An object is seen through this combination with nearly perpendicular light. Find the equivalent refractive index of the system which will allow the image to be formed at the same place.
The correct Answer is:A
To find the equivalent refractive index of a system of k transparent slabs arranged one over another, we can follow these steps:
Step 1: Understand the Problem
We have k slabs, each with a thickness ti and a refractive index μi. We need to find the equivalent refractive index μ of the entire system such that the image formed through this combination is at the same place as it would be without the slabs.
Step 2: Calculate the Shift for Each Slab
The shift Si caused by each slab can be calculated using the formula:
Si=ti(1−1μi)
This formula accounts for the fact that light travels slower in a medium with a refractive index greater than 1.
Step 3: Total Shift for the System
The total shift S for the entire system of k slabs is the sum of the individual shifts:
S=S1+S2+S3+…+Sk=k∑i=1ti(1−1μi)
Step 4: Equivalent Shift for a Single Slab
Now, if we replace the entire system of slabs with a single equivalent slab of thickness T (where T=t1+t2+…+tk) and refractive index μ, the shift for this equivalent slab can be expressed as:
S=T(1−1μ)
Step 5: Set the Two Shifts Equal
To ensure that the image is formed at the same place, we set the total shift of the slabs equal to the shift of the equivalent slab:
k∑i=1ti(1−1μi)=T(1−1μ)
Step 6: Substitute T
Substituting T=t1+t2+…+tk into the equation gives:
k∑i=1ti(1−1μi)=(t1+t2+…+tk)(1−1μ)
Step 7: Rearranging the Equation
Rearranging the equation leads to:
k∑i=1ti−k∑i=1tiμi=(t1+t2+…+tk)−(t1+t2+…+tk)μ
Step 8: Solve for μ
Now, we can solve for μ:
(t1+t2+…+tk)μ=k∑i=1tiμi
Thus, we can express μ as:
μ=(t1+t2+…+tk)∑ki=1tiμi
Final Answer
The equivalent refractive index μ of the system is given by:
μ=T∑ki=1tiμi
where T=t1+t2+…+tk.
---
We have k slabs, each with a thickness ti and a refractive index μi. We need to find the equivalent refractive index μ of the entire system such that the image formed through this combination is at the same place as it would be without the slabs.
The shift Si caused by each slab can be calculated using the formula:
Si=ti(1−1μi)
This formula accounts for the fact that light travels slower in a medium with a refractive index greater than 1.
The total shift S for the entire system of k slabs is the sum of the individual shifts:
S=S1+S2+S3+…+Sk=k∑i=1ti(1−1μi)
Now, if we replace the entire system of slabs with a single equivalent slab of thickness T (where T=t1+t2+…+tk) and refractive index μ, the shift for this equivalent slab can be expressed as:
S=T(1−1μ)
To ensure that the image is formed at the same place, we set the total shift of the slabs equal to the shift of the equivalent slab:
k∑i=1ti(1−1μi)=T(1−1μ)
Substituting T=t1+t2+…+tk into the equation gives:
k∑i=1ti(1−1μi)=(t1+t2+…+tk)(1−1μ)
Rearranging the equation leads to:
k∑i=1ti−k∑i=1tiμi=(t1+t2+…+tk)−(t1+t2+…+tk)μ
Now, we can solve for μ:
(t1+t2+…+tk)μ=k∑i=1tiμi
Thus, we can express μ as:
μ=(t1+t2+…+tk)∑ki=1tiμi
The equivalent refractive index μ of the system is given by:
μ=T∑ki=1tiμi
where T=t1+t2+…+tk.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXAMPLE
9 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Question For short Answer
18 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Objective -2
7 Videos
- GAUSS LAWBOOK - HC VERMA ENGLISHCHAPTER - GAUSS LAWEXERCISE - Short Question
7 Videos
- LIGHT WAVESBOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Question for short Answer
11 Videos
Similar Questions
The n transparent slabs of refractive index1.5 each having thickness 1cm, 2 cm,…to n cm are arranged one over another. A point object is seen through this combineation with near perpendicular light. If the shift of object by the combination is 1 cm, then find the value of n.
View SolutionAn object is seen through a glass slab of thickness 36cm and refractive index 3/2. The observer, and the slab are dipped in water (μ=4/3). The shift produced in the position of the object is
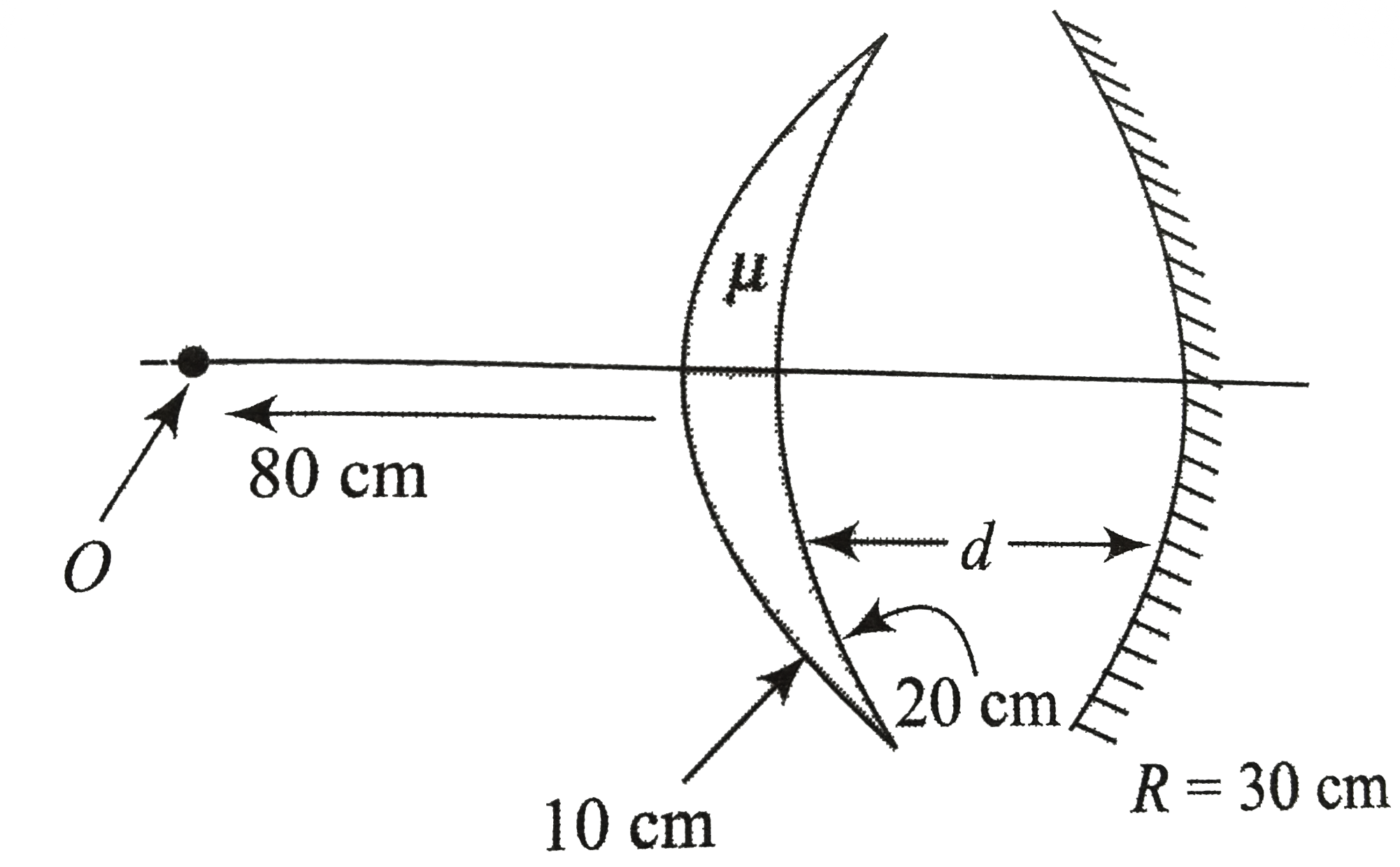
View SolutionIf final image after two refractions through the lens and one reflection from the mirror forms at the same point O. Refrative index of the material of the lens μ=3/2. Then find d.
View SolutionThree slabs of same surface area but different conductivities k1,k2,k3 and different thickness t1,t2,t3 are placed in close contact. After steady state this combination behaves as a single slab. Find its effective thermal conductivity.

View SolutionA convex lens of focal length f1 is placed in front of a luminous point object. The separation between the object and the lens is 3f1 . A glass slab of thikness t is placed between the object and the lens. A real image of the object is formed at the shortest possible distance from the object.
a. Find the refractive index of the slab.
b. If a concave lens of very large focal length f2 is placed in contact with the convex lens, find the shifting of the image.
View SolutionFigure shows three transparent media of refractive indices μ1,μ2andμ3. A point object O is placed in the medium μ2. If the entire medium on the right of the spherical surface has refractive index μ1, the image forms at O'. If this entire medium has refractive index μ3 the image form at O''. In the situation shown

View SolutionA ray of light passes through two slabs of same thickness. In the first slab n1 waves are formed and in the second slab n2. Find refractive <br> index of second medium with respect to first.
View SolutionA ray of light is incident on a parallel slab of thickness t and refractive index n. If the angle of incidence θ is small, than the lateral displacement in the incident and emergent ray will be
View SolutionA ray of light travelling in air is inciden at angle of inciden 30∘ on one surface of slab in which refractive index varies with y. The light travels along the curve y=4x2 ( y and x are in meter) in the slab. Find out the refractive index of the slab at y=1/2m in the slab.

View SolutionTwo transparent slabs having equal thickness but different refractive indices μ1andμ2, are pasted side by side to form a composite slab. This slab is placed just after the double slit in a Young's experiment so that the light from one slit goes through one material and the light from the other slit goes through the other material. What should be the minimum thickness of the slab so that there is a minimum at the point P0 which is equidistant from the slits ?
View Solution
