A charge +Q is uniformly distributed in a spherical volume of radius R. A particle of charge +q and mass m projected with velocity v0 the surface of the sherical volume to its centre inside a smooth tunnel dug across the sphere. The minimum value of v0 such tht it just reaches the centre (assume that thee is no resistance on the particle except electrostatic force) of he sphericle volume is
√Qq2πε0mR
√Qqπε0mR
√2Qqπε0mR
√Qq4πε0mR
The correct Answer is:D
To solve the problem of finding the minimum velocity v0 required for a particle of charge +q and mass m to reach the center of a spherical volume with a uniformly distributed charge +Q, we can use the principle of conservation of mechanical energy. Here’s a step-by-step solution:
Step 1: Understand the Initial and Final States
- The particle is projected from the surface of the sphere with velocity v0.
- The initial kinetic energy (KE) of the particle is given by:
KEinitial=12mv20
- The initial potential energy (PE) when the particle is at the surface of the sphere is given by:
PEinitial=kQqR
where k=14πϵ0.
Step 2: Determine the Final State
- At the center of the sphere, we want to find the potential energy (PE) and kinetic energy (KE).
- The potential energy at the center of the sphere is given by:
PEfinal=kQq⋅(3R)=3kQqR
- If the particle just reaches the center, we can assume that its final kinetic energy is zero (for minimum v0):
KEfinal=0
Step 3: Apply Conservation of Mechanical Energy
Using the conservation of mechanical energy, we can equate the total initial energy to the total final energy:
KEinitial+PEinitial=KEfinal+PEfinal
Substituting the expressions we have:
12mv20+kQqR=0+3kQqR
Step 4: Rearranging the Equation
Rearranging the equation gives:
12mv20=3kQqR−kQqR
12mv20=2kQqR
Step 5: Solve for v0
Now, we can solve for v20:
mv20=4kQqR
v20=4kQqmR
Taking the square root gives:
v0=√4kQqmR
Step 6: Substitute k
Substituting k=14πϵ0:
v0=
⎷4⋅14πϵ0⋅QqmR=√Qqπϵ0mR
Final Answer
Thus, the minimum value of v0 required for the particle to just reach the center of the spherical volume is:
v0=√Qqπϵ0mR
- The particle is projected from the surface of the sphere with velocity v0.
- The initial kinetic energy (KE) of the particle is given by:
KEinitial=12mv20
- The initial potential energy (PE) when the particle is at the surface of the sphere is given by:
PEinitial=kQqR
where k=14πϵ0.
- At the center of the sphere, we want to find the potential energy (PE) and kinetic energy (KE).
- The potential energy at the center of the sphere is given by:
PEfinal=kQq⋅(3R)=3kQqR
- If the particle just reaches the center, we can assume that its final kinetic energy is zero (for minimum v0):
KEfinal=0
Using the conservation of mechanical energy, we can equate the total initial energy to the total final energy:
KEinitial+PEinitial=KEfinal+PEfinal
Substituting the expressions we have:
12mv20+kQqR=0+3kQqR
Rearranging the equation gives:
12mv20=3kQqR−kQqR
12mv20=2kQqR
Now, we can solve for v20:
mv20=4kQqR
v20=4kQqmR
Taking the square root gives:
v0=√4kQqmR
Substituting k=14πϵ0:
v0=
⎷4⋅14πϵ0⋅QqmR=√Qqπϵ0mR
Thus, the minimum value of v0 required for the particle to just reach the center of the spherical volume is:
v0=√Qqπϵ0mR
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.14videos
ELECTROSTATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.29videos
ELECTROSTATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Example Type 83videos
ELECTROSTATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises50videos
ELECTROSTATIC POTENTIAL AND CAPACITORS
- BOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions135videos
GRAVITATION
Similar Questions
A positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring.
 View Solution
View SolutionA positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring .The
 View Solution
View SolutionCharge q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
View SolutionA charge +Q is uniformly distributed over a thin ring with radius R. A negative point charge −Q and mass m starts from rest at a point far away from the centre of the ring and moves towards the centre. Find the velocity of this particle at the moment it passes through the centre of the ring .
View SolutionA charge Q is filled in a spherical volume of Radius R . Use the concept of energy density , find energy stored inside the charge volume.
View SolutionA charge q is uniformly distributed over a quarter circular ring of radius r. The magnitude of electric field strength at the centre of the ring will be
View SolutionA positive charge Q is uniformly distributed throughout the volume of a dielectric sphere of radius R. A point mass having charge +q and mass m is fired toward the center of the sphere with velocity v from a point at distance r(r>R) from the center of the sphere. Find the minimum velocity v so that it can penetrate (R/2) distance of the sphere. Neglect any resistance other than electric interaction. Charge on the small mass remains constant throughout the motion.
 View Solution
View SolutionA unit positive point charge of mass m is projected with a velocity V inside the tunnel as shown. The tunnel has been made inside a uniformly charged nonconducting sphere. The minimum velocity with which the point charge should be projected such that it can reach the opposite end of the tunnel is equal to
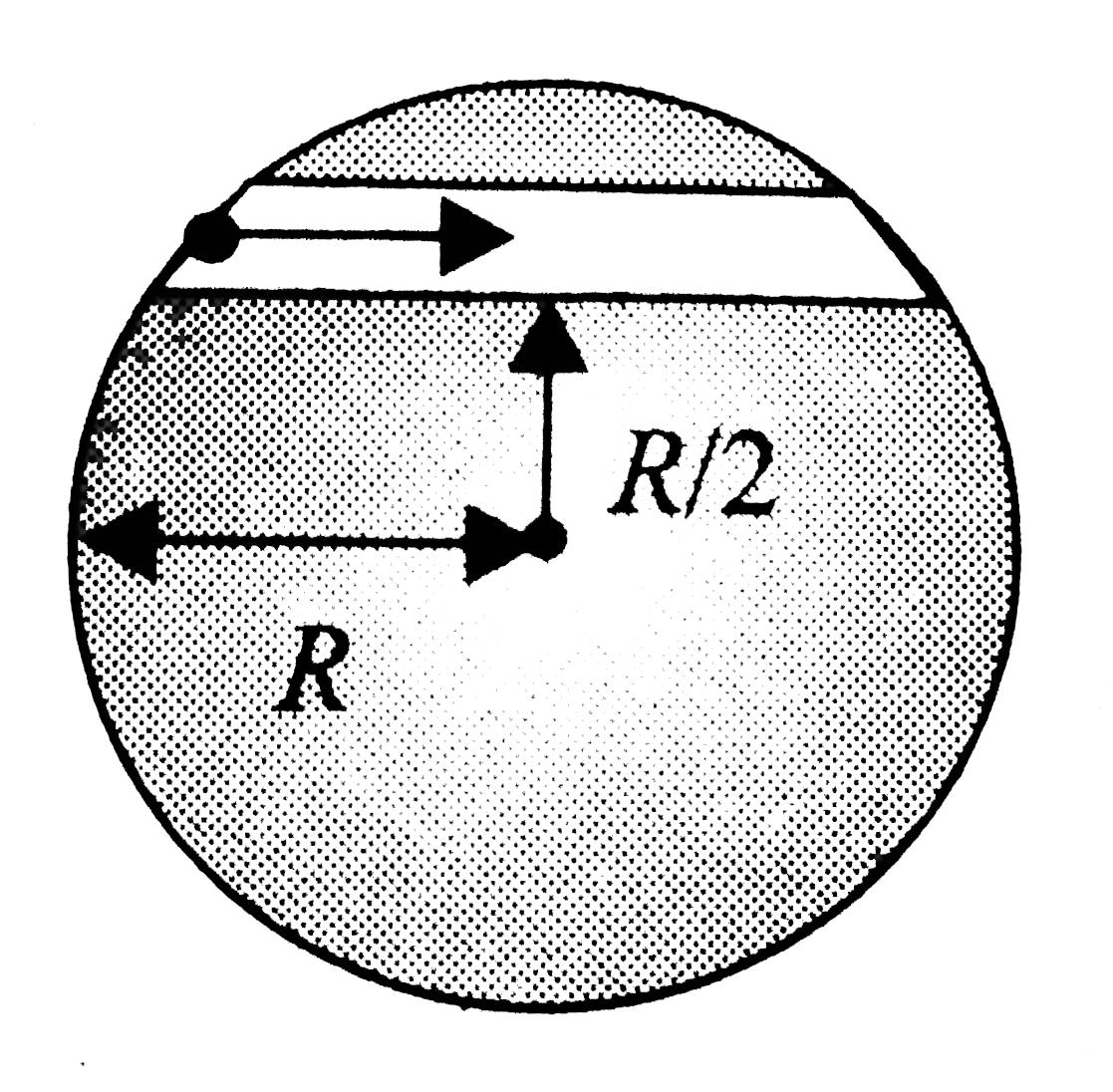 View Solution
View SolutionA charge q0 is distributed uniformly on a ring of radius R. A sphere of equal radius R constructed with its centre on the circumference of the ring. Find the electric flux through the surface of the sphere.
View SolutionA bullet of mass m and charge q is fired towards a solid uniformly charge sphere of radius R and total charge +q. If it strikes the surface of sphere with speed u, find the minimum value of u so that it can penetrate through the sphere. (Neglect all resistance force or friction acting on bullet except electrostatic forces)
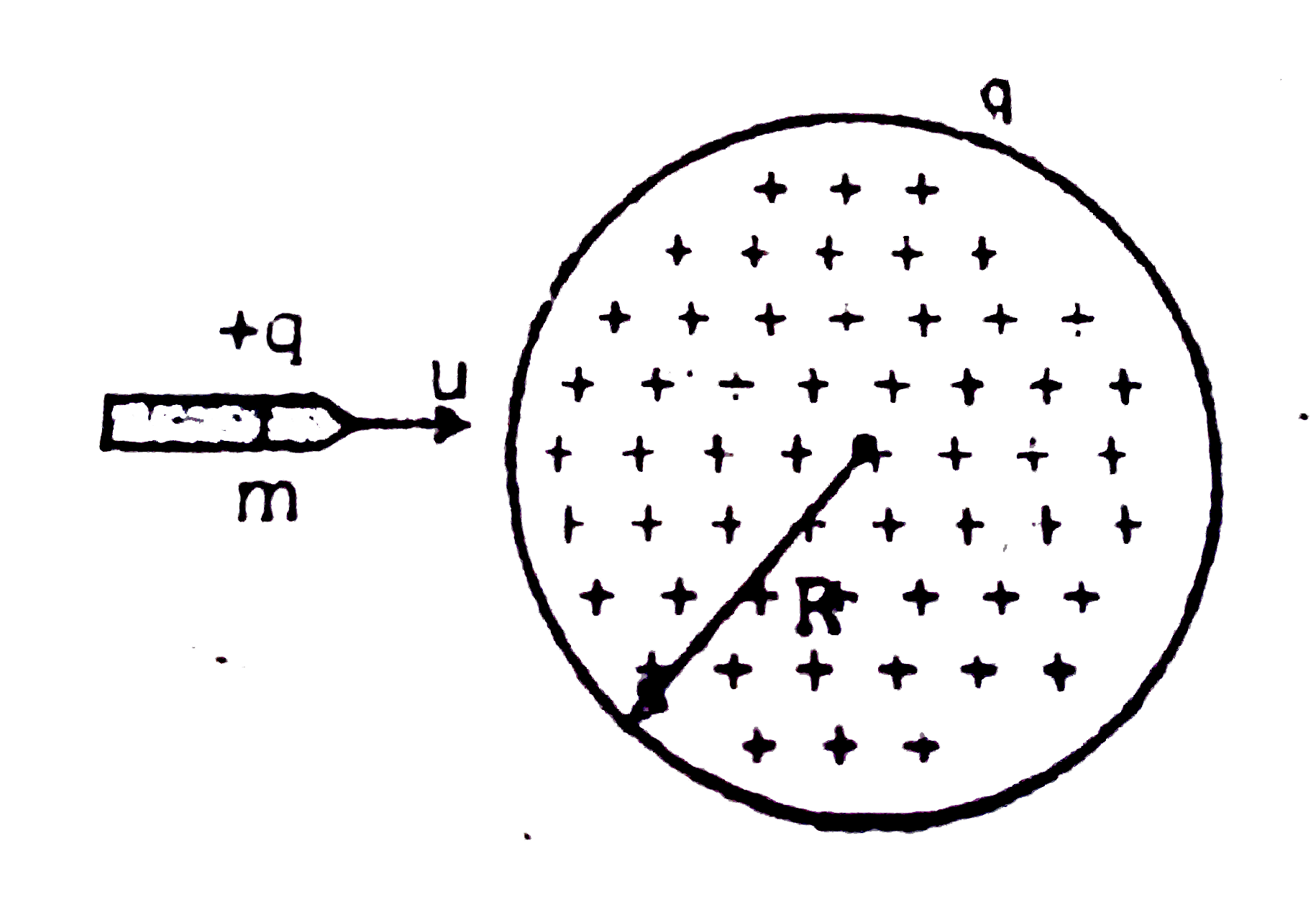 View Solution
View Solution

