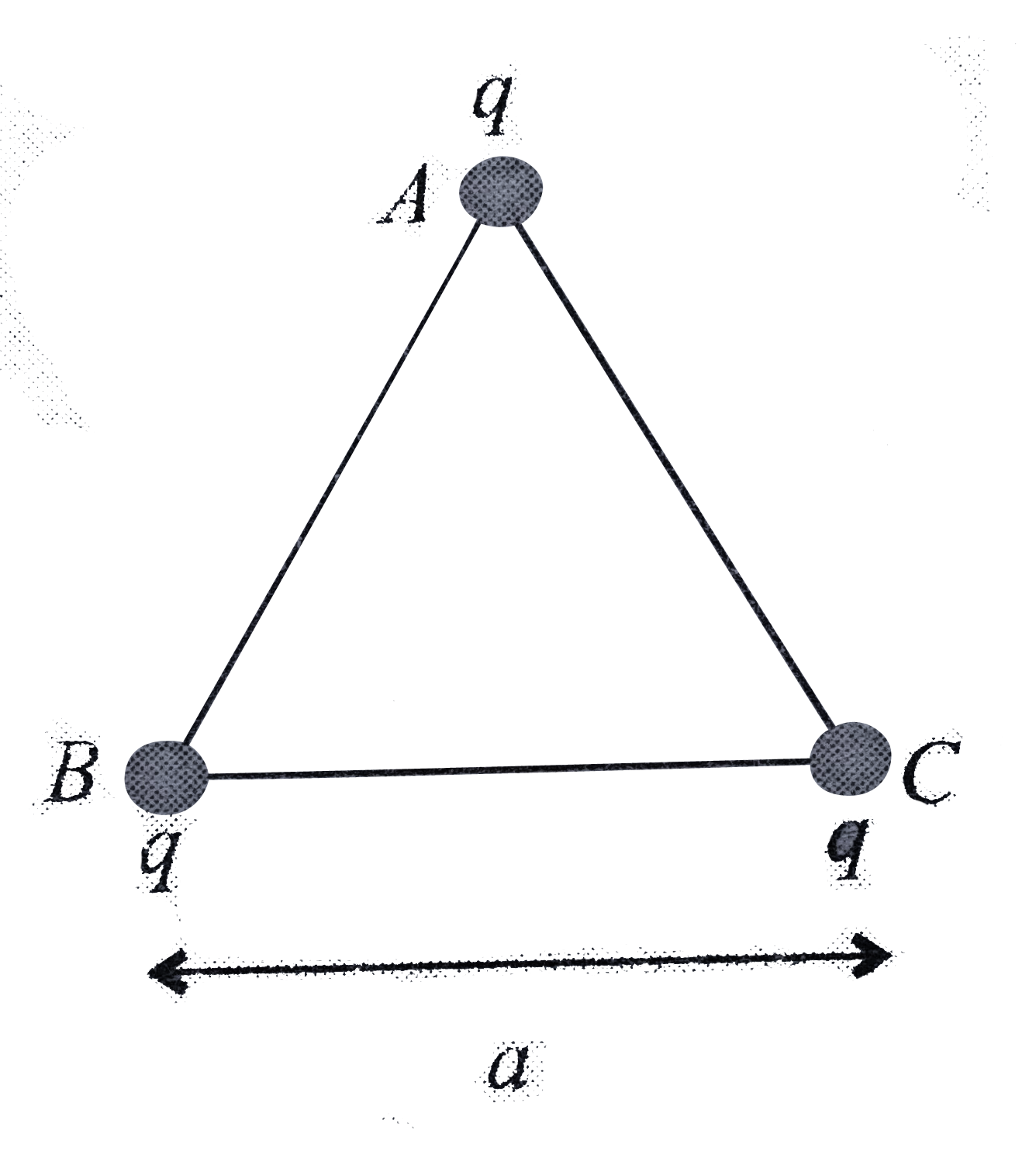
Two identical charges are placed at the two corners of an equilateral triangle. The potential energy of the system is U. The work done in bringing an identical charge from infinity to the third vertex is
U
2U
3U
4U
The correct Answer is:B
To solve the problem step by step, we will analyze the situation involving the potential energy of the system of charges.
Step 1: Understand the Configuration
We have two identical charges, let's say each charge is Q, placed at two corners of an equilateral triangle. The distance between these two charges is a.
Step 2: Calculate Initial Potential Energy
The potential energy U of the system with just the two charges at points A and B can be calculated using the formula for the potential energy between two point charges:
U=kQ2a
where k is Coulomb's constant.
Step 3: Bringing the Third Charge
Now, we want to bring a third identical charge Q from infinity to the third vertex C of the triangle.
Step 4: Calculate Final Potential Energy
When the third charge is brought to vertex C, we need to consider the potential energy contributions from all pairs of charges:
1. Between charge at A and C:
UAC=kQ2a
2. Between charge at B and C:
UBC=kQ2a
3. Between charges at A and B (already calculated):
UAB=kQ2a
Now, the total final potential energy Uf of the system when the third charge is at C is:
Uf=UAB+UAC+UBC=kQ2a+kQ2a+kQ2a=3⋅kQ2a=3U
Step 5: Calculate Work Done
The work done W in bringing the charge from infinity to point C is equal to the change in potential energy of the system:
W=Uf−U=3U−U=2U
Final Answer
Thus, the work done in bringing the identical charge from infinity to the third vertex is:
W=2U
---
We have two identical charges, let's say each charge is Q, placed at two corners of an equilateral triangle. The distance between these two charges is a.
The potential energy U of the system with just the two charges at points A and B can be calculated using the formula for the potential energy between two point charges:
U=kQ2a
where k is Coulomb's constant.
Now, we want to bring a third identical charge Q from infinity to the third vertex C of the triangle.
When the third charge is brought to vertex C, we need to consider the potential energy contributions from all pairs of charges:
1. Between charge at A and C:
UAC=kQ2a
2. Between charge at B and C:
UBC=kQ2a
3. Between charges at A and B (already calculated):
UAB=kQ2a
Uf=UAB+UAC+UBC=kQ2a+kQ2a+kQ2a=3⋅kQ2a=3U
The work done W in bringing the charge from infinity to point C is equal to the change in potential energy of the system:
W=Uf−U=3U−U=2U
Thus, the work done in bringing the identical charge from infinity to the third vertex is:
W=2U
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.14videos
ELECTROSTATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.29videos
ELECTROSTATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Example Type 83videos
ELECTROSTATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises50videos
ELECTROSTATIC POTENTIAL AND CAPACITORS
- BOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions135videos
GRAVITATION
Similar Questions
Four identical point charges q are placed at four corners of a square of side a. Find the potential energy of the charge system.
 .View Solution
.View SolutionFour identical charges q are placed at the corners of a square of side a. Find the potential energy of one of the charges due to the remaining charges.
 .View Solution
.View Solution
Knowledge Check
- Question 1 - Select One
In the question number 4, work done in bringing a charge of 4×10−9 C form infinity to that point is
A2.4×10−4JB1.8×10−4JC3.2×10−5JD4.1×10−5J - Question 2 - Select One
Electric charges q,q,–2q are placed at the corners of an equilateral triangle ABC of side l. The magnitude of electric dipole moment of the system is
AqlB√3qlCzeroD4ql
Two equal charges q are placed at a distance of 2a and a third charge −2q is placed at the midpoint. The potential energy of the system is
View SolutionThree identical charges are placed at the vertices of an equilateral triangle. The force experienced by each charge, (if k=14πε0) is
View SolutionSix equal point charges q each are placed at six corners of a hexago of side a. Find out potential energy of charge system
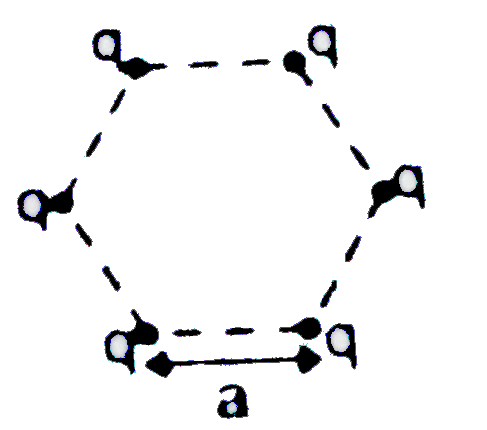 View Solution
View SolutionIf 3 charges are placed at the vertices of equilateral triangle of charge 'q' each. What is the net potential energy, if the side of equilateral Δ is lcm ?
View SolutionElectric charges q,q,–2q are placed at the corners of an equilateral triangle ABC of side l. The magnitude of electric dipole moment of the system is
View SolutionThree equal charges q are placed at the corners of an equilateral triangle of side A.
(i) Find out potential energy of charge system.
(ii) Calculate work required to decrease the side of triangle to a/2.
(iii) If the charges are released from the shown position and each of them has same mass m, then find the speed of each particle when they lie on triangle of side 2a.
 .View Solution
.View Solution

