A sphere of charges of radius R carries a positive charge whose volume chasrge density depends only on the distance r from the ball's centre as ρ=ρ0(1−rR), where ρ0 is constant. Assume epsilon as theh permittivity of space.
The magnitude of electric field as a function of the distance r inside the sphere is given by
E=ρ0ε[r3−r24R]
E=ρ0ε[r4−r33R]
E=ρ0ε[r3+r2(4R)]
E=ρ0ε[r4+r23R]
The correct Answer is:A
To find the electric field inside a sphere with a varying charge density, we can follow these steps:
Step 1: Understand the charge density
The volume charge density is given by:
ρ(r)=ρ0(1−rR)
where ρ0 is a constant, r is the distance from the center of the sphere, and R is the radius of the sphere.
Step 2: Use Gauss's Law
To find the electric field E at a distance r from the center, we apply Gauss's Law:
ΦE=Qencϵ0
where ΦE is the electric flux through a Gaussian surface of radius r and Qenc is the charge enclosed within that surface.
Step 3: Calculate the electric flux
The electric flux through the Gaussian surface is given by:
ΦE=E⋅4πr2
Thus, we have:
E⋅4πr2=Qencϵ0
Step 4: Determine the enclosed charge Qenc
To find Qenc, we need to integrate the charge density over the volume of the sphere up to radius r:
Qenc=∫r0ρ(r′)dV
The differential volume element dV in spherical coordinates is:
dV=4πr′2dr′
Substituting the charge density:
Qenc=∫r0ρ0(1−r′R)4πr′2dr′
Step 5: Perform the integration
Now, we can expand and integrate:
Qenc=4πρ0∫r0(r′2−r′3R)dr′
Calculating the integrals separately:
∫r0r′2dr′=r33,and∫r0r′3dr′=r44
So,
Qenc=4πρ0(r33−r44R)
Step 6: Substitute Qenc back into Gauss's Law
Now substitute Qenc into Gauss's Law:
E⋅4πr2=4πρ0(r33−r44R)ϵ0
Cancelling 4π from both sides:
Er2=ρ0ϵ0(r33−r44R)
Step 7: Solve for E
Finally, we can solve for E:
E=ρ0ϵ0(r3−r24R)
Final Result
The magnitude of the electric field as a function of the distance r inside the sphere is:
E(r)=ρ0ϵ0(r3−r24R)
The volume charge density is given by:
ρ(r)=ρ0(1−rR)
where ρ0 is a constant, r is the distance from the center of the sphere, and R is the radius of the sphere.
To find the electric field E at a distance r from the center, we apply Gauss's Law:
ΦE=Qencϵ0
where ΦE is the electric flux through a Gaussian surface of radius r and Qenc is the charge enclosed within that surface.
The electric flux through the Gaussian surface is given by:
ΦE=E⋅4πr2
Thus, we have:
E⋅4πr2=Qencϵ0
To find Qenc, we need to integrate the charge density over the volume of the sphere up to radius r:
Qenc=∫r0ρ(r′)dV
The differential volume element dV in spherical coordinates is:
dV=4πr′2dr′
Substituting the charge density:
Qenc=∫r0ρ0(1−r′R)4πr′2dr′
Now, we can expand and integrate:
Qenc=4πρ0∫r0(r′2−r′3R)dr′
Calculating the integrals separately:
∫r0r′2dr′=r33,and∫r0r′3dr′=r44
So,
Qenc=4πρ0(r33−r44R)
Now substitute Qenc into Gauss's Law:
E⋅4πr2=4πρ0(r33−r44R)ϵ0
Cancelling 4π from both sides:
Er2=ρ0ϵ0(r33−r44R)
Finally, we can solve for E:
E=ρ0ϵ0(r3−r24R)
The magnitude of the electric field as a function of the distance r inside the sphere is:
E(r)=ρ0ϵ0(r3−r24R)
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.1
4 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.2
9 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Example Type 8
3 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
A sphere of charges of radius R carries a positive charge whose volume charge density depends only on the distance r from the ball's centre as ρ=ρ0(1−rR), whereρ0 is constant. Assume epsilon as theh permittivity of space.
The magnitude of the electric field as a functiion of the distance r outside the balll is given by
View SolutionA sphere of charges of radius R carries a positive charge whose volume chasrge density depends only on the distance r from the ball's centre as ρ=ρ0(1−rR), where ρ0 is constant. Assume epsilon as theh permittivity of space.
the maximum electric field intensity is
View SolutionA sphere of charges of radius R carries a positive charge whose volume chasrge density depends only on the distance r from the ball's centre as ρ=ρ0(1−rR), where ρ0 is constant. Assume epsilon as theh permittivity of space.
The value of distance rm at which electric field intensity is maximum is given by
View SolutionA ball of radius R carries a positive charge throughout its volume, such that the volume density of charge depends on distance r from the ball's centre as ρ=ρ0(1−r®), where ρ0 is a constant. Assuming the permittivity of ball to be one , find magnitude of electric field as a function of distance r, both inside and outside the ball.
Strategy : The field has a spherical symmetry . For a point outside the ball (r>R)
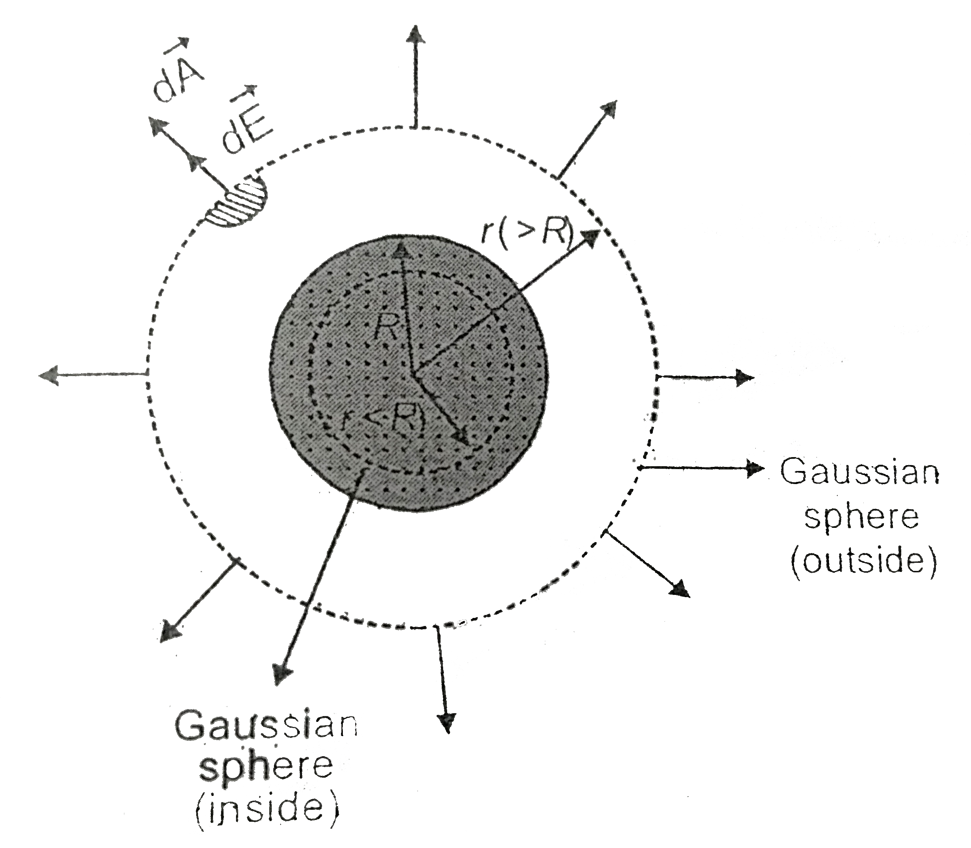
ϕ=∮→E.¯¯¯¯¯dA=E×4πr2
By Gauss law , ϕ=Qε0
⇒Eout=Q4πε0r2, where Q is total charge
For a point inside the ball (r<R)
ϕ=∮→E.¯¯¯¯¯dA=E×4πr2
By Gauss law, ϕ=qencε0
⇒Ein=qenc4πε0r2
where qenc= charge in a sphere of radius r(<R)
To find the enclosed charge , consider a spherical shell of radius r and thickness dr
Its volume dV=4πr2dr
Charge dq=ρdV
⇒dq=ρ0(1−rR)4πr2dr
⇒q=∫r0ρ0(1−rR)4πr2dr
=ρ04π[∫r0r2dr−∫r0r3Rdr]=ρ04π[r33−r44R]

View SolutionA sphere of radius R carries charge such that its volume charge density is proportional to the square of the distance from the centre. What is the ratio of the magnitude of the electric field at a distance 2 R from the centre to the magnitude of the electric field at a distance of R/2 from the centre?
View SolutionA ball of radius R carries a positive charges whose volume density at a point is given as ρ=ρ0(1−r/R), Where ρ0 is a constant and r is the distance of the point from the center. Assume the permittivities of the ball and the enviroment to be equal to unity.
(a) Find the magnitude of the electric field strength as a function of the distance r both inside and outside the ball.
(b) Find the maximum intensity Emax and the corresponding distance rm.
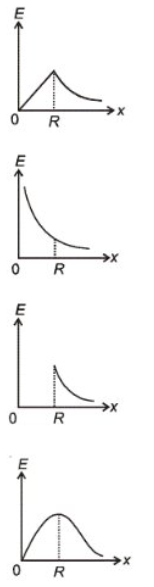
View SolutionA non-conducting solid sphere of radius R, has a uniform charge density. The graph representing variation of magnitude of electric field (E) as a function of distance (x) from the centre of the sphere is
View SolutionA hallow metal sphere of radius R is uniformly charged. The electric field due to the sphere at a distance r from the centre:
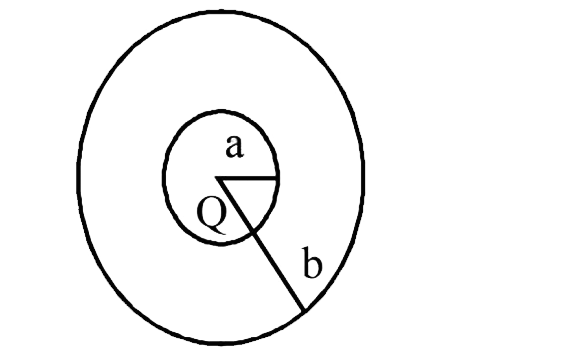
View SolutionThe region between two concentric spheres of radii 'a' and 'b', respectively (see figure), have volume charge density ρ=Ar, where A is a constant and r is the distance from the centre. At the centre of the spheres is a point charge Q. The value of A such that the electric field in the region between the spheres will be constant, is:
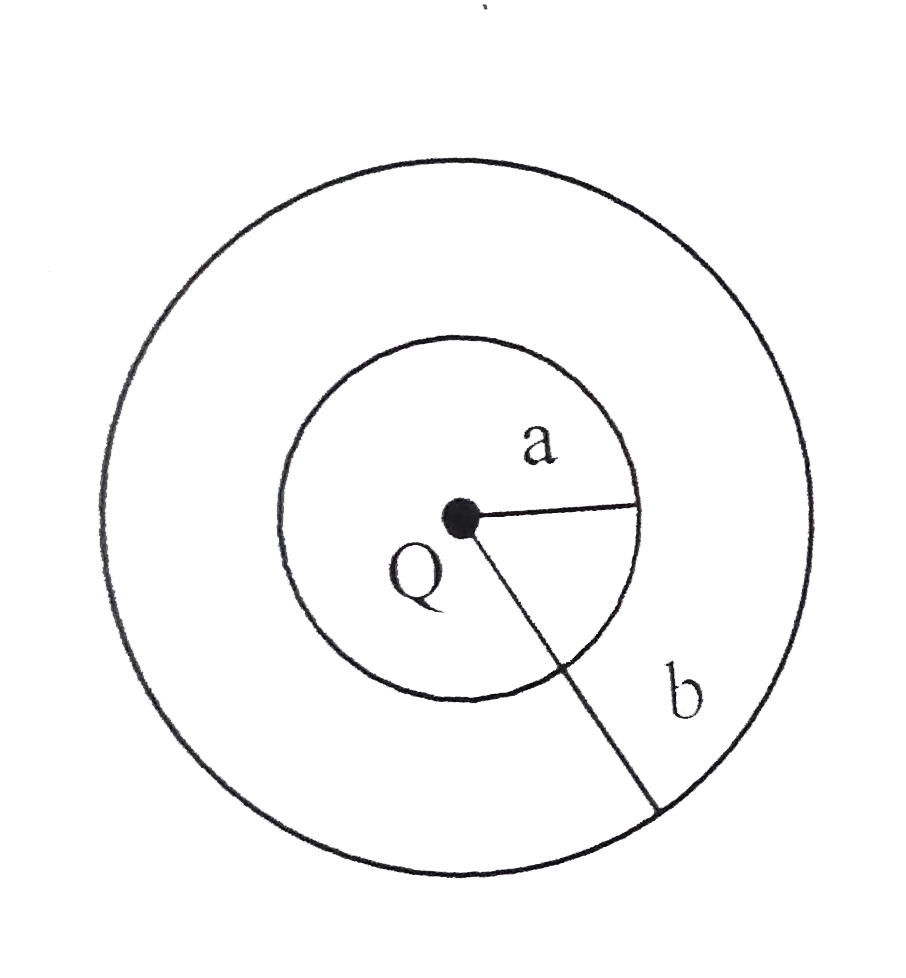
View SolutionThe region between two concentric spheres of radii ‘a’ and ‘b’, respectively (see figure), has volume charge density p=Ar, where A is a constant and r is the distance from the centre. At the centre of the spheres is a point charge Q. The value of A such that the electric field in the region between the spheres will be constant, is :
View Solution
