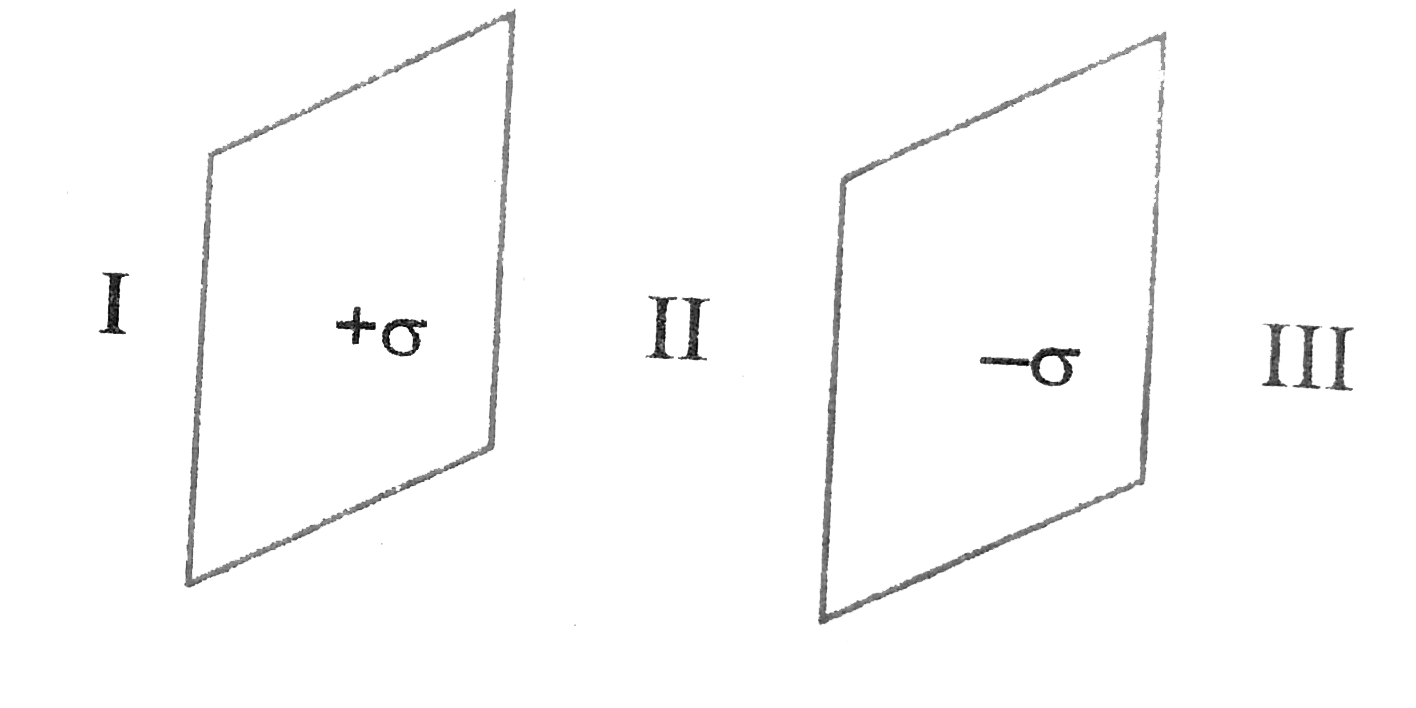
Two large, thin metal plates are parallel and close to each other. On their faces, the plates have surfaces charge densities of opposite sign and of magnitude coulomb per square metre. What is electric field
(i) to the left of the plates ?
(ii) to the right of the plates ?
(iii) in between the plates ?
To solve the problem step by step, we will analyze the electric field produced by two large, thin parallel plates with surface charge densities of opposite signs.
Given:
- Surface charge density, σ=0.177×10−11C/m2
- The plates are oppositely charged.
Step 1: Understanding the Electric Field due to a Single Plate
The electric field E due to a single infinite plane sheet with surface charge density σ is given by the formula:
E=σ2ϵ0
where ϵ0 is the permittivity of free space, approximately 8.85×10−12C2/N m2.
Step 2: Electric Field to the Left of the Plates
1. Consider a point to the left of the plates (Point A):
- The electric field due to the positively charged plate (let's denote it as Plate 1) will point away from the plate.
- The electric field due to the negatively charged plate (Plate 2) will point towards the plate.
Since both fields are in opposite directions and equal in magnitude:
Eleft=EPlate 1+EPlate 2=σ2ϵ0−σ2ϵ0=0
Step 3: Electric Field to the Right of the Plates
2. Consider a point to the right of the plates (Point B):
- The electric field due to Plate 1 points away from it (to the right).
- The electric field due to Plate 2 points towards it (also to the right).
Since both fields are in the same direction:
Eright=EPlate 1+EPlate 2=σ2ϵ0+σ2ϵ0=σϵ0
Step 4: Electric Field Between the Plates
3. Consider a point between the plates (Point C):
- The electric field due to Plate 1 points away from it (to the right).
- The electric field due to Plate 2 points towards it (to the left).
Since both fields are in opposite directions and equal in magnitude:
Ebetween=EPlate 1−EPlate 2=σ2ϵ0+σ2ϵ0=σϵ0
Step 5: Substituting the Values
Now, we can substitute σ and ϵ0 into the equations to find the electric fields.
For Points A and B (Left and Right):
- Eleft=0
- Eright=0.177×10−118.85×10−12
Calculating Eright:
Eright=0.177×10−118.85×10−12≈0.02N/C
For Point C (Between the Plates):
- Ebetween=0.177×10−118.85×10−12
Calculating Ebetween:
Ebetween=0.177×10−118.85×10−12≈0.02N/C
Final Results:
(i) Electric field to the left of the plates: E=0N/C
(ii) Electric field to the right of the plates: E≈0.02N/C
(iii) Electric field in between the plates: E≈0.02N/C
- Surface charge density, σ=0.177×10−11C/m2
- The plates are oppositely charged.
The electric field E due to a single infinite plane sheet with surface charge density σ is given by the formula:
E=σ2ϵ0
where ϵ0 is the permittivity of free space, approximately 8.85×10−12C2/N m2.
1. Consider a point to the left of the plates (Point A):
- The electric field due to the positively charged plate (let's denote it as Plate 1) will point away from the plate.
- The electric field due to the negatively charged plate (Plate 2) will point towards the plate.
Since both fields are in opposite directions and equal in magnitude:
Eleft=EPlate 1+EPlate 2=σ2ϵ0−σ2ϵ0=0
2. Consider a point to the right of the plates (Point B):
- The electric field due to Plate 1 points away from it (to the right).
- The electric field due to Plate 2 points towards it (also to the right).
Since both fields are in the same direction:
Eright=EPlate 1+EPlate 2=σ2ϵ0+σ2ϵ0=σϵ0
3. Consider a point between the plates (Point C):
- The electric field due to Plate 1 points away from it (to the right).
- The electric field due to Plate 2 points towards it (to the left).
Since both fields are in opposite directions and equal in magnitude:
Ebetween=EPlate 1−EPlate 2=σ2ϵ0+σ2ϵ0=σϵ0
Now, we can substitute σ and ϵ0 into the equations to find the electric fields.
- Eleft=0
- Eright=0.177×10−118.85×10−12
Eright=0.177×10−118.85×10−12≈0.02N/C
- Ebetween=0.177×10−118.85×10−12
Ebetween=0.177×10−118.85×10−12≈0.02N/C
(i) Electric field to the left of the plates: E=0N/C
Topper's Solved these Questions
- ELECTROSTATICSBook:DC PANDEY ENGLISHChapter:ELECTROSTATICSExercise:Example Type 5
Explore 5 Videos
- ELECTROSTATICSBook:DC PANDEY ENGLISHChapter:ELECTROSTATICSExercise:Example Type 6
Explore 4 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBook:DC PANDEY ENGLISHChapter:ELECTROSTATIC POTENTIAL AND CAPACITORSExercise:(C) Chapter exercises
Explore 50 Videos
- GRAVITATIONBook:DC PANDEY ENGLISHChapter:GRAVITATIONExercise:All Questions
Explore 135 Videos
Similar Questions
Two large, thin metal plates are parallel and close to each other. On their inner faces, the plates have surface charge densities of opposite signs and of magnitude 17.0×10−22C/m2. What is E : between the plates?
View SolutionTwo large, thin metal plates are parallel and close to each other. On their inner faces, the plates have surface charge densities of opposite signs and of magnitude 17.0×10−22C/m2. What is E : between the plates?
View Solution
Knowledge Check
Two large, thin plates are parallel and close to each other. On their inner faces, the plates have surface charge densities of opposite signs and of magnitude 16×10−22Cm−2. The electric field between the plates is
A1.8×10−10NC−1B1.9×10−10NC−1C1.6×10−10NC−1D1.5×10−10NC−1Two large parallel conducting plates are placed close to each other ,the inner surface of the two plates have surface charge densities +σ and −σ .The outer surfaces are without charge.The electric field has a magnitude of
A(sigma)/(epsi_(0)) in the region between the platesB''σε0 in the region between the platesC0Dnone of these
Two large thin metal plates are parallel and close to each other. On their inner faces, the plates have surface charge densities of opposite signs and magnitude 27×10−22Cm−2. The electric field →E in region II in between the plates is
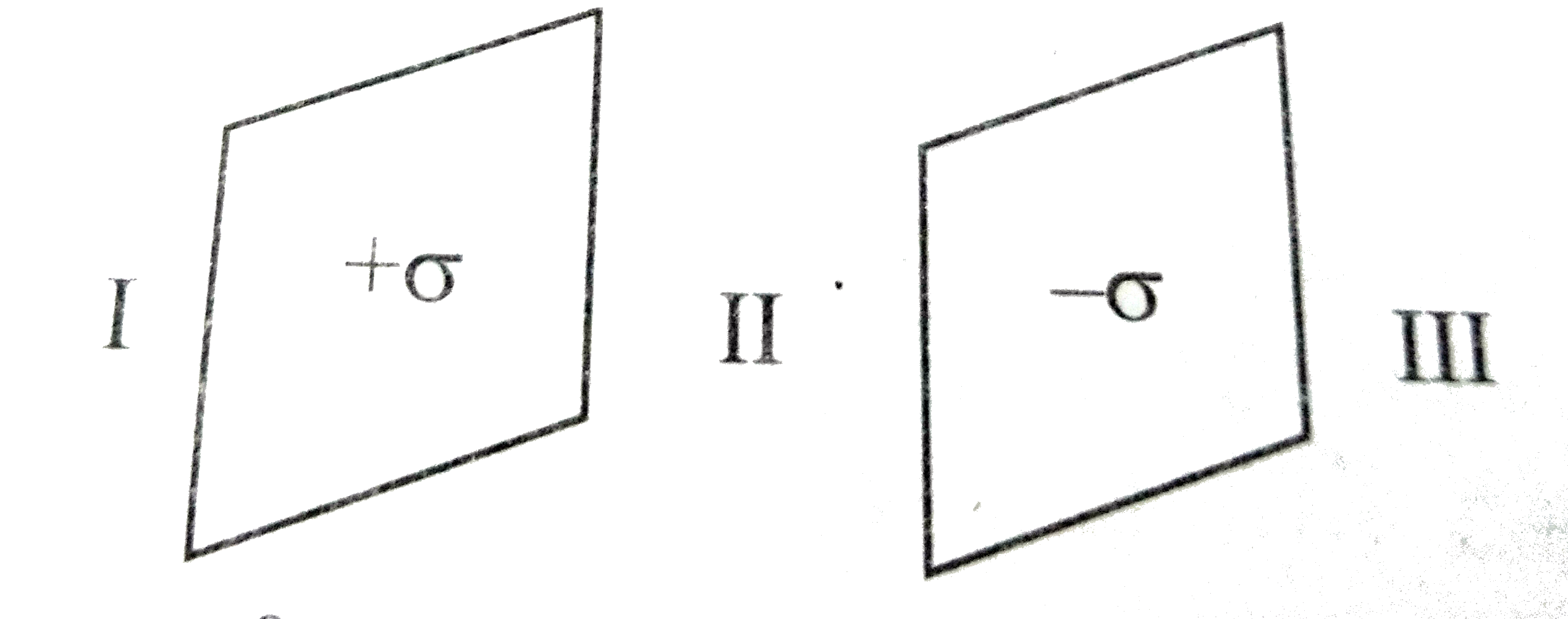
View SolutionTwo large this metal plates are parallel and close to each other. On their inner faces, the plates have surface charge densities of opposite signs and magnitude 17.0×10−22C/m2. What is →E : (a) In the outer region of the first plate. (b) In the outer region of the secound plate, and (c) Between the plates ? See Fig.
View SolutionTwo large conducting plates are placed parallel to each other nad they carry equal and opposite charges with surface density σ as shown in figure (30-E6). Find the electric field (a) at the left of the plates, (b) in between the plates and © at the right of the plates.

View SolutionTwo large conducting plates X and Y. each having large surface area A (on one side), are placed parallel to each other as shown in figure (30-E7). The plate X is given a charge Q whereas the other is neutral. Find (a) the surface charge density at the inner surface of the plates X, (b) the electric field at a point to the left of the plates, (c) the electric field at a point in between the plates and (d) the electric field at a point to the right of the plates.

View SolutionTwo parallel large thin metal sheets have equal surface charge densities (σ=26.4×10−12C/m2) of opposite signs. The electric field between these sheets is
View SolutionIf we introduce a large thin metal plate between two point charges, what will happen to the force between the charges?
View Solution