A machine gun is mounted on the top of a tower of height h. At what angle should the gun be inclined to cover a maximum range of firing on the ground below ? The muzzle speed of bullet is 150ms−1. Take g=10ms−2.
The correct Answer is:θ=sin−1√u22(u2+gh).
To solve the problem of determining the angle at which a machine gun should be inclined to cover the maximum range of firing from the top of a tower, we can follow these steps:
1. Identify the Given Data:
- Height of the tower, h (not specified in the transcript, but we will keep it as h).
- Muzzle speed of the bullet, v=150m/s.
- Acceleration due to gravity, g=10m/s2.
2. Set Up the Problem:
- When the gun is fired at an angle α from the horizontal, the initial velocity can be resolved into horizontal and vertical components:
- Horizontal component: vx=vcosα
- Vertical component: vy=vsinα
3. Write the Equation for Vertical Motion:
- The vertical displacement of the bullet when it hits the ground can be described by the equation:
−h=vyt−12gt2
- Substituting for vy:
−h=150sinα⋅t−5t2
- Rearranging gives:
5t2−150sinα⋅t−h=0
- This is a quadratic equation in t.
4. Solve for Time t:
- Using the quadratic formula t=−b±√b2−4ac2a:
t=150sinα±√(150sinα)2+20h10
- We will use the positive root since time cannot be negative:
t=150sinα+√22500sin2α+20h10
5. Calculate the Horizontal Range R:
- The horizontal range R is given by:
R=vx⋅t=150cosα⋅t
- Substituting t:
R=150cosα⋅(150sinα+√22500sin2α+20h10)
- Simplifying gives:
R=1500cosα(15sinα+√2250sin2α+2h)
6. Maximize the Range:
- To find the angle α that maximizes R, we differentiate R with respect to α and set the derivative to zero:
dRdα=0
- This involves applying the product and chain rules of differentiation.
7. Solve for α:
- After differentiating and simplifying, you will find that the optimal angle α for maximum range can be calculated, leading to:
α≈43.47∘
Conclusion:
The angle at which the machine gun should be inclined to cover the maximum range of firing on the ground below is approximately 43.47∘.
- Height of the tower, h (not specified in the transcript, but we will keep it as h).
- Muzzle speed of the bullet, v=150m/s.
- Acceleration due to gravity, g=10m/s2.
- When the gun is fired at an angle α from the horizontal, the initial velocity can be resolved into horizontal and vertical components:
- Horizontal component: vx=vcosα
- Vertical component: vy=vsinα
- The vertical displacement of the bullet when it hits the ground can be described by the equation:
−h=vyt−12gt2
- Substituting for vy:
−h=150sinα⋅t−5t2
- Rearranging gives:
5t2−150sinα⋅t−h=0
- This is a quadratic equation in t.
- Using the quadratic formula t=−b±√b2−4ac2a:
t=150sinα±√(150sinα)2+20h10
- We will use the positive root since time cannot be negative:
t=150sinα+√22500sin2α+20h10
- The horizontal range R is given by:
R=vx⋅t=150cosα⋅t
- Substituting t:
R=150cosα⋅(150sinα+√22500sin2α+20h10)
- Simplifying gives:
R=1500cosα(15sinα+√2250sin2α+2h)
- To find the angle α that maximizes R, we differentiate R with respect to α and set the derivative to zero:
dRdα=0
- This involves applying the product and chain rules of differentiation.
- After differentiating and simplifying, you will find that the optimal angle α for maximum range can be calculated, leading to:
α≈43.47∘
The angle at which the machine gun should be inclined to cover the maximum range of firing on the ground below is approximately 43.47∘.
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Single Correct
76 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Multiple Correct
11 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.4
11 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
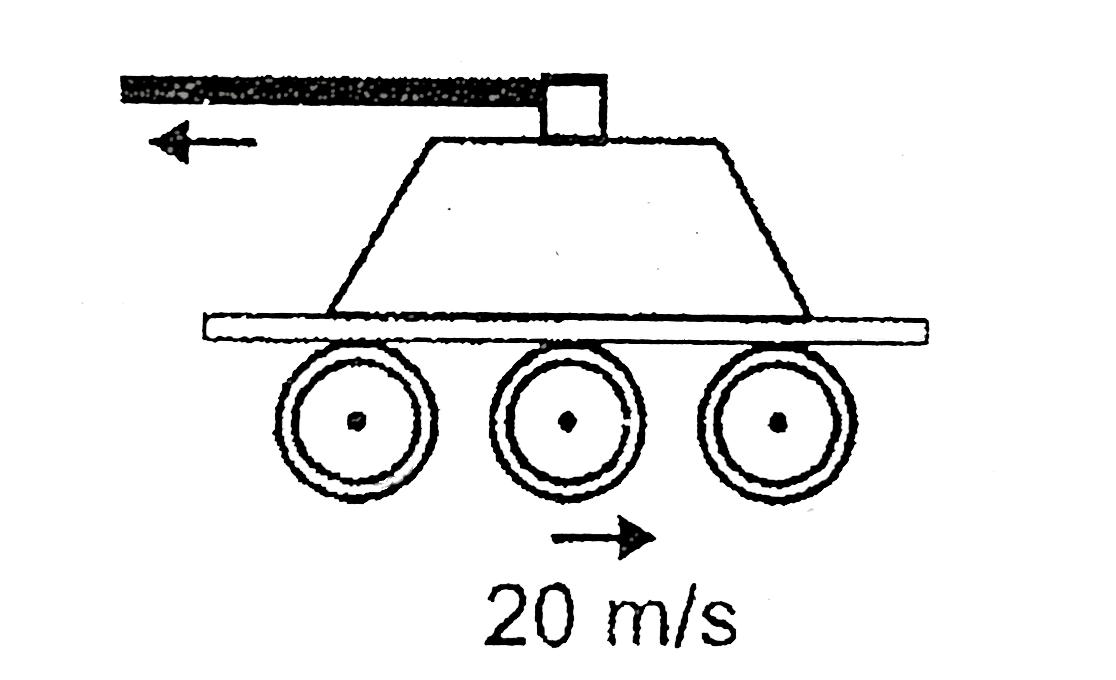
A machine gun is mounted on an armored car moving with a speed of 20ms−1. The gun point against the direction of motion of car. The muzzle speed of bullet is equal to speed of sound in air i.e., 340ms−1. The time difference between bullet actually reaching and sound of firing reaching at a target 544m away from car at the instant of firing is reaching at a target 544m away from car at the instant of firing is
View SolutionA stone is dropped from the top of a tower of height h. Aftre 1s another stone is droppped from the balcony 20m below the top. Both reach the bottom simultaneously. What is the value of h ? Take g=10ms−2.
View SolutionA machine gun fires a bullet of mass 40 g with a velocity 1200ms−1. The man holding it can exert a maximum force of 144 N on the gun. How many bullets can be fire per second at the most?
View SolutionA machine gun fires a bullet of mass 40 g with a velocity 1200ms−1. The man holding it can exert a maximum force of 144 N on the gun. How many bullets can be fire per second at the most?
View SolutionA gun mounted on the top of a moving truck t is aimed in the backward direction at an angle of 30° to the vertical. If the muzzle velocity of the bullet is 4ms−1 the value of speed of the truck that will make the bullet come of out vertically is
View SolutionA bomber plane moving at a horizontal speed of 20m/s releases a bomb at a height of 80m above ground as shown. At the same instant a Hunter of negligible height starts running from a point below it, to catch the bomb with speed 10m/s. After two seconds he relized that he cannot make it, he stops running and immediately hold his gun and fires in such direction so that just before bomb hits the ground, bullet will hit it. What should be the firing speed of bullet
(Take g=10m/s2)

View SolutionThe range of a rifle bullet on level ground is 60 m. The range (in m) upon incline of 30∘ is . (Take g=10m/s2)
View SolutionA stone dropped from the top of a tower of height 300 m high splashes into the water of a pond near the base of the tower. When is the splash heard at the top ? Given that the speed of sound in air is 340ms−1?(g=9.8ms−2).
View SolutionA bullet is projected upwards from the top of a tower of height 90 m with the velocity 30ms−1 making an angle 30∘ with the horizontal. Find the time taken by it to reach the ground is (g=10ms−2)
View SolutionA boy standing at the top of a tower of 20m of height drops a stone. Assuming g=10ms−2, the velocity with which it hits the ground is :-
View Solution
