Question

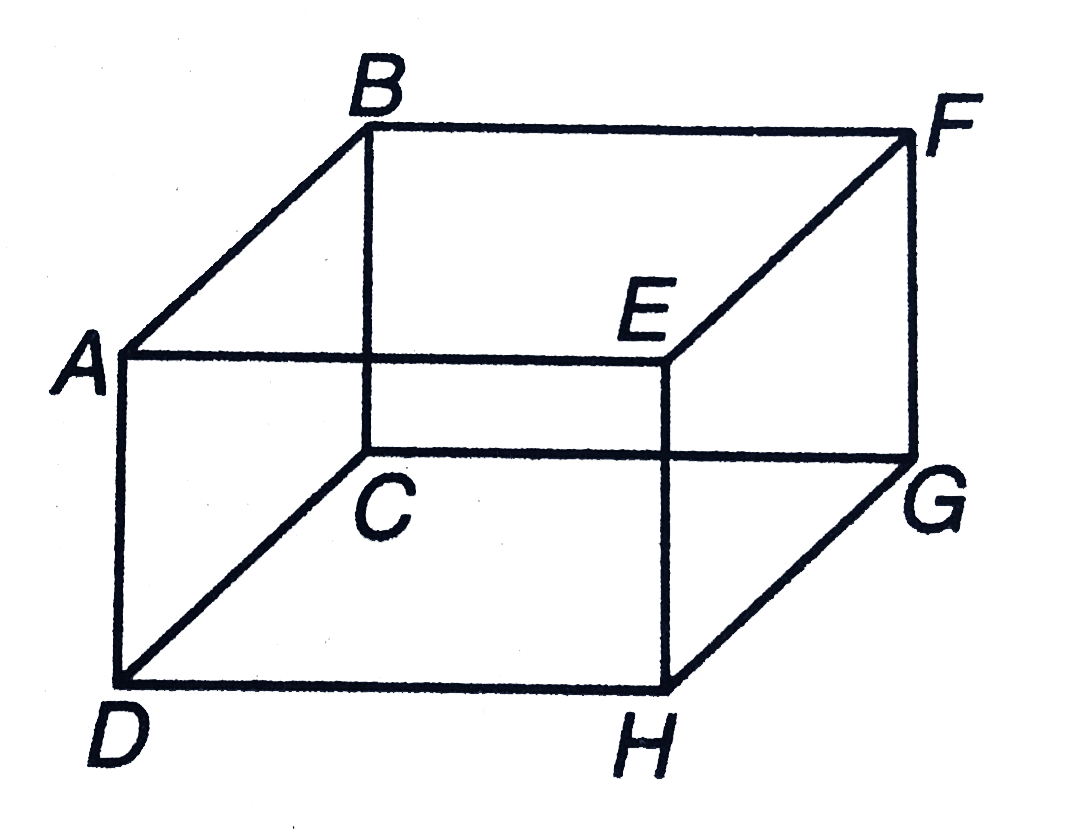
A small spherically symmetrica charge q is placed at one vertex of a cube as shown. The flux through the faces ABCD, and HGEF are, respectively.

Options

A. `(q)/( 24epsilon_(0)),(q)/(24epsilon_(0))`
B. `0, ( q)/( 8 epsilon_(0))`
C. `(q)/( 8 epsilon_(0)) , 0`
D. `(q)/( 24 epsilon_(0)),0`

Answer

4

Explanation

To solve the problem of finding the electric flux through the faces ABCD and HGEF of a cube with a charge $$q$$ placed at one of its vertices, we can follow these steps:

Step 1: Understand the Geometry
The charge \( q \) is located at one vertex of the cube. The cube has 8 vertices, and since the charge is at one vertex, it will influence the flux through the faces that are adjacent to this vertex.

Step 2: Use Gauss's Law
According to Gauss's Law, the electric flux \( \Phi \) through a closed surface is given by:
\( \Phi = \frac{Q_{\text{enclosed}}}{\epsilon_0} \)
where \( Q_{\text{enclosed}} \) is the total charge enclosed by the surface and \( \epsilon_0 \) is the permittivity of free space.

Step 3: Determine the Enclosed Charge
Since the charge \( q \) is at the vertex of the cube, it is only partially enclosed by the cube. In fact, only \( \frac{1}{8} \) of the charge \( q \) is effectively enclosed by the cube because the cube can be thought of as being one of 8 identical cubes that could be formed around the charge at the vertex.

Thus, the enclosed charge \( Q_{\text{enclosed}} \) for the cube is:
\( Q_{\text{enclosed}} = \frac{q}{8} \)

Step 4: Calculate the Total Flux Through the Cube
Using Gauss's Law, the total electric flux through the entire cube is:
\( \Phi_{\text{total}} = \frac{Q_{\text{enclosed}}}{\epsilon_0} = \frac{q/8}{\epsilon_0} = \frac{q}{8\epsilon_0} \)

Step 5: Distribute the Flux Among the Faces
The total flux through the cube will be distributed among the three faces that are adjacent to the vertex where the charge is located (faces ABCD, ABFE, and ADEF). Since the charge is symmetrically placed at the vertex, the flux through these three faces will be equal.

Thus, the flux through each of the three adjacent faces is:
\( \Phi_{\text{face}} = \frac{\Phi_{\text{total}}}{3} = \frac{q/(8\epsilon_0)}{3} = \frac{q}{24\epsilon_0} \)

Step 6: Determine the Flux Through HGEF
The face HGEF is opposite to the vertex where the charge is located. Since there is no charge enclosed by this face, the electric flux through HGEF is:
\( \Phi_{\text{HGEF}} = 0 \)

Final Summary
- The flux through the face ABCD is \( \frac{q}{24\epsilon_0} \).
- The flux through the face HGEF is \( 0 \).

Final Answer
The flux through the faces ABCD and HGEF are, respectively:
\( \frac{q}{24\epsilon_0}, 0 \)