Question

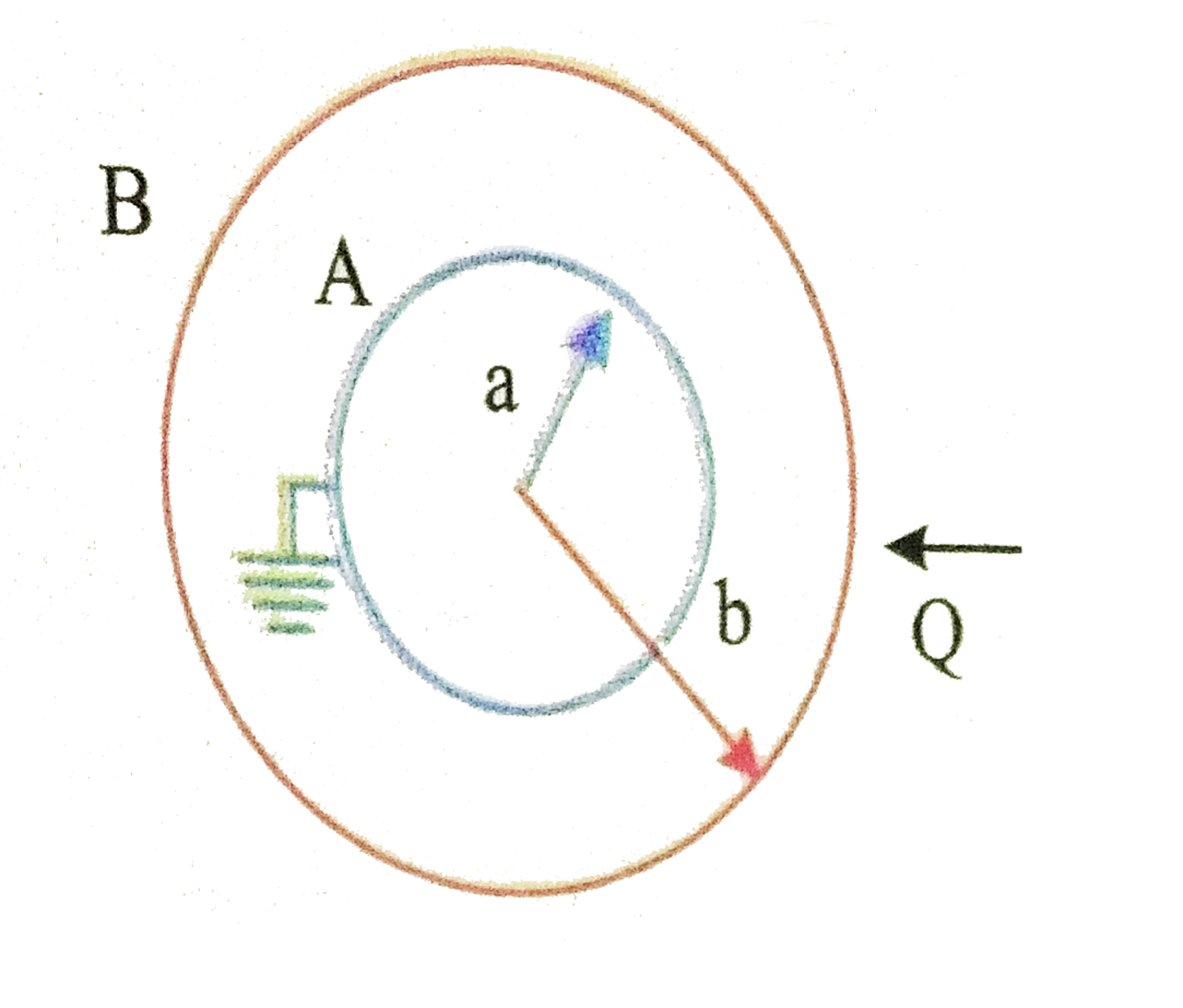
There are two concentric conducting shells. The potential of outer shell is 10 V and that of inner shell is 15 V. If the outer shell is grounded, the potential of iner shell becomes $/$ remains.

Options

A. 25 V
B. 15 V
C. 10 V
D. 5 V

Answer

D

Explanation

To solve the problem of two concentric conducting shells, we need to follow these steps:

Step 1: Understand the Initial Conditions
We have two concentric conducting shells:
- The potential of the outer shell (V_outer) is 10 V.
- The potential of the inner shell (V_inner) is 15 V.

Step 2: Grounding the Outer Shell
When the outer shell is grounded, its potential becomes 0 V. This means that the charge distribution on the outer shell will adjust to ensure that its potential is zero.

Step 3: Use the Formula for Potential
The potential (V) at a point due to a spherical shell of charge is given by:
\( V = \frac{kQ}{r} \)
where:
- \( k \) is Coulomb's constant,
- \( Q \) is the charge of the shell,
- \( r \) is the distance from the center to the point where the potential is being measured.

Step 4: Set Up the Equations
1. For the outer shell (before grounding):
\( V_{outer} = \frac{kQ_{outer}}{R} + \frac{kQ_{inner}}{r} = 10 \, \text{V} \)

2. For the inner shell:
\( V_{inner} = \frac{kQ_{outer}}{R} + \frac{kQ_{inner}}{r} = 15 \, \text{V} \)

Step 5: Subtract the Equations
By subtracting the equation for the outer shell from the equation for the inner shell, we can isolate the terms involving the charges:
\( \left(\frac{kQ_{inner}}{r} - \frac{kQ_{outer}}{R}\right) = 15 - 10 \)
This simplifies to:
\( \frac{kQ_{inner}}{r} - \frac{kQ_{outer}}{R} = 5 \tag{1} \)

Step 6: Grounding Effect on Outer Shell
When the outer shell is grounded, its potential becomes 0 V:
\( 0 = \frac{kQ_{outer}}{R} + \frac{kQ_{inner}}{r} \)
This can be rearranged to:
\( \frac{kQ_{outer}}{R} = -\frac{kQ_{inner}}{r} \tag{2} \)

Step 7: Substitute Equation (2) into Equation (1)
Substituting equation (2) into equation (1):
\( -\frac{kQ_{inner}}{r} - \frac{kQ_{inner}}{R} = 5 \)
Factoring out \( kQ_{inner} \):
\( kQ_{inner} \left(-\frac{1}{r} - \frac{1}{R}\right) = 5 \)

Step 8: Calculate the New Potential of Inner Shell
Now, we need to find the new potential of the inner shell after grounding:
\( V_{inner,new} = \frac{kQ_{outer}}{R} + \frac{kQ_{inner}}{r} \)
Using the grounded condition:
\( V_{inner,new} = -\frac{kQ_{inner}}{r} + \frac{kQ_{inner}}{r} \)
This results in:
\( V_{inner,new} = 5 \, \text{V} \)

Conclusion
Thus, when the outer shell is grounded, the potential of the inner shell becomes 5 V.